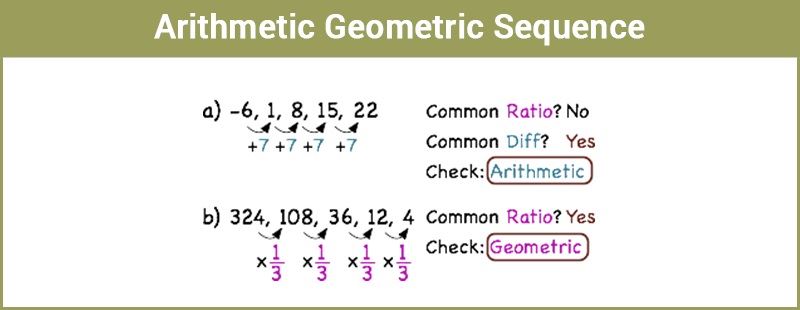
Arithmetic Geometric Sequence

Arithmetic - Geometric Sequence

Arithmetic Geometric sequence is the fusion of an arithmetic sequence and a geometric sequence. In this article, we are going to discuss the arithmetic-geometric sequences and the relationship between them. Also, get the brief notes on the geometric mean and arithmetic mean with more examples.

What is Arithmetic Sequence?

An arithmetic sequence is of the form:

\(\begin{array}{l}a, ~a+d,~ a+2d,~……~a~+~(n~-~2)d,~ a~+~(n~-~1)d\end{array} \)
;

where

\(\begin{array}{l}a\end{array} \)
is the first term,

\(\begin{array}{l}d\end{array} \)
is the common difference

\(\begin{array}{l}a~+~(n~-~1)d\end{array} \)
 is
\(\begin{array}{l}n^{th}\end{array} \)
 term of A.P.

What is a Geometric Sequence?

Similarly, a geometric sequence is of the form
\(\begin{array}{l}b,~ br, ~br^2~,~ ……~br^{n~-~2}, br^{n~-~1}\end{array} \)

here

\(\begin{array}{l}b\end{array} \)
is the first term,

\(\begin{array}{l}r\end{array} \)
is the common ratio

\(\begin{array}{l}br^{n~-~1}\end{array} \)
 is
\(\begin{array}{l}n^{th}\end{array} \)
 term of the G.P.

What is Arithmetic Geometric Sequence?

Let
\(\begin{array}{l}a_1,~ a_2, ~a_3,~ …….~a_n\end{array} \)
 be an A.P and
\(\begin{array}{l}b_1,~ b_2,~ b_3,~ …….~b_n\end{array} \)
 be a G.P
Then,
\(\begin{array}{l}a_1 ~b_1,~ a_2~ b_2,~ a_3~ b_3,~ ……, ~a_n~ b_n\end{array} \)
 is called as an arithmetic geometric sequence and it is of the form,
\(\begin{array}{l}ab,~ (a~+~d)br, ~(a~+~2d)br^2,~ …….,~[a~+~(n-2)d]~br^{n~-~2}, ~[a~+~(n~-~1)d]br^{n~-~1}\end{array} \)

Sum of n terms of the above sequence is found as follows:

\(\begin{array}{l}S_n =ab+ (a+d)br +(a+2d)br^2+…….+ [a+(n-2)d]br^{n-2}+ [a+(n-1)d]br^{n-1}\end{array} \)
—- (1)

Now, multiplying each term with

\(\begin{array}{l}r\end{array} \)
gives,

\(\begin{array}{l}rS_n = abr+(a+d)br^2+ (a+2d)br^3+….+[a+(n-2)d]br^{n-1}+[a+(n-1)d]br^n\end{array} \)
 ––(2)

Subtract equation (2) from equation (1), we get

\(\begin{array}{l}S_n~-~rS_n = ab~+~dbr~+~dbr^2~+~dbr^3~+~ ……~+ ~dbr^{n-1}~–~[a~+~(n~-~1)d]br^n\end{array} \)
 —(3)

In the above series, if we exclude the first term and the last term,

\(\begin{array}{l}dbr~+~dbr^2~+~ dbr^3~ +~ ……….~+ ~dbr^{n~-~1}\end{array} \)
 is a G.P
Sum of
\(\begin{array}{l}n\end{array} \)
 terms of G.P is a
\(\begin{array}{l}\frac{1~-~r^n}{1~-~r}\end{array} \)
,
Therefore,
\(\begin{array}{l}dbr~+~dbr^2~+~ dbr^3~ +~ ……….~+ ~dbr^{n~-~1}\end{array} \)
 =
\(\begin{array}{l}dbr\frac{1~-~r^{n~-~1}}{1~-~r}\end{array} \)

Now, (3) becomes as,

(1-r) Sn =
\(\begin{array}{l}ab ~+~ dbr\frac{1~-~r^{n~-~1}}{1~-~r}~-~[a~+~(n~-~1)d]br^n\end{array} \)
,

where

\(\begin{array}{l}r~≠~0\end{array} \)

Sn =
\(\begin{array}{l}\frac{ab}{1~-~r}~+~dbr\frac{1~-~r^{n~-~1}}{(1-r)^2}~ -~\frac{[a~+~(n-1)d]br^n}{1~-~r}\end{array} \)

If the common ratio of the sequence lies between -1 and 1, then

\(\begin{array}{l} \lim\limits_{n→∞}~r^n\end{array} \)
 =
\(\begin{array}{l}0\end{array} \)

Therefore, the sum of infinite terms of the sequence in (1) is,

S =
\(\begin{array}{l}\frac{ab}{1~-~r} ~+ ~\frac{dbr}{(1-r)^2}\end{array} \)
 [-1<r<1]

Geometric Mean

Consider two positive numbers a and b, the geometric mean of these two numbers is

\(\begin{array}{l}√ab\end{array} \)
.

For example; Geometric mean of 3 and 27 is √(3×27)=9

The numbers 3, 9, 27 is in a G.P with common ratio 3.

In general; between 2 positive numbers a and b, we can insert as many numbers as we like such that the resulting sequence forms a G.P.

Let G1, G2, G3……Gn be the n numbers inserted between the positive numbers a and b such that,

a,G1,G2,G3……Gn forms a G.P. “a” is the first term and b is (n+2)th term.

b = ar{n+1},

\(\begin{array}{l} r = \left(\frac{b}{a}\right)^{\frac{1}{n~+~1}}\end{array} \)

It gives, G1 = ar =
\(\begin{array}{l}a\left(\frac{b}{a}\right)^{\frac{1}{n~+~1}}\end{array} \)
G2 = ar2 =
\(\begin{array}{l}a\left(\frac{b}{a}\right)^{\frac{2}{n~+~1}}\end{array} \)
G2 = ar3 =
\(\begin{array}{l}a(\frac{b}{a})^{\frac{3}{n~+~1}}\end{array} \)
Gn = arn =
\(\begin{array}{l}a(\frac{b}{a})^{\frac{n}{n~+~1}}\end{array} \)

Relation Between Arithmetic Mean (A.M) and Geometric Mean (G.M)

Consider two positive numbers
\(\begin{array}{l}a\end{array} \)
 and
\(\begin{array}{l}b\end{array} \)
;
\(\begin{array}{l}A.M\end{array} \)
 =
\(\begin{array}{l}\frac{a~+~b}{2}\end{array} \)
\(\begin{array}{l}G.M\end{array} \)
 =
\(\begin{array}{l}√ab\end{array} \)
\(\begin{array}{l}A.M~-~G.M\end{array} \)
 =
\(\begin{array}{l}\frac{a~+~b}{2}~-~√ab\end{array} \)
 =
\(\begin{array}{l}\frac{a~+~b~-~2√ab}{2}\end{array} \)
\(\begin{array}{l}A.M~-~G.M\end{array} \)
 =
\(\begin{array}{l}\frac{(√a~-~√b)^2}{2}\end{array} \)
 which is greater than or equal to 0.

Therefore,

\(\begin{array}{l}A.M~≥~G.M\end{array} \)

Arithmetic Geometric Sequence Example

Example 1:

Insert 3 numbers between 4 and 64 so that the resulting sequence forms a G.P.

Solution:

Let G1,G2,G3 be the three numbers to be inserted between 4 and 64,

\(\begin{array}{l} r = (\frac{b}{a})^{\frac{1}{n~+~1}} = (\frac{64}{4})^{\frac{1}{3~+~1}} = (16)^{\frac{1}{4}} = 2\end{array} \)

G1 =

\(\begin{array}{l}4~×~2\end{array} \)
= 8

G2 =

\(\begin{array}{l}8~×~2\end{array} \)
= 16

G3 =

\(\begin{array}{l}16~×~2\end{array} \)
= 32

Example 2:

A.M and G.M of two numbers are 5 and 4 respectively. Find the two numbers.

Solution:

Let the numbers be
\(\begin{array}{l}a\end{array} \)
 and
\(\begin{array}{l}b\end{array} \)
,
\(\begin{array}{l}\frac{a~+~b}{2}\end{array} \)
 =
\(\begin{array}{l}5\end{array} \)
,
\(\begin{array}{l}a~+~b\end{array} \)
 =
\(\begin{array}{l}5~×~2\end{array} \)
 =
\(\begin{array}{l}10\end{array} \)
         —(1)
\(\begin{array}{l}√ab\end{array} \)
 =
\(\begin{array}{l}4\end{array} \)
,
\(\begin{array}{l}ab\end{array} \)
 =
\(\begin{array}{l}16\end{array} \)
\(\begin{array}{l}(a~+~b)^2~-~(a~-~b)^2\end{array} \)
 =
\(\begin{array}{l}4ab\end{array} \)
\(\begin{array}{l}{10}^2~-~(a~-~b)^2\end{array} \)
 =
\(\begin{array}{l}4~×~16\end{array} \)
 =
\(\begin{array}{l}64\end{array} \)
\(\begin{array}{l}(a~-~b)^2\end{array} \)
 =
\(\begin{array}{l}100~-~64\end{array} \)
 =
\(\begin{array}{l}36\end{array} \)
\(\begin{array}{l}a~-~b\end{array} \)
 =
\(\begin{array}{l}±6\end{array} \)
           —(2)

By solving (1) and (2),

\(\begin{array}{l}2a\end{array} \)
 =
\(\begin{array}{l}16\end{array} \)
,
\(\begin{array}{l}a\end{array} \)
 =
\(\begin{array}{l}\frac{16}{2}\end{array} \)
 =
\(\begin{array}{l}8\end{array} \)
\(\begin{array}{l}b\end{array} \)
 =
\(\begin{array}{l}10~-~a\end{array} \)
 =
\(\begin{array}{l}10~-~8\end{array} \)
 =
\(\begin{array}{l}2\end{array} \)

Thus, some examples of the arithmetic geometric progression are discussed above. To learn different kinds of progressions in Maths, download BYJU’S – The Learning App.

Test your Knowledge on Sequences
Quiz Image

Put your understanding of this concept to test by answering a few MCQs. Click ‘Start Quiz’ to begin!

Select the correct answer and click on the “Finish” button
Check your score and answers at the end of the quiz

Congrats!

Visit BYJU’S for all Maths related queries and study materials

Your result is as below

0 out of 0 arewrong
0 out of 0 are correct
0 out of 0 are Unattempted
MATHS Related Links
P Value Different Mathematical Symbols
Bar Chart Definition Level Of Significance
Dilation Curved Line
Sin 30 Degrees Composition Of Functions & Inverse Of A Function
Area Of Right Angled Triangle Formula Multiples - Common Multiples And Common Factors

Comments

Leave a Comment

Your Mobile number and Email id will not be published.

*

*

close
close

Play

&

Win