Quadrilaterals and Triangles: Angle sum property
A plane figure bounded by finite line segments to form a closed figure is defined as polygon.Based on the number of sides or vertices a polygon can be classified as n-sided polygon. A polygon bounded by three finite line segments is known as triangle. It is the smallest possible polygon. A four-sided polygon is known as a quadrilateral.
The sum of interior angles of any n-sided polygon is given by (n-2) × 180°. Thus, the sum of interior angles of a triangle is 180° and the sum of interior angles of a quadrilateral is 360°. Let us try to prove this mathematically.
Angle Sum Property of Triangles:
Consider a ∆ABC, as shown in the figure below.
Construction: Draw a line
Figure 1: Proof of angle sum property of triangles
Since PQ is a straight line, it can be concluded that:
∠PAB + ∠BAC + ∠QAC = 180° ….(1)
Since PQ||BC and AB, AC are transversals to the parallel lines,
Also, ∠QAC = ∠ACB and ∠PAB = ∠CBA (pair of alternate angles)
Substituting the value of ∠QAC and∠PAB in equation (1),
∠ACB + ∠BAC + ∠CBA = 180°
Thus, the sum of interior angles of a triangle is always 180°.
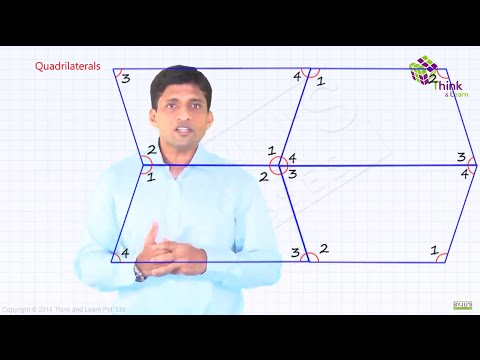
Angle Sum Property of Quadrilaterals:
Consider a quadrilateral ABCD
Figure 2: Proof of angle sum property of quadrilaterals
Constructions: Draw diagonal BD as shown below:
Figure 2: Proof of angle sum property of quadrilaterals
Figure 3
From figure 3;
∠B = ∠3 + ∠4 …..(1)
∠D = ∠1 + ∠2 …..(2)
In ∆ABD,
∠A + ∠1 + ∠3 = 180° …………………………(3) (Angle Sum Property of triangles)
Similarly, in ∆BCD,
∠C + ∠2 + ∠4 = 180°………………………….(4) (Angle Sum Property of triangles)
Adding equation (3) and (4);
⇒ ∠A + ∠1 + ∠3 + ∠C + ∠2 + ∠4 = 360°
Substituting ∠B and ∠DFrom equation (1) and (2) respectively, we have:
∠A + ∠B + ∠C + ∠D = 360°
Thus, it can be seen that the sum of interior angles in a quadrilateral is always 360°.
According to exterior angle property of a polygon, sum of exterior angles of a polygon is always 360° and hencethe sum of exterior angles of atriangle and a quadrilateral is 360°.
Also, quadrilateralABCD is divided into two triangles by diagonalBD, ∆ABD and ∆BCD. The measure of sum of interior angles of ABCD is twice the sum of interior angles of a triangle i.e. 2 × 180°.’
Comments