Potential Energy Curve

Introduction to Potential Energy Curve

Potential energy curves govern the characteristics of materials. The composition, bonding, crystal structure, mechanical processing, and microstructure of every material influence its potential energy curve, and as a result, its attributes. A critical examination of the potential energy curve aids in a better understanding of the material’s properties.

The structure and material properties are controlled by the kind, strength, and directionality, such as melting temperature, thermal expansion, elastic stiffness, electrical properties, ductility, and toughness.

Table of Contents

• Potential Energy Surfaces
• Potential Energy Curves (1-D Potential Energy Surfaces)
• Analysis of Potential Energy Curves
• Conclusions
• Frequently Asked Questions – FAQs

Potential Energy Surfaces

A potential energy surface (PES) expresses the potential energy, particularly a collection of atoms, in terms of a set of characteristics, most commonly the atoms’ locations. The surface may specify energy as a function of one or more coordinates; if only one coordinate is present, the surface is referred to as a potential energy curve or energy profile. The landscape analogy is valid: the energy value is a function of two bond lengths for a system with two degrees of freedom (e.g. two bond lengths).

According to the Potential Energy Surface, each geometry (both external and internal) of the atoms of the molecules in a chemical reaction is connected with a unique potential energy. This results in a smooth energy “landscape,” and chemistry can be examined through the lens of topology (of particles evolving over “valleys” “and passes”).

Potential Energy Curves (1-D Potential Energy Surfaces)

The PES is a molecule’s energy as a function of its nuclei’s locations, r. The energy of a system of two atoms is proportional to their separation. The energy is 0 at long distances, implying “no contact.” Attractive forces prevail at distances of many atomic diameters, whereas, at extremely near distances, repulsive forces prevail, causing the energy to grow. The attractive and repulsive effects are balanced at the curve’s minimum point. Plots that depict this relationship can help define specific features of a chemical bond.

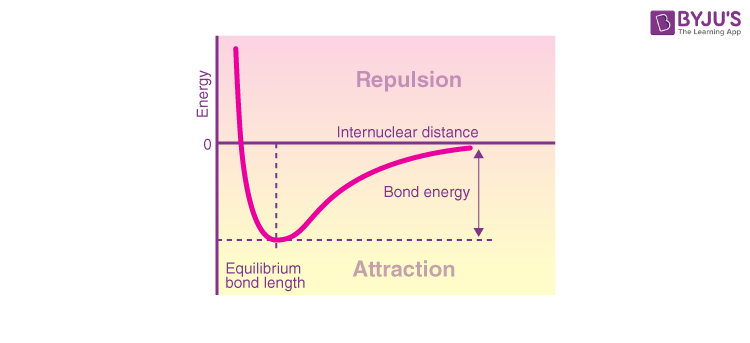
The bond length is determined by the internuclear distance at which the potential energy minimum occurs. Because thermal motion causes the two atoms to bounce about this distance, this is more accurately known as the equilibrium bond length. In general, the shorter the bond, the stronger the bond.

Analysis of Potential Energy Curves

Crystal Structure Packing and Its Effect on Bonding Energies

Various atoms organise themselves in different crystalline formations depending on their nature. The bonding energy, the shape, and the depth of the potential well are all determined by the order in which atoms associate with their neighbours.

The relevant potential energy curves for random and dense ordered packing of atoms are illustrated below. Figure (a) Random atom packing and associated potential energy curve; (b) Dense ordered atom packing and associated potential energy curve.

Mechanical Properties

Mechanical characteristics define how materials deform (elongate, compress, twist) or break due to applied stress, time, temperature, and other factors.

Thermal Properties

Heat capacity, thermal expansion, and thermal conductivity are used to describe a material’s response to heat. The principle mode of thermal energy assimilation in solids is through an increase in atom vibration energy. Because of atomic bonding, nearby atoms’ vibrations are connected, resulting in phonons, which are lattice waves that transport energy through the material.

Electrical Properties

Electrical conductivity and resistivity are material properties that are not affected by geometry. The electrical conductivity of solid materials varies greatly. The quantity of electrons available to participate in the conduction process determines the level of electrical conductivity.

As the number of electrons grouped increases, the energy levels broaden into bands, as shown in the diagram.

Conclusions

A PES is a conceptual tool that can be used to help with molecular geometry and chemical reaction kinetics analysis. From material to material, the magnitude of bonding energy and the form of the potential energy curve differ. Significant bond energy, high melting temperature, large elastic modulus, and a small coefficient of thermal expansion are all indicators of a deep and narrow trough in the curve.

The well’s diameter and asymmetry in the potential energy curve show diverse materials’ qualities.

Metal ductility is inextricably linked to the properties of the metallic bond. Metals have varied bond energy, a high melting temperature, a high elastic modulus, and moderate thermal expansion in their potential energy curves. The low melting temperature, low elastic modulus, and significant linear expansion coefficient characterise polymers’ potential curve(s).

Many materials have multiple types of bonding, such as ionic and covalent bonding in ceramics, covalent and secondary bonding in polymers, and covalent and ionic bonding in semi-conductors. These must be taken into account when obtaining attributes from potential energy curves.