How to Find Moment of Inertia of i Section

Moment of Inertia is the quantity that expresses an object’s resistance to change its angular acceleration. It is a measure of rotational inertia of the body. To calculate the moment of inertia of i section the value of the centre of mass of the i section should be known. The value of the area, the moment of inertia of individual segments and the vertical distance from the centroid of the segment can be calculated using the data given in the problem.

How to Find Moment of Inertia of “I” Section

The following steps should be followed to find the moment of inertia of the I section.

Step 1: The beam sections should be segmented into parts

The I beam section should be divided into smaller sections. The moment of inertia must be calculated for the smaller segments. Here, the section is divided into three rectangular segments.

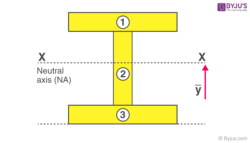
Moment of Inertia of i section 1

Step 2: Mark the neutral axis

The neutral axis is the horizontal line passing through the centre of mass. The centre of mass is calculated using the following formula

\(\begin{array}{l}\bar{y}= \frac{\sum Y_i A_i}{\sum A_i}\end{array} \)

Y_iis the centre of mass of the individual rectangle

A_iis the area of the individual rectangle

Step 3: Calculating the Moment of Inertia

Using the parallel axis theorem the total momentum is calculated as

\(\begin{array}{l}I_{total}=\sum (\bar{I_{i}}+A_{i}d_{i}^{2})\end{array} \)

where,

\(\begin{array}{l}(\bar{I_{i}})\ \text{is the inertia of the individual segment about its own centroid axis.}\end{array} \)

A_iis the individual segment.

d_i is the vertical distance from the centroid of the segment.

The moment of inertia of each of the rectangular parts must be calculated. The moment of inertia equation of a rectangle about its centroid axis is

\(\begin{array}{l}\bar{I} = \frac{1}{12}bh^{3}\end{array} \)

Where,

b is the base of the rectangle.

h is the height of the rectangle.

Example

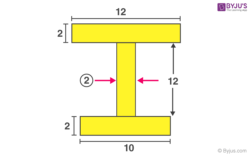
Moment of inertia of i section 2

Let us understand the above equation with an example

Finding the centre of mass of the I section

\(\begin{array}{l}\bar{y}= \frac{\sum Y_i A_i}{\sum A_i}\end{array} \)

={[(2 + 12 + (2/2))(12 x 2)] + [2+ (12/2)(12 x2)]} + [(2/2)(10 x2)/ {20 + 24 x+24}

= {15(24) + 8(24) + 1(20)}/(20 + 24 +24)

= 360 + 192 + 20/(20 + 24 + 24)

= 572/68

= 8.4

Centre of mass of the I section is given at 4 mm

Segment 1: Upper rectangle

\(\begin{array}{l}(\bar{I_{1}}) = \frac{1}{12}\times 12\times 2^3\end{array} \)

= 8

A₁ = (12 x 2) = 24

\(\begin{array}{l}d_{1}= \left | y_{1}-\bar{y} \right |\end{array} \)

= |(2+12+{2/2}) – 8.4 | = 6.6

\(\begin{array}{l}(\bar{I_{1}})+ A_1d_1^2\end{array} \)

= (8 + 24(6.6)²) = (8 + 1045) = 1053

Segment 2: Middle rectangle

\(\begin{array}{l}(\bar{I_{2}})= \frac{1}{12}\times 12\times 2^3\end{array} \)

= 8

A₂ = (12 x 2) = 24

\(\begin{array}{l}d_{2} = \left | y_{2}-\bar{y} \right |\end{array} \)

= |(2+ (12/2) – 8.4|= (8 – 8.4) = 0.4

\(\begin{array}{l}(\bar{I_{2}})+ A_2d_2^2\end{array} \)

= (8 + 24(0.4)²) =11.84

Segment 3: Lower rectangle

\(\begin{array}{l}(\bar{I_{3}})= \frac{1}{12}\times 10\times 2^3\end{array} \)

= 6.66

A₂ = (10 x 2) = 20

\(\begin{array}{l}d_{3} = \left | y_{3}-\bar{y} \right |\end{array} \)

= |((2/2)- 8.4)|= 7.4

\(\begin{array}{l}(\bar{I_{3}})+A_1d_1^2\end{array} \)

= (6.66 + (20)(7.4)²)= 1101.9

\(\begin{array}{l}I_{total}=\sum (\bar{I_{i}}+A_{i}d_{i}^{2})\end{array} \)

\(\begin{array}{l}=(\bar{I_{1}})+A_1d_1^2 + (\bar{I_{2}}) + A_1d_2^2 + (\bar{I_{3}}) + A_1d_1^2\end{array} \)

=1053 + 11.84

+ 1101.9 = 2166 mm⁴

Moment of inertia of T section, I_total = 2166 mm⁴

Parallel Axis Theorem

Comments

Leave a Comment Cancel reply