Thermodynamics IIT JEE Study Material

Thermodynamics deals with the interaction of one body with another in terms of quantities of heat and work. In thermodynamics, the system is defined as a quantity of matter or a region in space under investigation. Everything external to the system is the environment or surroundings. The imaginary or actual surface separating the system from its surroundings is known as the boundary. It may be either fixed or movable.

Closed System (m = constant)

When there is an exchange of energy (work and heat) but no matter between the system and its surrounding, the system is known as a control mass or closed system. For example, a greenhouse is a closed system that exchanges heat but no work with its surroundings. Depending on the properties of its boundary, a system exchanges work, heat or both. An adiabatic boundary does not allow the exchange of heat from the system to surroundings, and a rigid body does not allow the exchange of work between the system and surroundings.

Isolated Systems

An isolated system does not allow the exchange of heat, work or matter between the system and surroundings. For example, an insulated container, such as an insulated gas cylinder.

i.e., m_in=m_out=E_in=E_out=0

Open System

In an open or control volume system, there is an exchange of energy, i.e., heat, work and matter, between the system and surroundings. A boundary that allows the exchange of matter is known as the permeable boundary. For example, the ocean, turbine, water heater, car radiator, nozzle, etc. To analyse an open system, the energy of the system is always taken equal to the energy leaving the system.

Other Types of Systems

Rigid system: It is a type of closed system in which the system interacts with the surroundings by exchanging heat only.

Adiabatic system: It is a closed or open system in which energy is transferred to its surroundings only as work (without transfer of heat or matter).

Properties of a System

Intensive properties: Intensive properties are independent of the size of a system. For example, density, molar volume, surface tension, refractive index, viscosity, specific heat, temperature, free energy per mole, pressure, etc. They are not additive in nature.

Extensive properties: These are properties that are dependent on the size of the system. For example, the number of moles, volume, mass, entropy, free energy, enthalpy, heat capacity, internal energy, etc. They are additive in nature. The specific properties are per unit mass extensive properties. For example, specific mass, specific energy, specific volume, etc.

Thermodynamic Process

The thermodynamic process deals with the change of a system’s state from one state to another. The different types of thermodynamic processes are isothermal, adiabatic, isobaric and isochoric.

In an isothermal process, the temperature of the system remains constant [dE and dT = 0] during the state change. This process is carried out in a thermostat, and the energy exchange takes place without any change in temperature. In an adiabatic process, the exchange of energy between the system and surroundings does not occur [dQ = 0]. It is carried out in a perfectly insulated container. The temperature of the system might change during an adiabatic process. In an isobaric process, the pressure of the system remains constant [dP = 0]. In an isochoric process, the volume of the system remains constant [dV = 0].

Cyclic Thermodynamic Process [ΔH = 0 and ΔE = 0]

1. In a cyclic process, a system returns to its initial state after undergoing a number of different thermodynamic processes.

2. A process that is carried out on its own without any external help is known as a spontaneous process.

3. In the quasi-static or reversible process, the system and its surroundings can be returned from its final state to its initial state without any changes in the thermodynamic properties of the universe. The irreversible processes are also known as the natural processes because all the processes that occur in nature are irreversible processes. It occurs due to the finite gradient between the two states of the system.

Zeroth Law of Thermodynamics

According to the zeroth law of thermodynamics, if two thermodynamic systems are in thermal equilibrium with the 3rd system, then all three systems are in thermal equilibrium with each other.

First Law of Thermodynamics

ΔE = Q + W

Where,

ΔE = Change in the internal energy of the system

Q = Heat transfer (to or from the system)

W = Work done (either by the system or on the system)

If a system expands at a constant pressure P, from volume V₁ to V₂, then the 1st law equation can be rewritten as

ΔE = q – P ΔV [ΔV= V₂ -V₁]

Work Done in Different Thermodynamics Processes

In an irreversible process, work done 

In an isothermal reversible process, work done 

Also,

In an adiabatic reversible process, work done

Where, γ = Poisson’s ratio

Entropy (S)

Entropy changes with the change in the state of a system. The entropy change is given by

Where q_rev = Heat supplied reversibly and isothermally

Entropy is maximum when the system is in equilibrium, i.e.,

Second Law of Thermodynamics

The second law of thermodynamics states that the state of the entropy of the entire universe is always increasing with time. Also, the changes in the entropy in the universe can never be -ve.

Carnot Cycle

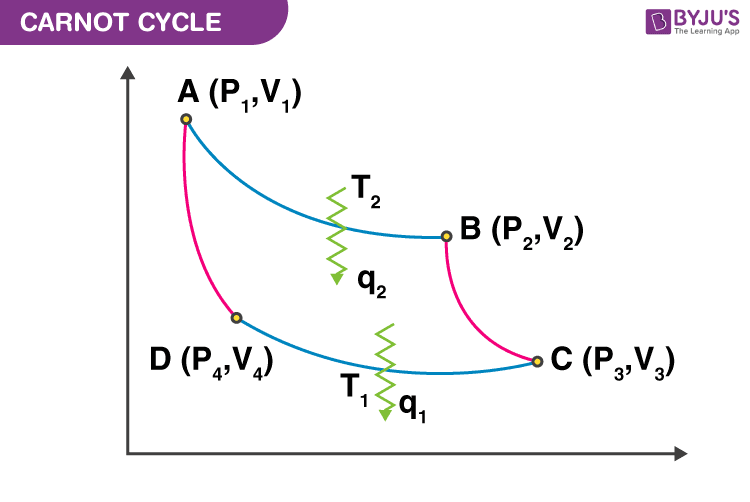
1. A → B is an isothermal reversible expansion process.

2. B → C is an adiabatic reversible expansion process.

3. C → D is an isothermal reversible compression process.

4. D → A is an adiabatic reversible compression process.

Now, the work done in different thermodynamic processes are

• \(\begin{array}{l}\mathbf{W_{AB}\;=\;-\;n\;R\;T_{2}\;ln\;\frac{V_{2}}{V_{1}}}\end{array} \)
• \(\begin{array}{l}\mathbf{W_{BC}\;=\;C_{v}\;(T_{1}\;-\;T_{2})}\end{array} \)
• \(\begin{array}{l}\mathbf{W_{CD}\;=\;-\;n\;R\;T_{1}\;ln\;\frac{V_{4}}{V_{3}}}\end{array} \)
• \(\begin{array}{l}\mathbf{W_{DA}\;=\;C_{v}\;(T_{2}\;-\;T_{1})}\end{array} \)

Carnot Efficiency

Entropy Change

1. Reversible irreversible isothermal contraction and expansion (ideal gas)

2. Isobaric heating or cooling

3. Isochoric heating or cooling

4. Adiabatic process

5. For irreversible process

6. For reversible adiabatic compression and expansion

Third Law of Thermodynamics

Statement: At an absolute 0 temperature, the entropy of a perfectly crystalline substance is 0.

By utilising the third law of thermodynamics, the absolute value of entropy can be evaluated at room temperature (for pure substances). The standard absolute entropy is the entropy of a substance in its standard temperature and pressure, i.e., at 1 atm and 298 K.

The absolute entropies can be utilised in calculating the changes in entropy by using the relation:

Gibbs Free Energy

G = H – TS and at constant pressure and temperature

1. For reversible process:

2. For spontaneous process:

3. For equilibrium:

Thermodynamics Important Topics

Thermodynamics Important Questions

Thermodynamics Top 7 Questions

Thermodynamics JEE Solutions

Thermodynamics JEE Main Important Questions

Top 15 Most Important and Expected JEE Questions of Thermodynamics

Thermodynamics – Most Important JEE Advanced Questions