In analytic geometry, an asymptote of a curve is a line such that the distance between the curve and the line approaches zero as they tend to infinity. In some contexts, such as algebraic geometry, an asymptote is defined as a line which is tangent to a curve at infinity. There are two types of asymptote: one is horizontal and other is vertical. Also, a special type of asymptote exists that is an oblique asymptote. Below mentioned are the asymptote formulas.
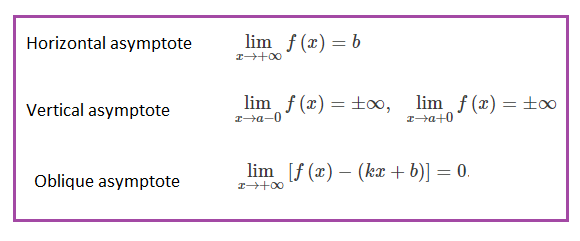
This can be shown graphically as:

Solved Example
Question 1: Find the asymptotes for
\(\begin{array}{l}f(x) = \frac{2x^{2} + 2x}{x^{2}+1}\end{array} \)
Solution:
We can see at once that there are no vertical asymptotes as the denominator can never be zero.
\(\begin{array}{l}x^{2}\end{array} \)
+ 1 = 0\(\begin{array}{l}x^{2}\end{array} \)
= –1 has no real solution.Thus, this refers to the vertical asymptotes.
Now see what happens as x gets infinitely large:\[\lim_{x\rightarrow\infty}\;\frac{2x^{2}+2x}{x^{2}+1}\]
The method we have used before to solve this type of problem is to divide through by the highest power of x.
=
\(\begin{array}{l}\LARGE\lim_{x\rightarrow\infty}\;\frac{2x^{2}+2x}{x^{2}+1}\end{array} \)
=
\(\begin{array}{l}\LARGE \frac{\frac{2x^{2}}{x^{2}} + \frac{2x}{x^{2}}}{\frac{x^{2}}{x^{2}} + \frac{1}{x^{2}}}\end{array} \)
=
\(\begin{array}{l}\LARGE\frac{2+0}{1+0}\end{array} \)
=
\(\begin{array}{l}\LARGE2\end{array} \)
This is the horizontal asymptotes of the give function.
| More topics in Asymptote Formula | |
| Slant Asymptote Formula | |
Comments