Velocity of a Wave on a String

If a string is stretched between two horizontal poles and plucked, a simple wave is created in the string. As this wave moves through the string, that portion of the string will acquire elastic energy and kinetic energy. In general, a wave in a string can be defined as the disturbance that travels through the string and transports energy and momentum. The waves in a string are transverse waves.

Wave Velocity in String

The velocity of the wave in a string depends on the characteristics of the medium. For example, if a thick rope and a thin guitar string are vibrated, the waves will move faster in the guitar string. Therefore, the velocity of the string depends on the linear densities of the two strings. Linear density is the mass per unit length.

Linear density μ = mass of string/length of string = m/l

The wave velocity of the string also depends on the tension of the strings. Let us consider the example of guitar strings. The tension of the guitar string can be adjusted by turning the spindles around which the strings are wrapped. If the tension on one of the strings is increased and the other string is kept loose, and if both the strings are plucked, we can notice that the waves move faster in the stretched string in which the tension is more.

Therefore, the wave velocity is given by

Derivation of Velocity of Wave

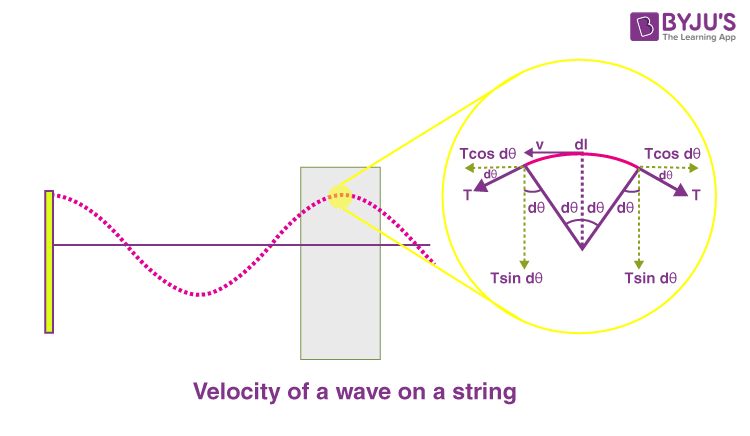
To understand how the speed of the wave on the string will depend on the linear mass density and the tension, a single symmetrical pulse is considered instead of the sinusoidal wave. For convenience, we will choose a frame of reference in which the pulse will remain stationary. That is, we can consider a boy skating along with the pulse, keeping it constantly in view. In this frame, the string appears to move past the boy from right to left.

A small element of length dl is considered within the pulse. This element will form the arc of the circle, which has the radius R and subtends an angle of 2dθ at the centre of the circle. Each end of the string is pulled tangentially by a force equal to the tension in the string. The horizontal component of the tension Tcosdθ cancels out. The vertical component Tsindθ adds to form the restoring force.

Restoring Force, F = 2(Tsindθ) = T(2dθ) = Tdl/R ———-(1)

For small angles dθ, we can approximate sin dθ = θ. From the figure, we know that 2dθ is equal to dl/R.

The linear mass density of the element, μ = mass/length= dm/dl

⇒ Mass, dm = μdl ———–(2)

The element dl is in the arc of the circle. Thus, it will have a centripetal acceleration towards the centre of the circle.

Acceleration, a = v²/R ————-(3)

From Newton’s Second Law, we know

Force = Mass x Acceleration

From equations (1), (2), and (3), we have

Tdl/R = ( μdl) x (v²/R)

Solving the above equation, we have

Solved Example

1. Let us consider a six-string guitar; the linear density of the high E string is 3.09 x 10^-4 kg/m. The linear density of the low E string is 5.78 x 10^-3 kg/m.

(a) If the high E string is plucked to produce a wave to produce a tension of 56.40 N. Then what will the velocity of the wave in the string be?

(b) The low E string has a linear mass density of almost 20 times greater than the high E string. For the velocity of the wave to be the same in both strings, should the tension of the low E string be smaller or greater than the high E string?

(c) Calculate the tension in the low E string for the same wave speed.

Solution

(a) The velocity of the wave,

(b) From the equation of velocity of the wave,

(c) Since the value of linear mass density and wave speed is known. The tension in the low E string can be determined using the formula.

T = μv² = (5.78 x 10^-3 kg/m)(427.23 m/s)²

= 1055 N

Wave on String Rapid Revision

The Velocity of Wave on String