Algebraic Identities

The algebraic equations which are valid for all values of variables in them are called algebraic identities. They are also used for the factorization of polynomials. In this way, algebraic identities are used in the computation of algebraic expressions and solving different polynomials. You have already learned about a few of them in the junior grades. In this article, we will recall them and introduce you to some more standard algebraic identities, along with examples.

Standard Algebraic Identities List

All the standard Algebraic Identities are derived from the Binomial Theorem, which is given as:

Some Standard Algebraic Identities list are given below:

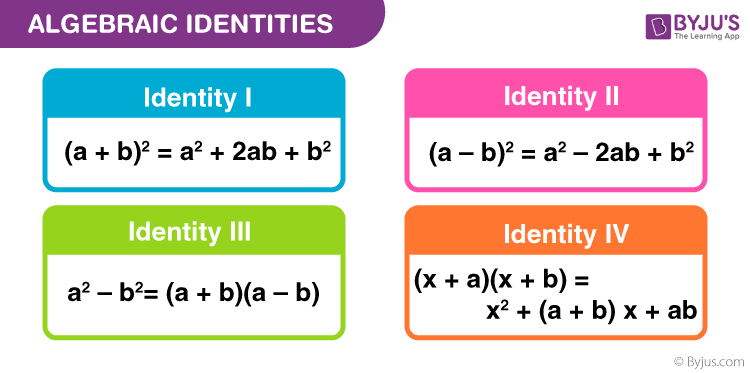
Identity I: (a + b)² = a² + 2ab + b²

Identity II: (a – b)² = a² – 2ab + b²

Identity III: a² – b²= (a + b)(a – b)

Identity IV: (x + a)(x + b) = x² + (a + b) x + ab

Identity V: (a + b + c)² = a² + b² + c² + 2ab + 2bc + 2ca

Identity VI: (a + b)³ = a³ + b³ + 3ab (a + b)

Identity VII: (a – b)³ = a³ – b³ – 3ab (a – b)

Identity VIII: a³ + b³ + c³ – 3abc = (a + b + c)(a² + b² + c² – ab – bc – ca)

Solved Examples of Algebraic Identities

Example 1:

Find the product of (x + 1)(x + 1) using standard algebraic identities.

Solution: 

(x + 1)(x + 1) can be written as (x + 1)². Thus, it is of the form Identity I where a = x and b = 1. So we have,

(x + 1)² = (x)² + 2(x)(1) + (1)² = x² + 2x + 1

Example 2:

Factorise (x⁴ – 1) using standard algebraic identities.

Solution: 

(x⁴ – 1) is of the form Identity III where a = x² and b = 1. So we have,

(x⁴ – 1) = ((x²)²– 1²) = (x² + 1)(x² – 1)

The factor (x² – 1) can be further factorised using the same Identity III where a = x and b = 1. So,

(x⁴ – 1) = (x² + 1)((x)² –(1)²) = (x² + 1)(x + 1)(x – 1)

Eample 3:

Factorise 16x² + 4y² + 9z² – 16xy + 12yz – 24zx using standard algebraic identities.

Solution:

16x² + 4y² + 9z²– 16xy + 12yz – 24zx is of the form Identity V. So we have,

16x² + 4y² + 9z² – 16xy + 12yz – 24zx = (4x)² + (-2y)² + (-3z)² + 2(4x)(-2y) + 2(-2y)(-3z) + 2(-3z)(4x)= (4x – 2y – 3z)² = (4x – 2y – 3z)(4x – 2y – 3z)

Example 4:

Expand (3x – 4y)³ using standard algebraic identities.

Solution:

(3x– 4y)³ is of the form Identity VII where a = 3x and b = 4y. So we have,

(3x – 4y)³ = (3x)³ – (4y)³– 3(3x)(4y)(3x – 4y) = 27x³ – 64y³ – 108x²y + 144xy²

Example 5:

Factorize (x³ + 8y³ + 27z³ – 18xyz) using standard algebraic identities.

Solution:

(x³ + 8y³ + 27z³ – 18xyz)is of the form Identity VIII where a = x, b = 2y and c = 3z. So we have,

(x³ + 8y³ + 27z³ – 18xyz) = (x)³ + (2y)³ + (3z)³ – 3(x)(2y)(3z)= (x + 2y + 3z)(x² + 4y² + 9z² – 2xy – 6yz – 3zx)

To learn more about algebraic identities, download BYJU’S The Learning App.