Moment Of Inertia Of Flywheel

Moment of inertia of a flywheel is calculated using the given formula;

Where I = moment of inertia of the flywheel.Here, the symbols denote;

m = rings’ mass.

N = flywheel rotation.

n = number of windings of the string.

h = height of the weight assembly.

g = acceleration due to gravity.

r = radius of the axle.

Or, we can also use the following expression;

Flywheels are nothing but circular disc-shaped objects which are mainly used to store energy in machines.

Determining The Moment Of Inertia Of Flywheel

To determine the moment of inertia of a flywheel we will have to consider a few important factors. First, we have to set up a flywheel along with apparatus like a weight hanger, slotted weights, metre scale and we can even keep a stopwatch.

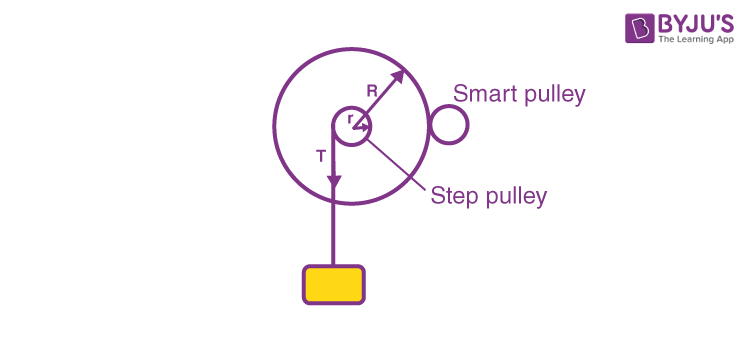
Then we make some assumptions. We will take the mass as (m) for the weight hanger as well as the hanging ring. The height will be (h). Now we consider an instance where the mass will descend to a new height. There will be some loss in potential energy and for which we write the equation as;

P_loss = mgh

Meanwhile, there is a gain in kinetic energy when the flywheel and axle are rotating. We express it as;

K_flywheel = (½) Iω²

I = moment of inertia

ω = angular velocity

Similarly, the kinetic energy for descending weight assembly is expressed as;

K_weight = (½) Iv²

Here, v = veocity

We also have to take into account the work that is done in overcoming the friction. This can be found out by;

W_friction = nW_f

In this case,

n = number of windings of the string

W_f = work done in overcoming frictional torque

If we state the law of conservation of energy then we obtain;

P_loss = K_flywheel + K_weight + W_friction

We will substitute the values and the equation will now become;

mgh = (½)Iω² + (½) mv² + nW_f

Moving on to the next phase, we look at the flywheel assembly’s kinetic energy that is used in rotating (N) number of times against the frictional torque. We get;

NW_f = (½ ) Iω² and W_f = (1 / 2N) Iω²

Further, we establish a relation between the velocity (v) of the weight assembly and the radius (r) of the axle. The equation is given as;

v = ωr

We have to substitute the values for W_f and v.

mgh = (½) Iω² + (½ )mr²ω² + (n / N) x ½ Iω²

If we solve the equation for finding the moment of inertia, we obtain;

⇒ Check Other Object’s Moment of Inertia:

• Moment Of Inertia Of Circle
• Moment Of Inertia Of A Quarter Circle
• Moment Of Inertia Of Semicircle
• Moment Of Inertia Of A Sphere
• Moment Of Inertia Of A Disc

Parallel Axis Theorem