Square Root of 35

Square root of 35 is equal to 5.9160797831…, i.e. √35 = 5.9160797831… Let’s understand the meaning of square root and the methods used to evaluate the square root of 35. When we multiply the value of the square root of a number by itself, it produces the original number. Therefore, the square root is the inverse or reverse process of squaring a number. If √35 = p, then p² = 35. The square root of 35 is denoted by √35, where ‘√’ is the radical symbol and 35 is the radicand.

Square Root of 35 Rational or Irrational

As mentioned above, the square root of 35 will not be a whole number and hence, the number 35 is not a perfect square. Also, the decimal expansion of the square root of 35 is non-repeating and non terminating, so it is an irrational number.

Facts about the Square Root of 35:

How to Find the Square Root of 35?

We can find the square root of any given number in three different ways. The methods of finding the square root of a number are as follows:

• Repeated subtraction method
• Prime factorisation method
• Long division method

Let’s learn all these methods of finding the square root of 35 step by step here.

Square Root of 35 by Repeated Subtraction

In this method, we should subtract the successive odd numbers, i.e. 1, 3, 5, 7,… till we obtain zero, starting from 35 and 1. The number of odd numbers we subtract in this process is treated as the square root of 35.

• 35 – 1 = 34
• 34 – 3 = 31
• 31 – 5 = 26
• 26 – 7 = 19
• 19 – 9 = 10
• 10 – 11 = -1

A negative value cannot be the square root of a number; also, we didn’t get 0 in the above subtraction but almost reached there. Thus, from the above process, we can say that the square root of 35 lies between 5 and 6, as well as it is nearer to 5. However, it is to be noted that the square root of non-perfect square numbers cannot be evaluated using the repeated subtraction method.

Click here to learn more about the square root of a number by the repeated subtraction method.

Square Root of 35 by Prime Factorisation

First, we need to write the prime factorisation of 35.

Prime factorisation of 35 = 5 × 7

In the process of finding the square root, we generally group the same factors in pairs.

In this case, there are no such similar pairs.

Thus, we cannot determine the exact value of √35 using prime factorisation.

Let’s use the long division method to find the value of √35.

Square Root of 35 by Division Method

Finding the square root of 35 by the long division method is one of the most important techniques in Maths. Go through the steps given below to learn the method of evaluating the square root of 35 using division.

Step 1: We know that 35 is not a perfect square; we need to put the decimal point and write pairs of 0s.

Step 2: Find the largest number whose square is less than or equal to the number in the leftmost group, i.e. 35. We know that 5² < 35 < 6², so we can consider 5. Take this number as the divisor and the quotient with the number in the leftmost group as the dividend (35). By dividing the number, we get 10 as the remainder.

Step 3: Now, bring down 00 and add the divisor with the quotient and enter it with a blank on its right. Now, we need to identify the largest possible digit, which will also become the new digit in the quotient, so that when the new divisor is multiplied by the new quotient, the product is less than or equal to the dividend. In this case, 109 × 9 = 981, so we choose the new digit as 9. Then, perform the division and get the remainder.

Step 4: Again, bring down the next pair 00 and add the divisor with the quotient and enter it with a blank on its right as we did in the previous step. We can repeat this process as many times as required.

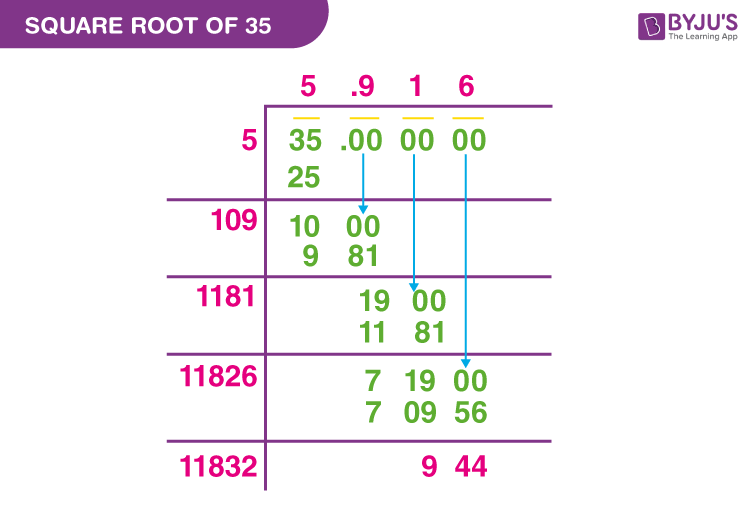
Therefore, the square root of 35 is equal to 5.916…

Square Root of Numbers

Square root of 2 = 1.4142…

Square root of 5 = 2.2361…

Square root of 8 = 2.8284…

Square root of 10 = 3.1623…

Square root of 11 = 3.3166…

Video Lessons on Square Roots

Visualising square roots

Finding Square roots

Solved Example

Question: Evaluate the value of 5 + √35.

Solution:

We know that the square root of 35 is equal to 5.916 (rounded to the nearest hundred)

Now, 5 + √35

= 5 + 5.916 {since √35 = 5.916}

= 10.916

Therefore, 5 + √35 = 5.916.