NCERT Solutions for Class 9 Maths Chapter 12 - Heron's Formula Exercise 12.2

*According to the CBSE Syllabus 2023-24, this chapter has been renumbered as Chapter 10.

The best way to understand Heron’s Formula with ease is through NCERT Solutions for Class 9 Maths Chapter 12 – Heron’s Formula Exercise 12.2 and 12.1. These exercises are designed to test your conceptual grasp of the subject.

From an educational point of view, this elementary concept is significant in various fields of study. Therefore, it is essential to have a good grasp of the concept. Another key point is that these solutions are designed by highly competent teachers with many years of relevant experience.

Therefore, NCERT Solutions is one of the best guides for studies. Important topics are presented in an easy-to-understand format. Moreover, the use of complex jargon is barred throughout the content. Furthermore, content is updated as per the prescribed CBSE syllabus.

Download PDF of NCERT Solutions for Class 9 Maths Chapter 12 – Heron’s Formula Exercise 12.2

Download PDF

carouselExampleControls111

Previous



Next

Access other exercise solutions of Class 9 Maths Chapter 12 – Heron’s Formula

Exercise 12.1 Solutions – 6 Questions

Access Answers of Maths NCERT Class 9 Chapter 12 – Heron’s Formula Exercise 12.2

1. A park, in the shape of a quadrilateral ABCD, has C = 90°, AB = 9 m, BC = 12 m, CD = 5 m and AD = 8 m. How much area does it occupy?

Solution:

First, construct a quadrilateral ABCD and join BD.

We know that

C = 90°, AB = 9 m, BC = 12 m, CD = 5 m and AD = 8 m

The diagram is:

Now, apply Pythagoras theorem in ΔBCD

BD² = BC² +CD²

⇒ BD² = 12²+5²

⇒ BD² = 169

⇒ BD = 13 m

Now, the area of ΔBCD = (½ ×12×5) = 30 m²

The semi perimeter of ΔABD

(s) = (perimeter/2)

= (8+9+13)/2 m

= 30/2 m = 15 m

Using Heron’s formula,

Area of ΔABD

= 6√35 m² = 35.5 m² (approximately)

∴ The area of quadrilateral ABCD = Area of ΔBCD+Area of ΔABD

= 30 m²+35.5m² = 65.5 m²

2. Find the area of a quadrilateral ABCD in which AB = 3 cm, BC = 4 cm, CD = 4 cm, DA = 5 cm and AC = 5 cm.

Solution:

First, construct a diagram with the given parameter.

Now, apply Pythagorean theorem in ΔABC,

AC² = AB²+BC²

⇒ 5² = 3²+4²

⇒ 25 = 25

Thus, it can be concluded that ΔABC is a right angled at B.

So, area of ΔBCD = (½ ×3×4) = 6 cm²

The semi perimeter of ΔACD (s) = (perimeter/2) = (5+5+4)/2 cm = 14/2 cm = 7 m

Now, using Heron’s formula,

Area of ΔACD

= 2√21 cm² = 9.17 cm² (approximately)

Area of quadrilateral ABCD = Area of ΔABC + Area of ΔACD = 6 cm² +9.17 cm² = 15.17 cm²

3. Radha made a picture of an aeroplane with coloured paper as shown in Fig 12.15. Find the total area of the paper used.

Solution:

For the triangle I section:

It is an isosceles triangle and the sides are 5 cm, 1 cm and 5 cm

Perimeter = 5+5+1 = 11 cm

So, semi perimeter = 11/2 cm = 5.5 cm

Using Heron’s formula,

Area = √[s(s-a)(s-b)(s-c)]

= √[5.5(5.5- 5)(5.5-5)(5.5-1)] cm²

= √[5.5×0.5×0.5×4.5] cm²

= 0.75√11 cm²

= 0.75 × 3.317cm²

= 2.488cm² (approx)

For the quadrilateral II section:

This quadrilateral is a rectangle with length and breadth as 6.5 cm and 1 cm respectively.

∴ Area = 6.5×1 cm²=6.5 cm²

For the quadrilateral III section:

It is a trapezoid with 2 sides as 1 cm each and the third side as 2 cm.

Area of the trapezoid = Area of the parallelogram + Area of the equilateral triangle

The perpendicular height of the parallelogram will be

= 0.86 cm

And, the area of the equilateral triangle will be (√3/4×a²) = 0.43

∴ Area of the trapezoid = 0.86+0.43 = 1.3 cm² (approximately).

For triangle IV and V:

These triangles are 2 congruent right angled triangles having the base as 6 cm and height 1.5 cm

Area triangles IV and V = 2×(½×6×1.5) cm² = 9 cm²

So, the total area of the paper used = (2.488+6.5+1.3+9) cm² = 19.3 cm²

4. A triangle and a parallelogram have the same base and the same area. If the sides of the triangle are 26 cm, 28 cm and 30 cm, and the parallelogram stands on the base 28 cm, find the height of the parallelogram.

Solution:

Given,

It is given that the parallelogram and triangle have equal areas.

The sides of the triangle are given as 26 cm, 28 cm and 30 cm.

So, the perimeter = 26+28+30 = 84 cm

And its semi perimeter = 84/2 cm = 42 cm

Now, by using Heron’s formula, area of the triangle =

= √[42(42-26)(42-28)(42-30)] cm²

= √[42×16×14×12] cm²

= 336 cm²

Now, let the height of parallelogram be h.

As the area of parallelogram = area of the triangle,

28 cm× h = 336 cm²

∴ h = 336/28 cm

So, the height of the parallelogram is 12 cm.

5. A rhombus shaped field has green grass for 18 cows to graze. If each side of the rhombus is 30 m and its longer diagonal is 48 m, how much area of grass field will each cow be getting?

Solution:

Draw a rhombus-shaped field first with the vertices as ABCD. The diagonal AC divides the rhombus into two congruent triangles which are having equal areas. The diagram is as follows.

Consider the triangle BCD,

Its semi-perimeter = (48 + 30 + 30)/2 m = 54 m

Using Heron’s formula,

Area of the ΔBCD

= 432 m²

∴ Area of field = 2 × area of the ΔBCD = (2 × 432) m² = 864 m²

Thus, the area of the grass field that each cow will be getting = (864/18) m² = 48 m²

6. An umbrella is made by stitching 10 triangular pieces of cloth of two different colours (see Fig.12.16), each piece measuring 20 cm, 50 cm and 50 cm. How much cloth of each colour is required for the umbrella?

Solution:

For each triangular piece, The semi perimeter will be

s = (50+50+20)/2 cm = 120/2 cm = 60cm

Using Heron’s formula,

Area of the triangular piece

=

= √[60(60-50)(60-50)(60-20)] cm²

= √[60×10×10×40] cm²

= 200√6 cm²

∴ The area of all the triangular pieces = 5 × 200√6 cm² = 1000√6 cm²

7. A kite in the shape of a square with a diagonal 32 cm and an isosceles triangle of base 8 cm and sides 6 cm each is to be made of three different shades as shown in Fig. 12.17. How much paper of each shade has been used in it?

Solution:

As the kite is in the shape of a square, its area will be

A = (½)×(diagonal)²

Area of the kite = (½)×32×32 = 512 cm^2.

The area of shade I = Area of shade II

512/2 cm² = 256 cm²

So, the total area of the paper that is required in each shade = 256 cm²

For the triangle section (III),

The sides are given as 6 cm, 6 cm and 8 cm

Now, the semi perimeter of this isosceles triangle = (6+6+8)/2 cm = 10 cm

By using Heron’s formula, the area of the III triangular piece will be

=

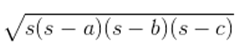
= √[10(10-6)(10-6)(10-8)] cm²

= √(10×4 ×4×2) cm²

= 8√5 cm² = 17.92 cm² (approx.)

8. A floral design on a floor is made up of 16 tiles which are triangular, the sides of the triangle being 9 cm, 28 cm and 35 cm (see Fig. 12.18). Find the cost of polishing the tiles at the rate of 50p per cm².

Solution:

The semi perimeter of the each triangular shape = (28+9+35)/2 cm = 36 cm

By using Heron’s formula,

The area of each triangular shape will be

= 36√6 cm² = 88.2 cm²

Now, the total area of 16 tiles = 16×88.2 cm² = 1411.2 cm²

It is given that the polishing cost of tiles = 50 paise/cm²

∴ The total polishing cost of the tiles = Rs. (1411.2×0.5) = Rs. 705.6

9. A field is in the shape of a trapezium whose parallel sides are 25 m and 10 m. The non-parallel sides are 14 m and 13 m. Find the area of the field.

Solution:

First, draw a line segment BE parallel to the line AD. Then, from B, draw a perpendicular on the line segment CD.

Now, it can be seen that the quadrilateral ABED is a parallelogram. So,

AB = ED = 10 m

AD = BE = 13 m

EC = 25-ED = 25-10 = 15 m

Now, consider the triangle BEC,

Its semi perimeter (s) = (13+14+15)/2 = 21 m

By using Heron’s formula,

Area of ΔBEC =

= 84 m²

We also know that the area of ΔBEC = (½)×CE×BF

84 cm² = (½)×15×BF

BF = (168/15) cm = 11.2 cm

So, the total area of ABED will be BF×DE i.e. 11.2×10 = 112 m²

∴ Area of the field = 84 + 112 = 196 m²

This formula is used to find the area of a triangle when the length of the 3 sides is the only data available.

Heron also contributed in several other ways, the most notable one being the invention of the Aeolipile. The Aeolipile was the very first steam engine.

Though it was technically a steam engine, it didn’t resemble an engine. And no practical applications could be found for this invention. It was projected as a toy and a novelty item for the ancient Greeks.

Explore other important concepts related to Heron’s Formula, learn how to solve numerical problems and more only on NCERT Solutions For Class 9 Maths – the best resources online to help you study better.

Key Features of NCERT Solutions for Class 9 Maths Chapter 12 Heron’s Formula Exercise 12.2

1. Well-structured content
2. All relevant formulas highlighted
3. Jargon-free and simple language used
4. Solutions drafted by well-qualified teachers
5. Questions are updated regularly
6. Breakdown of tough questions for easy comprehension

Explore: NCERT Solutions Class 9