NCERT Exemplar Solutions Class 9 Maths Chapter 1 – Free PDF Download
NCERT Exemplar Class 9 Maths Chapter 1 Number System is provided here for students to prepare well for exams. These exemplar problems and solutions are designed by experts in accordance with the CBSE Syllabus for Class 9, which covers the following topics of the Number System:
- Rational numbers and irrational numbers
- Finding rational numbers between two given numbers
- Locating irrational numbers in a number line
- Real numbers and their decimal expansions are terminating or non-terminating, recurring or non-recurring.
- Finding irrational numbers between two given numbers
- Operations performed on real numbers
- Rationalising the denominator
- Laws of exponents for real numbers
To facilitate easy learning and help students understand the concepts discussed in Chapter 1, free NCERT Exemplars are provided here, which can be downloaded in the form of a PDF. Students can use these materials as a reference tool for studying as well as practising sums. The exemplars also contain solved questions relevant to the exercise problems present in the NCERT textbook.
Download the PDF of NCERT Exemplar Solutions for Class 9 Maths Chapter 1 – Number Systems
Access Answers to NCERT Exemplar Solutions for Class 9 Maths Chapter 1 Number Systems
Exercise 1.1 Page No: 2
Write the correct answer in each of the following:
1. Every rational number is
(A) a natural number
(B) an integer
(C) a real number
(D) a whole number
Solution:
(C) a real number
Explanation:
We know that rational and irrational numbers taken together are known as real numbers. Therefore, every real number is either a rational number or an irrational number. Hence, every rational number is a real number.
Hence, (C) is the correct option.
2. Between two rational numbers
(A) there is no rational number
(B) there is exactly one rational number
(C) there are infinitely many rational numbers
(D) there are only rational numbers and no irrational numbers
Solution:
(C) there are infinitely many rational numbers
Explanation:
Between two rational numbers, there is infinitely many rational numbers.
Hence, (C) is the correct option.
3. Decimal representation of a rational number cannot be
(A) terminating
(B) non-terminating
(C) non-terminating repeating
(D) non-terminating non-repeating
Solution:
(D) non-terminating non-repeating
Explanation:
The decimal representation of a rational number cannot be non-terminating and non-repeating.
Hence, (D) is the correct option.
4. The product of any two irrational numbers is
(A) always an irrational number
(B) always a rational number
(C) always an integer
(D) sometimes rational, sometimes irrational
Solution:
(D) sometimes rational, sometimes irrational
Explanation:
The product of any two irrational numbers is sometimes rational and sometimes irrational.
Hence, (D) is the correct option.
5. The decimal expansion of the number √2 is
(A) a finite decimal
(B) 1.41421
(C) non-terminating recurring
(D) non-terminating non-recurring
Solution:
(D) non-terminating non-recurring
Explanation:
The decimal expansion of the number √2 = 1.41421356237…
Hence, (D) is the correct option.
6. Which of the following is irrational?
(A) √4/√9
(B) √12/√3
(C) √7
(D) √81
Solution:
(C) √7
Explanation:
(A) √4/√9 = 2/3
(B) √12/√3 = 2√3/√3 = 2
(C) √7 = 2.64575131106
(D) √81 = 9
Here, (C) √7 = 2.64575131106 is a non-terminating decimal expansion.
Hence, (C) is the correct option.
7. Which of the following is irrational?

Solution:
(D) 0.4014001400014…
Explanation:
A number is irrational if and only if its decimal representation is non-terminating and non-recurring.
(A) is a terminating decimal and, therefore, cannot be an irrational number.
(B) is a non-terminating and recurring decimal and, therefore, cannot be irrational.
(C) is a non-terminating and recurring decimal and, therefore, cannot be irrational.
(D) is a non-terminating and non-recurring decimal and therefore is an irrational number.
Hence, (D) is the correct option.
8. A rational number between √2 and √3 is
(A) (√2+√3)/2
(B) (√2. √3)/2
(C) 1.5
(D) 1.8
Solution:
(C) 1.5
Explanation:
√2 =1.4142135…. and √3 =1.732050807….
(A) (√2+√3)/2 = 1.57313218497… is a non-terminating and non-recurring decimal and, therefore, is an irrational number.
(B) (√2. √3)/2 = 1.22474487139… is a non-terminating and non-recurring decimal and, therefore, is an irrational number.
(C) 1.5 is a terminating decimal and, therefore, is a rational number.
(D) 1.8 is a terminating decimal and, therefore, is a rational number.
Here both 1.5 and 1.8 are rational numbers. But, 1.8 does not lie in between √2 =1.4142135…. and √3 =1.732050807…. Whereas 1.5 lies in between √2 =1.4142135…. and √3 =1.732050807….
Hence, (C) is the correct option.
9. The value of 1.999… in the form p/q, where p and q are integers and q ≠ 0, is
(A) 19/10
(B) 1999/1000
(C) 2
(D) 1/9
Solution:
(C) 2
Explanation:
(A) 19/10 = 1.9
(B) 1999/1000= 1.999
(C) 2
(D) 1/9 = 0.111….
Let x = 1.9999….. — (1)
Multiply equation (1) with 10
10x = 19.9999….. — (2)
Subtract equation (1) from equation(2),
We get,
9x = 18
x = 18 / 9
x = 2
Therefore,
x = 1.9999… = 2
Hence, (C) is the correct option.
10. 2√3 + √3 is equal to
(A) 2√6
(B) 6
(C) 3√3
(D) 4√6
Solution:
(C) 3√3
Explanation:
2√3 + √3
Taking √3 common,
We get,
√3(2+1) = √3(3) = 3√3
Hence, (C) is the correct option.
Exercise 1.2 Page No: 6
1. Let x and y be rational and irrational numbers, respectively. Is x + y necessarily an irrational number? Give an example in support of your answer.
Solution:
Yes, if x and y are rational and irrational numbers, respectively, then x+ y is an irrational number.
For example,
Let x = 5 and y = √2.
Then, x+y = 5 + √2 = 5 + 1.414… = 6.414…
Here, 6.414 is a non-terminating and non-recurring decimal and therefore is an irrational number.
Hence, x + y is an irrational number.
2. Let x be rational and y be irrational. Is xy necessarily irrational? Justify your answer with an example.
Solution:
No, if x is a rational number and y is an irrational number, then xy is not necessarily an irrational number. It can be rational if x = 0, which is a rational number.
For example:
Let y = √2, which is irrational.
Consider x = 2, which is rational.
Then, x × y = 2 × √2 = 2√2, which is irrational.
Consider x = 0, which is rational.
Then xy = 0 × √2 = 0, which is rational.
Therefore, we can conclude that the product of a rational and an irrational number is always irrational, only if the rational number is not zero.
Exercise 1.3 Page No: 9
1. Find which of the variables x, y, z and u represent rational numbers and which irrational numbers:
(i) x2 = 5
(ii) y2 = 9
(iii) z2 = .04
(iv) 𝑢2 = 17/4
Solution:
(i) x2 = 5
On solving, we get
⇒ x = ± √5
Hence, x is an irrational number.
(ii) y2 = 9
On solving, we get
⇒ y = ± 3
Hence, y is a rational number.
(iii) z2 = .04
On solving, we get
⇒ z = ± 0.2
Hence, z is a rational number.
(iv) u2 = 17/4
On solving, we get
⇒ u = ± √17/2
√17 is irrational.
Hence, u is an irrational number
2. Find three rational numbers between
(i) –1 and –2
(ii) 0.1 and 0.11
(iii) 5/7 and 6/7
(iv) 1/4 and 1/5
Solution:
(i) –1 and –2
Three rational numbers between –1 and –2 are –1.1, –1.2 and –1.3.
(ii) 0.1 and 0.11
Three rational numbers between 0.1 and 0.11 are 0.101, 0.102 and 0.103.
(iii) 5/7 and 6/7
5/7 can be written as (5 × 10)/(7 × 10) = 50/70
Similarly,
6/7 can be written as (6 × 10)/(7 × 10) = 60/70
Three rational numbers between 5/7 and 6/7 = three rational numbers between 50/70 and 60/70.
Three rational numbers between 5/7 and 6/7 are 51/70, 52/70, 53/70.
(iv) 1/4 and 1/5
Here, according to the question,
LCM of 4 and 5 is 20.
Let us make the denominators common, 80.
(4 × 20) = 80 and (5 × 16) = 80
Hence,
1/4 can be written as (1 × 20)/(4 × 20) = 20/80
Similarly,
1/5 can be written as (1 × 16)/(5 × 16) = 16/80
Three rational numbers between 1/4 and 1/5 = three rational numbers between 16/80 and 20/80.
Therefore, the three rational numbers are 17/80, 18/80 and 19/80.
3. Insert a rational number and an irrational number between the following:
(i) 2 and 3
(ii) 0 and 0.1
(iii) 1/3 and 1/2
(iv) –2/5 and 1/2
(v) 0.15 and 0.16
(vi) √2 and √3
(vii) 2.357 and 3.121
(viii) .0001 and .001
(ix) 3.623623 and 0.484848
(x) 6.375289 and 6.375738.
Solution:
(i) 2 and 3
So, the rational number between 2 and 3 = 2.5
And, the irrational number between 2 and 3 = 2.040040004…
(ii) 0 and 0.1
So, the rational number between 0 and 0.1 = 0.05
And, irrational number between 0 and 0.1 = 0.007000700007…
(iii) 1/3 and 1/2
LCM of 3 and 2 is 6.
1/3 = 0.33
1/3 can be written as (1 × 20)/(3 × 20) = 20/60
½ = 0.5
1/2 can be written as (1 × 30)/(2 × 30) = 30/60
So, the rational number between 1/3 and 1/2 = 25/60
And, irrational number between 1/3 and 1/2 = irrational number between 0.33 and 0.5 = 0.414114111…
(iv) – 2/5 and 1/2
LCM of 5 and 2 is 10.
-2/5 = -0.4
-2/5 can be written as (-2 × 2)/(5 × 2) = -4/10
1/2 = 0.5
1/2 can be written as (1 × 5)/(2 × 5) = 5/10
So, rational number between -2/5 and 1/2 = rational number between -4/10 and 5/10 = 1/10
And, irrational number between -2/5 and 1/2 = irrational number between -0.4 and 0.5 = 0.414114111…
(v) 0.15 and 0.16
The rational number between 0.15 and 0.16 = 0.151
The irrational number between 0.15 and 0.16 = 0.151551555…
(vi) √2 and √3
√2 = 1.41 and √3 = 1.732
Rational number between √2 and √3 = rational number between 1.41 and 1.732 = 1.5
The irrational number between √2 and √3 = irrational number between 1.41 and 1.732 = 1.585585558…
(vii) 2.357 and 3.121
The rational number between 2.357 and 3.121 = 3
The irrational number between 2.357 and 3.121 = 3.101101110…
(viii) .0001 and .001
Rational number between .0001 and .001 = 0.00011
The irrational number between .0001 and .001 = 0.0001131331333…
(ix) 3.623623 and 0.484848
The rational number between 3.623623 and 0.484848 = 1
The irrational number between 3.623623 and 0.484848 = 1.909009000…
(x) 6.375289 and 6.375738.
The rational number between 6.375289 and 6.375738 = 6.3753
The irrational number between 6.375289 and 6.375738 = 6.375414114111…
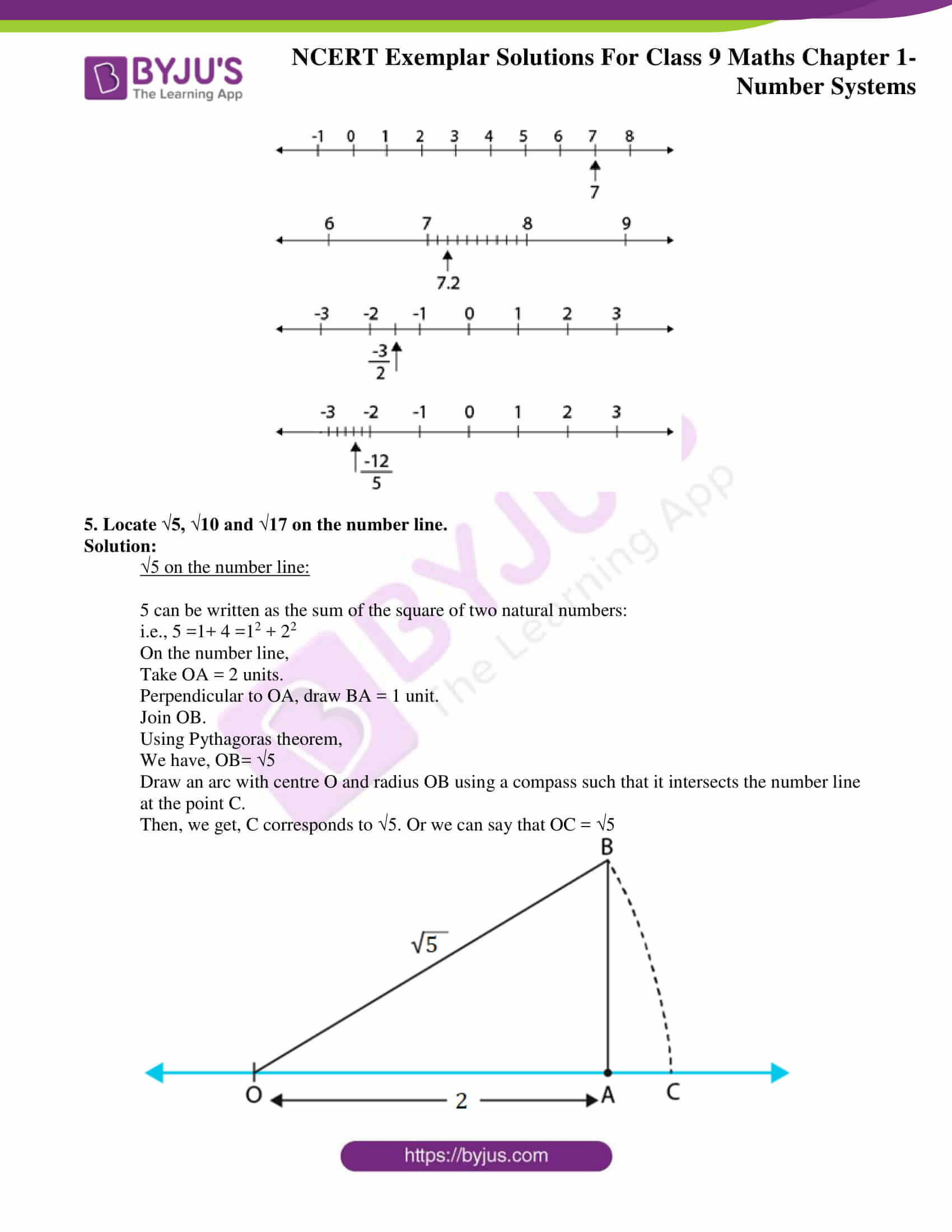
4. Represent the following numbers on the number line:
7, 7.2, −3/2, −12/5
Solution:

5. Locate √5, √10 and √17 on the number line.
Solution:
√5 on the number line:
5 can be written as the sum of the square of two natural numbers:
i.e., 5 =1+ 4 =12 + 22
On the number line,
Take OA = 2 units.
Perpendicular to OA, draw BA = 1 unit.
Join OB.
Using the Pythagoras theorem,
We have, OB= √5
Draw an arc with centre O and radius OB using a compass such that it intersects the number line at point C.
Then, we get, C corresponds to √5. Or we can say that OC = √5

√10 on the number line:
10 can be written as the sum of the square of two natural numbers:
i.e., 10 =1+ 9 =12 + 32
On the number line,
Take OA = 3 units.
Perpendicular to OA, draw BA = 1 unit.
Join OB.
Using the Pythagoras theorem,
We have, OB= √10
Draw an arc with centre O and radius OB using a compass such that it intersects the number line at point C.
Then, point C corresponds to √10. Or we can say that OC = √10

√17 on the number line:
17 can be written as the sum of the square of two natural numbers:
i.e., 17 =1+ 16 =12 + 42
On the number line,
Take OA = 4 units.
Perpendicular to OA, draw BA = 1 unit.
Join OB.
Using the Pythagoras theorem,
We have, OB= √17
Draw an arc with centre O and radius OB using a compass such that it intersects the number line at point C.
Then, point C corresponds to √17. Or, we can say that OC = √17

6. Represent geometrically the following numbers on the number line:
(i) √4.5
(ii) √5.6
(iii) √8.1
(iv) √2.3
Solution:
(i) √4.5
Draw a line segment such that AB = 4.5 units.
Mark C at a distance of 1 unit from B.
Mark O is the mid-point of AC.
Draw a semicircle with centre O and radius OC.
Draw a line perpendicular to AC, passing through B and intersecting the semicircle at D.
Now, BD = √4.5.
Draw an arc with centre B and radius BD, meeting AC produced at E.
Then, BE = BD = √4.5 units.

(ii) √5.6
Draw a line segment such that AB = 5.6 units.
Mark C at a distance of 1 unit from B.
Mark O is the mid-point of AC.
Draw a semicircle with centre O and radius OC.
Draw a line perpendicular to AC, passing through B and intersecting the semicircle at D.
Now, BD = √5.6
Draw an arc with centre B and radius BD, meeting AC produced at E.
Then BE = BD = √5.6 units.

(iii) √8.1
Draw a line segment such that AB = 8.1 units.
Mark C at a distance of 1 unit from B.
Mark O, is the mid-point of AC.
Draw a semicircle with centre O and radius OC.
Draw a line perpendicular to AC, passing through B and intersecting the semicircle at D.
Now, BD = √8.1.
Draw an arc with centre B and radius BD, meeting AC produced at E.
Then BE = BD = √8.1 units.

(iv) √2.3
Draw a line segment such that AB = 2.3 units.
Mark C at a distance of 1 unit from B.
Mark O is the mid-point of AC.
Draw a semicircle with centre O and radius OC.
Draw a line perpendicular to AC, passing through B and intersecting the semicircle at D.
Now, BD = √2.3.
Draw an arc with centre B and radius BD, meeting AC produced at E.
Then BE = BD = √2.3 units.

7. Express the following in the form p/q, where p and q are integers and q ≠ 0 :
(i) 0.2
(ii) 0.888…
(iii) 
(iv) 
(v) 0.2555…
(vi) 
(vii) .00323232…
(viii) .404040…
Solution:
(i) 0.2
We know that,
0/2 can be written as,
0.2 = 2/10 = 1/5
(ii) 0.888…
Assume that 𝑥 = 0.888 …
⇒ 𝑥 = 0.8 ……………. Eq.(1)
Multiply L.H.S and R.H.S by 10,
We get
10 𝑥 = 8.8 ……………. Eq.(2)
Subtracting equation (1) from (2),
We get
10 𝑥 − 𝑥 = 8.8 − 0.8
⇒ 9𝑥 = 8
⇒ 𝑥 = 8/9
(iii)

Assume that 𝑥 = 5.2 ……………. Eq.(1)
Multiply L.H.S and R.H.S by 10,
We get
10 𝑥 = 52.2 …………… Eq. (2)
Subtracting equation (1) from (2),
We get
10 𝑥 − 𝑥 = 52.2 − 5.2
⇒ 9𝑥 = 47
⇒ 𝑥 = 47/9
(iv)

Assume that 𝑥 = 0.001 ……………. Eq. (1)
Multiply L.H.S and R.H.S by 1000,
We get
1000 𝑥 = 1.001 …………… Eq. (2)
Subtracting equation (1) from (2),
We get
1000𝑥 − 𝑥 = 1.001 − 0.001
⇒ 999𝑥 = 1
⇒ 𝑥 = 1/999
(v) 0.2555…
Assume that 𝑥 = 0.2555 …
⇒ x = 0.25 ……………. Eq. (1)
Multiply L.H.S and R.H.S by 10,
We get
10 x = 2.5 ……………. Eq. (2)
Multiply L.H.S and R.H.S by 100,
We get
100 x = 25.5 …………. Eq. (3)
Subtracting equation (2) from (3),
We get
100 x-10x = 25.5 – 2.5
⇒ 90𝑥 = 23
⇒ 𝑥 = 23/90
(vi)

Let 𝑥 = 0.134 ………….…. Eq. (1)
Multiply L.H.S and R.H.S by 10,
We get
10 𝑥 = 1.34 ………………. Eq. (2)
Multiply L.H.S and R.H.S by 1000,
We get
1000 𝑥 = 134.34 …………. Eq. (3)
Subtracting equation (2) from (3),
We get
1000 𝑥 − 10𝑥 = 134.34 − 1.34
⇒ 990𝑥 = 133
⇒ 𝑥 = 133/990
(vii) .00323232…
Let 𝑥 = 0.00323232 …
⇒ x = 0.0032 ………….…. Eq. (1)
Multiply L.H.S and R.H.S by 100,
We get,
100x = 0.32 ……………. Eq. (2)
Multiply L.H.S and R.H.S by 10000,
We get
10000 x = 32.32 …………. Eq. (3)
Subtracting equation (2) from (3),
We get
10000 x-100x = 32.32 – 0.32
⇒ 9900𝑥 = 32
⇒ 𝑥 = 32/9900 = 8/2475
(viii) .404040…
Let 𝑥 = 0.404040 …
⇒ 𝑥 = 0. 40 ………..….…. (1)
Multiply L.H.S and R.H.S by 100,
We get
100 𝑥 = 40.40 ……….…. (2)
Subtracting equation (1) from (2),
We get
100 𝑥 − 𝑥 = 40.40 − 0.40
⇒ 99𝑥 = 40
⇒ 𝑥 = 40/99
Exercise 1.4 Page No: 12
1. Express  in the form p/q, where p and q are integers and q ≠ 0.
in the form p/q, where p and q are integers and q ≠ 0.
Solution:
Let x = 0.6
Multiply by 10 on L.H.S and R.H.S,
10x = 6
x = 6/10
x = 3/5
So, the p/q form of 0.6 = 3/5
Let y = 0.77777…
Multiply by 10 on L.H.S and R.H.S,
10y = 7.7777…
10y – y = 7.7777777……. – 0.7777777…………..
9y = 7
y = 7/9
So the p/q form of 0.7777… = 7/9
Let z = 0.47777…
Multiply by 10 on L.H.S and R.H.S,
10z = 4.7777…
10z – z = 4.7777777… – 0.47777777…
9z = 4.2999
z ≈ 4.3/9
z = 43/90
So the p/q form of 0.4777… = 43/90
Therefore, p/q form of

is,
x+y+z = 3/5 + 7/9 + 43/90
= (54 + 70 + 43)/90
= 167/90
2. Simplify:

Solution:

Let us first make the denominators the same,
To make the denominators the same, cross-multiply the first and second terms of the equation.

Now, again make the denominators the same by cross-multiplying the obtained term and the third term of the given equation in the question.

3. If √2 =1.414, √3 =1.732, then find the value of

Solution:

Let us first make the denominators the same by cross multiplication method

Observing the denominator, we can say that,
Denominators are of the form,
(a + b) × (a – b) = (a2 – b2)
Here a = 3√3
b = 2√2
a2 = (3√3)2 = 27
b2 = (2√2)2 = 8

| Also Access |
| NCERT Exemplar for Class 9 Maths Chapter 1 |
| CBSE Notes for Class 9 Maths Chapter 1 |
BYJU’S also provides study resources, such as notes, exemplar books, NCERT Solutions and question papers for students to prepare well and score good marks in their exams. Also, it is recommended to solve sample papers and previous years’ question papers which gives an idea of question types asked in the annual exam from Chapter 1, Number System. Solving the NCERT Exemplar questions will help students to understand the chapter and to practise higher-level questions related to the Number System.
Download BYJU’S – The Learning App and get personalised videos, explaining different types of Maths topics, such as number systems and different types of numbers, etc., and experience a new way of learning to understand the concepts easily.
Frequently Asked Questions on NCERT Exemplar Solutions for Class 9 Maths Chapter 1
What are the topics covered in Chapter 1 of NCERT Exemplar Solutions for Class 9 Maths?
1. Rational numbers and irrational numbers
2. Finding rational numbers between two given numbers
3. Locating irrational numbers in a number line
4. Real numbers and their decimal expansions are terminating or non-terminating, recurring or non-recurring.
5. Finding irrational numbers between two given numbers
6. Operations performed on real numbers
7. Rationalising the denominator
8. Laws of exponents for real numbers
What are rational numbers according to NCERT Exemplar Solutions for Class 9 Maths Chapter 1?
For example, 3/2 is a rational number. It means integer 3 is divided by another integer 2.

















Good work for byjus
very useful solutions