RD Sharma Solutions for Class 10 Maths Chapter 6 Trigonometric Identities

RD Sharma Solutions Class 10 Maths Chapter 6 – Free PDF Download

RD Sharma Solutions for Class 10 Maths Chapter 6 – Trigonometric Identities are provided here. The branch of Mathematics which deals with the measurement of the sides and the angles of a triangle is known as trigonometry. Students who find it difficult to understand the concepts covered in this chapter can make their learning process smooth and easy using RD Sharma Solutions. These solutions are well structured by our subject expert team at BYJU’S to help students grasp the in-depth knowledge of concepts which are vital for examinations.

Trigonometric Identities is the 6th Chapter of RD Sharma Solutions Class 10. This chapter consists of two exercises. Students can find the precise answers for these exercises in RD Sharma Solutions for Class 10. The previous chapter was about trigonometric ratios and the relations between them. But this chapter explains the trigonometric identities in a comprehensive manner in accordance with the student’s intelligence quotient.

RD Sharma Solutions for Class 10 Maths Chapter 6 Trigonometric Identities Here

Download PDF Here

carouselExampleControls111

Previous



Next

Access the RD Sharma Solutions for Class 10 Maths Chapter 6 – Trigonometric Identities

RD Sharma Solutions for Class 10 Maths Chapter 6 Exercise 6.1 Page No: 6.43

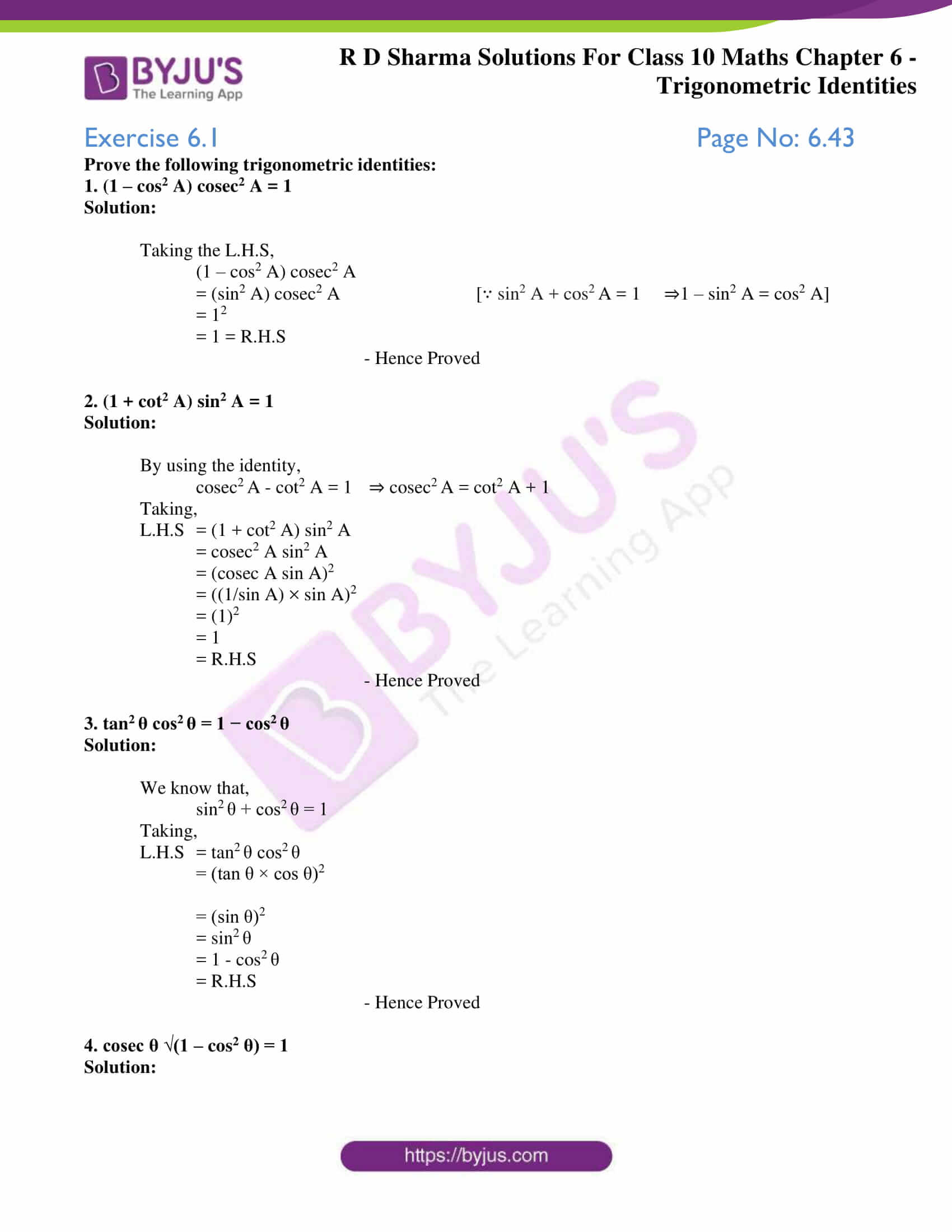
Prove the following trigonometric identities:

1. (1 – cos² A) cosec² A = 1

Solution:

Taking the L.H.S.,

(1 – cos² A) cosec² A

= (sin² A) cosec² A [∵ sin² A + cos² A = 1 ⇒1 – sin² A = cos² A]

= 1²

= 1 = R.H.S.

– Hence, proved.

2. (1 + cot² A) sin² A = 1 

Solution: 

By using the identity,

cosec² A – cot² A = 1 ⇒ cosec² A = cot² A + 1

Taking,

L.H.S. = (1 + cot² A) sin² A

= cosec² A sin² A

= (cosec A sin A)²

= ((1/sin A) × sin A)²

= (1)²

= 1

= R.H.S.

– Hence, proved.

3. tan² θ cos² θ = 1 − cos² θ 

Solution: 

We know that,

sin² θ + cos² θ = 1

Taking,

L.H.S. = tan² θ cos² θ

= (tan θ × cos θ)²

= (sin θ)²

= sin² θ

= 1 – cos² θ

= R.H.S.

– Hence, proved.

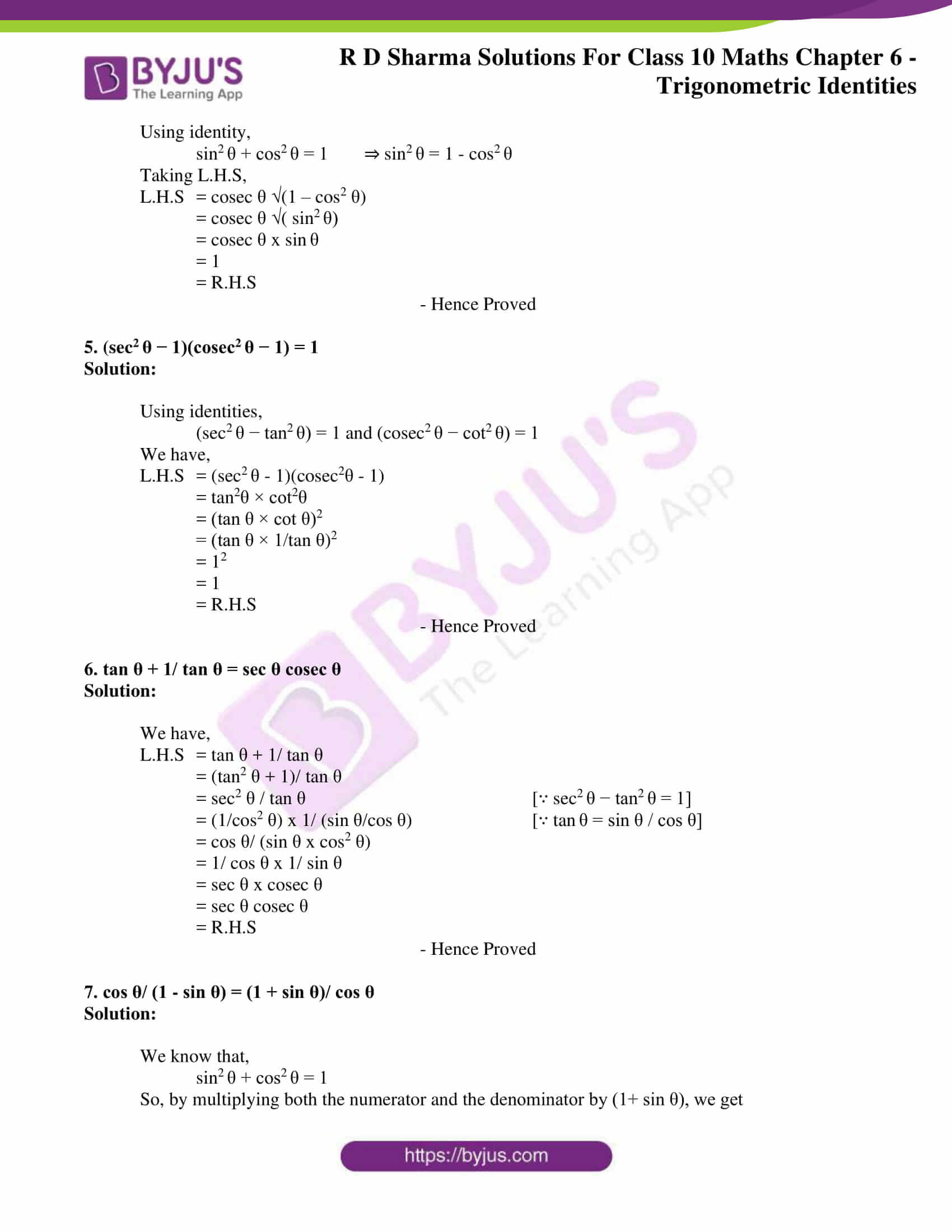
4. cosec θ √(1 – cos² θ) = 1

Solution:

Using identity,

sin² θ + cos² θ = 1  ⇒ sin² θ = 1 – cos² θ

Taking L.H.S.,

L.H.S = cosec θ √(1 – cos² θ)

= cosec θ √( sin² θ)

= cosec θ x sin θ

= 1

= R.H.S.

– Hence, proved.

5. (sec² θ − 1)(cosec² θ − 1) = 1 

Solution:

Using identities,

(sec² θ − tan² θ) = 1 and (cosec² θ − cot² θ) = 1

We have,

L.H.S. = (sec² θ – 1)(cosec²θ – 1)

= tan²θ × cot²θ

= (tan θ × cot θ)²

= (tan θ × 1/tan θ)²

= 1²

= 1

= R.H.S.

– Hence, proved.

6. tan θ + 1/ tan θ = sec θ cosec θ

Solution:

We have,

L.H.S. = tan θ + 1/ tan θ

= (tan² θ + 1)/ tan θ

= sec² θ / tan θ [∵ sec² θ − tan² θ = 1]

= (1/cos² θ) x 1/ (sin θ/cos θ) [∵ tan θ = sin θ / cos θ]

= cos θ/ (sin θ x cos² θ)

= 1/ cos θ x 1/ sin θ

= sec θ x cosec θ

= sec θ cosec θ

= R.H.S.

– Hence, proved.

7. cos θ/ (1 – sin θ) = (1 + sin θ)/ cos θ

Solution:

We know that,

sin² θ + cos² θ = 1

So, by multiplying both the numerator and the denominator by (1+ sin θ), we get

L.H.S. = R.H.S.

– Hence, proved.

8. cos θ/ (1 + sin θ) = (1 – sin θ)/ cos θ

Solution:

We know that,

sin² θ + cos² θ = 1

So, by multiplying both the numerator and the denominator by (1- sin θ), we get

L.H.S. = R.H.S.

– Hence, proved.

9. cos² θ + 1/(1 + cot² θ) = 1

Solution:

We already know that,

cosec² θ − cot² θ = 1 and sin² θ + cos² θ = 1

Taking L.H.S.,

= cos² A + sin² A

= 1

= R.H.S.

– Hence, proved.

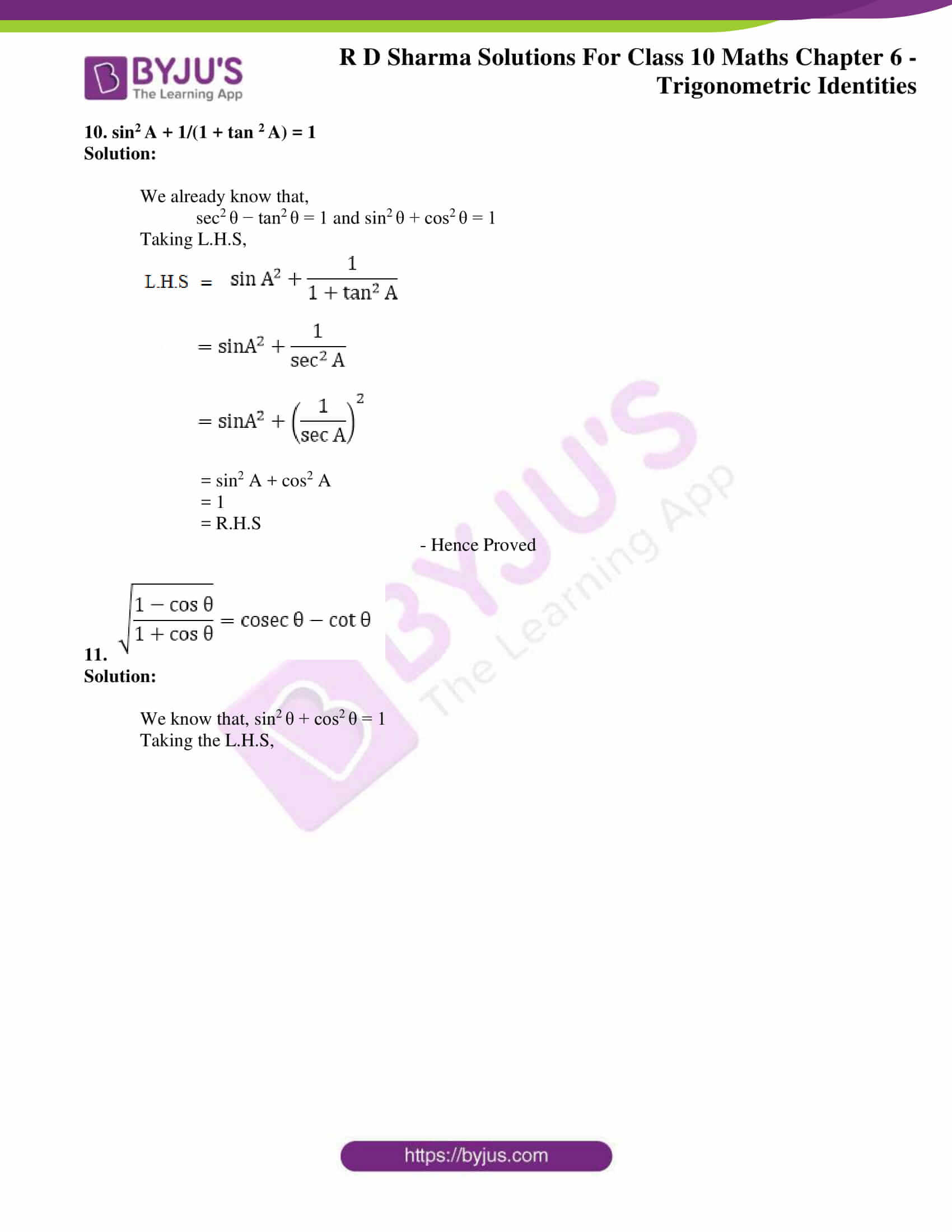
10. sin² A + 1/(1 + tan ² A) = 1

Solution:

We already know that,

sec² θ − tan² θ = 1 and sin² θ + cos² θ = 1

Taking L.H.S.,

= sin² A + cos² A

= 1

= R.H.S.

– Hence, proved.

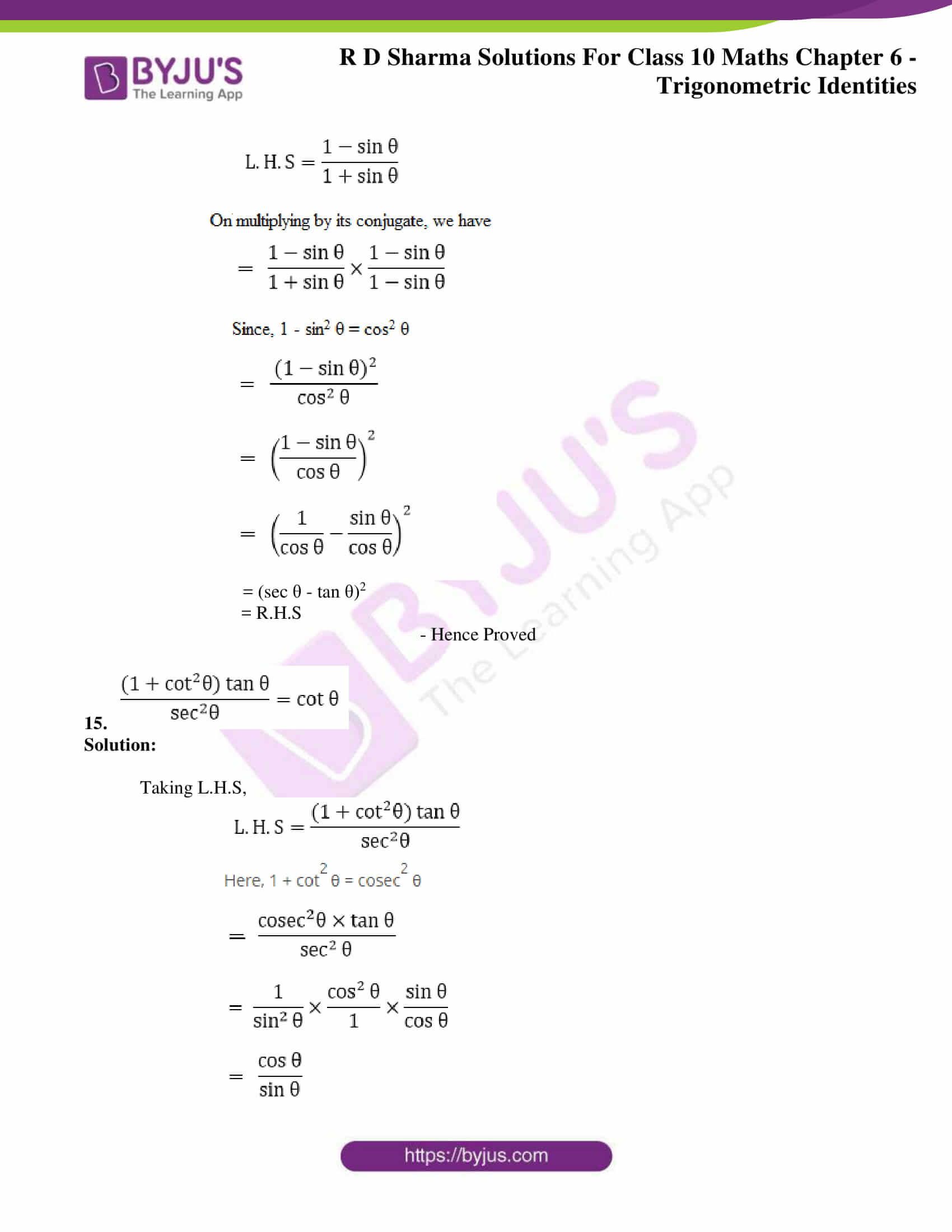
11.

Solution:

We know that,

sin² θ + cos² θ = 1

Taking the L.H.S.,

= cosec θ – cot θ

= R.H.S.

– Hence, proved.

12. 1 – cos θ/ sin θ = sin θ/ 1 + cos θ

Solution:

We know that,

sin² θ + cos² θ = 1

So, by multiplying both the numerator and the denominator by (1+ cos θ), we get

= R.H.S.

– Hence, proved.

13. sin θ/ (1 – cos θ) = cosec θ + cot θ

Solution:

Taking L.H.S.,

= cosec θ + cot θ

= R.H.S.

– Hence, proved.

14. (1 – sin θ) / (1 + sin θ) = (sec θ – tan θ)²

Solution:

Taking the L.H.S.,

= (sec θ – tan θ)²

= R.H.S.

– Hence, proved.

15.

Solution:

Taking L.H.S.,

= cot θ

= R.H.S.

– Hence, proved.

16. tan² θ − sin² θ = tan² θ sin² θ 

Solution:

Taking L.H.S.,

L.H.S = tan² θ − sin² θ 

= tan² θ sin² θ

= R.H.S.

– Hence, proved.

17. (cosec θ + sin θ)(cosec θ – sin θ) = cot²θ + cos²θ 

Solution:

Taking L.H.S. = (cosec θ + sin θ)(cosec θ – sin θ)

On multiplying, we get

= cosec² θ – sin² θ

= (1 + cot² θ) – (1 – cos² θ) [Using cosec² θ − cot² θ = 1 and sin² θ + cos² θ = 1]

= 1 + cot² θ – 1 + cos² θ

= cot² θ + cos² θ

= R.H.S.

– Hence, proved.

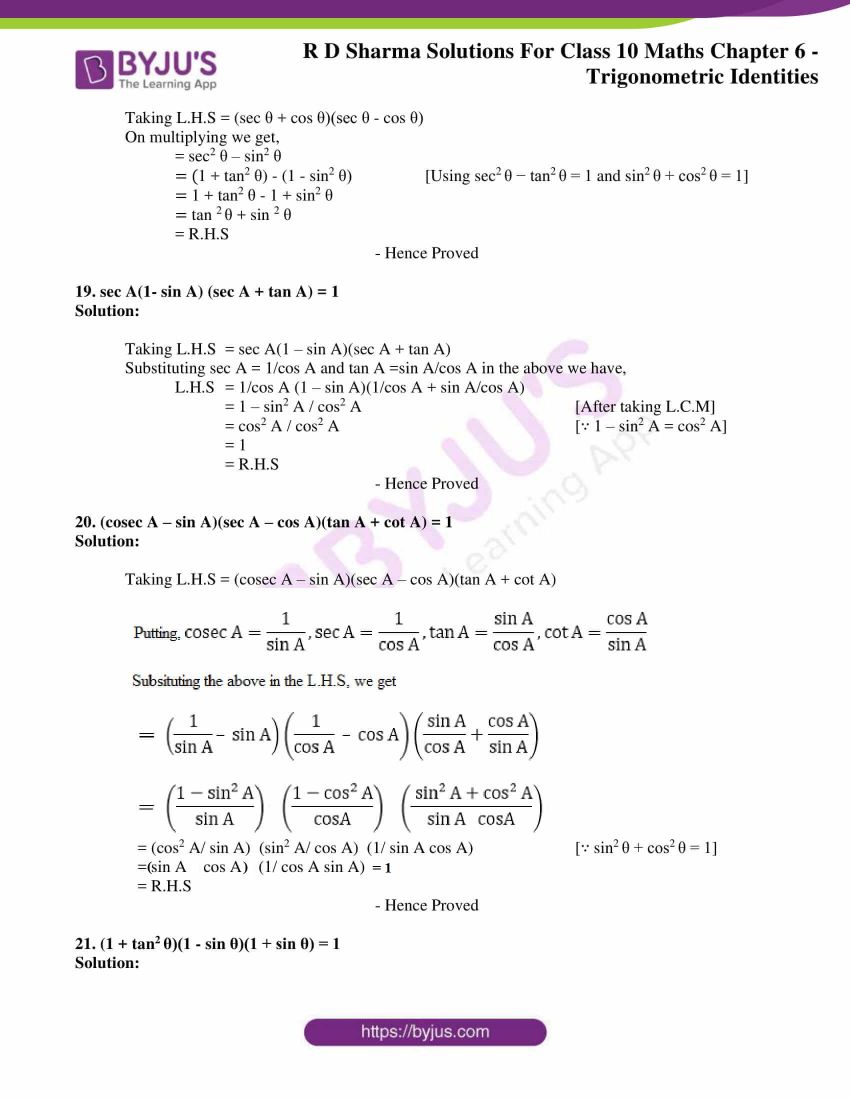
18. (sec θ + cos θ) (sec θ – cos θ) = tan² θ + sin² θ 

Solution:

Taking L.H.S. = (sec θ + cos θ)(sec θ – cos θ)

On multiplying, we get,

= sec² θ – sin² θ

= (1 + tan² θ) – (1 – sin² θ) [Using sec² θ − tan² θ = 1 and sin² θ + cos² θ = 1]

= 1 + tan² θ – 1 + sin² θ

= tan ² θ + sin ² θ

= R.H.S.

– Hence, proved.

19. sec A(1- sin A) (sec A + tan A) = 1

Solution:

Taking L.H.S. = sec A(1 – sin A)(sec A + tan A)

Substituting sec A = 1/cos A and tan A =sin A/cos A in the above, we have,

L.H.S = 1/cos A (1 – sin A)(1/cos A + sin A/cos A)

= 1 – sin² A / cos² A [After taking L.C.M]

= cos² A / cos² A [∵ 1 – sin² A = cos² A]

= 1

= R.H.S.

– Hence, proved.

20. (cosec A – sin A)(sec A – cos A)(tan A + cot A) = 1 

Solution:

Taking L.H.S. = (cosec A – sin A)(sec A – cos A)(tan A + cot A)

= (cos² A/ sin A) (sin² A/ cos A) (1/ sin A cos A) [∵ sin² θ + cos² θ = 1]

= (sin A  cos A)  (1/ cos A sin A)

= 1

= R.H.S.

– Hence, proved.

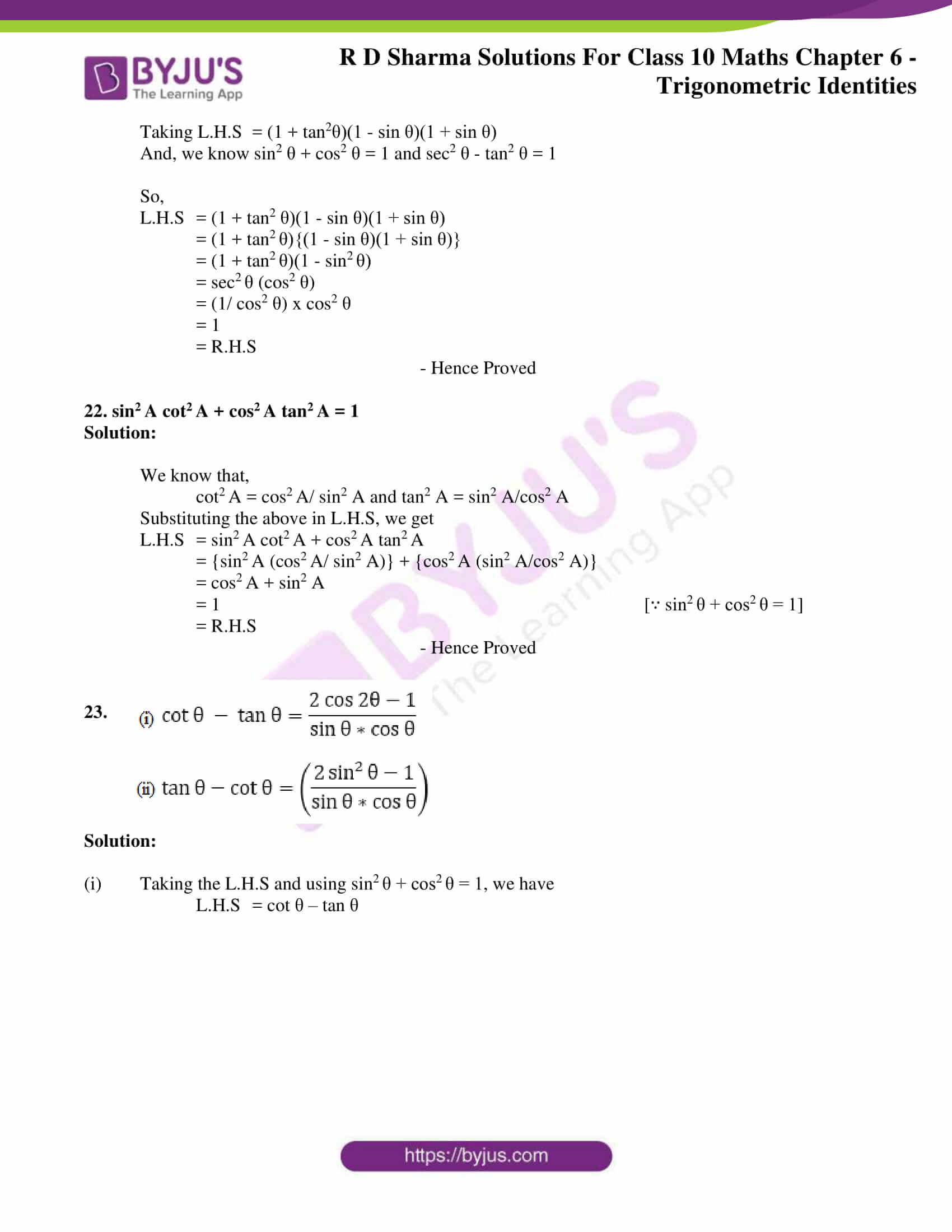
21. (1 + tan² θ)(1 – sin θ)(1 + sin θ) = 1

Solution:

Taking L.H.S. = (1 + tan²θ)(1 – sin θ)(1 + sin θ)

And, we know sin² θ + cos² θ = 1 and sec² θ – tan² θ = 1

So,

L.H.S = (1 + tan² θ)(1 – sin θ)(1 + sin θ)

= (1 + tan² θ){(1 – sin θ)(1 + sin θ)}

= (1 + tan² θ)(1 – sin² θ)

= sec² θ (cos² θ)

= (1/ cos² θ) x cos² θ

= 1

= R.H.S.

– Hence, proved.

22. sin² A cot² A + cos² A tan² A = 1

Solution:

We know that,

cot² A = cos² A/ sin² A and tan² A = sin² A/cos² A

Substituting the above in L.H.S., we get

L.H.S = sin² A cot² A + cos² A tan² A

= {sin² A (cos² A/ sin² A)} + {cos² A (sin² A/cos² A)}

= cos² A + sin² A

= 1 [∵ sin² θ + cos² θ = 1]

= R.H.S.

– Hence, proved.

23.

Solution:

(i) Taking the L.H.S and using sin² θ + cos² θ = 1, we have

L.H.S = cot θ – tan θ

= R.H.S.

– Hence, proved.

(ii) Taking the L.H.S. and using sin² θ + cos² θ = 1, we have

L.H.S. = tan θ – cot θ

= R.H.S.

– Hence, proved.

24. (cos² θ/ sin θ) – cosec θ + sin θ = 0

Solution:

Taking L.H.S. and using sin² θ + cos² θ = 1, we have

= – sin θ + sin θ

= 0

= R.H.S.

– Hence, proved.

25.

Solution:

Taking L.H.S.,

= 2 sec² A

= R.H.S.

– Hence, proved.

26.

Solution:

Taking the LHS and using sin² θ + cos² θ = 1, we have

= 2/ cos θ

= 2 sec θ

= R.H.S.

– Hence, proved.

27.

Solution:

Taking the LHS and using sin² θ + cos² θ = 1, we have

= R.H.S.

• Hence, proved.

28.

Solution:

Taking L.H.S.,

Using sec² θ − tan² θ = 1 and cosec² θ − cot² θ = 1

= R.H.S.

– Hence, proved.

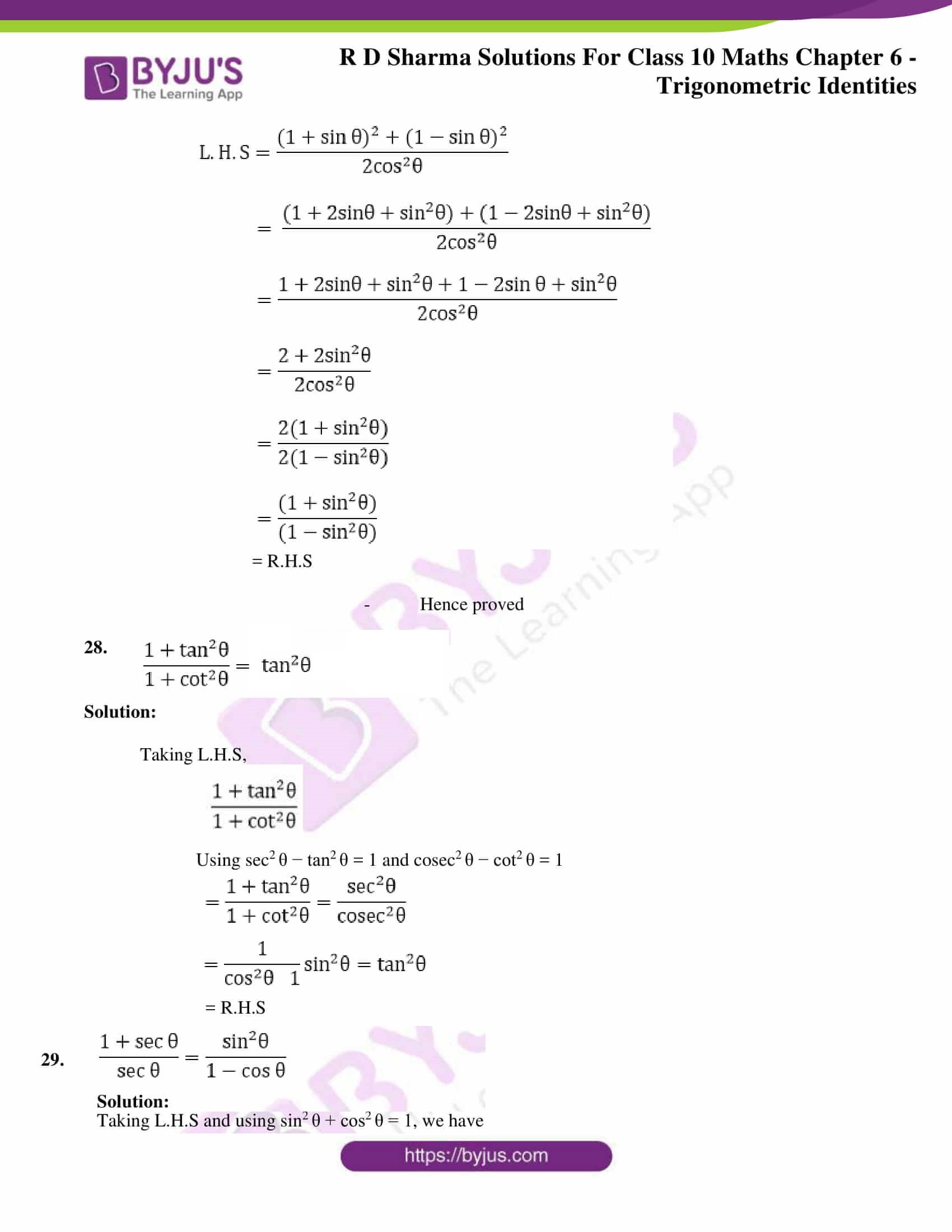
29.

Solution:

Taking L.H.S. and using sin² θ + cos² θ = 1, we have

= R.H.S.

– Hence, proved.

30.

Solution:

Taking LHS, we have

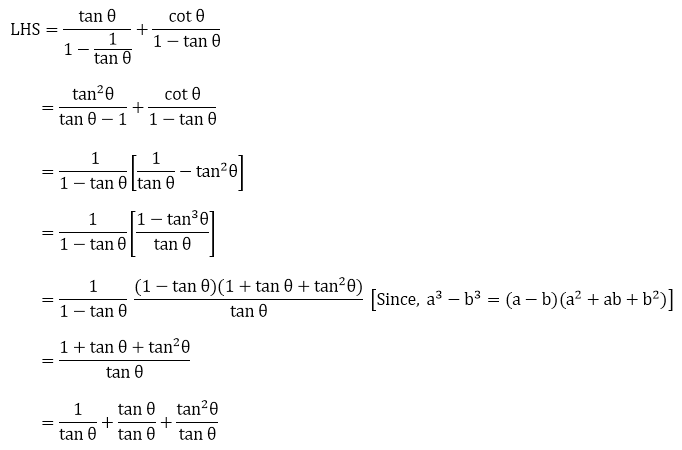
= 1 + tan θ + cot θ

= R.H.S.

– Hence, proved.

31. sec⁶ θ = tan⁶ θ + 3 tan² θ sec² θ + 1

Solution: 

From trig. identities we have,

sec² θ − tan² θ = 1

On cubing both sides,

(sec²θ − tan²θ)³ = 1

sec⁶ θ − tan⁶ θ − 3sec² θ tan² θ(sec² θ − tan² θ) = 1

sec⁶ θ − tan⁶ θ − 3sec² θ tan² θ = 1

⇒ sec⁶ θ = tan⁶ θ + 3sec² θ tan² θ + 1

L.H.S. = R.H.S.

Hence, proved.

32. cosec⁶ θ = cot⁶ θ + 3cot² θ cosec² θ + 1

Solution:

From trig. identities we have,

cosec² θ − cot² θ = 1

On cubing both sides,

(cosec² θ − cot² θ)³ = 1

cosec⁶ θ − cot⁶ θ − 3cosec² θ cot² θ (cosec² θ − cot² θ) = 1

cosec⁶ θ − cot⁶ θ − 3cosec² θ cot² θ = 1

⇒ cosec⁶ θ = cot⁶ θ + 3 cosec² θ cot² θ + 1

L.H.S. = R.H.S.

Hence, proved.

33.

Solution:

Taking L.H.S. and using sec² θ − tan² θ = 1 ⇒ 1 + tan² θ = sec² θ

= R.H.S.

– Hence, proved.

34.

Solution:

Taking L.H.S. and using the identity sin²A + cos²A = 1, we get

sin²A = 1 − cos²A

⇒ sin²A = (1 – cos A)(1 + cos A)

Hence, proved.

35.

Solution:

We have,

Rationalising the denominator and numerator with (sec A + tan A) and using sec² θ − tan² θ = 1, we get

= R.H.S.

– Hence, proved.

36.

Solution:

We have,

On multiplying the numerator and denominator by (1 – cos A), we get

= R.H.S.

– Hence, proved.

37. (i)

Solution:

Taking L.H.S. and rationalising the numerator and denominator with √(1 + sin A), we get

= R.H.S.

Hence, proved.

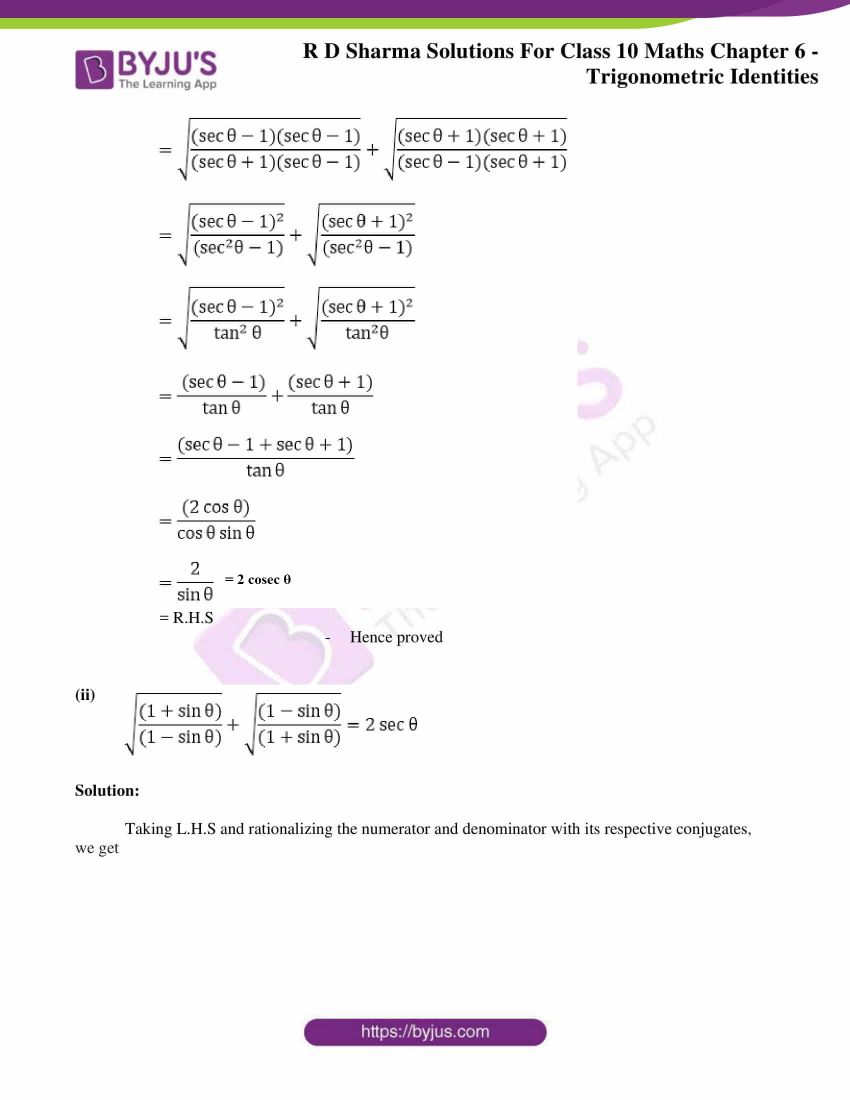
(ii)

Solution:

Taking L.H.S. and rationalising the numerator and denominator with their respective conjugates, we get

= 2 cosec A

= R.H.S.

– Hence, proved.

38. Prove that:

(i)

Solution:

Taking L.H.S. and rationalising the numerator and denominator with their respective conjugates, we get

= 2 cosec θ

= R.H.S.

– Hence, proved.

(ii)

Solution:

Taking L.H.S. and rationalising the numerator and denominator with their respective conjugates, we get

= R.H.S.

– Hence, proved.

(iii)

Solution:

Taking L.H.S. and rationalising the numerator and denominator with their respective conjugates, we get

= 2 cosec θ

= R.H.S.

– Hence, proved.

(iv)

Solution:

Taking L.H.S., we have

= R.H.S.

– Hence, proved.

39.

Solution:

Taking LHS = (sec A – tan A)², we have

= R.H.S.

– Hence, proved.

40.

Solution:

Taking L.H.S. and rationalising the numerator and denominator with (1 – cos A), we get

= (cosec A – cot A)²

= (cot A – cosec A)²

= R.H.S.

– Hence, proved.

41.

Solution:

Considering L.H.S. and taking L.C.M. and on simplifying, we have,

= 2 cosec A cot A = RHS

Hence, proved.

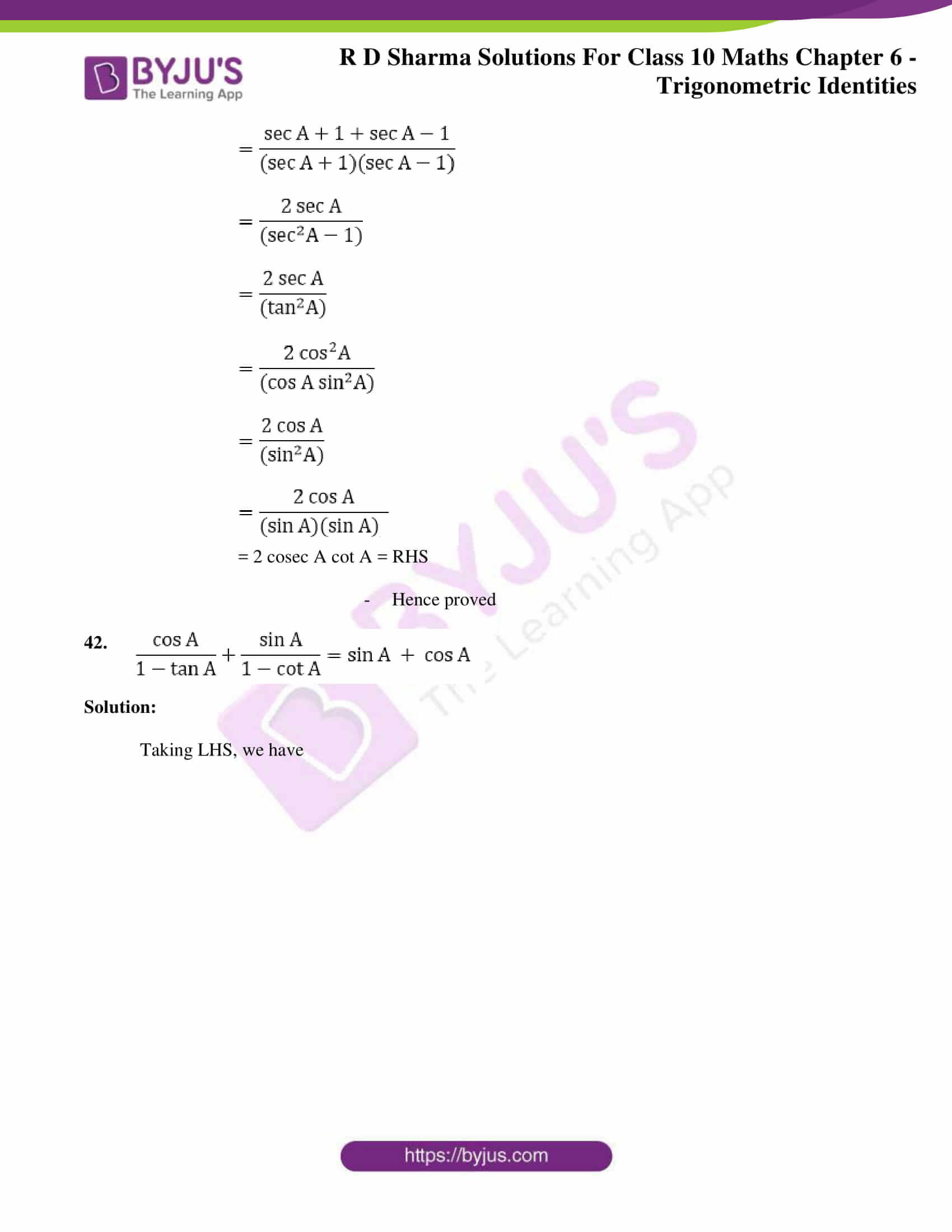
42.

Solution:

Taking LHS, we have

= cos A + sin A

= RHS

– Hence, proved.

43.

Solution:

Considering L.H.S. and taking L.C.M. and on simplifying, we have,

= 2 sec² A

= RHS

Hence, proved.

RD Sharma Solutions for Class 10 Maths Chapter 6 Exercise 6.2 Page No: 6.54

1. If cos θ = 4/5, find all other trigonometric ratios of angle θ. 

Solution:

We have,

cos θ = 4/5

And we know that,

sin θ = √(1 – cos² θ)

⇒ sin θ = √(1 – (4/5)²)

= √(1 – (16/25))

= √[(25 – 16)/25]

= √(9/25)

= 3/5

∴ sin θ = 3/5

Since, cosec θ = 1/ sin θ

= 1/ (3/5)

⇒ cosec θ = 5/3

And, sec θ = 1/ cos θ

= 1/ (4/5)

⇒ cosec θ = 5/4

Now,

tan θ = sin θ/ cos θ

= (3/5)/ (4/5)

⇒ tan θ = 3/4

And, cot θ = 1/ tan θ

= 1/ (3/4)

⇒ cot θ = 4/3

2. If sin θ = 1/√2, find all other trigonometric ratios of angle θ.

Solution:

We have,

sin θ = 1/√2

And we know that,

cos θ = √(1 – sin² θ)

⇒ cos θ = √(1 – (1/√2)²)

= √(1 – (1/2))

= √[(2 – 1)/2]

= √(1/2)

= 1/√2

∴ cos θ = 1/√2

Since, cosec θ = 1/ sin θ

= 1/ (1/√2)

⇒ cosec θ = √2

And, sec θ = 1/ cos θ

= 1/ (1/√2)

⇒ sec θ = √2

Now,

tan θ = sin θ/ cos θ

= (1/√2)/ (1/√2)

⇒ tan θ = 1

And, cot θ = 1/ tan θ

= 1/ (1)

⇒ cot θ = 1

3.

Solution:

Given,

tan θ = 1/√2

By using sec² θ − tan² θ = 1,

4.

Solution:

Given,

tan θ = 3/4

By using sec² θ − tan² θ = 1,

sec θ = 5/4

Since, sec θ = 1/ cos θ

⇒ cos θ = 1/ sec θ

= 1/ (5/4)

= 4/5

So,

5. 

Solution:

Given, tan θ = 12/5

Since, cot θ = 1/ tan θ = 1/ (12/5) = 5/12

Now, by using cosec² θ − cot² θ = 1,

cosec θ = √(1 + cot² θ)

= √(1 + (5/12)² )

= √(1 + 25/144)

= √(169/ 25)

⇒ cosec θ = 13/5

Now, we know that

sin θ = 1/ cosec θ

= 1/ (13/5)

⇒ sin θ = 5/13

Putting the value of sin θ in the expression, we have,

= 25/ 1

= 25

6.

Solution:

Given,

cot θ = 1/√3

Using cosec² θ − cot² θ = 1, we can find cosec θ

cosec θ = √(1 + cot² θ)

= √(1 + (1/√3)²)

= √(1 + (1/3)) = √((3 + 1)/3)

= √(4/3)

⇒ cosec θ = 2/√3

So, sin θ = 1/ cosec θ = 1/ (2/√3)

⇒ sin θ = √3/2

And we know that

cos θ = √(1 – sin² θ)

= √(1 – (√3/2)²)

= √(1 – (3/4))

= √((4 – 3)/4)

= √(1/4)

⇒ cos θ = 1/2

Now, using cos θ and sin θ in the expression, we have

= 3/5

7.

Solution:

Given,

cosec A = √2

Using cosec² A − cot² A = 1, we find cot A

= 4/2

= 2