1
Question
In how many of the the distinct permutations of the letters in MISSISSIPPI do the four I's not come together?
Open in App
Solution
The word MISSISSIPPI has one M, four I’s, four S’s, two P’s and a total of 11 letters.
The number of all type of arrangements possible with the given alphabets
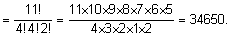
Let us first find the case when all the I’s together and so take it as one packet or unit. So now we have one M, one unit of four I’s, four S’s, two P’s and a total of 8 units.
Therefore the number of arrangements possible when all the I’s is together
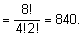
Hence, the distinct permutations of the letters of the word MISSISSIPPI when four I’s do not come together = 34650 – 840 = 33810.
The word MISSISSIPPI has one M, four I’s, four S’s, two P’s and a total of 11 letters.
The number of all type of arrangements possible with the given alphabets
![]()
Let us first find the case when all the I’s together and so take it as one packet or unit. So now we have one M, one unit of four I’s, four S’s, two P’s and a total of 8 units.
Therefore the number of arrangements possible when all the I’s is together
![]()
Hence, the distinct permutations of the letters of the word MISSISSIPPI when four I’s do not come together = 34650 – 840 = 33810.
Suggest Corrections
14
