Let K be the set of all real values of x where the function fx-sin -x-x-2x- -cos-x- is not differentiable- Then the set K is equal to-

The correct option is C ϕ (an empty set) Differentiability Let #160; f(x) be a real valued function defined on an open interval (a, b) and #160; # ...

You visited us 3 times! Enjoying our articles? Unlock Full Access!

Byju's Answer

Standard XII

Mathematics

Graphical Interpretation of Differentiability

Let K be th...

Question

Let $K$ be the set of all real values of $x$ where the function $f (x) = s i n | x | - | x | + 2 (x - π) c o s | x |$ is not differentiable. Then the set $K$ is equal to:

{π}

No worries! We‘ve got your back. Try BYJU‘S free classes today!

{0}

No worries! We‘ve got your back. Try BYJU‘S free classes today!

ϕ (a n e m p t y s e t)

Right on! Give the BNAT exam to get a 100% scholarship for BYJUS courses

{0, π}

No worries! We‘ve got your back. Try BYJU‘S free classes today!

Open in App

Solution

The correct option is C $ϕ (a n e m p t y s e t)$
Differentiability -
Let f(x) be a real valued function defined on an open interval (a, b) and $x\epsilon$ (a, b).Then the function f(x) is said to be differentiable at $x_{\circ }$ if
$\lim_{h\rightarrow 0}\:\frac{f(x_{0}+h)-f(x_{0})}{(x_{0}+h)-x_{0}}$

$or\:\:\:\lim_{x\rightarrow x_{0}}\:\frac{f(x)-f(x_{0})}{x-x_{0}}$
-
Checking differentiability at x=0
for x>0,
$f(x)=\sin x-x+2(x-\pi)\cos x$
$f{}'(x)=\cos x-1+2\cos x-2(x-\pi)\sin x$
RHD = $f{}'(0+)=1-1+2-2(-\pi)\cdot 0=2$
for x<0,
$f(x)=-\sin x+x+2(x-\pi)\cos x$
$f{}'(x)=-\cos x+1+2\cos x-2(x-\pi)\sin x$
LHD = $f{}'(0-)=-1+1+2-2(-\pi)\cdot 0=2$
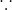$\because$ LHD = RHD
differentiable at x = 0 => differentiable everywhere

So, option 2 correct

Suggest Corrections

Similar questions

Q. Let

K

be the set of all real values of

x

where the function

f (x) = sin | x | - | x | + 2 (x - π) cos | x |

is not differentiable. Then the set

K

is equal to :

Q. Let

K

be the set of all real values of

x

where the function

f (x) = sin | x | - | x | + 2 (x - π) cos | x |

is not differentiable. Then the set

K

is equal to :

Q. Let

S

be the set of all points where the function

f (x) = | x - π | (e^{| x |} - 1) sin | x |

is not differentiable, then

S

Q. Let

S

be the set of points where the function,

f (x) = ∣ ∣ 2 - | x - 3 | ∣ ∣, x \in R

, is not differentiable. Then the value of

\sum x \in S f (f (x))

is equal to

Q. Let

{y}

and

[y]

denote fractional part function and greatest integer function respectively.
If

f (x) = {sin}^{- 1} {[3 x + 2] - {3 x + (x - {2 x})}}

for

x \in (0, \frac{π}{12})

and

(g \circ f) (x) = x

for all

x \in (0, \frac{π}{12}),

then

g^{'} (\frac{π}{6})

is equal to

Join BYJU'S Learning Program

Let K be the set of all real values of x where the function f(x)=sin|x|−|x|+2(x−π)cos|x| is not differentiable. Then the set K is equal to:

Let $K$ be the set of all real values of $x$ where the function $f (x) = s i n | x | - | x | + 2 (x - π) c o s | x |$ is not differentiable. Then the set $K$ is equal to: