1
Question
The solution of the equation [sinx+cosx]1+sin2x=2, −π≤x≤π is
The solution of the equation [sinx+cosx]1+sin2x=2, −π≤x≤π is
Open in App
Solution
The correct option is C 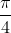
Let I=[sinx+cosx]1+sin2x=[√2sin(π4+x)]1+sin2x
At, x=π4,
I=[√2sin(π4+π4)]1+sin2π4
=(√2)2=2
Here, option (c) is correct.
Let I=[sinx+cosx]1+sin2x=[√2sin(π4+x)]1+sin2x
At, x=π4,
I=[√2sin(π4+π4)]1+sin2π4
=(√2)2=2
Here, option (c) is correct.
