Two coherent narrow slits emitting the sound of wavelength λ in the same phase are placed parallel to each other at a small separation of The sound is detected by moving a detector on the screen ∑ at a distance from the slit as shown in figure (below). Find the distance such that the intensity at P is equal to the intensity at O.

Two coherent narrow slits emitting the sound of wavelength λ in the same phase are placed parallel to each other at a small separation of The sound is detected by moving a detector on the screen ∑ at a distance from the slit as shown in figure (below). Find the distance such that the intensity at P is equal to the intensity at O.

Step 1: Given data:
Two coherent narrow slits S1 and S2 are given to emit the sound of wavelength
The separation between the two slits is given as=
The distance at which the sound is detected by moving a detector on the screen ∑ from the slit is given as
Step 2: Calculating the maximum intensity at P:
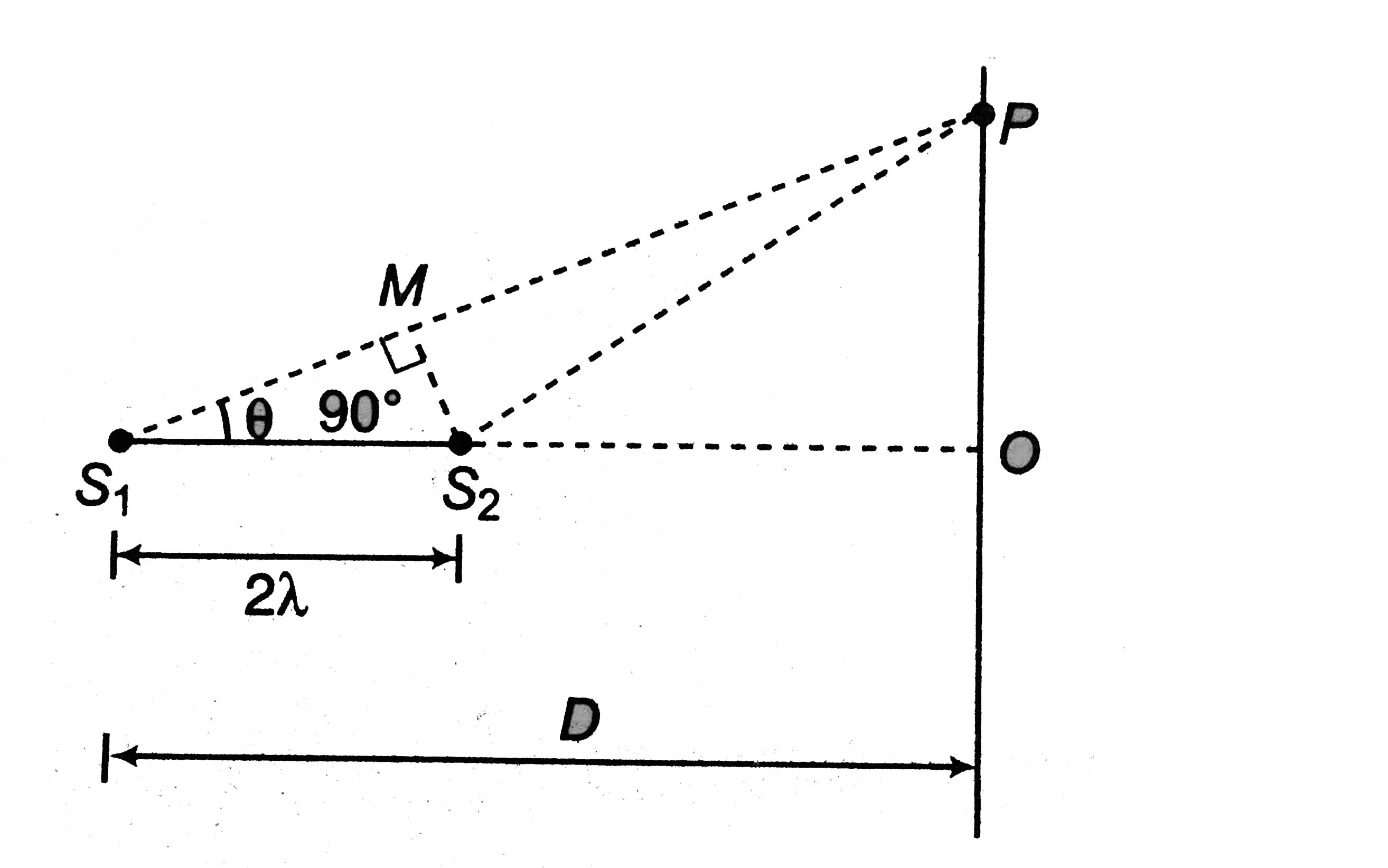
S1 and S2 are given in to be the same phase, At O, there will be maximum intensity.
Hence the maximum intensity at P, can be calculated as:
From right-angled triangles,
If λ is small, then λ2 is negligible.
For constructive interference, path difference is .
Step 3: Calculating distance at which the intensity at P is equal to the intensity at O:
When
When
Thus, when, the intensity at P will be equal to the intensity at O.
Hence, the distance at which the intensity at P is equal to the intensity at O is given as .
Step 1: Given data:
Two coherent narrow slits S1 and S2 are given to emit the sound of wavelength
The separation between the two slits is given as=
The distance at which the sound is detected by moving a detector on the screen ∑ from the slit is given as
Step 2: Calculating the maximum intensity at P:

S1 and S2 are given in to be the same phase, At O, there will be maximum intensity.
Hence the maximum intensity at P, can be calculated as:
From right-angled triangles,
If λ is small, then λ2 is negligible.
For constructive interference, path difference is .
Step 3: Calculating distance at which the intensity at P is equal to the intensity at O:
When
When
Thus, when, the intensity at P will be equal to the intensity at O.
Hence, the distance at which the intensity at P is equal to the intensity at O is given as .

