Why sinx is projection of OP on y axis and why cosx is projection of OP on x axis?
Why sinx is projection of OP on y axis and why cosx is projection of OP on x axis?
We begin by looking at a right angled triangle where the hypotenuse has a length of 1 unit.
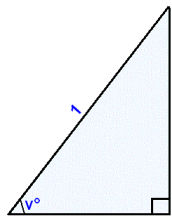
Opposite side = sin v°
Adjacent side = cos v°
In a right angled triangle
sin v° = opposite side/hypotenuse
and
cos v° = adjacent side/hypotenuse.
If the hypotenuse in a triangle has length 1 then it follows that
sin v° = opposite side and cos v° = adjacent side.
We now consider a circle drawn in a coordinate system.
A circle with a radius of 1 unit and it’s centre in (0, 0) is called the Unit circle.
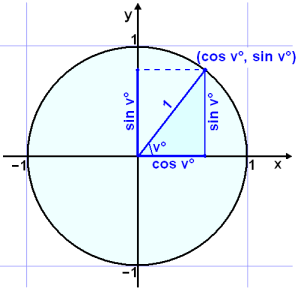The Unit circle
If we draw a radius that makes an angle of v° with the positive arm of the x axis and drop a perpendicular as the diagram shows we get a right angled triangle with sides of length cosv° and sin v°. This means that the coordinates of the point where the radius intersects the circle must be (cos v°, sin v°).
We begin by looking at a right angled triangle where the hypotenuse has a length of 1 unit.
| Opposite side = sin v° |
| Adjacent side = cos v° |
In a right angled triangle
sin v° = opposite side/hypotenuse
and
cos v° = adjacent side/hypotenuse.
If the hypotenuse in a triangle has length 1 then it follows that
sin v° = opposite side and cos v° = adjacent side.
We now consider a circle drawn in a coordinate system.
A circle with a radius of 1 unit and it’s centre in (0, 0) is called the Unit circle.
The Unit circle
If we draw a radius that makes an angle of v° with the positive arm of the x axis and drop a perpendicular as the diagram shows we get a right angled triangle with sides of length cosv° and sin v°. This means that the coordinates of the point where the radius intersects the circle must be (cos v°, sin v°).
