1
Question
If the bisector of the vertical angle of a triangle bisects the base, prove that the triangle is isosceles.
If the bisector of the vertical angle of a triangle bisects the base, prove that the triangle is isosceles.
Open in App
Solution
Prove that the triangle is isosceles
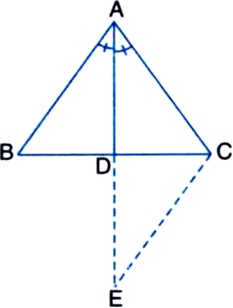
Given, in is a bisector of and meet base at , such that
Construction: Produce to meet such that
Now, in and
Thus by CPCT rule,
and
Also,
In
Hence, is isosceles
Prove that the triangle is isosceles

Given, in is a bisector of and meet base at , such that
Construction: Produce to meet such that
Now, in and
Thus by CPCT rule,
and
Also,
In
Hence, is isosceles
Suggest Corrections
65
