Linear Equations

Linear equations are those equations that are of first order. Linear equations are also first-degree equations as it has highest exponent of variables as 1.  Some of the examples of linear equations are as follows:

• 2x – 3 = 0, 
• 2y = 8
•  m + 1 = 0,
•  x/2 = 3
•  x + y = 2
• 3x – y + z = 3

When the equation has a homogeneous variable (i.e. only one variable), then this type of equation is known as a Linear equation in one variable.

Linear Equation Definition

What is a linear equation definition and example? An Equation having the maximum order of 1 is known as a Linear equation.

Below are some examples of linear equations in 1 variable, 2 variables and 3 variables:

There are different forms to write linear equations. Some of them are 

Where m = slope of a line; (x_0, y_0) intercept of x-axis and y-axis.

Standard Form 

Linear equations are the combination of constants and variables. 

The standard form of a linear equation in one variable is represented as

where, a ≠ 0, b ≠ 0 , x and y are the variables.

The standard form of a linear equation in two variables is represented as

The standard form of a linear equation in three variables is represented as

How to Solve Linear Equations

Solving Linear Equations in One Variable

Both sides of the equation are supposed to be balanced for solving a linear equation. Equality sign denotes that the expressions on either side of the ‘equal to’ sign are equal. Since the equation is balanced, for solving it certain mathematical operations are performed on both sides of the equation in a manner that it does not affect the balance of the equation. [Use BODMAS Rule]

For Example:

2x – 10/2 = 3(x – 1)

Step 1: Clear the fraction

2x – 5 = 3(x – 1)

Step 2: Simplify Both sides equations

 2x – 5 = 3x – 3

2x = 3x + 2

2x – 3x = 2

Step 3: Isolate x

x = -2

Solving Linear Equations in Two Variables

Solving Linear Equations having 2 variables, In mathematics, there are different methods. Following are some of them:

1. Method of substitution
2. Cross multiplication method
3. Method of elimination
4. Determinant methods

We must choose set of 2 equations to find the values of 2 variables. Such as ax + by + c = 0 and dx + ey + f = 0, also called a system of equations with two variables, where x and y are two variables and a, b, c, d, e, f are constants, and a, b, d and e are not be zero. Else single equation has an infinite number of solutions.

Solving Linear Equations in Three Variables

To solve Linear Equations having 3 variables, we need a set of 3 equations as given below to find the values of unknowns. Matrix method is one of the popular methods to solve system of linear equations with 3 variables.

a₁x + b₁ y + c₁z + d₁ = 0

a₂x + b₂ y + c₂ z + d₂ = 0 and

a₃x + b₃ y + c₃ z + d₃ = 0

Learn more on the related Topics:

• Application of linear equations
• Linear Equations in One variable 
• Linear Equations Two Variables
• Graphing Of Linear Equations
• Linear Equations In Two Variables Class 9

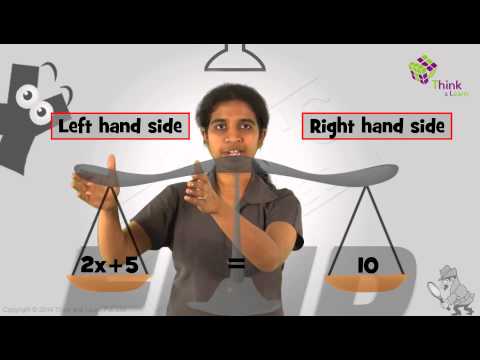
Linear Equations Example

Example 1: Solve x  = 12(x +2)

Solution: 

x  = 12(x  + 2)

x = 12x + 24

Subtract 24 from each side

x – 24 = 12x + 24 – 24

x – 24 = 12x

Simplify

11x  = -24

Isolate x, by dividing each side by 11

11x / 11 = -24/11

x = -24/11

Example 2: Solve x – y = 12 and 2x + y = 22

Solution: 

Name the equations

x – y = 12                        ———- (1)

2x + y = 22                       ———- (2)

Isolate Equation (1) for x,

x = y + 12

Substitute y + 12 for x in equation (2)

2(y+12) + y = 22

3y + 24 = 22

3y = -2

or y = -2/3

Substitute the value of y in x = y + 12

x = y + 12

x = -2/3 + 12

x = 34/3

Answer: x = 34/3 and y = -2/3