Square Root of 13

The square root of 13 is given by √13 in the radical form and as (13)^½ in exponential form. It is equal to 3.60555128. Since 3.60555128 is not a whole number but a fraction, therefore, 13 is not a perfect square. The square root of 13 is a value X, such that X² = 13. Since 13 is a prime number, we cannot determine its square root using the prime factorization method. But we can use here the long division method to find the square root of 13. Let us learn more in this article.

What is the Square Root of 13?

The square root of 13 is the number that can be multiplied by itself to get the original number. Since 13 is not a perfect square, therefore, its square root will not result in a whole number. As we know, square root is the reverse method of squaring a number. Let us say, x is a number that is squared to get the value equal to 13, such that x² = 13, then, √13 = x.

So, the square root of 13 is approximately equal to 3.60555128 because the square of 3.60555128 is equal to 13.

√13 = 3.60555128

How to Find the Square Root of 13?

Basically, we have two methods to find the square root of any number. But in the case of 13, we cannot use the prime factorisation method to find its square root.

Prime factorisation of 13 → 13

Thus, the only prime factor that can divide 13 is 13 itself. Hence, we cannot simplify it further. Let us move to another method to find its square root.

Square Root of 13 By Long Division Method

Before proceeding to find the square root, we can write 13 as 13.000000. It doesn’t make any difference in the number. Now we will use the long division method given as follows.

• Step 1: Pair the digits of 13.000000 from left to right such as 13.00 00 00
• Step 2: We need to think of a number that can be multiplied by itself to get a value less than or equal to the dividend 13. Thus, 3 is the divisor such that, 3 x 3 = 9.
• Step 3: Subtract 9 from 13 to get 4 and take down the next pair, 00, to make the new dividend equal to 400
• Step 4: Add 3 to the previous divisor. 3 + 3 = 6. Now, put a digit, say A, next to 6 such that 6A x A is less than or equal to 400. Hence, 66 x 6 = 396
• Step 5: Subtract 396 from 400 to get 4. Again, take down the next two zeros, so that 400 is the next dividend
• Step 6: Add 6 to the previous divisor, i.e.,66 to get 72. Think of a digit that can be placed next to 72, such that 72B x B is less than or equal to 400 (dividend). Hence, 720 x 0 = 000
• Step 7: Subtract 000 from 400 to get 400 again. Again, take down the next two zeros for the next division
• Step 8: Add 0 to the previous divisor such that, 720 + 0 = 720.
• Step 9: To divide 40000, we have to think of a digit next to 720 such that 720C x C is less than or equal to 40000. Hence, 7205 x 5 = 36025
• Step 10: Subtracting 36025 from 40000, we get 3975.
• Step 11: Now we have got the quotient value up to three decimal places, i.e., 3.605. We can repeat the above steps to get a more accurate value.

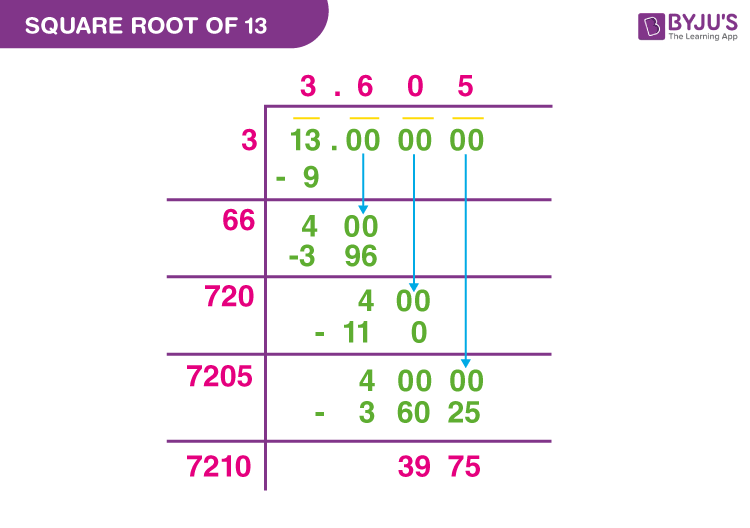
Therefore, the required square root of 13 is the quotient achieved in the above division method, i.e., √13 = 3.605, approximately.

Square Root of 13 – Rational or Irrational

• A rational number is a number that can be expressed as a ratio, such as P/Q, where Q is not equal to 0. It is usually a terminating or recurring decimal.
• An irrational number cannot be expressed as P/Q. It is a non-terminating and non-repeating decimal.
• The square root of 13 is a non-terminating and non-repeating decimal. Hence, it is an irrational number.

Related Articles

• Squares upto 50
• Square Root Tricks
• Squares and Square Roots
• Square Root of 10
• Square Root of 20
• Square Root of 120
• Square Root of 144
• Square Root of 289
• Square Root of 576

Video Lessons on Square Roots

Visualising square roots

Finding Square roots

Solved Examples

Q.1: What is the value of √13 + √100?

Solution: As we know;

√13 = 3.606

√100 = 10

Therefore,

√13 + √100

⇒ 3.606 + 10

⇒ 3.606 (up to three places of the decimal)

Q.2: What is the value of the square of root of 13 multiplied by 13?

Solution: Square of root of 13 = (√13)²

Since square root gets cancelled with square, thus,

(√13)²= 13

Now,

13 multiplied by 13 = 13 x 13 = 169

Q.3: If the length of the side of a square is √13 units, then what is the area of the square?

Solution: Given,

Length of side of square, s = √13 units

According to the formula,

Area of square = s²

A = (√13)²

A = 13 sq.units.

Therefore, the area of a square is 13 sq. units.

Practice Questions

1. Find the value of y if, √13 – y² = 0.
2. What is the value of √-13?
3. Find: √13 x 1/3.60555128 = ?