Scientific notation

Scientific notation is a form of presenting very large numbers or very small numbers in a simpler form. As we know, the whole numbers can be extended till infinity, but we cannot write such huge numbers on a piece of paper. Also, the numbers which are present at the millions place after the decimal needed to be represented in a simpler form. Thus, it is difficult to represent a few numbers in their expanded form. Hence, we use scientific notations. Also learn, Numbers In General Form.

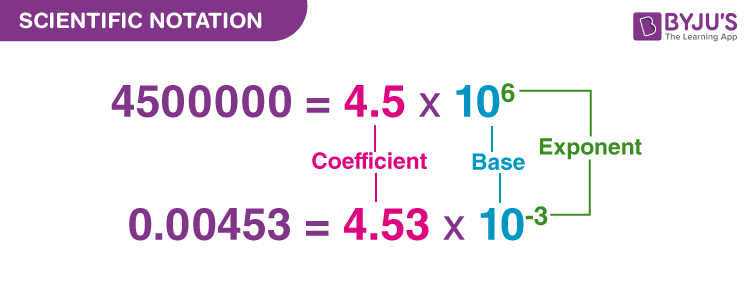
For example, 100000000 can be written as 10⁸, which is the scientific notation. Here the exponent is positive. Similarly, 0.0000001 is a very small number which can be represented as 10^-8, where the exponent is negative.

Scientific Notation Definition

As discussed in the introduction, the scientific notation helps us to represent the numbers which are very huge or very tiny in a form of multiplication of single-digit numbers and 10 raised to the power of the respective exponent. The exponent is positive if the number is very large and it is negative if the number is very small. Learn power and exponents for better understanding.

The general representation of scientific notation is:

Also, read:

• Scientific notation formula calculator
• Scientific Notation Calculator

Scientific Notation Rules

To determine the power or exponent of 10, we must follow the rule listed below:

• The base should be always 10
• The exponent must be a non-zero integer, that means it can be either positive or negative
• The absolute value of the coefficient is greater than or equal to 1 but it should be less than 10
•  Coefficients can be positive or negative numbers including whole and decimal numbers
• The mantissa carries the rest of the significant digits of the number

Let us understand how many places we need to move the decimal point after the single-digit number with the help of the below representation.

1. If the given number is multiples of 10 then the decimal point has to move to the left, and the power of 10 will be positive.
   Example: 6000 = 6 × 10³ is in scientific notation.
2. If the given number is smaller than 1, then the decimal point has to move to the right, so the power of 10 will be negative.
   Example: 0.006 = 6 × 0.001 = 6 × 10^-3 is in scientific notation.

Scientific Notation Examples

The examples of scientific notation are:
490000000 = 4.9×10⁸
1230000000 = 1.23×10⁹
50500000 = 5.05 x 10⁷
0.000000097 = 9.7 x 10^-8
0.0000212 = 2.12 x 10^-5

Positive and Negative Exponent

When the scientific notation of any large numbers is expressed, then we use positive exponents for base 10. For example:
20000 = 2 x 10⁴, where 4 is the positive exponent.

When the scientific notation of any small numbers is expressed, then we use negative exponents for base 10. For example:
0.0002 = 2 x 10^-4, where -4 is the negative exponent.

From the above, we can say that the number greater than 1 can be written as the expression with positive exponent, whereas the numbers less than 1 with negative exponent.

Problems and Solutions

Question 1: Convert 0.00000046 into scientific notation.

Solution: Move the decimal point to the right of 0.00000046 up to 7 places.

The decimal point was moved 7 places to the right to form the number 4.6

Since the numbers are less than 10 and the decimal is moved to the right. Hence, we use a negative exponent here.

⇒ 0.00000046 = 4.6 × 10^-7

This is the scientific notation.

Question 2: Convert 301000000 in scientific notation.

Solution: Move the decimal to the left 8 places so it is positioned to the right of the leftmost non zero digits 3.01000000. Remove all the zeroes and multiply the number by 10.

Now the number has become = 3.01.

Since the number is greater than 10 and the decimal is moved to left, therefore, we use here a positive exponent.

Hence, 3.01 × 10⁸ is the scientific notation of the number.

Question 3:Convert 1.36 × 10⁷ from scientific notation to standard notation.

Solution: Given, 1.36 × 10⁷ in scientific notation.

Exponent = 7

Since the exponent is positive we need to move the decimal place 7 places to the right.

Therefore,

1.36 × 10⁷ = 1.36 × 10000000 = 1,36,00,000.

Practice Questions

Problem 1: Convert the following numbers into scientific notation.

1. 28100000
2. 7890000000
3. 0.00000542

Problem 2: Convert the following into standard form.

1. 3.5 × 10⁵
2. 2.89 × 10^-6
3. 9.8 × 10^-2