To draw a straight line the minimum number of points required is two. Given any two points, a straight line can be drawn. How many minimum points are sufficient to draw a unique circle? Well, let’s try to find out.
Let us consider a point and try to draw circle passing through that point.
It can be seen that through a single point P infinite circles passing through it can be drawn.
Now, let us take two points P and Q and see what happens?
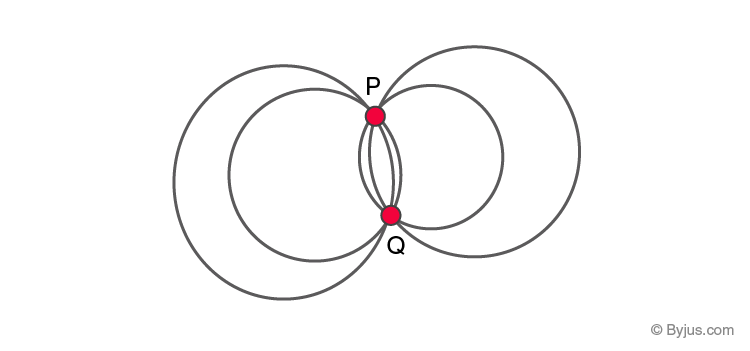
Again we see that an infinite number of circles passing through points P and Q can be drawn. Let us now take 3 points. For a circle passing through 3 points, two cases can arise.
- Three points can be collinear
- Three points can be non-collinear
Let us study both the cases individually.
- A circle passing through 3 points: Points are collinear
Consider three points P, Q and R which are collinear.
It can be seen that if three points are collinear any one of the points either lie outside the circle or inside it. Therefore, a circle passing through 3 points, where the points are collinear is not possible.
- A circle passing through 3 points: Points are non-collinear
To draw a circle through three non-collinear points join the points as shown:
Draw perpendicular bisectors of PQ and RQ. Let the bisectors AB and CD meet at O.
With O as the centre and radius OP or OQ or OR draw a circle. We get a circle passing through 3 point P, Q, and R.
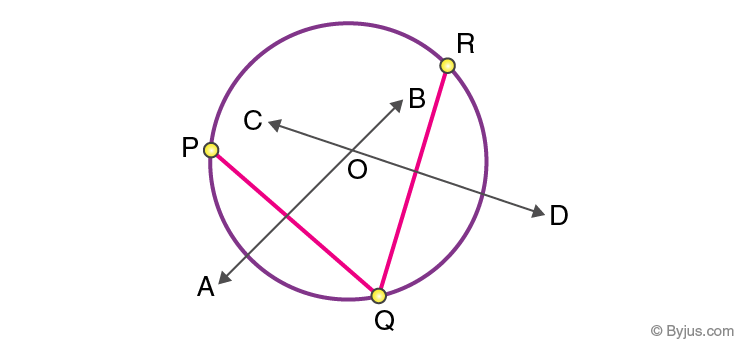
It is observed that only a unique circle will pass through all the three points. It can be stated as a theorem and the proof is explained as follows.
Given: Three non-collinear points A, B and C
To prove: One and only one circle can be drawn through A, B, and C
Construction: Join AB and BC. Draw perpendicular bisectors of AB and BC. Let these perpendicular bisectors meet at a point O.
Proof:
From above it follows that a unique circle passing through 3 points can be drawn given that the points are non-collinear. To know more about the area of a circle, equation of a circle, and its properties download BYJU’S-The Learning App.
Practise This Question
