Area of Triangle

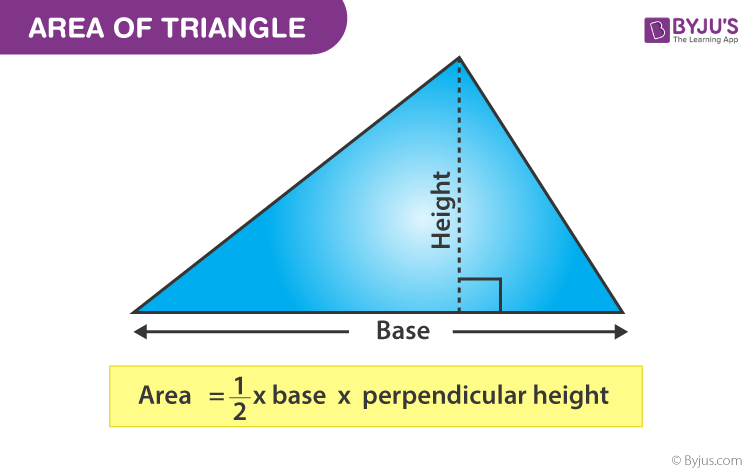
Area of a triangle is the region enclosed by it, in a two-dimensional plane. As we know, a triangle is a closed shape that has three sides and three vertices. Thus, the area of a triangle is the total space occupied within the three sides of a triangle. The general formula to find the area of the triangle is given by half of the product of its base and height.

In general, the term “area” is defined as the region occupied inside the boundary of a flat object or figure. The measurement is done in square units with the standard unit being square meters (m²). For the computation of area, there are predefined formulas for squares, rectangles, circles, triangles, etc. In this article, we will learn the area of triangle formulas for different types of triangles, along with some example problems.

What is the Area of a Triangle?

The area of a triangle is defined as the total region that is enclosed by the three sides of any particular triangle. Basically, it is equal to half of the base times height, i.e. A = 1/2 × b × h. Hence, to find the area of a tri-sided polygon, we have to know the base (b) and height (h) of it. It is applicable to all types of triangles, whether it is scalene, isosceles or equilateral. To be noted, the base and height of the triangle are perpendicular to each other. The unit of area is measured in square units (m², cm²).

Example: What is the area of a triangle with base b = 3 cm and height h = 4 cm?

Using the formula,

Area of a Triangle, A = 1/2 × b × h

= 1/2 × 4 (cm) × 3 (cm)

= 2 (cm) × 3 (cm)

= 6 cm²

Apart from the above formula, we have Heron’s formula to calculate the triangle’s area when we know the length of its three sides. Also, trigonometric functions are used to find the area when we know two sides and the angle formed between them in a triangle. We will calculate the area for all the conditions given here.

Area of a Triangle Formula

The area of the triangle  is given by the formula mentioned below:

where b and h are the base and height of the triangle, respectively.

Now, let’s see how to calculate the area of a triangle using the given formula. The area formulas for all the different types of triangles, like an area of an equilateral triangle, right-angled triangle, an isosceles triangle along with how to find the area of a triangle with 3 sides using Heron’s formula with examples are given below.

Area of a Right Angled Triangle

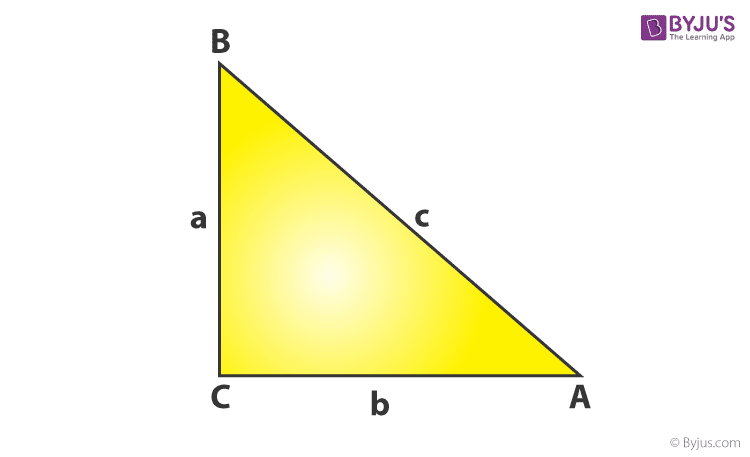
A right-angled triangle, also called a right triangle has any one angle equal to 90°. Therefore, the height of the triangle will be the length of the perpendicular side.

Area of a Right Triangle = A = ½ × Base × Height (Perpendicular distance)

From the above figure,

Area of triangle ACB = 1/2 × a × b

Area of an Equilateral Triangle

An equilateral triangle is a triangle where all the sides are equal. The perpendicular drawn from the vertex of the triangle to the base divides the base into two equal parts. To calculate the area of the equilateral triangle, we have to know the measurement of its sides.

• Area of an Equilateral Triangle = A = (√3)/4 × side²

Area of an Isosceles Triangle

An isosceles triangle has two of its sides equal and also the angles opposite the equal sides are equal.

Area of Triangle with Three Sides (Heron’s Formula)

The area of a triangle with 3 sides of different measures can be calculated using Heron’s formula. Heron’s formula includes two important steps. The first step is to find the semi perimeter of a triangle by adding all three sides of a triangle and dividing it by 2. The next step is to apply the semi-perimeter of triangle value in the main formula called “Heron’s Formula” to find the area of a triangle.

where, s is semi-perimeter of the triangle = s = (a+b+c) / 2

We have seen that the area of special triangles could be obtained using the triangle formula. However, for a triangle with the sides being given, the calculation of height would not be simple. For the same reason, we rely on Heron’s Formula to calculate the area of the triangles with unequal lengths.

Area of a Triangle Given Two Sides and the Included Angle  (SAS)

Now, the question comes, when we know the two sides of a triangle and an angle included between them, then how to find its area.

Let us take a triangle ABC, whose vertex angles are ∠A, ∠B, and ∠C, and sides are a,b and c, as shown in the figure below.

Now, if any two sides and the angle between them are given, then the formulas to calculate the area of a triangle is given by:

Area (∆ABC) = ½ bc sin A

Area (∆ABC) = ½ ab sin C

Area (∆ABC) = ½ ca sin B

These formulas are very easy to remember and also to calculate.

For example, If, in ∆ABC,  A = 30° and b = 2, c = 4 in units. Then the area will be;

Area (∆ABC) = ½ bc sin A

= ½ (2) (4) sin 30

= 4 x ½   (since sin 30 = ½)

= 2 sq.unit.

Related Articles

Area of a Triangle Solved Examples

Example 1:

Find the area of an acute triangle with a base of 13 inches and a height of 5 inches.

Solution:

A = (½)× b × h sq.units

⇒ A = (½) × (13 in) × (5 in)

⇒ A = (½) × (65 in²)

⇒ A = 32.5 in²

Example 2:

Find the area of a right-angled triangle with a base of 7 cm and a height of 8 cm.

Solution:

A = (½) × b × h sq.units

⇒ A = (½) × (7 cm) × (8 cm)

⇒ A = (½) × (56 cm²)

⇒ A = 28 cm²

Example 3:

Find the area of an obtuse-angled triangle with a base of 4 cm and a height 7 cm.

Solution:

A = (½) × b × h sq.units

⇒ A = (½) × (4 cm) × (7 cm)

⇒ A = (½) × (28 cm²)

⇒ A = 14 cm²