In continuity and differentiability class 12, we will learn important concepts of differentiability, continuity, and relationship between them. Also, other important concepts like the differentiation of inverse trigonometric functions and new functions like logarithmic and exponential functions. This article provides a brief introduction with example.
Continuity and Differentiability Class 12 Concepts
The topics and subtopics covered in continuity and differentiability class 12 are:
- Introduction
- Continuity
- Algebra of a continuous function
- Differentiability
- Derivatives of composite functions
- Derivatives of implicit functions
- Derivatives of inverse trigonometric functions
- Exponential and Logarithmic Functions
- Logarithmic Differentiation
- Derivatives of Functions in Parametric Forms
- Second-Order Derivative
- Mean Value Theorem
Continuity and Differentiability Class 12 Notes
Definition of Continuity:
(i) The continuity of a real function (f) on a subset of the real numbers is defined when the function exists at point c and is given as-
(ii) A real function (f) is said to be continuous if it is continuous at every point in the domain of f.
Consider a function f(x), and the function is said to be continuous at every point in [a, b] including the endpoints a and b.
Continuity of “f” at a means,
Continuity of “f” at b means,
Differentiability formula
Assume that if f is a real function and c is a point in its domain. The derivative of f at c is defined by 0
The derivative of a function f at c is defined by-
Theorem 1: Algebra of continuous functions:
If the two real functions, say f and g, are continuous at a real number c, then
(i) f + g is continuous at x=c.
(ii) f – g is continuous at x=c.
(iii) f. g is continuous at x=c.
(iv)f/g is continuous at x=c, (provided g(c) ≠ 0).
Theorem 2: Suppose f and g are real-valued functions such that (f o g) is defined at c. If g is continuous at c and if f is continuous at g (c), then (f o g) is continuous at c.
Theorem 3: If a function f is differentiable at a point c, then it is also continuous at that point.
Theorem 4 (Chain Rule): Let f be a real-valued function which is a composite of two functions u and v; i.e., f = v o u.
Suppose t = u(x) and if both dt/dx and dv/dt exist, we have df/dx = (dv/dt). (dt/dx)
Theorem 5:
(1) The derivative of ex with respect to x is ex; i.e., d/dx(ex) = ex.
(2) The derivative of log x with respect to x is 1/x.
i.e., d/dx(log x) =1/x.
Theorem 6 (Rolle’s Theorem): Let f : [a, b] → R be continuous on [a, b] and differentiable on (a, b), such that f(a) = f(b), where a and b are some real numbers. Then there exists some c in (a, b) such that f'(c) = 0.
Continuity and Differentiability Class 12 Example
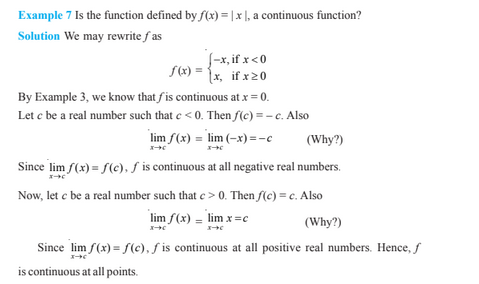
Video Lessons on Class 12 Important Calculus Questions


For more topics on class 12 concepts, register with BYJU’S – The Learning App for more examples and personalized videos to learn with ease.
| More Class 12 Concepts | |
| Linear Programming For class 12 | Matrices For Class 12 |
| Probability For Class 12 | Relations and Functions For Class 12 |
Comments