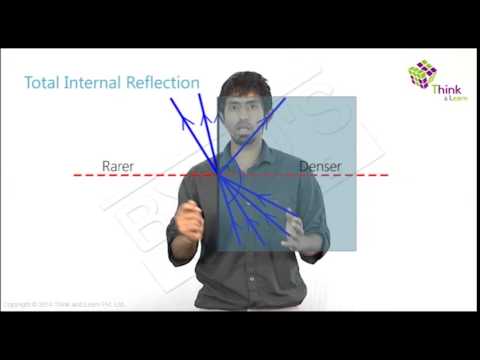
In Optics, The angle of incidence to which the angle of refraction is 90° is called the critical angle. The ratio of velocities of a light ray in the air to the given medium is a refractive index. Thus, the relation between the critical angle and refractive index can be established as the Critical angle is inversely proportional to the refractive index.
Critical Angle And Refractive Index
The relationship between critical angle and refractive index can be mathematically written as –
| \(SinC=\frac{1}{\mu _{b}^{a}}\) |
Where,
C is the critical angle.
μ is the refractive index of the medium.
a and b represent two medium in which light ray travels.
Critical Angle And Refractive Index Formula
| Formula | SI Unit | |
| Critical angle to Refractive index | \(SinC=\frac{1}{\mu _{b}^{a}}\) | degree |
| Refractive index to Critical angle | \(\mu _{b}^{a}=\frac{1}{sinC}\) | No SI unit |
Critical angle and refractive index relation derivation
The relationship between critical angle and refractive index can be derived as –
Consider a ray of light,
- Let the angle of incidence i be critical angle C
- Let the angle of refraction r=90º
- Refractive index of the rarer medium be μa
- Refractive index of the denser medium be μb
Applying Snells Law
- \(\frac{sin\;i}{sin\;r}=\frac{\mu _{a}}{\mu _{b}}\)
- \(\Rightarrow \mu _{b}sinC=\mu _{a}sin90^{0}\)
- \(\Rightarrow \frac{\mu _{b}}{\mu _{a}}=\frac{1}{sinC}\)
Thus, we arrive at formula expressing the critical angle and refractive index relation –
\(\mu _{b}^{a}=\frac{1}{sinC}\)Hope you understood the relation and conversion between the Critical Angle and Refractive Index in Optics.
Physics Related Topics:
| Optical density |
| Phase angle |
| Refraction and Dispersion of Light through a Prism |
| Polarisation by scattering |
Stay tuned to BYJU’S for more such interesting articles. Also, register to “BYJU’S-The Learning App” for loads of interactive, engaging physics-related videos and an unlimited academic assist.
