What is Electric Field Line?
A field line is drawn tangential to the net at a point. Thus at any point, the tangent to the electric field line matches the direction of the electric field at that point. Secondly, the relative density of field lines around a point corresponds to the relative strength (magnitude) of the electric field at that point. In other words, if you see more electric field lines in the vicinity of point A as compared to point B, then the electric field is stronger at point A.
Properties of Electric Field Lines
- The field lines never intersect each other.
- The field lines are perpendicular to the surface of the charge.
- The magnitude of charge and the number of field lines, both are proportional to each other.
- The start point of the field lines is at the positive charge and end at the negative charge.
- For the field lines to either start or end at infinity, a single charge must be used.
Electric Field Lines Attraction and Repulsion
Electric field lines always point away from a positive charge and towards a negative point. In fact, electric fields originate at a positive charge and terminate at a negative charge.
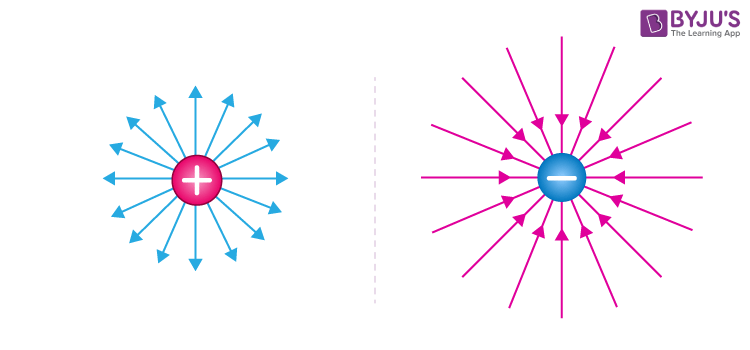
As said before field lines are a great way to visualize electric fields. You can almost feel the attraction between unlike charges and the repulsion between like charges as though they are trying to push each other away.
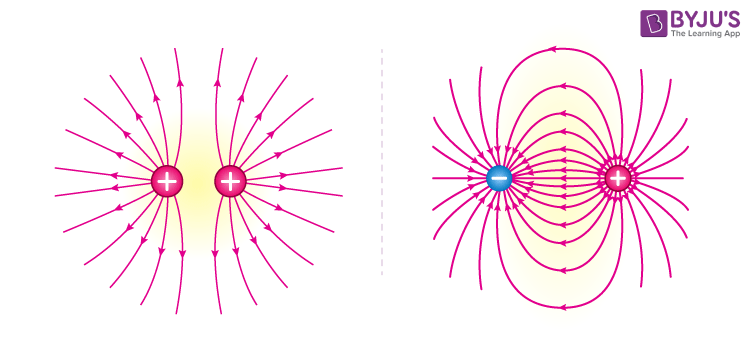
Rules for Drawing Electric Field Lines
Following are the rules for drawing electric field lines:
- The field line begins at the charge and ends either at the charge or at infinity.
- When the field is stronger, the field lines are closer to each other.
- The number of field lines depends on the charge.
- The field lines should never crossover.
- Electric field and electric field line are tangent at the point where they pass through.
Read more on electric fields by clicking on the link below
Recommended Video:
Stay tuned with BYJU’S to learn more about other concepts of Physics.
Frequently Asked Questions – FAQs
What is an electric field line?
What are the properties of electric field lines?
- The field lines are perpendicular to the surface of the charge.
- The magnitude of charge and the number of field lines, both are proportional to each other.
- The start point of the field lines is at the positive charge and ends at the negative charge.
- For the field lines to either start or end at infinity, a single charge must be used.
What are the rules for drawing electric field lines?
- The field line begins at the charge and ends either at the charge or at infinity.
- When the field is stronger, the field lines are closer to each other.
- The number of field lines depends on the charge.
- The field lines should never crossover.
- Electric field and electric field line are tangent at the point where they pass through.

Comments