In Physics, we often use the terms force, speed, velocity and work, and these quantities are classified as a scalar or vector quantities. A scalar quantity is a physical quantity with only magnitudes, such as mass and electric charge. On the other hand, a vector quantity is a physical quantity that has both magnitudes and directions like force and weight. In this article, let us familiarize ourselves with vectors and scalars.
|
Table of Contents |
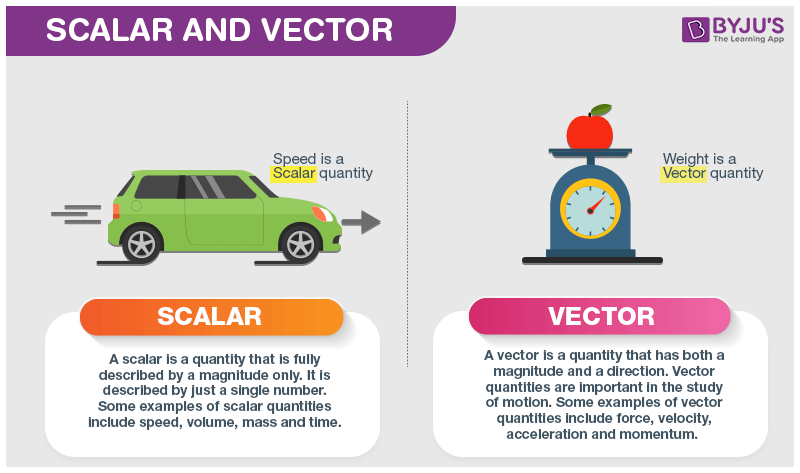
What Is a Scalar Quantity?
A scalar quantity is defined as the physical quantity with only magnitude and no direction. Such physical quantities can be described just by their numerical value without directions. The addition of these physical quantities follows the simple rules of algebra, and here, only their magnitudes are added.
Examples of Scalar Quantities
Some examples of scalar include:
- Mass
- Speed
- Distance
- Time
- Volume
- Density
- Temperature
What Is a Vector Quantity?
A vector quantity is defined as the physical quantity that has both directions as well as magnitude.
A vector with a value of magnitude equal to one is called a unit vector and is represented by a lowercase alphabet with a “hat” circumflex, i.e. “û“.
Visualise Unit Vector with the Help of the Video Given Below

Examples of Vector Quantities
Examples of vector quantity include:
- Linear momentum
- Acceleration
- Displacement
- Momentum
- Angular velocity
- Force
- Electric field
- Polarization
Difference Between Scalars and Vectors
The difference between Scalars and Vectors is crucial to understand in physics learning.
We have listed the various differences between a scalar and vector in the table below:
| Vector | Scalar | |
|---|---|---|
| Definition | A physical quantity with both the magnitude and direction. | A physical quantity with only magnitude. |
| Representation | A number (magnitude), direction using unit cap or arrow at the top and unit. | A number (magnitude) and unit |
| Symbol | Quantity symbol in bold and an arrow sign above | Quantity symbol |
| Direction | Yes | No |
| Example | Velocity and Acceleration | Mass and Temperature |
Vector Addition and Subtraction
After understanding what is a vector, let’s learn vector addition and subtraction. The addition and subtraction of vector quantities do not follow the simple arithmetic rules. A special set of rules are followed for the addition and subtraction of vectors. Following are some points to be noted while adding vectors:
- Addition of vectors means finding the resultant of a number of vectors acting on a body.
- The component vectors whose resultant is to be calculated are independent of each other. Each vector acts as if the other vectors were absent.
- Vectors can be added geometrically but not algebraically.
- Vector addition is commutative in nature, i.e., \(\begin{array}{l}\underset{A}{\rightarrow}+\underset{B}{\rightarrow}=\underset{B}{\rightarrow}+\underset{A}{\rightarrow}\end{array} \)
Now, about vector subtraction, it is the same as adding the negative of the vector to be subtracted. To better understand, let us look at the example given below.
Let us consider two vectors, A and B, as shown in the figure below. We need to subtract vector B from vector A. It is just the same as adding vector B and vector A. The resultant vector is shown in the figure below.

Vector Notation
For vector quantity usually, an arrow is used on the top as shown below, which represents the vector value of the velocity and also explains that the quantity has both magnitudes as well as direction.
Similar Reading:
| Triangle Law of Vector Addition | Scalar And Vector Products |
| Position And Displacement Vectors | Resolution Of A Vector In A Plane – Rectangular Components |
Scalar and Vector Solved Problems
Q1: Given below is a list of quantities. Categorize each quantity as being either a vector or a scalar.
| 20 degrees Celsius |
| 5 mi, North |
| 256 bytes |
| 5 m |
| 30 m/sec, East |
| 4000 Calories |
Answer:
| 20 degrees Celsius | Scalar |
| 5 mi, North | Vector |
| 256 bytes | Scalar |
| 5 m | Scalar |
| 30 m/sec, East | Vector |
| 4000 Calories | Scalar |
Q2: Ashwin walks 10 m north, 12 m east, 3 m west and 5 m south and then stops to drink water. What is the magnitude of his displacement from his original point?
Answer: We know that displacement is a vector quantity; hence the direction Ashwin walks will be positive or negative along an axis.
To find the total distance travelled along the y-axis, let us consider the movement towards the north to be positive and the south to be negative.
He moved a net of 5 meters to the north along the y-axis.
Similarly, let us consider his movement towards the east to be positive and the west to be negative.
He moved a net of 9 m to the east.
Using Pythagoras theorem, the resultant displacement can be found as follows:
Substituting the values, we get
Q3. What is the magnitude of a unit vector?
Answer: The magnitude of a unit vector is unity. A unit vector has no units or dimensions.
Frequently Asked Questions – FAQS
What is vector and scalar quantity in Physics?
How are vector and scalar different?
How are vectors and scalars quantities alike?
What are the examples of scalar?
What are the examples of vectors?
Stay tuned to BYJU’S and Fall in Loev with Learning!

Why Kinetic Energy is a scalar quantity while velocity is a vector quantity?
Kinetic energy is a scalar quantity because it doesn’t have a direction, unlike velocity. The kinetic energy of an object is completely described by magnitude alone.
its velocity square and the dot product of two vectors is a scalar quantity .hence,
kinetic energy isa scalar quantity
How weight is vector quantity
The gravitational acceleration is a vector quantity which has magnitude and direction. As a result, the weight of the object is a vector quantity because of its gravitational acceleration.
Why do we need vector and scalar?
Many quantities in Physics are vectors and when we try to add two vectors without considering their directions we usually end up with wrong results. Hence, vectors are important for accurate results.
Even though scalars signify just the magnitude, they are used all the time in Physics.
What is use in daily life of scalar and vector quantities
A scalar quantity describes only magnitude. Scalar quantities are everywhere, from the speed of a moving object to the energy of a nuclear power plant. On the other hand, vector quantities help to determine the direction or orientation of physical phenomena, processes or events. Many quantities, such as displacements, forces and velocity, will need to be represented as vectors for analysis
Scalar and vector quantities have different uses in daily life:
– Using a scalar quantity like temperature to regulate the heating or cooling of a room.
– Measuring the distance between two points using a scalar quantity like the length.
– Using a vector quantity like velocity to measure the speed of a moving object and its direction of motion.
– Navigating a route using vectors like displacement and direction to reach a destination.
motion, area & enthalpy are which quantities scaler/vector
Motion is too vague to represent a quantity of any kind. Motion is represented by velocity (vector) or speed (scalar) when the motion is restricted to one dimension. Other representations of motion include angular speed (scalar) and angular velocity (vector).
An area, as such, is a scalar quantity, but you can define a vector corresponding to that area, and that vector is called an area vector. An area vector is a vector whose magnitude is equal to the area of a plane and whose direction is normal to the plane of the area. In vector calculus and three-dimensional geometry, an area vector combines an area quantity with a direction, thus representing an oriented area in three dimensions.
Enthalpy is a scalar quantity because it does not possess any directions.