ML Aggarwal Solutions for Class 10 Maths Chapter 18 Trigonometric Identities

ML Aggarwal Solutions for Class 10 Maths Chapter 18 Trigonometric Identities is the best resource for students who are looking for reference material. It’s highly suggested that students use these solutions to solve problems as they are in accordance with the latest syllabus of the ICSE board. Students aiming to score well in the examinations can access the ML Aggarwal Solutions for Class 10 Maths Chapter 18 Trigonometric Identities PDF, which is available in the link given below.

Chapter 18 explains the proof of various trigonometric identities and solutions of trigonometric equations, which are crucial from the exam perspective. The solutions are created by subject experts at BYJU’S in order to help students prepare confidently for the board exams and other competitive exams at various levels. Referring to the PDF of ML Aggarwal Solutions will build a strong foundation over the concepts covered in this chapter.

ML Aggarwal Class 10 Maths Chapter 18 :

Download PDF

carouselExampleControls111

Previous



Next

Access ML Aggarwal Solutions for Class 10 Maths Chapter 18 Trigonometric Identities

Exercise 18

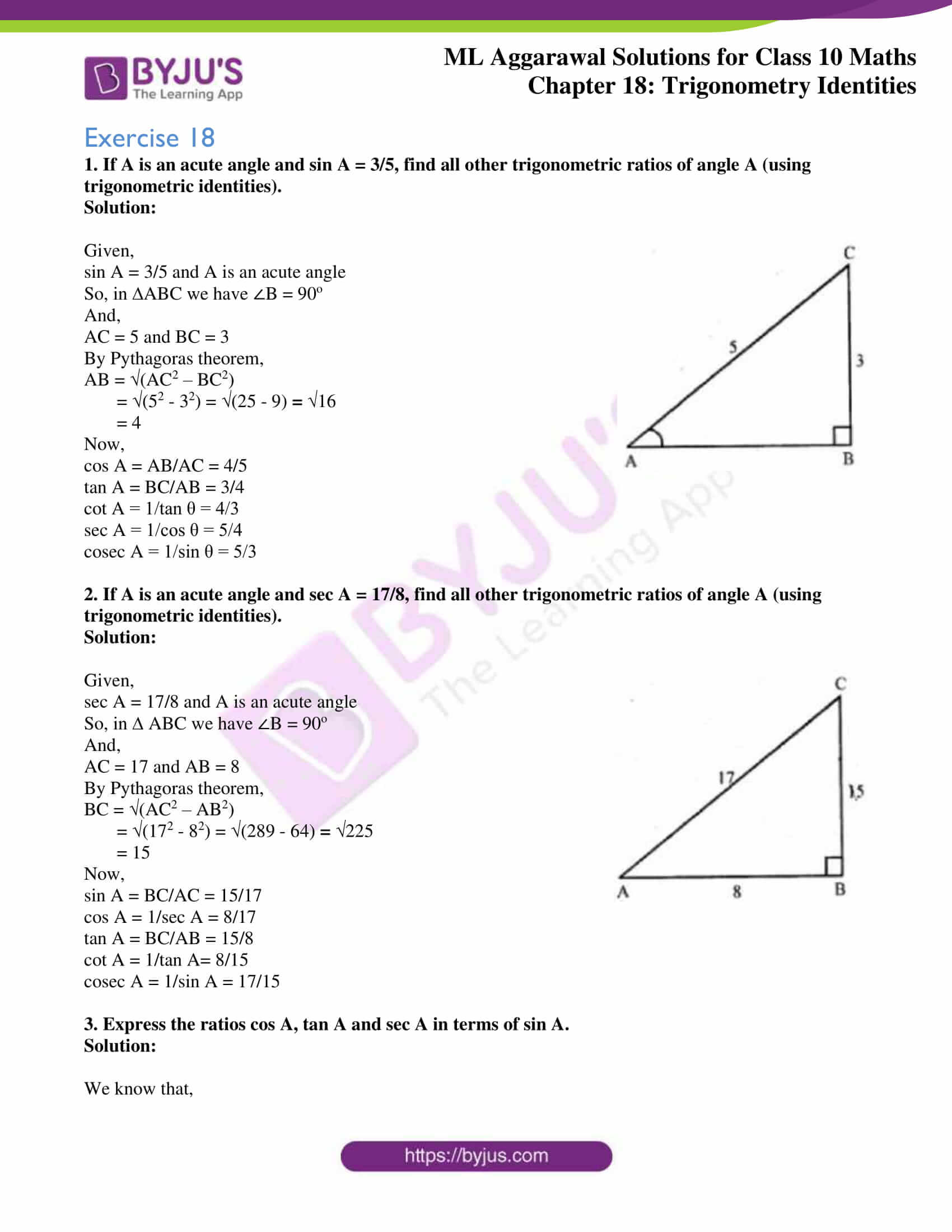
1. If A is an acute angle and sin A = 3/5, find all other trigonometric ratios of angle A (using trigonometric identities).

Solution:

Given,

sin A = 3/5 and A is an acute angle

So, in ∆ABC we have ∠B = 90^o

And,

AC = 5 and BC = 3

By Pythagoras theorem,

AB = √(AC² – BC²)

= √(5² – 3²) = √(25 – 9) = √16

= 4

Now,

cos A = AB/AC = 4/5

tan A = BC/AB = 3/4

cot A = 1/tan θ = 4/3

sec A = 1/cos θ = 5/4

cosec A = 1/sin θ = 5/3

2. If A is an acute angle and sec A = 17/8, find all other trigonometric ratios of angle A (using trigonometric identities).

Solution:

Given,

sec A = 17/8 and A is an acute angle

So, in ∆ ABC we have ∠B = 90^o

And,

AC = 17 and AB = 8

By Pythagoras theorem,

BC = √(AC² – AB²)

= √(17² – 8²) = √(289 – 64) = √225

= 15

Now,

sin A = BC/AC = 15/17

cos A = 1/sec A = 8/17

tan A = BC/AB = 15/8

cot A = 1/tan A= 8/15

cosec A = 1/sin A = 17/15

3. Express the ratios cos A, tan A and sec A in terms of sin A.

Solution:

We know that,

sin² A + cos² A = 1

So,

cos A = √(1 – sin² A)

tan A = sin A/cos A = sin A/ √(1 – sin² A)

sec A = 1/cos A = 1/ (√1 – sin² A)

4. If tan A = 1/√3, find all other trigonometric ratios of angle A.

Solution:

Given, tan A = 1/√3

In the right ∆ ABC,

tan A = BC/AB = 1/√3

So,

BC = 1 and AB = √3

By Pythagoras theorem,

AC = √(AB² + BC²) = √[(√3)² + (1)²]

= √(3 + 1) = √4 = 2

Hence,

sin A = BC/AC = ½

cos A = AB/AC = √3/2

cot A = 1/tan A = √3

sec A = 1/cos A = 2/√3

cosec A = 1/sin A = 2/1 = 2

5. If 12 cosec θ = 13, find the value of (2 sin θ – 3 cos θ)/ (4 sin θ – 9 cos θ)

Solution:

Given,

12 cosec θ = 13

⇒ cosec θ = 13/12

In right ∆ ABC,

∠A = θ

So, cosec θ = AC/BC = 13/12

AC = 13 and BC = 12

By Pythagoras theorem,

AB = √(AC² – BC²)

= √[(13)² – (12)²]

= √(169 – 144)

= √25

= 5

Now,

sin θ = BC/AC = 12/13

cos θ = AB/AC = 5/13

Hence,

Without using trigonometric tables, evaluate the following (6 to 10):

6. (i) cos² 26^o + cos 64^o sin 26^o + (tan 36^o/ cot 54^o)

(ii) (sec 17^o/ cosec 73^o) + (tan 68^o/ cot 22^o) + cos² 44^o + cos² 46^o

Solution:

Given,

(i) cos² 26^o + cos 64^o sin 26^o + (tan 36^o/ cot 54^o)

= cos² 26^o + cos (90^o – 16^o) sin 26^o + [tan 36^o/ cot (90^o – 54^o)]

= [cos² 26^o + sin² 26^o] + (tan 36^o/ tan 36^o)

= 1 + 1 = 2

(ii) (sec 17^o/ cosec 73^o) + (tan 68^o/ cot 22^o) + cos² 44^o + cos² 46^o

= [sec 17^o/ cosec (90^o – 73^o)] + [(tan 90^o – 22^o)/ cot 22^o] + cos² (90^o – 44^o) + cos² 46^o

= [sec 17^o/ sec 17^o] + [cot 22^o/ cot 22^o] + [sin² 46^o + cos² 46^o]

= 1 + 1 + 1

= 3

7. (i) (sin 65^o/ cos 25^o) + (cos 32^o/sin 58^o) – sin 28^o sec 62^o + cosec² 30^o

(ii) (sin 29^o/ cosec 61^o) + 2 cot 8° cot 17° cot 45° cot 73° cot 82° – 3(sin² 38° + sin² 52°).

Solution:

Given,

(i) (sin 65^o/ cos 25^o) + (cos 32^o/sin 58^o) – sin 28^o sec 62^o + cosec² 30^o

= (sin 65^o/ cos (90^o – 65^o)) + (cos 32^o/sin (90^o – 32^o)) – sin 28^o sec (90^o – 28^o) + 2²

= (sin 65^o/sin 65^o) + (cos 32^o/cos 32^o) – [sin 28^o x cosec 28^o] + 4

= 1 + 1 – 1 + 4

= 5

(ii) (sin 29^o/ cosec 61^o) + 2 cot 8° cot 17° cot 45° cot 73° cot 82° – 3(sin² 38° + sin² 52°).

= (sin 29^o/ cosec (90^o – 29^o)) + [2 cot 8° cot 17° cot 45° cot (90° – 17^o) cot (90^o – 8^o)] – 3(sin² 38° + sin² (90° – 38^o))

= (sin 29^o/ sin 29^o) + [2 cot 8° cot 17° cot 45° tan 17^o tan 8^o] – 3(sin² 38° + cos² 38°)

= 1 + 2[(cot 8° tan 8^o) (cot 17° tan 17^o) cot 45°] – 3(1)

= 1 + 2[1 x 1 x 1] – 3

= 1 + 2 – 3

= 0

8. (i) (sin 35^o cos 55^o + cos 35^o sin 55 ^o)/ (cosec² 10^o – tan² 80 ^o)

(ii) sin² 34^o + sin² 56^o + 2 tan18^o tan 72^o – cot² 30^o

Solution:

Given,

(i) (sin 35^o cos 55^o + cos 35^o sin 55 ^o)/ (cosec² 10^o – tan² 80 ^o)

(ii) sin² 34^o + sin² 56^o + 2 tan18^o tan 72^o – cot² 30^o

= sin² 34^o + sin² (90^o – 34^o) + 2 tan18^o tan (90^o – 18^o) – cot² 30^o

= [sin² 34^o + cos² 34^o] + 2 tan18^o cot 18^o – cot² 30^o

= 1 + 2 x 1 – (√3)²

= 1 + 2 – 3

= 0

9. (i) (tan 25^o/ cosec 65^o)² + (cot 25^o/ sec 65^o)² + 2 tan 18^o tan 45^o tan 75^o

(ii) (cos² 25^o + cos² 65^o) + cosec θ sec (90^o – θ) – cot θ tan (90^o – θ)

Solution:

Given,

(i) (tan 25^o/ cosec 65^o)² + (cot 25^o/ sec 65^o)² + 2 tan 18^o tan 45^o tan 75^o

(ii) (cos² 25^o + cos² 65^o) + cosec θ sec (90^o – θ) – cot θ tan (90^o – θ)

= cos² 25^o + cos² (90^o – 25^o) + cosec θ sec (90^o – θ) – cot θ. cot θ

= (cos² 25^o + sin² 25^o) + (cosec² θ – cot² θ)

= 1 + 1 = 2

10. (i) 2(sec² 35° – cot² 55°) – 

(ii)

Solution:

Given,

11. Prove that following: 

(i) cos θ sin (90° – θ) + sin θ cos (90° – θ) = 1

(ii) tan θ/ tan (90^o – θ) + sin (90^o – θ)/ cos θ = sec² θ

(iii) (cos (90^o – θ) cos θ)/ tan θ + cos² (90^o – θ) = 1

(iv) sin (90^o – θ) cos (90^o – θ) = tan θ/ (1 + tan² θ)

Solution:

(i) L.H.S. = cos θ sin (90° – θ) + sin θ cos (90° – θ)

= cos θ x cos θ + sin θ x sin θ

= cos² θ + sin² θ

= 1 = R.H.S.

(ii) L.H.S = tan θ/ tan (90^o – θ) + sin (90^o – θ)/ cos θ

= tan θ/ cot θ + cos θ/ cos θ

= tan θ/ (1/tan θ) + 1

= tan² θ + 1 = sec² θ = R.H.S.

(iii) L.H.S. = (cos (90^o – θ) cos θ)/ tan θ + cos² (90^o – θ)

= (sin θ cos θ)/ tan θ + sin² θ

= (sin θ cos θ)/ (sin θ/ cos θ) + sin² θ

= cos² θ + sin² θ

= 1 = R.H.S.

Prove that following (12 to 30) identities, where the angles involved are acute angles for which the trigonometric ratios as defined:

12. (i) (sec A + tan A) (1 – sin A) = cos A 

(ii) (1 + tan² A) (1 – sin A) (1 + sin A) = 1.

Solution:

Given,

(i) L.H.S = (sec A + tan A) (1 – sin A)

(ii) L.H.S. = (1 + tan² A) (1 – sin A) (1 + sin A)

13. (i) tan A + cot A = sec A cosec A 

(ii) (1 – cos A) (1 + sec A) = tan A sin A.

Solution:

(i) L.H.S. = tan A + cot A

= sin A/cos A + cos A/sin A

= (sin² A + cos² A)/ (sin A cos A)

= 1/ (sin A cos A)

= sec A cosec A

= R.H.S

(ii) L.H.S. = (1 – cos A) (1 + sec A)

14. (i) 1/ (1 + cos A) + 1/ (1 – cos A) = 2 cosec² A

(ii) 1/(sec A + tan A) + 1/(sec A – tan A) = 2 sec A

Solution:

(i) L.H.S. = 1/ (1 + cos A) + 1/ (1 – cos A)

(ii)

15. (i) sin A/ (1 + cos A) = (1 – cos A)/ sin A

(ii) (1 – tan² A)/ (cot² A – 1) = tan² A

(iii) sin A/ (1 + cos A) = cosec A – cot A

Solution:

(i) L.H.S. = sin A/ (1 + cos A)

On multiplying and dividing by (1 – cos A), we have

16. (i) (sec A – 1)/(sec A + 1) = (1 – cos A)/(1 + cos A)

(ii) tan² θ/ (sec θ – 1)² = (1 + cos θ)/ (1 – cos θ)

(iii) (1 + tan A)² + (1 – tan A)² = 2 sec² A

(iv) sec² A + cosec² A = sec² A. cosec² A

Solution:

(iii) L.H.S. = (1 + tan A)² + (1 – tan A)²

= 1 + 2 tan A + tan² A + 1 – 2 tan A + tan² A

= 2 + 2 tan² A

= 2(1 + tan² A) [As 1 + tan² A = sec² A]

= 2 sec² A

= R.H.S.

(iv) L.H.S = sec² A + cosec² A

= 1/cos² A + 1/sin² A

= (sin² A + cos² A)/ (sin² A cos² A)

= 1/ (sin² A cos² A)

= sec² A cosec² A = R.H.S

17. (i) (1 + sin A)/ cos A + cos A/ (1 + sin A) = 2 sec A

(ii) tan A/ (sec A – 1) + tan A/ (sec A + 1) = 2cosec A

Solution:

(i) L.H.S. = (1 + sin A)/ cos A + cos A/ (1 + sin A)

18. (i) cosec A/ (cosec A – 1) + cosec A/ (cosec A + 1) = 2 sec² A

(ii) cot A – tan A = (2cos² A – 1)/ (sin A – cos A)

(iii) (cot A – 1)/ (2 – sec² A) = cot A/ (1 + tan A)

Solution:

(i) L.H.S. =

19. (i) tan² θ – sin² θ = tan² θ sin² θ

(ii) cos θ/ (1 – tan θ) – sin² θ/ (cos θ – sin θ) = cos θ + sin θ

Solution:

(i) L.H.S = tan² θ – sin² θ

20. (i) cosec⁴ θ – cosec² θ = cot⁴ θ + cot² θ 

(ii) 2 sec² θ – sec⁴ θ – 2 cosec² θ + cosec⁴ θ = cot⁴ θ – tan⁴ θ.

Solution:

(i) L.H.S. = cosec⁴ θ – cosec² θ

= cosec² θ (cosec² θ – 1)

= cosec² θ cot² θ [cosec² θ – 1 = cot² θ]

= (cot² θ + 1) cot² θ

= cot⁴ θ + cot² θ

= R.H.S.

(ii) L.H.S. = 2 sec² θ – sec⁴ θ – 2 cosec² θ + cosec⁴ θ

= 2 (tan² θ + 1) – (tan² θ + 1)² – 2 (1 + cot² θ) + (1 + cot² θ)²

= 2 tan² θ + 2 – (tan⁴ θ + 2 tan² θ + 1) – 2 – 2 cot² θ + (1 + 2 cot² θ + cot⁴ θ)

= 2 tan² θ + 2 – tan⁴ θ – 2 tan² θ – 1 – 2 – 2 cot² θ + 1 + 2 cot² θ + cot⁴ θ

= cot⁴ θ – tan⁴ θ = R.H.S.

21. (i) = cot θ

(ii) (tan³ θ – 1)/ (tan θ – 1) = sec² θ + tan θ

Solution:

22. (i) (1 + cosec A)/ cosec A = cos² A/ (1 – sin A)

(ii)

Solution:

23. (i) = tan A + sec A

(ii) = cosec A – cot A

Solution:

24. (i) = 2 cosec A

(ii) cos A cot A/ (1 – sin A) = 1 + cosec A

Solution:

25. (i) (1 + tan A)/ sin A + (1 + cot A)/ cos A = 2 (sec A + cosec A)

(ii) sec⁴ A – tan⁴ A = 1 + 2 tan² A

Solution:

(ii) sec⁴ A – tan⁴ A = 1 + 2 tan² A

L.H.S. = sec⁴ A – tan⁴ A

= (sec² A – tan² A) (sec² A + tan² A)

= (1 + tan⁴ A – tan⁴ A) (1 + tan⁴ A + tan⁴ A) [As sec² A = tan⁴ A + 1]

= 1 (1 + 2 tan² A)

= 1 + 2 tan² A = R.H.S.

26. (i) cosec⁶ A – cot⁶ A = 3 cot² A cosec² A + 1 

(ii) sec⁶ A – tan⁶ A = 1 + 3 tan² A + 3 tan⁴ A

Solution:

(i) cosec⁶ A – cot⁶ A = 3 cot² A cosec² A + 1

27.

Solution:

(i)

= (cos θ – 1) (2 – 1 – cos θ)/ (cos θ – 1)

= 1 – cos θ

Hence, L.H.S = R.H.S.

28. (i) (sin θ + cos θ) (sec θ + cosec θ) = 2 + sec θ cosec θ 

(ii) (cosec A – sin A) (sec A – cos A) sec²A = tan A

Solution:

(i) (sin θ + cos θ) (sec θ + cosec θ) = 2 + sec θ cosec θ

L.H.S. = (sin θ + cos θ) (sec θ + cosec θ)

= R.H.S.

(ii)

29.

Solution:

30. (i) 1/ (sec A + tan A) – 1/cos A = 1/cos A – 1/(sec A – tan A)

(ii) (sin A + sec A)² + (cos A + cosec A)² = (1 + sec A cosec A)²

(iii) (tan A + sin A)/ (tan A – sin A) = (sec A + 1)/ (sec A – 1)

Solution:

31. If sin θ + cos θ = √2 sin (90° – θ), show that cot θ = √2 + 1

Solution:

Given, sin θ + cos θ = √2 sin (90° – θ)

sin θ + cos θ = √2 cos θ

On dividing by sin θ, we have

1 + cot θ = √2 cot θ

1 = √2 cot θ – cot θ

(√2 – 1) cot θ = 1

cot θ = 1/ (√2 – 1)

Hence, cot θ = √2 + 1

32. If 7 sin² θ + 3 cos² θ = 4, 0° ≤ θ ≤ 90°, then find the value of θ.

Solution:

Given,

7 sin² θ + 3 cos² θ = 4, 0° ≤ θ ≤ 90°

3 sin² θ + 3 cos² θ + 4 sin² θ = 4

3 (sin² θ + 3 cos² θ) + 4 sin² θ = 4

3 (1) + 4 sin² θ = 4

4 sin² θ = 4 – 3

sin² θ = ¼

Taking square-root on both sides, we get

sin θ = ½

Thus, θ = 30^o

33. If sec θ + tan θ = m and sec θ – tan θ = n, prove that mn = 1.

Solution:

Given,

sec θ + tan θ = m

sec θ – tan θ = n

Now,

mn = (sec θ + tan θ) (sec θ – tan θ)

= sec² θ – tan² θ = 1

Thus, mn = 1

34. If x – a sec θ + b tan θ and y = a tan θ + b sec θ, prove that x² – y² = a² – b².

Solution:

Given,

x =
a sec θ + b tan θ,

y = a tan θ + b sec θ

Now,

x² – y² = (a sec θ + b tan θ)² – (a tan θ + b sec θ)²

– Hence proved.

35. If x = h + a cos θ and y = k + a sin θ, prove that (x – h)² + (y – k)² = a².

Solution:

Given,

x = h + a cos θ

y = k + a sin θ

Now,

x – h = a cos θ

y – k = a sin θ

On squaring and adding, we get

(x – h)² + (y – k)² = a² cos² θ + a² sin² θ

= a² (sin² θ + cos² θ)

= a² (1) [Since, sin² θ + cos² θ = 1]

– Hence proved

Chapter Test

1. (i) If θ is an acute angle and cosec θ = √5, find the value of cot θ – cos θ. 

(ii) If θ is an acute angle and tan θ = 8/15, find the value of sec θ + cosec θ. 

Solution:

Given that θ is an acute angle and cosec θ = √5

So,

sin θ = 1/√5

And, cos θ = √(1 – sin² θ)

cos θ = √(1 – (1/√5)²)

= √(1 – (1/5))

= √(4/5)

cos θ = 2/√5

Now,

cot θ – cos θ = (cos θ/sin θ) – cos θ

(ii) Given, θ is an acute angle and tan θ = 8/15

In fig. we have

tan θ = BC/AB = 8/15

So, BC = 8 and AB = 15

By Pythagoras theorem, we have

AC = √(AB² + BC²) = √(5² + 8²) = √(25 + 64) = √289

⇒ AC = 17

Now,

sec θ = AC/AB = 17/15

cosec θ = AC/BC = 17/8

So,

sec θ + cosec θ = 17/15 + 17/8

= (136 + 255)/ 120

= 391/120

=

2. Evaluate the following: 

(i) 2 x () – tan 45^o + tan 13^o tan 23^o tan 30^o tan 67^o tan 77^o

(ii) + sin² 63^o + cos 63^o sin 27^o

Solution:

3. If 4/3 (sec² 59^o – cot² 31^o) – 2/3 sin 90^o + 3 tan² 56^o tan² 34^o = x/2, then find the value of x.

Solution:

Given,

4/3 (sec² 59^o – cot² 31^o) – 2/3 sin 90^o + 3 tan² 56^o tan² 34^o = x/2

4. (i) cos A/ (1 – sin A) + cos A/ (1 + sin A) = 2 sec A

(ii) cos A/ (cosec A + 1) + cos A/ (cosec A – 1) = 2 tan A

Solution:

5. (i)

(ii) (cosec θ – sin θ) (sec θ – cos θ) (tan θ + cot θ) = 1.

Solution:

6. (i) sin² θ + cos⁴ θ = cos² θ + sin⁴ θ 

(ii)

Solution:

Given,

(i) sin² θ + cos⁴ θ = cos² θ + sin⁴ θ

L.H.S. = sin² θ + cos⁴ θ

= (1 – cos² θ) + cos⁴ θ

= cos⁴ θ – cos² θ + 1

= cos² θ (cos² θ – 1) + 1

= cos² θ (- sin² θ) + 1

= 1 – sin² θ cos² θ

Now,

R.H.S. = cos² θ + sin⁴ θ

= (1 – sin² θ) + sin⁴ θ

= sin⁴ θ – sin² θ + 1

= sin² θ (sin² θ – 1) + 1

= sin² θ (-cos² θ) + 1

= 1 – sin² θ cos² θ

Hence, L.H.S. = R.H.S.

7. (i) sec⁴ A (1 – sin⁴ A) – 2 tan² A = 1 

(ii)  = sec A + cosec A

Solution:

(i) sec⁴ A (1 – sin⁴ A) – 2 tan² A = 1

L.H.S. = sec⁴ A (1 – sin⁴ A) – 2 tan² A

8. (i) + sin θ cos θ = 1

(ii) (sec A – tan A)² (1 + sin A) = 1 – sin A.

Solution:

9. (i) = sin A + cos A

(ii) (sec A – cosec A) (1 + tan A + cot A) = tan A sec A – cot A cosec A 

(iii)

Solution:

10.

Solution:

11. 2 (sin⁶ θ + cos⁶ θ) – 3 (sin⁴ θ + cos⁴ θ) + 1 = 0

Solution:

Given,

2 (sin⁶ θ + cos⁶ θ) – 3 (sin⁴ θ + cos⁴ θ) + 1 = 0

L.H.S. = 2 (sin⁶ θ + cos⁶ θ) – 3 (sin⁴ θ + cos⁴ θ) + 1

= 2 [(sin² θ)³ + (cos² θ)³] – 3 (sin⁴ θ + cos⁴ θ) + 1

= 2 [(sin² θ + cos² θ) (sin⁴ θ + cos⁴ θ – sin² θ cos² θ)] – 3 (sin⁴ θ + cos⁴ θ) + 1

= 2 (sin⁴ θ + cos⁴ θ – sin² θ cos² θ) – 3 (sin⁴ θ + cos⁴ θ) + 1

= 2 sin⁴ θ + 2 cos⁴ θ – 2 sin² θ cos² θ – 3 sin⁴ θ – 3 cos⁴ θ + 1

= 1 – sin⁴ θ – cos⁴ θ – 2 sin² θ cos² θ

= 1 – [sin⁴ θ + cos⁴ θ + 2 sin² θ cos² θ]

= 1 – 1

= 0 = R.H.S.

12. If cot θ + cos θ = m, cot θ – cos θ = n, then prove that (m² – n²)² = 16.

Solution:

Given,

cot θ + cos θ = m … (i)

cot θ – cos θ = n … (ii)

Adding (i) and (ii), we get

13. If sec θ + tan θ = p, prove that sin θ = (p² – 1)/ (p² + 1)

Solution:

Given, sec θ + tan θ = p

14. If tan A = n tan B and sin A = m sin B, prove that cos² A = (m² – 1)/ (n² – 1)

Solution:

Given,

tan A = n tan B and sin A = m sin B

n = tan A/ tan B

m = sin A/ sin B

15. If sec A = x + 1/4x, then prove that sec A + tan A = 2x or 1/2x

Solution:

Given, sec A = x + 1/4x

We know that,

16. When 0° < θ < 90°, solve the following equations: 

(i) 2 cos² θ + sin θ – 2 = 0 

(ii) 3 cos θ = 2 sin² θ 

(iii) sec² θ – 2 tan θ = 0 

(iv) tan² θ = 3 (sec θ – 1).

Solution:

Given, 0° < θ < 90°

(i) 2 cos² θ + sin θ – 2 = 0

2 (1 – sin² θ) + sin θ – 2 = 0

2 – 2 sin² θ + sin θ – 2 = 0

– 2 sin² θ + sin θ = 0

sin θ (1 – 2 sin θ) = 0

So, either sin θ = 0 or 1 – 2 sin θ = 0

If sin θ = 0

⇒ θ = 0^o

And, if 1 – 2 sin θ = 0

sin θ = ½

⇒ θ = 30^o

Thus, θ = 0^o or 30^o

(ii) 3 cos θ = 2 sin² θ

3 cos θ = 2 (1 – cos² θ)

3 cos θ = 2 – 2 cos² θ

2 cos² θ + 3 cos θ – 2 = 0

2 cos² θ + 4 cos θ – cos θ – 2 = 0

2 cos θ (cos θ + 2) – 1(cos θ + 2)

(2 cos θ – 1) (cos θ + 2) = 0

So, either 2 cos θ – 1 = 0 or cos θ + 2 = 0

If 2 cos θ – 1 = 0

cos θ = ½

⇒ θ = 60^o

And, for cos θ + 2 = 0

⇒ cos θ = -2 which is not possible being out of range.

Thus, θ = 60^o

(iii) sec² θ – 2 tan θ = 0

(1 + tan² θ) – 2 tan θ = 0

tan² θ – 2 tan θ + 1 = 0

(tan θ – 1)² = 0

tan θ – 1 = 0

⇒ tan θ = 1

Thus, θ = 45^o

(iv) tan² θ = 3 (sec θ – 1)

(sec² θ – 1) = 3 sec θ – 3

sec² θ – 1 – 3 sec θ + 3 = 0

sec² θ – 3 sec θ + 2 = 0

sec² θ – 2 sec θ – sec θ + 2 = 0

sec θ (sec θ – 2) – 1 (sec θ = 2) = 0

(sec θ – 1) (sec θ – 2) = 0

So, either sec θ – 1 = 0 or sec θ – 2 = 0

If sec θ – 1 = 0

sec θ = 1

⇒ θ = 0^o

And, if sec θ – 2 = 0

sec θ = 2

⇒ θ = 60^o

Thus, θ = 0^o or 60^o