ML Aggarwal Solutions for Class 10 Maths Chapter 5 – Quadratic Equations in One Variable are provided here to help students prepare for their exams at ease. This chapter mainly deals with problems based on quadratic equations in one variable. Students having any doubts about understanding the concepts can refer to ML Aggarwal Solutions for Class 10 Maths. The solutions are created by subject matter experts having vast experience in the academic field, which helps students attain good marks in Maths. From the exam point of view, the solutions are solved in a simple and structured manner where students can secure an excellent score by practising the problems from the textbook. Solutions that are provided here will help you in getting acquainted with a wide variety of questions and, thus, develop problem-solving skills. Students can easily download the PDF of this chapter’s solutions, which are available in the links provided below and can use it for future reference as well.
Chapter 5 – Quadratic Equations in One Variable contains five exercises, and the ML Aggarwal Class 10 Solutions present on this page provide solutions to questions of each exercise discussed in this chapter.
ML Aggarwal Solutions for Class 10 Maths Chapter 5:
Access answers to ML Aggarwal Solutions for Class 10 Maths Chapter 5 – Quadratic Equations in One Variable
EXERCISE 5.1
1. In each of the following, determine whether the given numbers are roots of the given equations or not;
(i) x2 – 5x + 6 = 0; 2, -3
(ii) 3x2 – 13x – 10 = 0; 5, -2/3
Solution:
(i) x2 – 5x + 6 = 0; 2, -3
Let us substitute the given values in the expression and check,
When, x = 2
x2 – 5x + 6 = 0
(2)2 – 5(2) + 6 = 0
4 – 10 + 6 = 0
0 = 0
∴ x = 0
When, x = -3
x2 – 5x + 6 = 0
(-3)2 – 5(-3) + 6 = 0
9 + 15 + 6 = 0
30 = 0
∴ x ≠ 0
Hence, the value x = 2 is the root of the equation.
And value x = -3 is not a root of the equation.
(ii) 3x2 – 13x – 10 = 0; 5, -2/3
Let us substitute the given values in the expression and check,
When, x = 5
3x2 – 13x – 10 = 0
3(5)2 – 13(5) – 10 = 0
3(25) – 65 – 10 = 0
75 – 75 = 0
0 = 0
∴ x = 0
When, x = -2/3
3x2 – 13x – 10 = 0
3(-2/3)2 – 13(-2/3) – 10 = 0
4/9 + 26/3 – 10 = 0
4/3 + 26/3 – 10 = 0
30/3 – 10 = 0
10 – 10 = 0
∴ x = 0
Hence, the value x = 5, -2/3 are the roots of the equation.
2. In each of the following, determine whether the given numbers are solutions of the given equation or not:
(i) x2 – 3√3x + 6 = 0; x = √3, -2√3
(ii) x2 – √2x – 4 = 0; x = -√2, 2√2
Solution:
(i) x2 – 3√3x + 6 = 0; x = √3, -2√3
Let us substitute the given values in the expression and check,
When, x = √3
x2 – 3√3x + 6 = 0
(√3)2 – 3√3(√3) + 6 = 0
3 – 9 + 6 = 0
-9 + 9 =0
0 = 0
∴ √3 is the solution of the equation.
When, x = -2√3
x2 – 3√3x + 6 = 0
(-2√3)2 – 3√3(-2√3) + 6 = 0
4(3) +18 + 6 = 0
12 + 18 + 6 = 0
36 =0
∴ -2√3 is not the solution of the equation.
(ii) x2 – √2x – 4 = 0; x = -√2, 2√2
Let us substitute the given values in the expression and check,
When, x = -√2
x2 – √2x – 4 = 0
(-√2)2 – √2(-√2) – 4 = 0
2 + 2 – 4 = 0
4 – 4 = 0
0 = 0
∴ -√2 is the solution of the equation.
When, x = 2√2
x2 – √2x – 4 = 0
(2√2)2 – √2(2√2) – 4 = 0
4(2) – 4 – 4 = 0
4 – 4 = 0
0 = 0
∴ 2√2 is the solution of the equation.
3. (i) If –1/2 is a solution of the equation 3x² + 2kx – 3 = 0, find the value of k.
(ii) If 2/3 is a solution of the equation 7x² + kx – 3 = 0, find the value of k.
Solution:
(i) If –1/2 is a solution of the equation 3x² + 2kx – 3 = 0, find the value of k.
Let us substitute the given value x = -1/2 in the expression, we get
3x² + 2kx – 3 = 0
3(-1/2)2 + 2k(-1/2) – 3 = 0
3/4 – k – 3 = 0
¾ – 3 = k
By taking LCM
k = (3-12)/4
= -9/4
∴ Value of k = -9/4.
(ii) If 2/3 is a solution of the equation 7x² + kx – 3 = 0, find the value of k.
Let us substitute the given value x = 2/3 in the expression, we get
7x² + kx – 3 = 0
7(2/3)2 + k(2/3) – 3 = 0
7(4/9) + 2k/3 – 3 = 0
28/9 – 3 + 2k/3 = 0
2k/3 = 3 – 28/9
By taking LCM on the RHS
2k/3 = (27 – 28)/9
= -1/9
k = -1/9 × (3/2)
= -1/6
∴ Value of k = -1/6.
4. (i) If √2 is a root of the equation kx² + √2x – 4 = 0, find the value of k.
(ii) If a is a root of the equation x² – (a + b)x + k = 0, find the value of k.
Solution:
(i) If √2 is a root of the equation kx² + √2x – 4 = 0, find the value of k.
Let us substitute the given value x = √2 in the expression, we get
kx² + √2x – 4 = 0
k(√2)2 + √2(√2) – 4 = 0
2k + 2 – 4 = 0
2k – 2 = 0
k = 2/2
= 1
∴ Value of k = 1.
(ii) If a is a root of the equation x² – (a + b)x + k = 0, find the value of k.
Let us substitute the given value x = a in the expression, we get
x² – (a + b)x + k = 0
a2 – (a + b)a + k = 0
a2 – a2 – ab + k = 0
-ab + k = 0
k = ab
∴ Value of k = ab.
5. If 2/3 and -3 are the roots of the equation px² + 7x + q = 0, find the values of p and q.
Solution:
Let us substitute the given value x = 2/3 in the expression, we get
px² + 7x + q = 0
p(2/3)2 + 7(2/3) + q = 0
4p/9 + 14/3 + q = 0
By taking LCM
4p + 42 + 9q = 0
4p + 9q = – 42 … (1)
Now, substitute the value x = -3 in the expression, we get
px² + 7x + q = 0
p(-3)2 + 7(-3) + q = 0
9p + q – 21 = 0
9p + q = 21
q = 21 – 9p…. (2)
By substituting the value of q in equation (1), we get
4p + 9q = – 42
4p + 9(21 – 9p) = -42
4p + 189 – 81p = -42
189 – 77p = -42
189 + 42 = 77p
231 = 77p
p = 231/77
p = 3
Now, substitute the value of p in equation (2), we get
q = 21 – 9p
= 21 – 9(3)
= 21 – 27
= -6
∴ The value of p is 3 and q is -6.
EXERCISE 5.2
Solve the following equations (1 to 24) by factorization:
1. (i) x² – 3x – 10 = 0
(ii) x(2x + 5) = 3
Solution:
(i) x² – 3x – 10 = 0
Let us simplify the given expression,
x2 – 5x + 2x – 10 = 0
x(x – 5) + 2(x – 5) = 0
(x + 2) (x – 5) =0
So now,
(x + 2) = 0 or (x – 5) =0
x = -2 or x = 5
∴ Value of x = -2, 5
(ii) x(2x + 5) = 3
Let us simplify the given expression,
2x2 + 5x – 3 = 0
Now, let us factorize
2x2 + 6x – x – 3 = 0
2x(x + 3) -1(x + 3) = 0
(2x – 1) (x + 3) = 0
So now,
(2x – 1) = 0 or (x + 3) = 0
2x = 1 or x = -3
x = ½ or x = -3
∴ Value of x = ½, -3
2. (i) 3x2 – 5x – 12 = 0
(ii) 21x2 – 8x – 4 = 0
Solution:
(i) 3x2 – 5x – 12 = 0
Let us simplify the given expression,
3x2 – 9x + 4x – 12 = 0
3x(x – 3) + 4(x – 3) = 0
(3x + 4) (x – 3) =0
So now,
(3x + 4) = 0 or (x – 3) =0
3x = -4 or x = 3
x = -4/3 or x = 3
∴ Value of x = -4/3, 3
(ii) 21x2 – 8x – 4 = 0
Let us simplify the given expression,
21x2 – 14x + 6x – 4 = 0
7x(3x – 2) + 2(3x – 2) = 0
(7x + 2) (3x – 2) = 0
So now,
(7x + 2) = 0 or (3x – 2) = 0
7x = -2 or 3x = 2
x = -2/7 or x = 2/3
∴ Value of x = -2/7, 2/3
3. (i) 3x² = x + 4
(ii) x(6x – 1) = 35
Solution:
(i) 3x² = x + 4
Let us simplify the given expression,
3x2 – x – 4 = 0
Now, let us factorize
3x2 – 4x + 3x – 4 = 0
x(3x – 4) + 1(3x – 4) = 0
(x + 1) (3x – 4) = 0
So now,
(x + 1) = 0 or (3x – 4) = 0
x = -1 or 3x = 4
x = -1 or x = 4/3
∴ Value of x = -1, 4/3
(ii) x(6x – 1) = 35
Let us simplify the given expression,
6x2 – x – 35 = 0
Now, let us factorize
6x2 – 15x + 14x – 35 = 0
3x(2x – 5) + 7(2x – 5) = 0
(3x + 7) (2x – 5) = 0
So now,
(3x + 7) = 0 or (2x – 5) = 0
3x = -7 or 2x = 5
x = -7/3 or x = 5/2
∴ Value of x = -7/3, 5/2
4. (i) 6p² + 11p – 10 = 0
(ii) 2/3x2 −1/3x = 1
Solution:
(i) 6p² + 11p – 10 = 0
Let us factorize the given expression,
6p2 + 15p – 4p – 10 = 0
3p(2p + 5) – 2(2p + 5) = 0
(3p – 2) (2p + 5) = 0
So now,
(3p – 2) = 0 or (2p + 5) = 0
3p = 2 or 2p = -5
p = 2/3 or p = -5/2
∴ Value of p = 2/3, -5/2
(ii) 2/3x2 −1/3x = 1
Let us simplify the given expression,
2x2 – x = 3
2x2 – x – 3 = 0
Let us factorize the given expression,
2x2 – 3x + 2x – 3 = 0
x(2x – 3) + 1(2x – 3) = 0
(x + 1) (2x – 3) = 0
So now,
(x + 1) = 0 or (2x – 3) = 0
x = -1 or 2x = 3
x = -1 or x = 3/2
∴ Value of x = -1, 3/2
5. (i) 3(x – 2)2 = 147
(ii) 1/7(3x – 5)² = 28
Solution:
(i) 3(x – 2)2 = 147
Firstly let us expand the given expression,
3(x2 – 4x + 4) = 147
3x2 – 12x + 12 = 147
3x2 – 12x +12 – 147 = 0
3x2 – 12x – 135 = 0
Divide by 3, we get
x2 – 4x – 45 = 0
Let us factorize the expression,
x2 – 9x + 5x – 45 = 0
x(x – 9) + 5(x – 9) = 0
(x + 5) (x – 9) = 0
So now,
(x + 5) = 0 or (x – 9) = 0
x = -5 or x = 9
∴ Value of x = -5, 9
(ii) 1/7(3x – 5)2 = 28
Let us simplify the expression,
(3x – 5)2 = 28 × 7
(3x – 5)2 = 196
Now let us expand,
9x2 – 30x + 25 = 196
9x2 – 30x + 25 – 196 = 0
9x2 – 30x – 171 = 0
Divide by 3, we get
3x2 – 10x – 57 = 0
Let us factorize the expression,
3x2 – 19x + 9x – 57 = 0
x(3x – 19) + 3(3x – 19) = 0
(x + 3) (3x – 19) = 0
So now,
(x + 3) = 0 or (3x – 19) = 0
x = -3 or 3x = 19
x = -3 or x = 19/3
∴ Value of x = -3, 19/3
6. x2 – 4x – 12 = 0, when x ∈ N
Solution:
Let us factorize the expression,
x2 – 4x – 12 = 0
x2 – 6x + 2x – 12 = 0
x(x – 6) + 2(x – 6) = 0
(x + 2) (x – 6) = 0
So now,
(x + 2) = 0 or (x – 6) = 0
x = -2 or x = 6
∴ Value of x = 6 (Since -2 is not a natural number).
7. 2x² – 9x + 10 = 0, when
(i) x ∈ N
(ii) x ∈ Q
Solution:
Let us factorize the expression,
2x² – 9x + 10 = 0
2x2 – 4x – 5x + 10 = 0
2x(x – 2) – 5(x – 2) = 0
(2x – 5) (x – 2) = 0
So now,
(2x – 5) = 0 or (x – 2) = 0
2x = 5 or x = 2
x = 5/2 or x = 2
(i) When, x ∈ N then, x = 2
(ii) When, x ∈ Q then, x = 2, 5/2
8. (i) a²x² + 2ax + 1 = 0, a ≠ 0
(ii) x² – (p + q)x + pq = 0
Solution:
(i) a²x² + 2ax + 1 = 0, a ≠ 0
Let us factorize the expression,
a²x² + 2ax + 1 = 0
a²x² + ax + ax + 1 = 0
ax(ax + 1) + 1(ax + 1) = 0
(ax + 1) (ax + 1) = 0
So now,
(ax + 1) = 0 or (ax + 1) = 0
ax = -1 or ax = -1
x = -1/a or x = -1/a
∴ Value of x = -1/a, -1/a
(ii) x² – (p + q)x + pq = 0
Let us simplify the expression,
x² – (p + q)x + pq = 0
x2 – px – qx + pq = 0
x(x – p) – q(x – p) = 0
(x – q) (x – p) = 0
So now,
(x – q) = 0 or (x – p) = 0
x = q or x = p
∴ Value of x = q, p
9. a²x² + (a² + b²)x + b² = 0, a≠0
Solution:
Let us simplify the expression,
a²x² + (a² + b²)x + b² = 0
a²x² + a2x + b2x + b2 = 0
a2x(x + 1) + b2(x + 1) = 0
(a2x + b2) (x + 1) = 0
So now,
(a2x + b2) = 0 or (x + 1) = 0
a2x = -b2 or x = -1
x = -b2/a2 or x = -1
∴ Value of x = -b2/a2, -1
10. (i) √3x2 + 10x + 7√3 = 0 (ii) 4√3x2 + 5x – 2√3 = 0.
Solution:
(i) √3x2 + 10x + 7√3 = 0
Let us factorize the given expression,
√3x2 + 3x + 7x + 7√3 = 0 [As √3 × 7√3 = 3 × 7 = 21 and 3 + 7 = 10]
√3x(x + √3) + 7(x + √3) = 0
(√3x + 7) (x + √3) = 0
So now,
(√3x + 7) = 0 or (x + √3) = 0
√3x = -7 or x = -√3
x = -7/√3 or x = -√3
∴ Value of x = -7/√3, -√3
(ii) 4√3x2 + 5x – 2√3 = 0
Let us factorize the given expression,
4√3x2 + 8x – 3x – 2√3 = 0 [As, 4√3 × (-2√3) = -8 × 3 = -24 and 8 × (-3) = -24]
4x(√3x + 2) – √3(√3x + 2) = 0
(4x – √3) (√3x + 2) = 0
So now,
(4x – √3) = 0 or (√3x + 2) = 0
4x = √3 or √3x = -2
x = √3/4 or x = -2/√3
∴ Value of x = √3/4, -2/√3
11. (i) x2 – (1 + √2)x + √2 = 0 (ii) x + 1/x = 2(1/20).
Solution:
(i) x2 – (1 + √2)x + √2 = 0
Let us expand the given expression,
x2 – x – √2x + √2 = 0
Taking common, we have
x(x – 1) – √2(x – 1) = 0
(x – 1) (x – √2) = 0
So now,
(x – 1) = 0 or (x + √2) = 0
x = 1 or x = -√2
∴ Value of x = 1, -√2
(ii) x + 1/x = 2(1/20)
Rewriting the given expression, we have
(x2 + 1)/x = 41/20
On cross-multiplication, we get
20(x2 + 1) = 41x
20x2 + 20 = 41x
20x2 – 41x + 20 = 0
Let us factorize the expression now,
20x2 – 25x – 16x + 20 = 0
5x(4x – 5) – 4(4x – 5) = 0
(5x – 4) (4x – 5) = 0
So,
(5x – 4) = 0 or (4x – 5) = 0
5x = 4 or 4x = 5
x = 4/5 or x = 5/4
∴ Value of x = 4/5, 5/4
12. (i) 2/x2 – 5/x + 2 = 0, x ≠ 0 (ii) x2/15 – x/3 – 10 = 0.
Solution:
(i) 2/x2 – 5/x + 2 = 0
Taking L.C.M for the given expression,
(2 – 5x + 2x2)/x2 = 0
2x2 – 5x + 2 = 0
Now, on factorizing the above expression, we get
2x2 – 4x – x + 2 = 0
2x(x – 2) – 1(x – 2) = 0
(2x – 1) (x – 2) = 0
So,
(2x – 1) = 0 or (x – 2) = 0
2x = 1 or x = 2
x = ½ or x = 2
∴ Value of x = ½, 2
(ii) x2/15 – x/3 – 10 = 0
Taking L.C.M for the given expression,
(x2 – 5x – 150)/15 = 0
x2 – 5x – 150 = 0
Now, on factorizing the above expression, we get
x2 – 15x + 10x – 150 = 0
x(x – 15) + 10(x – 15) = 0
(x – 15) (x + 10) = 0
So,
(x – 15) = 0 or (x + 10) = 0
x = 15 or x = -10
∴ Value of x = 15, -10
13. (i) 3x – 8/x = 2 (ii) (x + 2)/(x + 3) = (2x – 3)/(3x – 7).
Solution:
(i) 3x – 8/x = 2
Taking L.C.M, we have
(3x2 – 8)/x = 2
3x2 – 8 = 2x
3x2 – 2x – 8 = 0
On factorizing the above expression, we get
3x2 – 6x + 4x – 8 = 0
3x(x – 2) + 4(x – 2) = 0
(3x + 4) (x – 2) = 0
So,
(3x – 4) = 0 or (x – 2) = 0
3x = 4 or x = 2
x = 4/3 or x = 2
∴ Value of x = 4/3, 2
(ii) (x + 2)/(x + 3) = (2x – 3)/(3x – 7)
Upon cross-multiplication, we get
(x + 2) (3x – 7) = (2x – 3) (x + 3)
3x2 – 7x + 6x – 14 = 2x2 + 6x – 3x – 9
3x2 – x – 14 = 2x2 + 3x – 9
3x2 – 2x2 – x – 3x – 14 + 9 = 0
x2 – 4x – 5 = 0
Factorizing the above expression, we get
x2 – 5x + x – 5 = 0
x(x – 5) + 1(x – 5) = 0
(x + 1) (x – 5) = 0
So,
x + 1 = 0 or x – 5 = 0
x = -1 or x = 5
∴ Value of x = -1, 5
14. (i) 8/(x + 3) – 3/(2 – x) = 2 (ii) x/(x – 1) + (x – 1)/x = 2½
Solution:
(i) 8/(x + 3) – 3/(2 – x) = 2
Taking L.C.M, we have
[8(2 – x) – 3(x + 3)]/[(x + 3)(2 – x)] = 2Upon cross-multiplication,
16 – 8x – 3x – 9 = 2 (x + 3) (2 – x)
7 – 11x = 2 (2x + 6 – x2 – 3x)
7 – 11x = 2 (6 – x2 – x)
7 – 11x = 12 – 2x2 – 2x
2x2 – 11x + 2x + 7 – 12 = 0
2x2 – 9x – 5 = 0
Now, let’s factorize the above equation to find x
2x2 – 10x + x – 5 = 0
2x(x – 5) + 1(x – 5) = 0
(2x + 1) (x – 5) = 0
So,
2x + 1 = 0 or x – 5 = 0
x = -1/2 or x = 5
∴ Value of x = -1/2, 5
(ii) x/(x – 1) + (x – 1)/x = 2½
Taking L.C.M, we have
[x2 + (x – 1)2] / x(x- 1) = 5/2(x2 + x2 – 2x + 1)/ (x2 – x) = 5/2
(2x2 – 2x + 1)/ (x2 – x) = 5/2
Upon cross-multiplication, we get
2 (2x2 – 2x + 1) = 5 (x2 – x)
4x2 – 4x + 2 = 5x2 – 5x
5x2 – 4x2 – 5x + 4x – 2 = 0
x2 – x – 2 = 0
Now, let’s factorize the above equation to find x
x2 – 2x + x – 2 = 0
x(x – 2) + 1(x – 2) = 0
(x + 1) (x – 2) = 0
So,
x + 1 = 0 or x – 2 = 0
x = -1 or x = 2
∴ Value of x = -1, 2
15. (i) (x + 1)/(x – 1) + (x – 2)/(x + 2) = 3
(ii) 1/(x – 3) – 1/(x + 5) = 1/6.
Solution:
(i) (x + 1)/(x – 1) + (x – 2)/(x + 2) = 3
[(x + 1) (x + 2) + (x – 2) (x – 1)]/[(x – 1)(x + 2)] = 3 [Taking L.C.M]On expanding, we get
x2 + 3x + 2 + x2 – 3x + 2 = 3 (x – 1) (x + 2)
2x2 + 4 = 3 (x2 + x – 2)
2x2 + 4 = 3x2 + 3x – 6
3x2 – 2x2 + 3x – 6 – 4 = 0
x2 + 3x – 10 = 0
Now, let’s factorize the above equation to find x
x2 + 5x – 2x – 10 = 0
x(x + 5) – 2(x – 5) = 0
(x + 5) (x – 5) = 0
So,
x + 5 = 0 or x – 5 = 0
x = -5 or x = 5
∴ Value of x = -5, 5
(ii) 1/(x – 3) – 1/(x + 5) = 1/6
Taking L.C.M, we have
[x + 5 – (x – 3)] / [(x – 3) (x + 5)] = 1/6(x + 5 – x + 3) / [(x – 3) (x + 5)] = 1/6
8/ [(x – 3) (x + 5)] = 1/6
Upon cross-multiplying, we have
8 × 6 = (x – 3) (x + 5)
48 = x2 + 5x – 3x – 15
x2 + 2x – 15 – 48 = 0
x2 + 2x – 63 = 0
Now, let’s factorize the above equation to find x
x2 + 9x – 7x – 63 = 0
x(x + 9) – 7(x + 9) = 0
(x – 7) (x + 9) = 0
So,
x – 7 = 0 or x + 9 = 0
x = 7 or x = -9
∴ Value of x = 7, -9
16. (i) a/(ax – 1) + b/(bx – 1) = a + b, a + b ≠ 0, ab ≠ 0
(ii) 1/(2a + b + 2x) = 1/2a + 1/b + 1/2x
Solution:
(i) a/(ax – 1) + b/(bx – 1) = a + b, a + b ≠ 0, ab ≠ 0
Let’s rearrange the equation for simple solving,
[a/(ax – 1) – b] + [b/(bx – 1) – a] = 0 [a – b(ax – 1)]/(ax – 1) + [b – a(bx – 1)]/(bx – 1) = 0(a – abx + b)/(ax – 1) + (b – abx + a)/(bx – 1) = 0
(a – abx + b) [1/(ax – 1) + 1/(bx – 1)] = 0 {Taking common terms out}
(a – abx + b) [(bx – 1 + ax – 1)/(ax – 1)(bx – 1)] = 0
(a – abx + b) [(ax + bx – 2)/ (ax – 1)(bx – 1)] = 0
So,
(a – abx + b) = 0 or (ax + bx – 2)/ [(ax – 1) (bx – 1)] = 0
If (a – abx + b) = 0,
a + b = abx
x = (a + b)/ab
And,
if (ax + bx – 2)/ [(ax – 1) (bx – 1)] = 0
ax + bx – 2 = 0
(a + b)x = 2
x = 2/(a + b)
∴ Value of x = (a + b)/ab, 2/(a + b)
(ii)
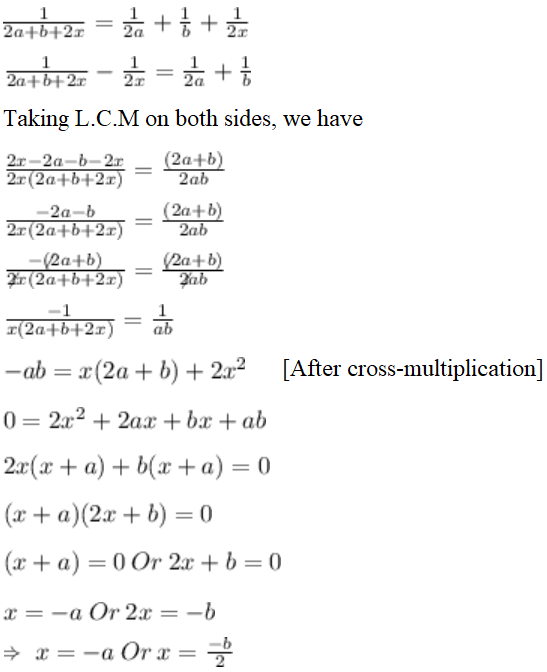

∴ Value of x = -a, -b/2
17. 1/(x + 6) + 1/(x – 10) = 3/(x – 4).
Solution:
Given equation,
1/(x + 6) + 1/(x – 10) = 3/(x – 4)
Taking L.C.M for the R.H.S of the equation,
[(x – 10) + (x + 6)]/ [(x + 6) (x – 10)] = 3/(x- 4)(2x – 4)/ (x2 – 4x – 60) = 3/(x- 4)
On cross-multiplying, we get
(2x – 4) (x – 4) = 3(x2 – 4x – 60)
2x2 – 8x – 4x + 16 = 3x2 – 12x – 180
2x2 – 12x + 16 = 3x2 – 12x – 180
3x2 – 2x2 – 12x + 12x – 180 – 16 = 0
x2 – 196 = 0
x2 = 196
x = √196
∴ x = ± 14
18. (i) √(3x + 4) = x (ii) √[x(x – 7)] = 3√2
Solution:
(i) √(3x + 4) = x
On squaring on both sides, we get
3x + 4 = x2
x2 – 3x – 4 = 0
Let us factorize the above expression,
x2 – 4x + x – 4 = 0
x(x – 4) + 1(x – 4) = 0
(x – 4) (x + 1) = 0
So,
x – 4 = 0 or x + 1 = 0
x = 4 or x = -1
∴ Value of x = 4, -1
(ii) √[x(x – 7)] = 3√2
On squaring on both sides, we get
x(x – 7) = (3√2)2
x2 – 7x = 9 × 2
x2 – 7x – 18 = 0
Let us factorize the above expression,
x2 – 9x + 2x – 18 = 0
x(x – 9) + 2(x – 9) = 0
(x – 9) (x + 2) = 0
So,
x – 9 = 0 or x + 2 = 0
x = 9 or x = -2
∴ Value of x = 9, -2
19. Use the substitution y = 3x + 1 to solve for x:
5(3x + 1)2 + 6(3x + 1) – 8 = 0.
Solution:
Given equation,
5(3x + 1)2 + 6(3x + 1) – 8 = 0
Upon substituting y = 3x + 1,
5y2 + 6y – 8 = 0
We get a quadratic equation in y
Now, solving for y by factorization, we get
5y2 + 10y – 4y – 8 = 0
5y(y + 2) – 4(y + 2) = 0
(5y – 4) (y + 2) = 0
So,
5y – 4 = 0 or y + 2 = 0
5y = 4 or y = -2
y = 4/5 or y = -2
Now, to find the value of x let’s back-substitute y
3x + 1 = 4/5 or 3x + 1 = -2
3x = 4/5 – 1 or 3x = -2 – 1
3x = (4 – 5)/5 or 3x = -3
3x = -1/5 or x = -3/3
x = -1/15 or x = -1
∴ Value of x = -1, -1/15
20. Find the values of x if p + 1 = 0 and x2 + px – 6 = 0.
Solution:
Given quadratic equation: x2 + px – 6 = 0
And, p + 1 = 0
So,
p = -1
Substituting the value of p in the given quadratic equation, we get
x2 + (-1)x – 6 = 0
x2 – x – 6 = 0
Solving for x by factorization, we have
x2 – 3x + 2x – 6 = 0
x(x – 3) + 2(x – 3) = 0
(x + 2) (x – 3) = 0
So,
x + 2 = 0 or x – 3 = 0
x = -2 or x = 3
∴ Value of x = -2, 3
21. Find the values of x if p + 7 = 0, q – 12 = 0 and x2 + px + q = 0.
Solution:
Given quadratic equation: x2 + px + q = 0
And, p + 7 = 0 and q – 12 = 0
So,
p = -7 and q = 12
Substituting the value of p and q in the given quadratic equation, we get
x2 + (-7)x + 12 = 0
x2 – 7x + 12 = 0
Solving for x by factorization, we have
x2 – 4x – 3x + 12 = 0
x(x – 4) – 3(x – 4) = 0
(x – 3) (x – 4) = 0
So,
x – 3 = 0 or x – 4 = 0
x = 3 or x = 4
∴ Value of x = 3, 4
22. If x = p is a solution of the equation x(2x + 5) = 3, then find the value of p.
Solution:
Given that, x = p is a solution of the equation x(2x + 5) = 3
Then, upon substituting x = p in must satisfy the equation
p(2p + 5) = 3
2p2 + 5p = 3
2p2 + 5p – 3 = 0
Factorizing the above expression, we get
2p2 + 6p – p – 3 = 0
2p(p + 3) – 1(p + 3) = 0
(2p – 1) (p + 3) = 0
So,
2p – 1 = 0 or p + 3 = 0
2p = 1 or p = -3
p = ½ or p = -3
∴ Value of p = ½, -3
23. If x = 3 is a solution of the equation (k + 2)x2 – kx + 6 = 0, find the value of k. Hence, find the other root of the equation.
Solution:
Given equation: (k + 2)x2 – kx + 6 = 0
And x = 3 is a solution of the equation
So, upon substituting x = 3 it must satisfy the equation
(k + 2)(3)2 – k(3) + 6 = 0
(k + 2)(9) – 3k + 6 = 0
9k + 18 – 3k + 6 = 0
6k + 24 = 0
6(k + 4) = 0
So,
k + 4 = 0
k = -4
Now, putting k = -4 in the given equation, we have
(-4 + 2)x2 – (-4)x + 6 = 0
-2x2 + 4x + 6 = 0
x2 – 2x – 3 = 0 [Dividing by -2 on both sides]
Factorizing the above expression, we get
x2 – 3x + x – 3 = 0
x(x – 3) + 1(x – 3) = 0
(x + 1)(x – 3) = 0
So,
x + 1 = 0 or x – 3 = 0
x = -1 or x = 3
Hence, the other root of the given equation is -1.
EXERCISE 5.3
Solve the following (1 to 8) equations by using the formula:
1. (i) 2x² – 7x + 6 = 0
(ii) 2x² – 6x + 3 = 0
Solution:
(i) 2x² – 7x + 6 = 0
Let us consider,
a = 2, b = -7, c = 6
So, by using the formula,

D = b2 – 4ac
= (-7)2 – 4(2) (6)
= 49 – 48
= 1
So,
x = [-(-7) ± √1] / 2(2)
= [7 + 1]/ 4 or [7 – 1]/4
= 8/4 or 6/4
= 2 or 3/2
∴ Value of x = 2, 3/2
(ii) 2x² – 6x + 3 = 0
Let us consider,
a = 2, b = -6, c = 3
So, by using the formula,

D = b2 – 4ac
= (-6)2 – 4(2) (3)
= 36 – 24
= 12
So,
x = [-(-6) ± √12] / 2(2)
= [6 ± 2√3] / 4
= [6 + 2√3]/ 4 or [6 – 2√3]/4
= 2(3 + √3)/4 or 2(3 – √3)/4
= (3 + √3)/2 or (3 – √3)/2
∴ Value of x = (3 + √3)/2, (3 – √3)/2
2. (i) 256x² – 32x + 1 = 0
(ii) 25x² + 30x + 7 = 0
Solution:
(i) 256x² – 32x + 1 = 0
Let us consider,
a = 256, b = -32, c = 1
So, by using the formula,

D = b2 – 4ac
= (-32)2 – 4(256) (1)
= 1024 – 1024
= 0
So,
x = [-(-32) ± √0] / 2(256)
= [32] / 512
= 1/16
∴ Value of x = 1/16
(ii) 25x² + 30x + 7 = 0
Let us consider,
a = 25, b = 30, c = 7
So, by using the formula,

D = b2 – 4ac
= (30)2 – 4(25) (7)
= 900 – 700
= 200
So,
x = [-(30) ± √200] / 2(25)
= [-30 ± √(100×2)]/ 50
= [-30 ± 10√2]/ 50
= [-3 ± √2]/ 5
= [-3 + √2)]/ 5 or [-3 – √2]/ 5
∴ Value of x = [-3 + √2)]/ 5, [-3 – √2]/ 5
3. (i) 2x² + √5x – 5 = 0
(ii) √3x² + 10x – 8√3 = 0
Solution:
(i) 2x² + √5x – 5 = 0
Let us consider,
a = 2, b = √5, c = -5
So, by using the formula,

D = b2 – 4ac
= (√5)2 – 4(2) (-5)
= 5 + 40
= 45
So,
x = [-(√5) ± √45] / 2(2)
= [-√5 ± 3√5)]/ 4
= [-√5 + 3√5)]/ 4 or [-√5 – 3√5]/ 4
= 2√5/4 or -4√5/4
= √5/2 or -√5
∴ Value of x = √5/2, -√5
(ii) √3x² + 10x – 8√3 = 0
Let us consider,
a = √3, b = 10, c = -8√3
So, by using the formula,

D = b2 – 4ac
= (10)2 – 4(√3) (-8√3)
= 100 + 96
= 196
So,
x = [-(10) ± √196] / 2(√3)
= [-10 ± 14] / 2(√3)
= [-10 + 14)]/ 2√3 or [-10 – 14)]/ 2√3
= 4/2√3 or -24/2√3
∴ Value of x = 4/2√3, -24/2√3
4. 

Solution:


D = b2 – 4ac
= (0)2 – 4(1) (-12)
= 0 + 48
= 48
So,
x = [-(0) ± √48] / 2(1)
= [±√48] / 2
= [±√(16×3)]/2
= ±4√3/2
= ±2√3
= 2√3 or -2√3
∴ Value of x = 2√3, -2√3

Let us cross-multiply, and we get
(x + 1) (2x + 3) = (x + 3) (3x + 2)
Now by simplifying, we get
2x2 + 3x + 2x + 3 = 3x2 + 9x + 2x + 6
2x2 + 5x + 3 – 3x2 – 11x – 6 = 0
-x2 – 6x – 3 = 0
x2 + 6x + 3 = 0
Let us consider,
a = 1, b = 6, c = 3
So, by using the formula,

D = b2 – 4ac
= (6)2 – 4(1) (3)
= 36 -12
= 24
So,
x = [-(6) ± √24] / 2(1)
= [-6 ± √(4×6)] / 2
= [-6 ± 2√6]/2
= -3 ± √6
= -3 + √6 or -3 – √6
∴ Value of x = -3 + √6, -3 – √6
5. (i) a (x² + 1) = (a² + 1) x, a ≠ 0
(ii) 4x² – 4ax + (a² – b²) = 0
Solution:
(i) a (x² + 1) = (a² + 1) x, a ≠ 0
Let us simplify the expression,
ax2 + a – a2x + x = 0
ax2 – (a2 + 1)x + a = 0
Let us consider,
a = a, b = -(a2 + 1), c = a
So, by using the formula,

D = b2 – 4ac
= (-(a2 + 1))2 – 4(a) (a)
= a4 + 2a2 + 1 – 4a2
= a4 – 2a2 + 1
= (a2 – 1)2
So,
x = [-(-(a2 + 1)) ± √(a2 – 1)2] / 2(a)
= [(a2 + 1) ± (a2 – 1)] / 2a
= [(a2 + 1) + (a2 – 1)] / 2a or [(a2 + 1) – (a2 – 1)] / 2a
= [a2 + 1 + a2 – 1]/2a or [a2 + 1 – a2 + 1]/2a
= 2a2/2a or 2/2a
= a or 1/a
∴ Value of x = a, 1/a
(ii) 4x² – 4ax + (a² – b²) = 0
Let us consider,
a = 4, b = -4a, c = (a2 – b2)
So, by using the formula,

D = b2 – 4ac
= (-4a)2 – 4(4) (a2 – b2)
= 16a2 – 16(a2 – b2)
= 16a2 – 16a2 + 16b2
= 16b2
So,
x = [-(-4a) ± √16b2] / 2(4)
= [4a ± 4b] / 8
= 4[a ± b] / 8
= [a ± b] / 2
= [a + b] / 2 or [a – b] / 2
∴ Value of x = [a + b] / 2, [a – b] / 2
6. (i) x − 1/x = 3, x ≠ 0
(ii) 1/ x + 1/(x − 2) = 3, x ≠ 0, 2
Solution:
(i) x−1/x = 3, x ≠ 0
Let us simplify the given expression,
By taking LCM
x2 – 1 = 3x
x2 – 3x – 1 = 0
Let us consider,
a = 1, b = -3, c = -1
So, by using the formula,

D = b2 – 4ac
= (-3)2 – 4(1) (-1)
= 9 + 4
= 13
So,
x = [-(-3) ± √13] / 2(1)
= [3 ± √13] / 2
= [3 + √13] / 2 or [3 – √13] / 2
∴ Value of x = [3 + √13] / 2 or [3 – √13] / 2
(ii) 1/ x + 1/(x−2) = 3, x ≠ 0, 2
Let us simplify the given expression,
By taking LCM
[(x – 2) + x] / [x(x – 2)] = 3 [x – 2 + x] / [x2 – 2x] = 32x – 2 = 3(x2 – 2x)
2x – 2 = 3x2 – 6x
3x2 – 6x – 2x + 2 = 0
3x2 – 8x + 2
Let us consider,
a = 3, b = -8, c = 2
So, by using the formula,

D = b2 – 4ac
= (-8)2 – 4(3) (2)
= 64 – 24
= 40
So,
x = [-(-8) ± √40] / 2(3)
= [8 ± 2√10] / 6
= 2[4 ± √10] / 6
= [4 ± √10] / 3
= [4 + √10] / 3 or [4 – √10] / 3
∴ Value of x = [4 + √10] / 3 or [4 – √10] / 3
7. Solve for x:

Solution:

So the equation becomes,
2x – 3/x = 5
By taking LCM
2x2 – 3 = 5x
2x2 – 5x – 3 = 0
Let us consider,
a = 2, b = -5, c = -3
So, by using the formula,

D = b2 – 4ac
= (-5)2 – 4(2) (-3)
= 25 + 24
= 49
So,
x = [-(-5) ± √49] / 2(2)
= [5 ± 7] / 4
= [5 + 7] / 4 or [5 – 7] / 4
= [12]/4 or [-2]/4
= 3 or -1/2
So, x = 3 or -1/2
Now,
Let us substitute in the equations,
When x = 3, then

By cross-multiplying,
2x – 1 = 3x + 9
3x + 9 – 2x + 1 = 0
x + 10 = 0
x = -10
When x = -1/2, then

By cross-multiplying,
2(2x – 1) = -(x + 3)
4x – 2 = -x – 3
4x – 2 + x + 3 = 0
5x + 1 = 0
5x = -1
x = -1/5
∴ Value of x = -10, -1/5
8. Solve the following quadratic equations for x and give your answer correct to 2 decimal places:
(i) x² – 5x – 10 = 0
(ii) x2 + 7x = 7
Solution:
(i) x² – 5x – 10 = 0
Let us consider,
a = 1, b = -5, c = -10
So, by using the formula,

D = b2 – 4ac
= (-5)2 – 4(1) (-10)
= 25 + 40
= 65
So,
x = [-(-5) ± √65] / 2(1)
= [5 ± √65] / 2
= [5 ± 8.06] / 2
= [5 + 8.06] / 2 or [5 – 8.06] / 2
= [13.06]/2 or [-3.06]/2
= 6.53 or -1.53
∴ Value of x = 6.53 or -1.53
(ii) x2 + 7x = 7
On rearranging the expression, we get
x2 + 7x – 7 = 0
Let us consider,
a = 1, b = 7, c = -7
So, by using the formula,

D = b2 – 4ac
= (7)2 – 4(1) (-7)
= 49 + 28
= 77
So,
x = [-7 ± √77] / 2(1)
= [-7 ± 8.77] / 2
= [-7 + 8.77] / 2 or [-7 – 8.77] / 2
= 1.77/2 or -15.77/2
= 0.885 or -7.885
∴ Value of x = 0.89 or -7.89
9. Solve the following equations by using quadratic formula and give your answer correct to 2 decimal places:
(i) 4x2 – 5x – 3 = 0
(ii) 2x – 1/x = 7
Solution:
(i) 4x2 – 5x – 3 = 0
Let us consider,
a = 4, b = -5, c = -3
So, by using the formula,
D = b2 – 4ac
= (-5)2 – 4(4) (-3)
= 25 + 48
= 73
So,
x = [-(-5) ± √73] / 2(4)
= [5 ± 8.54] / 8
= [5 + 8.54] / 8 or [5 – 8.54] / 8
= 13.54/8 or -3.54/8
= 1.6925 or -0.4425
∴ Value of x = 1.69 or -0.44
(ii) 2x – 1/x = 7
By taking LCM
2x2 – 1 = 7x
2x2 – 7x – 1 = 0
Let us consider,
a = 2, b = -7, c = -1
So, by using the formula,

D = b2 – 4ac
= (-7)2 – 4(2) (-1)
= 49 + 8
= 57
So,
x = [-(-7) ± √57] / 2(2)
= [7 ± 7.549] / 4
= [7 + 7.549] / 4 or [7 – 7.549] / 4
= 14.549/4 or -0.549/4
= 3.637 or -0.137
= 3.64 or -0.14
∴ Value of x = 3.64 or -0.14
10. Solve the following equations and give your answer correct to two significant figures.
(i) x2 – 4x – 8 = 0 (ii) x − 18/x = 6.
Solution:
(i) Given equation:
x2 – 4x – 8 = 0
Let us consider,
a = 1, b = -4, c = -8
So, by using the formula,

D = b2 – 4ac
= (-4)2 – 4(1) (-8)
= 16 + 32
= 48
So,
x = [-(-4) ± √48] / 2(1)
= [4 ± 6.93]/2
= [4 + 6.93]/2 or [4 – 6.93]/2
= [10.93]/2 or -2.93/2
= 5.465 or -1.465
∴ Value of x = 5.47 or -1.47
(ii) Given equation:
x−18/x = 6
By taking LCM
x2 – 18 = 6x
x2 – 6x – 18 = 0
Let us consider,
a = 1, b = -6, c = -18
So, by using the formula,

D = b2 – 4ac
= (-6)2 – 4(1) (-18)
= 36 + 72
= 108
So,
x = [-(-6) ± √108] / 2(1)
= [6 ± 10.39]/2
= [6 + 10.39]/2 or [6 – 10.39]/2
= [16.39]/2 or -4.39/2
= 8.19 or -2.19
∴ Value of x = 8.19 or -2.19
11. Solve the equation 5x² – 3x – 4 = 0 and give your answer correct to 3 significant figures:
Solution:
Given equation:
5x² – 3x – 4 = 0
Let us consider,
a = 5, b = -3, c = -4
So, by using the formula,

D = b2 – 4ac
= (-3)2 – 4(5) (-4)
= 9 + 80
= 89
So,
x = [-(-3) ± √89] / 2(5)
= [3 ± 9.43] / 10
= [3 + 9.43] / 10 or [3 – 9.43] / 10
= 12.433/10 or -6.43/10
= 1.24 or -0.643
∴ Value of x = 1.24 or -0.643
EXERCISE 5.4
1. Find the discriminate of the following equations and hence find the nature of roots:
(i) 3x² – 5x – 2 = 0
(ii) 2x² – 3x + 5 = 0
(iii) 16x² – 40x + 25 = 0
(iv) 2x² + 15x + 30 = 0
Solution:
(i) 3x² – 5x – 2 = 0
Let us consider,
a = 3, b = -5, c = -2
By using the formula,
D = b2 – 4ac
= (-5)2 – 4(3) (-2)
= 25 + 24
= 49
So,
Discriminate, D = 49
D > 0
∴ Roots are real and distinct.
(ii) 2x² – 3x + 5 = 0
Let us consider,
a = 2, b = -3, c = 5
By using the formula,
D = b2 – 4ac
= (-3)2 – 4(2) (5)
= 9 – 40
= -31
So,
Discriminate, D = -31
D < 0
∴ Roots are not real.
(iii) 16x² – 40x + 25 = 0
Let us consider,
a = 16, b = -40, c = 25
By using the formula,
D = b2 – 4ac
= (-40)2 – 4(16) (25)
= 1600 – 1600
= 0
So,
Discriminate, D = 0
D = 0
∴ Roots are real and equal.
(iv) 2x² + 15x + 30 = 0
Let us consider,
a = 2, b = 15, c = 30
By using the formula,
D = b2 – 4ac
= (15)2 – 4(2) (30)
= 225 – 240
= – 15
So,
Discriminate, D = -15
D < 0
∴ Roots are not real.
2. Discuss the nature of the roots of the following quadratic equations:
(i) 3x² – 4√3x + 4 = 0
(ii) x² – 1/2x + 4 = 0
(iii) – 2x² + x + 1 = 0
(iv) 2√3x² – 5x + √3 = 0
Solution:
(i) 3x² – 4√3x + 4 = 0
Let us consider,
a = 3, b = -4√3, c = 4
By using the formula,
D = b2 – 4ac
= (-4√3)2 – 4(3) (4)
= 16(3) – 48
= 48 – 48
= 0
So,
Discriminate, D = 0
D = 0
∴ Roots are real and equal.
(ii) x² – 1/2x + 4 = 0
Let us consider,
a = 1, b = -1/2, c = 4
By using the formula,
D = b2 – 4ac
= (-1/2)2 – 4(1) (4)
= 1/4 – 16
= -63/4
So,
Discriminate, D = -63/4
D < 0
∴ Roots are not real.
(iii) – 2x² + x + 1 = 0
Let us consider,
a = -2, b = 1, c = 1
By using the formula,
D = b2 – 4ac
= (1)2 – 4(-2) (1)
= 1 + 8
= 9
So,
Discriminate, D = 9
D > 0
∴ Roots are real and distinct.
(iv) 2√3x² – 5x + √3 = 0
Let us consider,
a = 2√3, b = -5, c = √3
By using the formula,
D = b2 – 4ac
= (-5)2 – 4(2√3) (√3)
= 25 – 24
= 1
So,
Discriminate, D = 1
D > 0
∴ Roots are real and distinct.
3. Find the nature of the roots of the following quadratic equations:
(i) x² – 1/2x – 1/2 = 0
(ii) x² – 2√3x – 1 = 0
If real roots exist, find them.
Solution:
(i) x² – 1/2x – 1/2 = 0
Let us consider,
a = 1, b = -1/2, c = -1/2
By using the formula,
D = b2 – 4ac
= (-1/2)2 – 4(1) (-1/2)
= 1/4 + 2
= (1+8)/4
= 9/4
So,
Discriminate, D = 9/4
D > 0
∴ Roots are real and unequal.
(ii) x² – 2√3x – 1 = 0
Let us consider,
a = 1, b = 2√3, c = -1
By using the formula,
D = b2 – 4ac
= (2√3)2 – 4(1) (-1)
= 12 + 4
= 16
So,
Discriminate, D = 16
D > 0
∴ Roots are real and unequal.
4. Without solving the following quadratic equation, find the value of ‘p’ for which the given equations have real and equal roots:
(i) px² – 4x + 3 = 0
(ii) x² + (p – 3)x + p = 0
Solution:
(i) px² – 4x + 3 = 0
Let us consider,
a = p, b = -4, c = 3
By using the formula,
D = b2 – 4ac
= (-4)2 – 4(p) (3)
= 16 – 12p
Since, roots are real.
16 – 12p = 0
16 = 12p
p = 16/12
= 4/3
∴ p = 4/3
(ii) x² + (p – 3)x + p = 0
Let us consider,
a = 1, b = (p – 3), c = p
By using the formula,
D = b2 – 4ac
= (p – 3)2 – 4(1) (p)
= p2 – 32 – 2(3) (p) – 4p
= p2 + 9 – 6p – 4p
= p2 – 10p + 9
Since, roots are real and have equal roots.
p2 – 10p + 9 = 0
Now let us factorize,
p2 – 9p – p + 9 = 0
p(p – 9) – 1 (p – 9) = 0
(p – 9) (p – 1) = 0
So,
(p – 9) = 0 or (p – 1) = 0
p = 9 or p = 1
∴ p = 1, 9
5. Find the value (s) of k for which each of the following quadratic equation has equal roots:
(i) x² + 4kx + (k2 – k + 2) = 0
(ii) (k – 4)x2 + 2(k – 4)x + 4 = 0
Solution:
(i) x² + 4kx + (k2 – k + 2) = 0
Let us consider,
a = 1, b = 4k, c = k2 – k + 2
By using the formula,
D = b2 – 4ac
= (4k)2 – 4(1) (k2 – k + 2)
= 16k2 – 4k2 + 4k – 8
= 12k2 + 4k – 8
As, roots are equal, D = 0
12k2 + 4k – 8 = 0
Dividing by 4 on both sides, we get
3k2 + k – 2 = 0
3k2 + 3k – k – 2 = 0
3k(k + 1) – 1(k + 2) = 0
(3k – 1) (k + 2) = 0
So,
(3k – 1) = 0 or (k + 2) = 0
k = 1/3 or k = -2
∴ k = 1/3, -2
(ii) (k – 4)x² + 2(k – 4)x + 4 = 0
Let us consider,
a = (k – 4), b = 2(k – 4), c = 4
By using the formula,
D = b2 – 4ac
= (2(k – 4))2 – 4(k – 4) (4)
= (4(k2 + 16 – 8k)) – 16(k – 4)
= 4(k2 – 8k + 16) – 16k + 64
= 4 [k2 – 8k + 16 – 4k + 16]
= 4 [k2 – 12k + 32]
Since, roots are equal.
4 [k2 – 12k + 32] = 0
k2 – 12k + 32 = 0
Now let us factorize,
k2 – 8k – 4k + 32 = 0
k(k – 8) – 4 (k – 8) = 0
(k – 8) (k – 4) = 0
So,
(k – 8) = 0 or (k – 4) ≠ 0
k = 8 or k ≠ 4
∴ k = 8
6. Find the value(s) of m for which each of the following quadratic equation has real and equal roots:
(i) (3m + 1)x² + 2(m + 1)x + m = 0
(ii) x² + 2(m – 1) x + (m + 5) = 0
Solution:
(i) (3m + 1)x² + 2(m + 1)x + m = 0
Let us consider,
a = (3m + 1), b = 2(m + 1), c = m
By using the formula,
D = b2 – 4ac
= (2(m + 1))2 – 4 (3m + 1) (m)
= 4(m2 + 1 + 2m) – 4m(3m + 1)
= 4(m2 + 2m + 1) – 12m2 – 4m
= 4m2 + 8m + 4 – 12m2 – 4m
= -8m2 + 4m + 4
Since, roots are equal.
D = 0
-8m2 + 4m + 4 = 0
Divide by 4, we get
-2m2 + m + 1 = 0
2m2 – m – 1 = 0
Now let us factorize,
2m2 – 2m + m – 1 = 0
2m(m – 1) +1 (m – 1) = 0
(m – 1) (2m + 1) = 0
So,
(m – 1) = 0 or (2m + 1) = 0
m = 1 or 2m = -1
m = 1 or m = -1/2
∴ m = 1, -1/2
(ii) x² + 2(m – 1) x + (m + 5) = 0
Let us consider,
a = 1, b = 2(m – 1), c = (m + 5)
By using the formula,
D = b2 – 4ac
= (2(m – 1))2 – 4 (1) (m + 5)
= [4(m2 + 1 – 2m)] – 4m – 20
= 4m2 – 8m + 4 – 4m – 20
= 4m2 – 12m – 16
Since roots are equal.
D = 0
4m2 – 12m – 16 = 0
Divide by 4, we get.
m2 – 3m – 4 = 0
Now let us factorize,
m2 – 4m + m – 4 = 0
m(m – 4) + 1 (m – 4) = 0
(m – 4) (m + 1) = 0
So,
(m – 4) = 0 or (m + 1) = 0
m = 4 or m = -1
∴ m = 4, -1
7. Find the values of k for which each of the following quadratic equation has equal roots:
(i) 9x² + kx + 1 = 0
(ii) x² – 2kx + 7k – 12 = 0
Also, find the roots for those values of k in each case.
Solution:
(i) 9x² + kx + 1 = 0
Let us consider,
a = 9, b = k, c = 1
By using the formula,
D = b2 – 4ac
= (k)2 – 4 (9) (1)
= k2 – 36
Since, roots are equal.
D = 0
k2 – 36 = 0
(k + 6) (k – 6) = 0
So,
(k + 6) = 0 or (k – 6) = 0
k = -6 or k = 6
∴ k = 6, -6
Now, let us substitute in the equation
When k = 6, then
9x² + kx + 1 = 0
9x2 + 6x + 1 = 0
(3x)2 + 2(3x)(1) + 12 = 0
(3x + 1)2 = 0
3x + 1 = 0
3x = -1
x = -1/3, -1/3
When k = -6, then
9x² + kx + 1 = 0
9x2 – 6x + 1 = 0
(3x)2 – 2(3x)(1) + 12 = 0
(3x – 1)2 = 0
3x – 1 = 0
3x = 1
x = 1/3, 1/3
(ii) x² – 2kx + 7k – 12 = 0
Let us consider,
a = 1, b = -2k, c = (7k – 12)
By using the formula,
D = b2 – 4ac
= (-2k)2 – 4 (1) (7k – 12)
= 4k2 – 28k + 48
Since roots are equal.
D = 0
4k2 – 28k + 48 = 0
Divide by 4, we get
k2 – 7k + 12 = 0
Now let us factorize,
k2 – 3k – 4k + 12 = 0
k(k – 3) – 4 (k – 3) = 0
(k – 3) (k – 4) = 0
So,
(k – 3) = 0 or (k – 4) = 0
k = 3 or k = 4
∴ k = 3, 4
Now, let us substitute in the equation
When k = 3, then
By using the formula,

= [-(-2k) ± √0] / 2(1)
= [2(3)]/2
= 3
x = 3, 3
When k = 4, then
By using the formula,

= [-(-2k) ± √0] / 2(1)
= [2(4)] / 2
= 8/2
= 4
x = 4, 4
8. Find the value(s) of p for which the quadratic equation (2p + 1)x² – (7p + 2)x + (7p – 3) = 0 has equal roots. Also find these roots.
Solution:
Given:
(2p + 1)x² – (7p + 2)x + (7p – 3) = 0
Let us compare with ax2+ bx + c = 0
So we get,
a = (2p + 1), b = – (7p + 2), c = (7p – 3)
By using the formula,
D = b2 – 4ac
0 = (– (7p + 2))2 – 4 (2p + 1) (7p – 3)
= 49p2 + 4 + 28p – 4[14p2 – 6p + 7p – 3]
= 49p2 + 4 + 28p – 56p2 – 4p + 12
= -7p2 + 24p + 16
Let us factorize,
-7p2 + 28p – 4p + 16 = 0
-7p(p – 4) – 4 (p – 4) = 0
(p – 4) (-7p – 4) = 0
So,
(p – 4) = 0 or (-7p – 4) = 0
p = 4 or -7p = 4
p = 4 or p = -4/7
∴ Value of p = 4, -4/7
9. Find the value(s) of p for which the equation 2x² + 3x + p = 0 has real roots.
Solution:
Given:
2x² + 3x + p = 0
Let us consider,
a = 2, b = 3, c = p
By using the formula,
D = b2 – 4ac
= (3)2 – 4 (2) (p)
= 9 – 8p
Since, roots are real.
9 – 8p ≥ 0
9 ≥ 8p
8p ≤ 9
p ≤ 9/8
10. Find the least positive value of k for which the equation x² + kx + 4 = 0 has real roots.
Solution:
Given:
x² + kx + 4 = 0
Let us consider,
a = 1, b = k, c = 4
By using the formula,
D = b2 – 4ac
= (k)2 – 4 (1) (4)
= k2 – 16
Since, roots are real and positive.
k2 – 16 ≥ 0
k2 ≥ 16
k ≥ 4
k = 4
∴ Value of k = 4
11. Find the values of p for which the equation 3x² – px + 5 = 0 has real roots.
Solution:
Given:
3x² – px + 5 = 0
Let us consider,
a = 3, b = -p, c = 5
By using the formula,
D = b2 – 4ac
= (-p)2 – 4 (3) (5)
= p2 – 60
Since, roots are real.
p2 – 60 ≥ 0
p2 ≥ 60
p ≥ ± √60
p ≥ ± 2√15
p ≥ + 2√15 or p ≤ -2√15
∴ Value of p = 2√15, -2√15
EXERCISE 5.5
1. (i) Find two consecutive natural numbers such that the sum of their squares is 61.
(ii) Find two consecutive integers such that the sum of their squares is 61.
Solution:
(i) Find two consecutive natural numbers such that the sum of their squares is 61.
Let us consider first natural number be ‘x’
Second natural number be ‘x + 1’
So according to the question,
x2 + (x + 1)2 = 61
let us simplify the expression,
x2 + x2 + 12 + 2x – 61 = 0
2x2 + 2x – 60 = 0
Divide by 2, we get
x2 + x – 30 = 0
Let us factorize,
x2 + 6x – 5x – 30 = 0
x(x + 6) – 5 (x + 6) = 0
(x + 6) (x – 5) = 0
So,
(x + 6) = 0 or (x – 5) = 0
x = -6 or x = 5
∴ x = 5 [Since -6 is not a positive number]
Hence the first natural number = 5
Second natural number = 5 + 1 = 6
(ii) Find two consecutive integers such that the sum of their squares is 61.
Let us consider first integer number be ‘x’
Second integer number be ‘x + 1’
So according to the question,
x2 + (x + 1)2 = 61
let us simplify the expression,
x2 + x2 + 12 + 2x – 61 = 0
2x2 + 2x – 60 = 0
Divide by 2, we get
x2 + x – 30 = 0
Let us factorize,
x2 + 6x – 5x – 30 = 0
x(x + 6) – 5 (x + 6) = 0
(x + 6) (x – 5) = 0
So,
(x + 6) = 0 or (x – 5) = 0
x = -6 or x = 5
Now,
If x = -6, then
First integer number = -6
Second integer number = -6 + 1 = -5
If x = 5, then
First integer number = 5
Second integer number = 5 + 1 = 6
2. (i) If the product of two positive consecutive even integers is 288, find the integers.
(ii) If the product of two consecutive even integers is 224, find the integers.
(iii) Find two consecutive even natural numbers such that the sum of their squares is 340.
(iv) Find two consecutive odd integers such that the sum of their squares is 394.
Solution:
(i) If the product of two positive consecutive even integers is 288, find the integers.
Let us consider first positive even integer number be ‘2x’
Second even integer number be ‘2x + 2’
So according to the question,
2x × (2x + 2) = 288
4x2 + 4x – 288 = 0
Divide by 4, we get
x2 + x – 72 = 0
Let us factorize,
x2 + 9x – 8x – 72 = 0
x(x + 9) – 8(x + 9) = 0
(x + 9) (x – 8) = 0
So,
(x + 9) = 0 or (x – 8) = 0
x = -9 or x = 8
∴ Value of x = 8 [since, -9 is not positive]
First even integer = 2x = 2 (8) = 16
Second even integer = 2x + 2 = 2(8) + 2 = 18
(ii) If the product of two consecutive even integers is 224, find the integers.
Let us consider first positive even integer number be ‘2x’
Second even integer number be ‘2x + 2’
So according to the question,
2x × (2x + 2) = 224
4x2 + 4x – 224 = 0
Divide by 4, we get
x2 + x – 56 = 0
Let us factorize,
x2 + 8x – 7x – 56 = 0
x(x + 8) – 7(x + 8) = 0
(x + 8) (x – 7) = 0
So,
(x + 8) = 0 or (x – 7) = 0
x = -8 or x = 7
∴ Value of x = 7 [since, -8 is not positive]
First even integer = 2x = 2 (7) = 14
Second even integer = 2x + 2 = 2(7) + 2 = 16
(iii) Find two consecutive even natural numbers such that the sum of their squares is 340.
Let us consider first positive even natural number be ‘2x’
Second even number be ‘2x + 2’
So according to the question,
(2x)2 + (2x + 2)2 = 340
4x2 + 4x2 + 8x + 4 – 340 = 0
8x2 + 8x – 336 = 0
Divide by 8, we get
x2 + x – 42 = 0
Let us factorize,
x2 + 7x – 6x – 56 = 0
x(x + 7) – 6(x + 7) = 0
(x + 7) (x – 6) = 0
So,
(x + 7) = 0 or (x – 6) = 0
x = -7 or x = 6
∴ Value of x = 6 [since, -7 is not positive]
First even natural number = 2x = 2 (6) = 12
Second even natural number = 2x + 2 = 2(6) + 2 = 14
(iv) Find two consecutive odd integers such that the sum of their squares is 394.
Let us consider first odd integer number be ‘2x + 1’
Second odd integer number be ‘2x + 3’
So according to the question,
(2x + 1)2 + (2x + 3)2 = 394
4x2 + 4x + 1 + 4x2 + 12x + 9 – 394 = 0
8x2 + 16x – 384 = 0
Divide by 8, we get
x2 + 2x – 48 = 0
Let us factorize,
x2 + 8x – 6x – 48 = 0
x(x + 8) – 6(x + 8) = 0
(x + 8) (x – 6) = 0
So,
(x + 8) = 0 or (x – 6) = 0
x = -8 or x = 6
When x = -8, then
First odd integer = 2x + 1 = 2 (-8) + 1 = -16 + 1 = -15
Second odd integer = 2x + 3 = 2(-8) + 3 = -16 + 3 = -13
When x = 6, then
First odd integer = 2x + 1 = 2 (6) + 1 = 12 + 1 = 13
Second odd integer = 2x + 3 = 2(6) + 3 = 12 + 3 = 15
∴ The required odd integers are -15, -13, 13, 15.
3. The sum of two numbers is 9 and the sum of their squares is 41. Taking one number as x, form ail equation in x and solve it to find the numbers.
Solution:
Given:
Sum of two numbers = 9
Let us consider the first number to be ‘x’
Second number is ‘9 – x’
So, according to the question,
(x)2 + (9 – x)2 = 41
x2 + 81 – 18x + x2 – 41 = 0
2x2 – 18x + 40 = 0
Divide by 2, we get
x2 – 9x + 20 = 0
Let us factorize,
x2 – 4x – 5x + 20 = 0
x(x – 4) – 5(x – 4) = 0
(x – 4) (x – 5) = 0
So,
(x – 4) = 0 or (x – 5) = 0
x = 4 or x = 5
When x = 4, then
First number = x = 4
Second number = 9 – x = 9 – 4 = 5
When x = 5, then
First number = x = 5
Second number = 9 – x = 9 – 5 = 4
∴ The required numbers are 4 and 5.
4. Five times a certain whole number is equal to three less than twice the square of the number. Find the number.
Solution:
Let us consider the number to be ‘x’
So according to the question,
5x = 2x2 – 3
2x2 – 3 – 5x = 0
2x2 – 5x – 3 = 0
Let us factorize,
2x2 – 6x + x – 3 = 0
2x(x – 3) + 1(x – 3) = 0
(x – 3) (2x + 1) = 0
So,
(x – 3) = 0 or (2x + 1) = 0
x = 3 or 2x = -1
x = 3 or x = -1/2
∴ The required number is 3 [since, -1/2 cannot be a whole number].
5. Sum of two natural numbers is 8 and the difference of their reciprocals is 2/15. Find the numbers.
Solution:
Let us consider two numbers as ‘x’ and ‘y’
So according to the question,
1/x – 1/y = 2/15 ….. (i)
It is given that, x + y = 8
So, y = 8 – x … (ii)
Now, substitute the value of y in equation (i), we get
1/x – 1/(8 – x) 2/15
By taking LCM,
[8 – x – x] / x(8 – x) = 2/15(8 – 2x) / x(8 – x) = 2/15
By cross-multiplying,
15(8 – 2x) = 2x(8 – x)
120 – 30x = 16x – 2x2
120 – 30x – 16x + 2x2 = 0
2x2 – 46x + 120 = 0
Divide by 2, we get
x2 – 23x + 60 = 0
let us factorize,
x2 – 20x – 3x + 60 = 0
x(x – 20) – 3 (x – 20) = 0
(x – 20) (x – 3) = 0
So,
(x – 20) = 0 or (x – 3) = 0
x = 20 or x = 3
Now,
Sum of two natural numbers, y = 8 – x = 8 – 20 = -12, which is a negative value.
So value of x = 3, y = 8 – x = 8 – 3 = 5
∴ The value of x and y are 3 and 5.
6. The difference between the squares of two numbers is 45. The square of the smaller number is 4 times the larger number. Determine the numbers.
Solution:
Let us consider the larger number to be ‘x’
Smaller number be ‘y’
So, according to the question,
x2 – y2 = 45 … (i)
y2 = 4x … (ii)
Now substitute the value of y in equation (i), we get
x2 – 4x = 45
x2 – 4x – 45 = 0
let us factorize,
x2 – 9x + 5x – 45 = 0
x(x – 9) + 5 (x – 9) = 0
(x – 9) (x + 5) = 0
So,
(x – 9) = 0 or (x + 5) = 0
x = 9 or x = -5
When x = 9, then
The larger number = x = 9
Smaller number = y => y2 = 4x
y = √4x = √4(9) = √36 = 6
When x = -5, then
The larger number = x = -5
Smaller number = y => y2 = 4x
y = √4x = √4(-5) = √-20 (which is not possible)
∴ The value of x and y are 9, 6.
7. There are three consecutive positive integers such that the sum of the square of the first and the product of the other two is 154. What are the integers?
Solution:
Let us consider the first integer to be ‘x’
Second integer be ‘x + 1’
Third integer be ‘x + 2’
So, according to the question,
x2 + (x + 1) (x + 2) = 154
let us simplify,
x2 + x2 + 3x + 2 – 154 = 0
2x2 + 3x – 152 = 0
Let us factorize,
2x2 + 19x – 16x – 152 = 0
x(2x + 19) – 8 (2x + 19) = 0
(2x + 19) (x – 8) = 0
So,
(2x + 19) = 0 or (x – 8) = 0
2x = -19 or x = 8
x = -19/2 or x = 8
∴ The value of x = 8 [since -19/2 is a negative value]
So,
First integer = x = 8
Second integer = x + 1 = 8 + 1 = 9
Third integer = x + 2 = 8 + 2 = 10
∴ The numbers are 8, 9, 10.
8. (i) Find three successive even natural numbers, the sum of whose squares is 308.
(ii) Find three consecutive odd integers, the sum of whose squares is 83.
Solution:
(i) Find three successive even natural numbers, the sum of whose squares is 308.
Let us consider first even natural number be ‘2x’
Second even number be ‘2x + 2’
Third even number be ‘2x + 4’
So, according to the question,
(2x)2 + (2x + 2)2 + (2x + 4)2 = 308
4x2 + 4x2 + 8x + 4 + 4x2 + 16x + 16 – 308 = 0
12x2 + 24x – 288 = 0
Divide by 12, we get
x2 + 2x – 24 = 0
Let us factorize,
x2 + 6x – 4x – 24 = 0
x(x + 6) – 4(x + 6) = 0
(x + 6) (x – 4) = 0
So,
(x + 6) = 0 or (x – 4) = 0
x = -6 or x = 4
∴ Value of x = 4 [since, -6 is not positive]
First even natural number = 2x = 2 (4) = 8
Second even natural number = 2x + 2 = 2(4) + 2 = 10
Third even natural number = 2x + 4 = 2(4) + 4 = 12
∴ The numbers are 8, 10, 12.
(ii) Find three consecutive odd integers, the sum of whose squares is 83.
Let the three numbers be ‘x’, ‘x + 2’, ‘x + 4’
So, according to the question,
(x)2 + (x + 2)2 + (x + 4)2 = 83
x2 + x2 + 4x + 4 + x2 + 8x + 16 – 83 = 0
3x2 + 12x – 63 = 0
Divide by 3, we get
x2 + 4x – 21 = 0
let us factorize,
x2 + 7x – 3x – 21 = 0
x(x + 7) – 3 (x + 7) = 0
(x + 7) (x – 3) = 0
So,
(x + 7) = 0 or (x – 3) = 0
x = -7 or x = 3
∴ The numbers will be x, x+2, x+4 => -7, -7+2, -7+4 => -7, -5, -3
Or the numbers will be x, x+2, x+4 => 3, 3+2, 3+4, => 3, 5, 7
9. In a certain positive fraction, the denominator is greater than the numerator by 3. If 1 is subtracted from both the numerator and denominator, the fraction is decreased by 1/14. Find the fraction.
Solution:
Let the numerator be ‘x’
and the denominator be ‘x+3’
So the fraction is x/(x+3)
According to the question,

By cross multiplying, we get
(x – 1) (14x + 42) = (x + 2) (13x – 3)
14x2 + 42x – 14x – 42 = 13x2 – 3x + 26x – 6
14x2 + 42x – 14x – 42 – 13x2 + 3x – 26x + 6 = 0
x2 + 5x – 36 = 0
let us factorize,
x2 + 9x – 4x – 36 = 0
x(x + 9) – 4 (x + 9) = 0
(x + 9) (x – 4) = 0
So,
(x + 9) = 0 or (x – 4) = 0
x = -9 or x = 4
So the value of x = 4 [since, -9 is a negative number]
When substitute the value of x = 4 in the fraction x/(x+3), we get
4/(4+3) = 4/7
∴ The required fraction is = 4/7
10. The sum of the numerator and denominator of a certain positive fraction is 8. If 2 is added to both the numerator and denominator, the fraction is increased by 4/35. Find the fraction.
Solution:
Let the denominator be ‘x’
So the numerator will be ‘8-x’
The obtained fraction is (8-x)/x
So according to the question,

By cross-multiplying,
35(4x – 16) = 4(x2 + 2x)
140x – 560 = 4x2 + 8x
4x2 + 8x – 140x + 560 = 0
4x2 – 132x + 560 = 0
Divide by 4, we get
x2 – 33x + 140 = 0
let us factorize,
x2 – 28x – 5x + 140 = 0
x(x – 28) – 5 (x – 28) = 0
(x – 28) (x – 5) = 0
So,
(x – 28) = 0 or (x – 5) = 0
x = 28 or x = 5
So the value of x = 5 [since x = 28 is not possible as the sum of the numerator and denominator is 8]
When substitute the value of x = 5 in the fraction (8-x)/x, we get
(8 – 5)/5 = 3/5
∴ The required fraction is = 3/5
11. A two digit number contains the bigger at ten’s place. The product of the digits is 27 and the difference between two digits is 6. Find the number.
Solution:
Let us consider the unit’s digit to be ‘x’
Ten’s digit = x+6
Number = x + 10(x+6)
= x + 10x + 60
= 11x + 60
So according the question,
x(x + 6) = 27
x2 + 6x – 27 = 0
let us factorize,
x2 + 9x – 3x – 27 = 0
x(x + 9) – 3 (x + 9) = 0
(x + 9) (x – 3) = 0
So,
(x + 9) = 0 or (x – 3) = 0
x = -9 or x = 3
so, value of x = 3 [since, -9 is a negative number]
∴ The number = 11x + 60
= 11(3) + 60
= 33 + 60
= 93
12. A two digit positive number is such that the product of its digits is 6. If 9 is added to the number, the digits interchange their places. Find the number. (2014)
Solution:
Let us consider 2-digit number to be ‘xy’ = 10x + y
Reversed digits = yx = 10y + x
So, according to the question,
10x + y + 9 = 10y + x
It is given that,
xy = 6
y = 6/x
so, by substituting the value in the above equation, we get
10x + 6/x + 9 = 10(6/x) + x
By taking LCM,
10x2 + 6 + 9x = 60 + x2
10x2 + 6 + 9x – 60 – x2 = 0
9x2 + 9x – 54 = 0
Divide by 9, we get
x2 + x – 6 = 0
let us factorize,
x2 + 3x – 2x – 6 = 0
x(x + 3) – 2 (x + 3) = 0
(x + 3) (x – 2) = 0
So,
(x + 3) = 0 or (x – 2) = 0
x = -3 or x = 2
Value of x = 2 [since, -3 is a negative value]
Now, substitute the value of x in y = 6/x, we get
y = 6/2 = 3
∴ 2-digit number = 10x + y = 10(2) + 3 = 23
13. A rectangle of area 105 cm² has its length equal to x cm. Write down its breadth in terms of x. Given that the perimeter is 44 cm, write down an equation in x and solve it to determine the dimensions of the rectangle.
Solution:
Given:
Perimeter of rectangle = 44cm
Length + breadth = 44/2 = 22cm
Let us consider length to be ‘x’
Breadth is ’22 – x’
So, according to the question,
x(22 – x) = 105
22x – x2 – 105 = 0
x2 – 22x + 105 = 0
let us factorize,
x2 – 15x – 7x + 105 = 0
x(x – 15) – 7(x – 15) = 0
(x – 15) (x – 7) = 0
So,
(x – 15) = 0 or (x – 7) = 0
x = 15 or x = 7
Since length > breadth, x = 7 is not admissible.
∴ Length = 15cm
Breadth = 22 – x = 22 – 15 = 7cm
14. A rectangular garden 10 m by 16 m is to be surrounded by a concrete walk of uniform width. Given that the area of the walk is 120 square meters, assuming the width of the walk to be x, form an equation in x and solve it to find the value of x. (1992)
Solution:
Given:
Length of garden = 16cm
Width = 10cm
Let the width of the walk be ‘x’ meter
Outer length = 16 + 2x
Outer width = 10 + 2x
So, according to the question,
(16 + 2x) (10 + 2x) – 16(10) = 120
160 + 32x + 20x + 4x2 – 160 – 120 = 0
4x2 + 52x – 120 = 0
Divide by 4, and we get
x2 + 13x – 30 = 0
x2 + 15x – 2x – 30 = 0
x(x + 15) – 2 (x + 15) = 0
(x + 15) (x – 2) = 0
So,
(x + 15) = 0 or (x – 2) = 0
x = -15 or x = 2
∴ The value of x is 2 [Since, -15 is a negative value]
15. The length of a rectangle exceeds its breadth by 5 m. If the breadth was doubled and the length reduced by 9 m, the area of the rectangle would have increased by 140 m². Find its dimensions.
Solution:
(ii) In the first case:
Let us consider the length of the rectangle to be ‘x’ meter
Width = (x – 5) meter
Area = lb
= x(x – 5) sq.m
In the second case:
Length = (x – 9) meter
Width = 2 (x – 5) meter
Area = (x – 9) 2(x – 5) = 2(x – 9) (x – 5) sq.m
So, according to the question,
2(x – 9) (x – 5) = x(x – 5) + 140
2(x2 – 14x + 45) = x2 – 5x + 140
2x2 – 28x + 90 – x2 + 5x – 140 = 0
x2 – 23x – 50 = 0
let us factorize,
x2 – 25x + 2x – 50 = 0
x(x – 25) + 2 (x – 25) = 0
(x – 25) (x + 2) = 0
So,
(x – 25) = 0 or (x + 2) = 0
x = 25 or x = -2
∴ Length of the first rectangle = 25meters. [Since, -2 is a negative value]
Width = x – 5 = 25 – 5 = 20meters
Area = lb
= 25 × 20 = 500 m2
16. The perimeter of a rectangular plot is 180 m and its area is 1800 m². Take the length of the plot as x m. Use the perimeter 180 m to write the value of the breadth in terms of x. Use the values of length, breadth and the area to write an equation in x. Solve the equation to calculate the length and breadth of the plot. (1993)
Solution:
Given,
The perimeter of a rectangular field = 180 m
And area = 1800 m²
Let’s assume the length of the rectangular field as ‘x’ m
We know that,
Perimeter of rectangular field = 2 (length + breadth)
So, (length + breadth) = perimeter/ 2
x + breadth = 180/2
⇒ breadth = 90 – x
Now, the area of the rectangular field is given as
Length × breadth = 1800
x × (90 – x) = 1800
90x – x2 = 1800
x2 – 90x + 1800 = 0
Upon factorization, we have
x2 – 60x – 30x + 1800 = 0
x(x – 60) – 30(x – 60) = 0
(x – 30) (x – 60) = 0
So,
x – 30 = 0 or x – 60 = 0
x = 30 or x = 60
As length is greater than its breadth,
Therefore, for the rectangular field
Length = 60 m and breadth = (90 – 60) = 30 m
17. The lengths of the parallel sides of a trapezium are (x + 9) cm and (2x – 3) cm and the distance between them is (x + 4) cm. If its area is 540 cm², find x.
Solution:
We know that,
Area of a trapezium = ½ × (sum of parallel sides) × (height)
Given, the length of parallel sides are (x + 9) and (2x – 3)
And height = (x + 4)
Now, according to the conditions in the problem
½ × (x + 9 + 2x – 3) × (x + 4) = 540
(3x + 6) (x + 4) = 540 × 2
3x2 + 12x + 6x + 24 = 1080
3x2 + 18x – 1056 = 0
x2 + 6x – 352 = 0 [Dividing by 3]
By factorization method, we have
x2 + 22x – 16x – 352 = 0
x(x + 22) – 16(x + 22) = 0
(x – 16) (x + 22) = 0
So,
x – 16 = 0 or x + 22 = 0
x = 16 or x = -22
As measurements cannot be negative x = -22 is not possible
Therefore, x = 16
18. If the perimeter of a rectangular plot is 68 m and the length of its diagonal is 26 m, find its area.
Solution:
Given,
Perimeter = 68 m and diagonal = 26 m
So, Length + breadth = Perimeter/2
= 68/2
= 34 m
Let’s consider the length of the rectangular plot to be ‘x’ m
Then, breadth = (34 – x) m
Now, the diagonal of the rectangular plot is given by
length2 + breadth2 = diagonal2 [By Pythagoras Theorem]
x2 + (34 – x)2 = 262
x2 + 1156 + x2 – 68x = 676
2x2 – 68x + 1156 – 676 = 0
2x2 – 68x + 480 = 0
x2 – 34x + 240 = 0 [Dividing by 2]
By factorization method, we have
x2 – 24x – 10x + 240 = 0
x(x – 24) – 10(x – 24) = 0
(x – 10) (x – 24) = 0
So,
x – 10 = 0 or x – 24 = 0
x = 10 or x = 24
As length is greater than breadth,
Thus, length = 24 m and breadth = (34 – 24) m = 10 m
And, the area of the rectangular plot = 24 × 10 = 240 m2.
19. If the sum of two smaller sides of a right-angled triangle is 17cm and the perimeter is 30cm, then find the area of the triangle.
Solution:
Given,
The perimeter of the triangle = 30 cm
Let’s assume the length of one of the two small sides as x cm
Then, the other side will be (17 – x) cm
Now, the length of hypotenuse = perimeter – the sum of the other two sides
= (30 – 17) cm
= 13 cm
According to the problem, by Pythagoras theorem, we have
x2 + (17 – x)2 = 132
x2 + 289 + x2 – 34x = 169
2x2 – 34x + 289 – 169 = 0
2x2 – 34x + 120 = 0
x2 – 17x + 60 = 0 [Dividing by 2]
By factorization method, we have
x2 – 12x – 5x + 60 = 0
x(x – 12) – 5(x – 12) = 0
(x – 5) (x – 12) = 0
So,
(x – 5) = 0 or (x – 12) = 0
x = 5 or x = 12
When, x = 5
First side = 5 cm and second side = (17 – 5) = 12 cm
And when x = 12
First side = 12 cm and second side = (17 – 12) = 5 cm
Thus,
Area of the triangle = ½ (5 × 12)
= 60/2
= 30 cm2
20. The hypotenuse of grassy land in the shape of a right triangle is 1 metre more than twice the shortest side. If the third side is 7 metres more than the shortest side, find the sides of the grassy land.
Solution:
Let’s consider the shortest side to be ‘x’ cm
Hypotenuse = 2x + 1
And third side = x + 7
Now, by Pythagoras theorem, we have
(2x + 1)2 = x2 + (x + 7)2
4x2 + 1 + 4x = x2 + x2 + 49 + 14x
4x2 – 2x2 + 4x – 14x + 1 – 49 = 0
2x2 – 10x – 48 = 0
x2 – 5x – 24 = 0 [Dividing by 2]
By factorization method, we have
x2 – 8x + 3x – 24 = 0
x(x – 8) + 3(x – 8) = 0
(x – 8) (x + 3) = 0
So,
x – 8 = 0 or x + 3 = 0
x = 8 or x = -3
As measurement of a side cannot be negative, x = 8
Therefore,
The shortest side = 8 m
Third side = x + 7 = 8 + 7 = 13 m
And hypotenuse = 2x + 1 = 8 × 2 + 1 = 16 + 1 = 17 m
Chapter Test
Solve the following equations (1 to 4) by factorisation:
1.(i) x² + 6x – 16 = 0 (ii) 3x² + 11x + 10 = 0
Solution:
(i) x² + 6x – 16 = 0
Let us factorize the given expression,
x2 + 8x – 2x – 16 = 0 [As 8 × (-2) = -16 and 8 – 2 = 6]
x(x + 8) – 2(x + 8) = 0
(x – 2) (x + 8) = 0
So now,
(x – 2) = 0 or (x + 8) = 0
x = 2 or x = -8
∴ Value of x = 2, -8
(ii) 3x² + 11x + 10 = 0
Let us factorize the given expression,
3x2 + 6x + 5x + 10 = 0 [As 3 × 10 = 30 and 6 + 5 = 11]
3x(x + 2) + 5(x + 2) = 0
(3x + 5) (x + 2) = 0
So now,
(3x + 5) = 0 or (x + 2) = 0
3x = -5 or x = -2
x = -5/3 or x = -2
∴ Value of x = -5/3, -2
2. (i) 2x² + ax – a² = 0 (ii) √3x² + 10x + 7√3 = 0
Solution:
(i) 2x² + ax – a² = 0
Let us factorize the given expression,
2x2 + 2ax – ax – a2 = 0 [As 2 × (-a2) = -2a2 and 2a – a = a]
2x(x + a) – a(x + a) = 0
(2x – a) (x + a) = 0
So now,
(2x – a) = 0 or (x + a) = 0
2x = a or x = -a
x = a/2 or x = -a
∴ Value of x = a/2, -a
(ii) √3x² + 10x + 7√3 = 0
Let us factorize the given expression,
√3x² + 3x + 7x + 7√3 = 0 [As √3 × (7√3) = 7 × (√3)2 = 21 and 7 + 3 = 10]
√3x(x + √3) + 7(x + √3) = 0
(√3x + 7) (x + √3) = 0
So now,
(√3x + 7) = 0 or (x + √3) = 0
√3x = -7 or x = -√3
x = -7/√3 or x = -√3
∴ Value of x = -7/√3, -√3
3. (i) x(x + 1) + (x + 2)(x + 3) = 42 (ii) 6/x – 2/(x – 1) = 1/(x – 2)
Solution:
(i) x(x + 1) + (x + 2)(x + 3) = 42
Let us simplify the given expression,
x2 + x + x2 + 2x + 3x + 6 = 42
2x2 + 6x + 6 – 42 = 0
2x2 + 6x – 36 = 0
x2 + 3x – 18 = 0 [Dividing by 2]
Now, let us factorize
x2 + 6x – 3x – 18 = 0 [As 6 × (-3) = -18 and 6 – 3 = 3]
x(x + 6) -3(x + 6) = 0
(x + 6) (x – 3) = 0
So now,
(x + 6) = 0 or (x – 3) = 0
x = -6 or x = 3
∴ Value of x = -6, 3
(ii) 6/x – 2/(x – 1) = 1/(x – 2)
Let us simplify the given expression,
[6(x – 1) – 2x]/ [x(x – 1)] = 1/(x – 2) [Taking L.C.M](6x – 6 – 2x)/(x2– x) = 1/(x – 2)
(4x – 6)/(x2– x) = 1/(x – 2)
(4x – 6) (x – 2) = (x2– x)
4x2 – 6x – 8x + 12 = x2– x
4x2 – x2 – 14x + x + 12 = 0
3x2 – 13x + 12 = 0
Now, let us factorize
3x2 – 9x – 4x + 12 = 0
3x(x – 3) – 4(x – 3) = 0
(3x – 4) (x – 3) = 0
So now,
(3x – 4) = 0 or (x – 3) = 0
3x = 4 or x = 3
x = 4/3 or x = 3
∴ Value of x = 4/3, 3
4. (i) √(x + 15) = x + 3 (ii) √(3x2 – 2x – 1) = 2x – 2
Solution:
(i) √(x + 15) = x + 3
Let us simplify the given expression,
x + 15 = (x + 3)2 [Squaring on both sides]
x + 15 = x2 + 9 + 6x
x2 + 6x – x + 9 – 15 = 0
x2 + 5x – 6 = 0
Now, let us factorize
x2 + 6x – x – 6 = 0
x(x + 6) -1(x + 6) = 0
(x – 1) (x + 6) = 0
So now,
(x – 1) = 0 or (x + 6) = 0
x = 1 or x = -6
∴ Value of x =1, -6
Let’s check:
When x = 6, then
L.H.S = √(x + 15)
= √(-6 + 15)
= √9
= 3
R.H.S = x + 3
= -6 + 3
= -3
Thus, L.H.S ≠ R.H.S
So, x = -6 is not a root
And, when x = 1, then
L.H.S = √(x + 15)
= √(1 + 15)
= √16
= 4
R.H.S = x + 3
= 1 + 3
= 4
Thus, L.H.S = R.H.S
So, x = 1 is a root of this equation
Therefore, x = 1.
(ii) √(3x2 – 2x – 1) = 2x – 2
Let us simplify the given expression,
3x2 – 2x – 1 = (2x – 2)2 [Squaring on both sides]
3x2 – 2x – 1 = 4x2 + 4 – 8x
4x2 – 3x2 – 8x + 2x + 4 + 1 = 0
x2 – 6x + 5 = 0
Now, let us factorize
x2 – 5x – x + 5 = 0
x(x – 5) -1(x – 5) = 0
(x – 1) (x – 5) = 0
So now,
(x – 1) = 0 or (x – 5) = 0
x = 1 or x = 5
∴ Value of x =1, 5
Let’s check:
When x = 5, then
L.H.S = √(3x2 – 2x – 1)
= √(3(5)2 – 2(5) – 1)
= √(3 × 25 – 2 × 5 – 1)
= √64 = 8
R.H.S = 2x – 2
= 2(5) – 2
= 10 – 2 = 8
Thus, L.H.S = R.H.S
So, x = 5 is a root
And, when x = 1, then
L.H.S = √(3x2 – 2x – 1)
= √(3(1)2 – 2(1) – 1)
= √(3 × 1 – 2 × 1 – 1)
= √0 = 0
R.H.S = 2x – 2
= 2(1) – 2
= 0
Thus, L.H.S = R.H.S
So, x = 1 is a root
Therefore, x = 1, 5.
Solve the following equations (5 to 8) by using formula:
5. (i) 2x2 – 3x – 1 = 0 (ii) x(3x + ½) = 6
Solution:
(i) 2x2 – 3x – 1 = 0
Let us consider,
a = 2, b = -3, c = -1
So, by using the formula,

D = b2 – 4ac
= (-3)2 – 4(2)(-1)
= 9 + 8
= 17
So,
x = [-(-3) ± √17] / 2(2)
= [3 ± √17] / 4
= [3 + √17]/ 4 or [3 – √17]/4
∴ Value of x = (3 + √17)/4, (3 – √17)/4
(ii) x(3x + ½) = 6
Let us simplify the given expression,
3x2 + x/2 = 6
(6x2 + x)/2 = 6 [Taking L.C.M]
6x2 + x = 12
6x2 + x – 12 = 0
Let us consider,
a = 6, b = 1, c = -12
So, by using the formula,

D = b2 – 4ac
= (1)2 – 4(6)(-12)
= 1 + 288
= 289
So,
x = [-(1) ± √289] / 2(6)
= [-1 ± 17] / 12
= [-1 + 17]/ 12 or [-1 – 17]/12
= 16/12 or -18/12
= 4/3 or -3/2
∴ Value of x = 4/3, -3/2
6. (i) (2x + 5)/(3x + 4) = (x + 1)/(x + 3)
(ii) 2/(x + 2) – 1/(x + 1) = 4/(x + 4) – 3/(x + 3)
Solution:
(i) (2x + 5)/(3x + 4) = (x + 1)/(x + 3)
Let’s simply the given expression,
(2x + 5) (x + 3) = (x + 1) (3x + 4)
2x2 + 6x + 5x + 15 = 3x2 + 3x + 4x + 4
2x2 + 11x + 15 = 3x2 + 7x + 4
3x2 – 2x2 + 7x – 11x + 4 – 15 = 0
x2 – 4x – 11 = 0
Let us consider,
a = 1, b = -4, c = -11
So, by using the formula

D = b2 – 4ac
= (-4)2 – 4(1) (-11)
= 16 + 44
= 60
So,
x = [-(-4) ± √60] / 2(1)
= [4 ± 2√15] / 2
= [4 + 2√15]/ 2 or [4 – 2√15]/ 2
= 2(2 + √15)/2 or 2(2 – √15)/2
= (2 + √15) or (2 – √15)
∴ Value of x = (2 + √15), (2 – √15)
(ii) 2/(x + 2) – 1/(x + 1) = 4/(x + 4) – 3/(x + 3)

So, we have
x2 + 7x + 12 – x2 – 3x – 2 = 0
4x + 10 = 0
2x + 5 = 0
x = -5/2
But if x = 0, then

Which is actually true
Therefore, x = 0, -5/2.
7. (i) (3x – 4)/7 + 7/(3x – 4) = 5/2, x ≠ 4/3
(ii) 4/x – 3 = 5/(2x + 3), x ≠ 0, -3/2
Solution:
(i) (3x – 4)/7 + 7/(3x – 4) = 5/2
Taking L.C.M, we get
[(3x – 4)2 + 72]/ [7(3x – 4)] = 5/22[(3x – 4)2 + 72] = 5 × [7(3x – 4)]
2(9x2 + 16 – 24x + 49) = 35 (3x – 4)
2(9x2 – 24x + 65) = 35 (3x – 4)
18x2 – 48x + 130 = 105x – 140
18x2 – 48x – 105x + 130 + 140 = 0
18x2 – 153x + 270 = 0
2x2 – 17x + 30 = 0 [Dividing by 9]
Let us consider,
a = 2, b = -17, c = 30
So, by using the formula,

D = b2 – 4ac
= (-17)2 – 4(2) (30)
= 289 – 240
= 49
So,
x = [-(-17) ± √49] / 2(2)
= [17 ± 7] / 4
= [17 + 7]/ 4 or [17 – 7]/4
= 24/4 or 10/4
= 6 or 5/2
∴ Value of x = 6, 5/2
(ii) 4/x – 3 = 5/(2x + 3), x ≠ 0, -3/2
Let’s simplify the given equation,
(4 – 3x)/x = 5/(2x + 3) [Taking L.C.M]
(4 – 3x) (2x + 3) = 5x
8x + 12 – 6x2 – 9x = 5x
6x2 + 5x + x – 12 = 0
6x2 + 6x – 12 = 0
x2 + x – 2 = 0 [Dividing by 6]
Let us consider,
a = 1, b = 1, c = -2
So, by using the formula

D = b2 – 4ac
= (1)2 – 4(1) (-2)
= 1 + 8
= 9
So,
x = [-(1) ± √9] / 2(1)
= [-1 ± 3] / 2
= [-1 + 3]/ 2 or [-1 – 3]/ 2
= 2/2 or -4/2
= 1 or -2
∴ Value of x = 1, -2
8. (i) x2 + (4 – 3a)x – 12a = 0
(ii) 10ax2 – 6x + 15ax – 9 = 0, a ≠ 0
Solution:
(i) x2 + (4 – 3a)x – 12a = 0
Let us consider,
a = 1, b = (4 – 3a), c = -12a
So, by using the formula

D = b2 – 4ac
= (4 – 3a)2 – 4(1) (-12a)
= 16 + 9a2 – 24a + 48a
= 16 + 9a2 + 24a
= (4 + 3a)2
So,
x = [-(4 – 3a) ± √(4 + 3a)2] / 2(1)
= [-4 + 3a ± (4 + 3a)] / 2
= [- 4 + 3a + (4 + 3a)]/ 2 or [-4 + 3a – (4 + 3a)]/ 2
= 6a/ 2 or -8/ 2
= 3a or -4
∴ Value of x = 3a, -4
(ii) 10ax2 – 6x + 15ax – 9 = 0, a ≠ 0
10ax2 – (6 – 15a)x – 9 = 0
Let us consider,
a = 10, b = -(6 – 15a), c = -9
So, by using the formula

D = b2 – 4ac
= (6 – 15a)2 – 4(10a) (-9)
= 36 + 225a2 – 180a + 360a
= 36 + 225a2 + 180a
= (6 + 15a)2
So,
x = [-(-(6 – 15a)) ± √(6 + 15a)2] / 2(10a)
= [6 – 15a ± (6 + 15a)] / 20a
= [6 – 15a + (6 + 15a)]/ 20a or [6 – 15a – (6 + 15a)]/ 20a
= 12/20a or -30/20a
= 3/5a or -3/2
∴ Value of x = 3/5a, -3/2
9. Solve for x using the quadratic formula. Write your answer correct to two significant figures: (x – 1)2 – 3x + 4 = 0.
Solution:
Given the quadratic equation,
(x – 1)2 – 3x + 4 = 0
x2 – 2x – 3x + 1 + 4 = 0
x2 – 5x + 5 = 0
Let us consider,
a = 1, b = -5, c = 5
So, by using the formula

D = b2 – 4ac
= (-5)2 – 4(1) (5)
= 25 – 20
= 5
So,
x = [-(-5) ± √5] / 2(1)
= [5 ± √5] / 2
= [5 + √5]/ 2 or [5 – √5]/ 2
= (5 + 2.236) /2 or (5 – 2.236)/2
= 7.236 /2 or 2.764 /2
= 3.618 or 1.382
∴ Value of x = 3.618, 1.382
10. Discuss the nature of roots of the following equations:
(i) 3x2 – 7x + 8 = 0 (ii) x2 – ½ x – 4 = 0
(iii) 5x2 – 6√5x + 9 = 0 (iv) √3x2 – 2x – √3 = 0
In case the real roots exist, then find them.
Solution:
(i) 3x2 – 7x + 8 = 0
Let us consider,
a = 3, b = -7, c = 8
By using the formula,
D = b2 – 4ac
= (-7)2 – 4(3) (8)
= 49 – 96
= -47
So,
Discriminate, D = -47
D < 0
∴ Roots are not real.
(ii) x2 – ½ x – 4 = 0
Let us consider,
a = 1, b = -1/2, c = -4
By using the formula,
D = b2 – 4ac
= (-1/2)2 – 4(1) (-4)
= 1/4 + 16
= 65/16
So,
Discriminate, D = 65/16
D > 0
∴ Roots are real and distinct.
So,
x = [-(-1/2) ± √(65/16)] / 2(1)
= [1/2 ± √65/4] / 2
= [1/2 + √65/4]/ 2 or [1/2 – √65/4]/ 2
= (2 + √65)/4 /2 or (2 – √65)/4 /2
= (2 + √65)/ 8 or (2 – √65)/ 8
∴ Value of x = (2 + √65)/ 8, (2 – √65)/ 8
(iii) 5x2 – 6√5x + 9 = 0
Let us consider,
a = 5, b = -6√5, c = 9
By using the formula,
D = b2 – 4ac
= (-6√5)2 – 4(5) (9)
= 180 – 180
= 0
So,
Discriminate, D = 0
D = 0
∴ Roots are equal and real.
So,
x = [-(-6√5) ± √0] / 2(5)
= 6√5/ 10
= 3√5/ 5
∴ Value of x = 3√5/ 5
(iv) √3x2 – 2x – √3
Let us consider,
a = √3, b = -2, c = -√3
By using the formula,
D = b2 – 4ac
= (-2)2 – 4(√3) (-√3)
= 4 + 4(3)
= 4 + 12
= 16
So,
Discriminate, D = 16
D > 0
∴ Roots are real and distinct.
So,
x = [-(-2) ± √16] / 2(√3)
= [2 ± 4] / 2√3
= [2 + 4]/ 2√3 or [2 – 4]/ 2√3
= 6/(2√3) or -2/(2√3)
= 3/√3 or -1/√3
= √3 or -1/√3
∴ Value of x = √3, -1/√3
11. Find the values of k so that the quadratic equation
(4 – k)x2 + 2(k + 2)x + (8k + 1) = 0 has equal roots.
Solution:
Given the quadratic equation,
(4 – k)x2 + 2(k + 2)x + (8k + 1) = 0
Let us consider,
a = (4 – k), b = 2(k + 2), c = (8k + 1)
By using the formula,
D = b2 – 4ac
= [2(k + 2)]2 – 4(4 – k) (8k + 1)
= 4(k2 + 4k + 4) – 4(32k – 8k2 + 4 – k)
= 4k2 + 16k + 16 – 128k + 32k2 – 16 + 4k
= 36k2 – 108k
= 36k(k – 3)
So,
Discriminate, D = 36k(k – 3)
As the roots are equal
Hence, D = 0
36k(k – 3) = 0
So,
36k = 0 or k – 3 = 0
k = 0 or k = 3
Therefore, the value of x = 0, 3.
12. Find the values of m so that the quadratic equation 3x2 – 5x – 2m = 0 has two distinct real roots.
Solution:
Given the quadratic equation,
3x2 – 5x – 2m = 0
Let us consider,
a = 3, b = -5, c = -2m
By using the formula,
D = b2 – 4ac
= (-5)2 – 4(3) (-2m)
= 25 + 24m
So,
Discriminate, D = 25 + 24m
As the roots are real and distinct
Hence, D > 0
25 + 24m > 0
24m > -25
So,
m > -25/24
Therefore, the value of m must be greater than -25/24.
13. Find the value(s) of k for which each of the following quadratic equation has equal roots:
(i) 3kx2 = 4(kx – 1) (ii) (k + 4)x2 + (k + 1)x + 1 = 0
Also, find the roots for that value(s) of k in each case.
Solution:
For a quadratic equation to have equal roots, discriminant (D) = 0
(i) 3kx2 = 4(kx – 1)
Let’s rearrange the given equation,
3kx2 = 4kx – 4
3kx2 – 4kx + 4 = 0
Let us consider,
a = 3k, b = -4k, c = 4
By using the formula,
D = b2 – 4ac
= (-4k)2 – 4(3k) (4)
= 16k2 – 48k
Now,
16k2 – 48 = 0
16k(k – 3) = 0
So,
k = 0 or k – 3 = 0
Thus, k = 0 or 3
As D = 0, by the quadratic formula, we have
x = -b/2a
= 4k/(2 × 3k)
= 4k/6k
= 2/3
Hence, the roots are 2/3, 2/3.
(ii) (k + 4)x2 + (k + 1)x + 1 = 0
Let us consider,
a = k + 4, b = k + 1, c = 1
By using the formula,
D = b2 – 4ac
= (k + 1)2 – 4(k + 4) (1)
= k2 + 1 + 2k – 4k – 16
= k2 – 2k – 15
Now,
k2 – 2k – 15 = 0
k2 – 5k + 3k – 15 = 0
k(k – 5) + 3(k – 5) = 0
(k + 3) (k – 5) = 0
So,
k + 3 = 0 or k – 5 = 0
k = -3 or k = 5
Thus, k = -3, 5
As D = 0, by the quadratic formula, we have
x = -b/2a
= -(k + 1)/[2 × (k + 4)]
= (-k – 1)/ (2k + 8)
Now, when k = 5, we get
x = (-5 – 1)/ (2 × 5 + 8)
= -6/18
= -1/3
Hence, the roots are -1/3, -1/3
And, when k = -3, we get
x = (-(-3) – 1)/ (2 × (-3) + 8)
= (3 – 1)/(-6 + 8)
= 2/2 = 1
Hence, the roots are 1, 1.
14. Find two natural numbers which differ by 3 and whose squares have the sum 117.
Solution:
Let the first natural number be x
Then, the second natural number will be x + 3
According to the condition given in the problem,
x2 + (x + 3)2 = 117
x2 + x2 + 9 + 6x = 117
2x2 + 9 + 6x – 117 = 0
2x2 + 6x – 108 = 0
x2 + 3x – 54 = 0 [Dividing by 2]
x(x + 9) – 6(x + 9) = 0
(x – 6) (x + 9) = 0
So,
x – 6 = 0 or x + 9 = 0
x = 6 or x = -9
As x should be a natural number,
x = 6
Hence, first number = 6 and second number = 6 + 3 = 9.
15. Divide 16 into two parts such that the twice the square of the larger part exceeds the square of the smaller part by 164.
Solution:
Let the larger part be considered x
Then, the smaller part will be (16 – x)
According to the conditions given in the problem, we have
2x2 – (16 – x)2 = 164
2x2 – (256 – 32x + x2) = 164
2x2 – 256 + 32x – x2 – 164 = 0
x2 + 32x – 420 = 0
Now, by factorization method
x2 + 42x – 10x – 420 = 0
x(x + 42) – 10(x + 42) = 0
(x – 10) (x + 42) = 0
So,
x – 10 = 0 or x + 42 = 0
x = 10 or x = -42, which is not possible as it is negative
Thus, x = 10
Therefore, the larger part = 10 and the smaller part = 16 – 10 = 6.
16. Two natural numbers are in the ratio 3 : 4. Find the numbers if the difference between their squares is 175.
Solution:
Given, the ratio of two natural numbers is 3 : 4
Let the numbers be taken as 3x and 4x
Then, according to the conditions in the problem, we have
(4x)2 – (3x)2 = 175
16x2 – 9x2 = 175
7x2 = 175
x2 = 175/7 = 25
So,
x = √25 = ± 5
But, the value of x cannot be -5 as its not a natural number
Thus, x = 5
Therefore,
The natural numbers are 3(5) and 4(5) i.e. 15 and 20.
17. Two squares have sides x cm and (x + 4) cm. The sum of their areas is 656 sq. cm. Express this as an algebraic equation and solve it to find the sides of the squares.
Solution:
We have,
Side of first square = x cm
And the side of second square = (x + 4) cm
Now according to the given conditions in the problem, we have
x2 + (x + 4)2 = 656
x2 + x2 + 16 + 8x = 656
2x2 + 8x + 16 – 656 = 0
2x2 + 8x – 640 = 0
x2 + 4x – 320 = 0 [Dividing by 2]
By factorization method, we have
x2 + 20x – 16x – 320 = 0
x(x + 20) – 16(x + 20) = 0
(x + 20) (x – 16) = 0
So,
x + 20 = 0 or x – 16 = 0
x = -20 or x = 16
Since, side of a square cannot be negative.
Thus, x = 16
Therefore,
Side of first square = 16 cm
And, the side of the second square = (16 + 4) = 20 cm
18. The length of a rectangular garden is 12 m more than its breadth. The numerical value of its area is equal to 4 times the numerical value of its perimeter. Find the dimensions of the garden.
Solution:
Let’s assume the breadth of the rectangular garden as x m
Then, length = (x + 12) m
So,
Area = l × b m2
= x × (x + 12) m2
And, perimeter = 2(l + b)
= 2 (x + x + 12)
= 2 (2x + 12) m
Now according to the given condition in the problem, we have
x(x + 12) = 4 × 2(2x + 12)
x2 + 12x = 16x + 96
x2 – 4x – 96 = 0
x2 – 12x + 8x – 96 = 0
x(x – 12) + 8(x – 12) = 0
(x + 8)(x – 12) = 0
So,
x + 18 = 0 or x – 12 = 0
x = -18 or x = 12
But x = -18 is not possible as it negative
Thus, x = 12
Therefore,
Breadth = 12 m and length = 12 + 12 = 24 m.
19. A farmer wishes to grow a 100 m² rectangular vegetable garden. Since he has with him only 30 m barbed wire, he fences three sides of the rectangular garden letting compound wall of his house act as the fourth side fence. Find the dimensions of his garden.
Solution:
Given,
Area of rectangular garden = 100 cm2
Length of barbed wire = 30 m
Let’s assume the length of the side opposite to wall to be x
And the length of other side = (30 – x)/ 2
So, the area = (30 – x)/ 2 × x
= (30x – x2)/ 2
⇒ (30x – x2)/ 2 = 100
30x – x2 = 200
x2 – 30x + 200 = 0
By factorization method, we have
x2 – 20x – 10x + 200 = 0
x(x – 20) – 10(x – 20) = 0
(x – 20) (x – 10) = 0
So,
x – 20 or x – 10 = 0
x = 20 or x = 10
Hence,
(i) If x = 20, then side opposite to the wall = 20 m
And other side will be = (30 – 20)/2 = 10/2 = 5 m
(ii) If x = 10, then side opposite to the wall = 10 m
And other side will be = (30 – 10)/2 = 20/2 = 10 m
Therefore,
Sides of the rectangular can be 20 m, 5 m or 10 m, 10 m.
20. The hypotenuse of a right-angled triangle is 1 m less than twice the shortest side. If the third side is 1 m more than the shortest side, find the sides of the triangle.
Solution:
Let’s consider the length of the shortest side = x m
Length of hypotenuse = 2x – 1
And third side = x + 1
Now according to the given condition in the problem, we have
x2 + (x + 1)2 = (2x – 1)2 [By Pythagoras theorem]
x2 + x2 + 2x + 1 = 4x2 + 1 – 4x
4x2 – 2x2 – 4x – 2x – 1 + 1 = 0
2x2 – 6x = 0
x2 – 3x = 0 [Dividing by 2]
x(x – 3) = 0
So,
x = 0 or x – 3 = 0
x = 0 or x = 3
But, x = 0 is not possible
Hence, x = 3
So,
Shortest side = 3 m
Hypotenuse = 2 x 3 – 1 = 6 – 1 = 5 m
And, third side = x + 1 = 3 + 1 = 4 m
Therefore, the sides of the triangle are 3m, 5m and 4m.























































































Comments