ML Aggarwal Solutions For Class 9 Maths Chapter 17 Trigonometric Ratios consists of accurate solutions, which help the students to complete their homework quickly and prepare well for the exams. It ensures that you get all the necessary information about all concepts included in the chapter. Class 9 is a critical level as it forms the base for students for the forthcoming academic years. So, students who aspire to attain good marks in Maths should practice ML Aggarwal Solutions for Class 9 Maths. Chapter 17 of ML Aggarwal Solutions deals with trigonometric ratios. In trigonometry, trigonometric ratios are derived from the sides of a right-angled triangle. There are six 6 ratios such as sine, cosine, tangent, cotangent, cosecant, and secant. This chapter of ML Aggarwal Solutions for Class 9 Maths contains one exercise with a chapter test. These solutions provided by BYJU’S cover all these concepts, with detailed explanations.
ML Aggarwal Solutions for Class 9 Maths Chapter 17 – Trigonometric Ratios
Access answers to ML Aggarwal Solutions for Class 9 Maths Chapter 17 – Trigonometric Ratios
Exercise 17
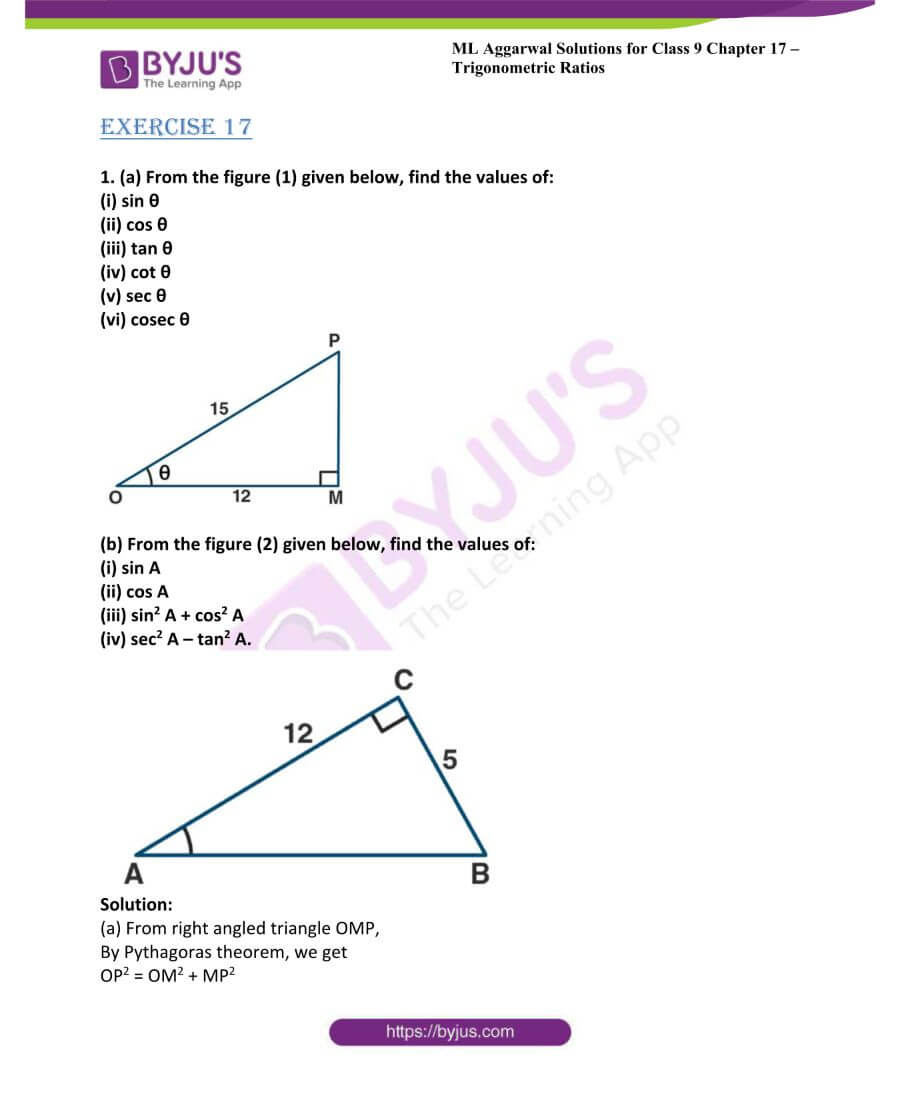
1. (a) From the figure (1) given below, find the values of:
(i) sin θ
(ii) cos θ
(iii) tan θ
(iv) cot θ
(v) sec θ
(vi) cosec θ

(b) From the figure (2) given below, find the values of:
(i) sin A
(ii) cos A
(iii) sin2 A + cos2 A
(iv) sec2 A – tan2 A.

Solution:
(a) From right-angled triangle OMP,
By Pythagoras theorem, we get
OP2 = OM2 +MP2
MP2 = OP2 + OM2
MP2 = (15)2 – (12)2
MP2 = 225 – 144
MP2 = 81
MP2 = 92
MP = 9
(i) sin θ = MP/OP
= 9/15
= 3/5
(ii) cos θ = OM/OP
= 12/15
= 4/5
(iii) tan θ = MP/OP
= 9/12
= ¾
(iv) cot θ = OM/MP
= 12/9
= 4/3
(v) sec θ = OP/OM
= 15/12
= 5/4
(vi) cosec θ = OP/MP
= 15/9
= /3
(b) From right-angled triangle ABC,
By Pythagoras theorem, we get
AB2 = AC2 + BC2
AB2 = (12)2 + (5)2
AB2 = 144 + 25
AB2 = 169
AB2 = 132
AB = 13
(i) sin A = BC/AB
= 5/13
(ii) cos A = AC/AB
= 12/13
(iii)Sin2 A + cos2 A = (BC/AB)2 + (AC/AB)2
= (5/13)2 + (12/13)2
= (25/169) + (144/169)
= (25 + 144)/ 169
= 169/169
= 1
Sin2 A + cos2 A = 1
(iv) Sec2 A – tan2 A = (AB/AC)2 – (BC/AC)2
= (13/12)2 – (5/12)2
= (169/144) – (25/144)
= (169 – 25)/ 144
= 144/144
= 1
Sec2 A – tan2 A = 1
2. (a) From the figure (1) given below, find the values of:
(i) sin B
(i) cos C
(iii) sin B + sin C
(iv) sin B cos C + sin C cos B.

(b) From the figure (2) given below, find the values of:
(i) tan x
(ii) cos y
(iii) cosec2 y – cot2 y
(iv) 5/sin x + 3/sin y – 3 cot y.

Solution:
From right-angled triangle ABC,
By Pythagoras theorem, we get
BC2 = AC2 + AB2
AC2 = BC2 – AB2
AC2 = (10)2 – (6)2
AC2 = 100 – 36
AC2 = 64
AC2 = 82
AC = 8
(i) sin B = perpendicular/ hypotenuse
= AC/BC
= 8/10
= 4/5
(ii) cos C = Base/hypotenuse
= AC/BC
= 8/10
= 4/5
(iii) sin B = Perpendicular/hypotenuse
= AC/BC
= 8/10
= 4/5
Sin C = perpendicular/hypotenuse
= AB/BC
= 6/10
= 3/5
Now,
Sin B + sin C = (4/5) + (3/5)
= (4 + 3)/5
= 7/5
(iv) sin B = 4/5
Cos C = 4/5
Sin C = perpendicular/ hypotenuse
= AB/BC
= 6/10
= 3/5
Cos B = Base/Hypotenuse
= AB/BC
= 6/10
= 3/5
sin B cos C + sin C cos B
= (4/5) × (4/5) × (3/5) × (3/5)
= (26/25) × (9/25)
= (16+9)/25
= 25/25
= 1
From Figure
AC = 13, CD = 5, BC =21,
BD = BC – CD
= 21 – 5
= 16

From right-angled ∆ACD,
By Pythagoras theorem we get
AC = AD2 + CD2
AD2 = AC2 – CD2
AD2 = (13)2 – (5)2
AD2 = 169 – 25
AD2 = 144)
AD2 = (12)2
AD = 12
From right-angled ∆ABD,
By Pythagoras angled ∆ABD
By Pythagoras theorem we get
AB2 = AD2 + BD2
AB2 = 400
AB2 = (20)2
AB = 20
(i) tan x = perpendicular/Base (in right-angled ∆ACD)
=CD/AD
= 5/12
(ii) cos y = Base/Hypotenuse (in right-angled ∆ABD)
= BD/AB
= (20)/12 – (5/3)
Cot y = Base/Perpendicular (in right ∆ABD)
=BD/AB
= 16/20 = 4/5
(iii) cos y = Hypotenuse/ perpendicular (in right-angled ∆ABD)
BD/AB
= 20/12
= 5/3
Cot y = Base/Perpendicular (in right ∆ABD)
AB/AD
= 16/12
= 4/3
Cosec2 y – cot2 y = (5/3)2 – (4/3)2
= (25/9) – (16/9)
= (25-16)/9
= 9/9
= 1
Hence, cosec2 y – cot2 y = 1
(iv) sin x = Perpendicular/Hypotenuse (in right-angled ∆ACD)
= AD/AB
= 12/20
= 3/5
Cot y = Base/Perpendicular (in right-angled ∆ABD)
= BD/AD
= 16/12
= 4/3
(5/sin x) + (3/sin y) – 3cot y
= 5/(5/13) + 3/(3/5) – 3 × 4/3
= 5 × 13/5 + 3 × 5/3 – 3 × 4/3
= 1 × 13/1 + 1 × 5/1 – 1 × 4/1
= 13 + 5 – 4 = 18 – 4
= 14
Hence 5/sin x + 3/sin y – 3cot y = 14
3. (a) From the figure (1) given below, find the value of sec θ.

(b) From the figure (2) given below, find the values of:
(i) sin x
(ii) cot x
(iii) cot2 x- cosec2 x
(iv) sec y
(v) tan2 y – 1/cos2 y.

Solution:
(a) From the figure, Sec θ = AB / BD
But in ∆ADC, ∠D = 90o
AC2 =AD2 + DC2 (Pythagoras Theorem)
(13)2 =AD2 + 25
AD2 = 169 -25
= 144
= (12)2
AD = 12
(in right ∆ABD)
AB2 = AD2 + BD2
= (12)2 + (16)2
= 144 + 256
= 400
= (20)2
AB = 20
Now, Sec θ = AB / BD
= 20/16
= 5/4
(b) let given ∆ABC
BD = 3, AC = 12, AD = 4
In right angled ∆ABD
By Pythagoras theorem
AB2 =AD2 + BD2
AB2 = (4)2 + (3)2
AB2 = 16 + 9
AB2 = 25
AB2 = (5)2
AB = 5
In right angled triangle ACD
By Pythagoras theorem,
AC2 = AD2 + CD2
CD2 = AC2 – AD2
CD2 = (12)2 – (4)2
CD2 = 144 – 16
CD2 = 128
CD = √128
CD = √64 × 2 CD
= 8√2
(i) sin x = perpendicular/Hypotenuse
= AD/AB
= 4/5
(ii) cot x = Base/Perpendicular
= BD/AD
= ¾
(iii) cot x = Base/ Perpendicular
BD/AD
= 3/4
(iv) cosec x = Hypotenuse / Perpendicular
AB/BD
= 5/4
Cot2 x – cosec2 x
= (3/4)2 – (5/4)2
= 9/16 – 25/16
(9 -25)/16
= -16/16
= -1
Perpendicular = Hypotenuse/Base (in right-angled ∆ACD)
= AD/CD
= 12/(8 √2)
= 3/(2 √2)
Cot y = Base/ Hypotenuse
= AD/CD
= 4/8 √ 2
= 1/2 √2
Cot y = Base / Hypotenuse (in right angled ∆ACD)
= CD/AC
= 8√2/12
= 2√/3
Now tan2 y = 1/cos2 y
= (1/2√2)2 – 1/(2√2/3)2
= ¼ × – ¼ × 2
= (1/8) – (9/8)
= (1-9)/8
= -8/8
= -1
tan2 y – 1/cos2 y = – 1.
4. (a) From the figure (1) given below, find the values of:
(i) 2 sin y – cos y
(ii) 2 sin x – cos x
(iii) 1 – sin x + cos y
(iv) 2 cos x – 3 sin y + 4 tan x
(b) In the figure (2) given below, ∆ABC is right-angled at B. If AB = y units, BC = 3 units and CA = 5 units, find
(i) sin x0
(ii) y.

Solution:
(a) In a right-angled ∆BCD,
Using Pythagoras theorem
BC2 = BD2 + CD2
Substituting the values
BC2 = 92 + 122
By further calculation
BC2 = 81 + 144 = 225
BC2 = 152
BC = 15
In a right-angled ∆ABC,
Using Pythagoras theorem
AC2 = AB2 + BC2
We can write it as
AB2 = AC2 – BC2
Substituting the values
AB2 = 252 – 152
By further calculation
AB2 = 625 – 225 = 400
So we get
AB2 = 202
AB = 20
(i) We know that
In right-angled ∆BCD
sin y = perpendicular/ hypotenuse
sin y = BD/ BC
Substituting the values
sin y = 9/15 = 3/5
In right-angled ∆BCD
cos y = base/hypotenuse
cos y = CD/BC
Substituting the values
cos y = 12/15 = 4/5
Here
2sin y – cos y = 2 × 3/5 – 4/5
We can write it as
= 6/5 – 4/5
= 2/5
Therefore, 2 sin y – cos y = 2/5
(ii) In right-angled ∆ABC
sin x = perpendicular/ hypotenuse
sin x = BC/AC
Substituting the values
sin x = 15/25 = 3/5
In right-angled ∆ABC
cos x = base/hypotenuse
cos x = AB/AC
Substituting the values
cos x = 20/25 = 4/5
Here
2 sin x – cos x = 2 × 3/5 – 4/5
We can write it as
= 6/5 – 4/5
= 2/5
Therefore, 2 sin x – cos x = 2/5.
(iii) In right-angled ∆ABC
sin x = perpendicular/hypotenuse
sin x = BC/AC
Substituting the values
sin x = 12/25 = 3/5
In right-angled ∆BCD
cos y = base/hypotenuse
cos y = CD/BC
Substituting the values
cos y = 12/15 = 4/5
Here
1 – sin x + cos y = 1 – 3/5 + 4/5
By further calculation
= (5 – 3 + 4)/ 5
So we get
= (9 – 3)/ 5
= 6/5
Therefore, 1 – sin x + cos y = 6/5.
(iv) In right-angled ∆BCD
cos x = base/hypotenuse
cos x = AB/AC
Substituting the values
cos x = 20/25 = 4/5
In right-angled ∆BCD
sin y = perpendicular/hypotenuse
sin y = BD/BC
Substituting the values
sin y = 9/15 = 3/5
In right-angled ∆ABC
tan x = perpendicular/base
tan x = BC/AB
Substituting the values
tan x = 15/20 = ¾
Here
2 cos x – 3 sin y + 4 tan x = 2 × 4/5 – 3 × 3/5 + 4 × ¾
By further calculation
= 8/5 – 9/5 3/1
Taking LCM
= (8 – 9 + 15)/5
So we get
= (23 – 9)/ 5
= 14/5
(b) It is given that
AB = y units, BC = 3 units, CA = 5 units
(i) In right-angled ∆ABC
sin x = perpendicular/hypotenuse
sin x = BC/AC
Substituting the values
sin x = 3/5
(ii) In right-angled ∆ABC
Using Pythagoras theorem
AC2 = BC2 + AB2
We can write it as
AB2 = AC2 – BC2
Substituting the values
AB2 = 52 – 32
By further calculation
AB2 = 25 – 9 = 16
So we get
AB2 = 42
AB = 4
y = 4 units
Therefore, y = 4 units.
5. In a right-angled triangle, it is given that angle A is an acute angle and that
tan A=5/12. Find the values of:
(i) cos A
(ii) cosec A- cot A.
Solution:
Here, ABC is right angled triangle
∠A is an acute angle and ∠C = 90o
tan A = 5/12
BC/AC =5/12
Let BC = 5x and AC = 12x
From right-angled ∆ABC
By Pythagoras theorem, we get
AB2 = (5x)2 + (12x)2
AB2 = 25x2 + 144x2
AB2 = 169x2
(i) cos A = Base/ Hypotenuse
= AC / AB
= 12x/13x
=12/13
(ii) cosec A = Hypotenuse/perpendicular
= AC / BC
= 13x /5x
= 13/5
cosec A – cot A = 13/5 – 12/5
= (13-12)/5
= 1/5
6. (a) In ∆ABC, ∠A = 900. If AB = 7 cm and BC – AC = 1 cm, find:
(i) sin C
(ii) tan B
(b) In ∆PQR, ∠Q = 900. If PQ = 40 cm and PR + QR = 50 cm, find:
(i) sin P
(ii) cos P
(iii) tan R.
Solution:
(a) In right ∆ABC
∠A = 900
AB = 7 cm
BC – AC = 1 cm
BC = 1 + AC

We know that
BC2 = AB2 + AC2
Substituting the value of BC
(1 + AC)2 = AB2 + AC2
1 + AC2 + 2AC = 72 + AC2
By further calculation
1 + AC2 + 2AC = 49 AC2
2AC = 49 – 1 – 48
So we get
AC = 48/2 = 24 cm
Here
BC = 1 + AC
Substituting the value
BC = 1 + 24 = 25 cm
(i) sin C = AB/BC = 7/25
(ii) tan B = AC/AB = 24/7
(b) In right ∆PQR
∠Q = 900
PQ = 40 cm
PQ + QR = 50 cm
We can write it as
PQ = 50 – QR

Using Pythagoras theorem
PR2 = PQ2 + QR2
(50 – QR)2 = (40)2 + QR2
By further calculation
2500 + QR2 – 100QR = 1600 + QR2
So we get
2500 – 1600 = 100QR
100QR = 900
By division
QR = 900/100 = 9
We get
PR = 50 – 9 = 41
(i) sin P = QR/PR = 9/41
(ii) cos P = PQ/PR = 40/41
(iii) tan R = PQ/QR = 40/9
7. In triangle ABC, AB = 15 cm, AC = 15 cm and BC = 18 cm. Find
(i) cos ∠ABC
(ii) sin ∠ACB.
Solution:
Here ABC is a triangle in which
AB = 15 cm, AC = 15 cm and BC = 18 cm
Draw AD perpendicular to BC , D is mid-point of BC.
Then, BD – DC = 9 cm
in right-angled triangle ABD,
By Pythagoras theorem, we get
AB2 = AD2 + BD2
AD2 = AB2 – BD2
AD2 = (15)2 – (9)2
AD2 = 225 – 81
AD2 = 144
AD – 12 cm
(i) cos ∠ABC = Base/ / Hypotenuse
(In right-angled ∆ABD, ∠ABC = ∠ABD)
= BD / AB
= 9/15
= 3/5
(ii) sin ∠ACB = sin ∠ACD
= perpendicular/ Hypotenuse
= AD/AC
= 12/15
= 4/5
8. (a) In the figure (1) given below, ∆ABC is isosceles with AB = AC = 5 cm and BC = 6 cm. Find
(i) sin C
(ii) tan B
(iii) tan C – cot B.
(b) In the figure (2) given below, ∆ABC is right-angled at B. Given that ∠ACB = θ, side AB = 2 units and side BC = 1 unit, find the value of sin2 θ + tan2 θ.
(c) In the figure (3) given below, AD is perpendicular to BC, BD = 15 cm, sin B = 4/5 and tan C = 1.
(i) Calculate the lengths of AD, AB, DC and AC.
(ii) Show that tan2 B – 1/cos2 B = – 1.

Solution:
(a) It is given that
∆ABC is isosceles with AB = AC = 5 cm and BC = 6 cm
Construct AD perpendicular to BC
D is the mid point of BC
So BD = CD
Here
BD = CD = 6/2 = 3 cm
In right-angled ∆ABD
Using Pythagoras theorem
AB2 = AD2 + BD2
We can write it as
AD2 = AB2 – BD2
Substituting the values
AD2 = 52 – 32
By further calculation
AD2 = 25 – 9 = 16
So we get
AD2 = 42
AD = 4 cm
(i) In right-angled ∆ACD
sin C = perpendicular/hypotenuse
sin C = AD/AC = 4/5
(ii) In right-angled ∆ABD
tan B = perpendicular/base
tan B = AD/BD = 4/3
(iii) In right-angled ∆ACD
tan C = perpendicular/base
tan C = AD/CD = 4/3
In right-angled ∆ABD
cot B = base/perpendicular
cot B = BD/AD = ¾
Here
tan C – cot B = 4/3 – ¾
Taking LCM
tan C – cot B = (16 – 9)/ 12 = 7/12
(b) It is given that
∆ABC is right-angled at B
AB = 2 units and BC = 1 unit
In right-angled ∆ABC
Using Pythagoras theorem
AC2 = AB2 + BC2
Substituting the values
AC2 = 22 + 12
AC2 = 4 + 1 = 5
So we get
AC2 = 5
AC = √5 units
In right-angled ∆ABC
sin θ = perpendicular/hypotenuse
sin θ = AB/AC = 2/√5
In right-angled ∆ABC
tan θ = perpendicular/base
tan θ = AB/BC = 2/1
We know that
sin2 θ + tan2 θ = (2/√5)2 + (2/1)2
By further calculation
= 4/5 + 4/1
Taking LCM
= (4 + 20)/ 5
= 24/5
= 4 4/5
(c) (i) In ∆ABC
AD is perpendicular to BC
BD = 15 cm
sin B = 4/5
tan C = 1
In ∆ABD
sin B = perpendicular/hypotenuse
sin B = AD/AB = 4/5
Consider AD = 4x and AB = 5x
Using Pythagoras theorem
In right-angled ∆ABD
AB2 = AD2 + BD2
We can write it as
BD2 = AB2 – AD2
Substituting the values
(15)2 = (5x)2 – (4x)2
225 = 25x2 – 16x2
By further calculation
225 = 9x2
x2 = 225/9 = 25
So we get
x = √25 = 5
Here
AD = 4 × 5 = 20
AB = 5 × 5 = 25
In right angled ∆ACD
tan C = perpendicular/base
So we get
tan C = AD/CD = 1/1
Consider AD = X then CD = x
In right-angled ∆ADC
Using Pythagoras theorem
AC2 = AD2 + CD2
Substituting the values
AC2 = x2 + x2 …..(1)
So the equation becomes
AC2 = 202 + 202
AC2 = 400 + 400 = 800
So we get
AC = √800 = 20√2
Length of AD = 20 cm
Length of AB = 25 cm
Length of DC = 20 cm
Length of AC = 20√2 cm
(ii) In right-angled ∆ABD
tan B = perpendicular/base
So we get
tan B = AD/BD
Substituting the values
tan B = 20/15 = 4/3
In right-angled ∆ABD
cos B = base/hypotenuse
So we get
cos B = BD/AB
Substituting the values
cos B = 15/25 = 3/5
Here
LHS = tan2 B – 1/cos2 B
Substituting the values
= (4/3)2 – 1/(3/5)2
By further calculation
= (4)2/(3)2 – (5)2/(3)2
= 16/9 – 25/9
So we get
= (16 – 25)/9
= -9/9
= – 1
= RHS
Hence, proved.
9. If sin θ =3/5 and θ is acute angle, find
(i) cos θ
(ii) tan θ.
Solution:
Let ∆ ABC be a right-angled at B
Let ∠ACB = θ
Given that, sin θ = 3/5
AB/AC = 3/5
Let AB = 3x
then AC = 5x
In right-angled ∆ ABC,
By Pythagoras theorem,
We get

(5x)2 = (3x)2 + BC2
BC2 = (5x)2 – (3x)2
BC2 = (2x)2
BC = 4x
(i) cos θ = Base/ Hypotenuse
= BC / AC
= 4x /5x
= 4/5
(ii) tan θ = perpendicular/Base
= AB/BC
= 3x/4x
= ¾
10. Given that tan θ = 5/12 and θ is an acute angle, find sin θ and cos θ.
Solution:
Consider ∆ ABC be right angled at B and ∠ACB = θ
It is given that
tan θ = 5/12
AB/BC = 5/12
Consider AB = 5x and BC = 12x

In right-angled ∆ ABC
Using Pythagoras theorem
AC2 = AB2 + BC2
Substituting the values
AC2 = (5x)2 + (12x)2
By further calculation
AC2 = 25x2 + 144x2 = 169x2
So we get
AC2 = (13x)2
AC = 13x
In right-angled ∆ ABC
sin θ = perpendicular/hypotenuse
So we get
sin θ = AB/AC = 5x/13x = 5/13
In right-angled ∆ ABC
cos θ = base/hypotenuse
So we get
cos θ = BC/AC
Substituting the values
cos θ = 12x/13x = 12/13
11. If sin θ = 6/10, find the value of cos θ + tan θ.
Solution:
Consider ∆ ABC be right-angled at B and ∠ACB = θ
It is given that
sin θ = AB/AC
sin θ = 6/10
Take AB = 6x then AC = 10x

In right-angled ∆ ABC
Using Pythagoras theorem
AC2 = AB2 + BC2
Substituting the values
(10x)2 = (6x)2 + BC2
By further calculation
BC2 = 100x2 – 36x2 = 64x2
So we get
BC2 = (8x)2
BC = 8x
In right-angled ∆ ABC
cos θ = base/hypotenuse
cos θ = BC/AC
Substituting the values
cos θ = 8x/10x = 4/5
In right-angled ∆ ABC
tan θ = perpendicular/base
tan θ = AB/BC
Substituting the values
tan θ = 6x/8x = ¾
Here
cos θ + tan θ = 4/5 + ¾
Taking LCM
= (16 + 15)/ 20
= 31/20
= 1 11/20
12. If tan = 4/3, find the value of sin θ + cos θ (both sin θ and cos θ are positive).
Solution:
Let ∆ABC be a right-angled
∠ACB = θ
Given that, tan θ = 4/3
(AB/BC = 4/3)
Give that, tan θ = 4/3
(AB/BC = 4/3)
Let AB = 4x,
then BC = 3x

In right-angled ∆ABC
By Pythagoras theorem, we get
AC2 = AB2 + BC2
AC2 = AB2 + BC2
AC2 = AB2 + BC2
AC2 = AB2 + BC2
(AC2 = (4x)2 + (3x)2
AC2 = 16x2 + 9x2
AC2 = 25x2
AC2 = (5x)2
AC = 5x
Sin θ = perpendicular/Hypotenuse
= AB/AC
= 4x/5x
= 4/5
Cos θ = Base/Hypotenuse
= BC/AC
= 3x/5x
= 3/5
Sin θ + cos θ
= 4/5 + 3/5
= (4 + 3)/5
= 7/5
Hence, Sin θ + cos θ = 7/5 = 1 2/5
13. 1f cosec = √5 and θ is less than 900, find the value of cot θ – cos θ.
Solution:
Given cosec θ = √5/1 = OP/PM
OP = √5 and PM = 1

Now OP2 = OM2 + PM2 using Pythagoras theorem
(√5)2 = OM2 + 12
5 = OM2 + 1
OM2 = 5 – 1
OM2 = 4
OM = 2
Now cot θ = OM/PM
= 2/1
= 2
Cos θ = OM/OP
= 2/√5
Now cot θ – Cos θ = 2 – (2/√5)
= 2 (√5 – 1)/ √5
14. Given sin θ = p/q, find cos θ + sin θ in terms of p and q.
Solution:
Given that sin θ = p/q

Which implies,
AB/AC = p/q
Let AB = px
And then AC = qx
In right angled triangle ABC
By Pythagoras theorem,
We get
AC2 = AB2 + BC2
BC2 = AC2 – AB2
BC2 = q2x2 – p2x2
BC2 = (q2 – p2)x2
BC = √( q2 – p2)x
In right angled triangle ABC,
Cos θ = base/ hypotenuse
= BC/AC
= √( q2 – p2)x/qx
= √( q2 – p2)/ q
Now,
Sin θ + cos θ = p/q + √( q2 – p2)/ q
= [p + √( q2 – p2)]/ q
15. If θ is an acute angle and tan = 8/15, find the value of sec θ + cosec θ.
Solution:
Given tan θ = 8/15
θ is an acute angle
in the figure triangle OMP is a right-angled triangle,
∠M = 90o and ∠Q = θ

Tanθ = PM/OL = 8/15
Therefore, PM = 8, OM = 15
But OP2 = OM2 + PM2 using Pythagoras theorem,
= 152 + 82
= 225 + 64
= 289
= 172
Therefore, OP = 17
Sec θ = OP/OM
= 17/15
Cosec θ = OP/PM
= 17/8
Now,
Sec θ + cosec θ = (17/15) + (17/8)
= (136 + 255)/ 120
= 391/120
= 3 31/120
16. Given A is an acute angle and 13 sin A = 5, evaluate:
(5 sin A – 2 cos A)/ tan A.
Solution:
Let triangle ABC be a right-angled triangle at B and A is an acute angle
Given that 13 sin A = 5
Sin A = 5/13
AB/Ac = 5/13

Let AB = 5x
AC = 13 x
In right angled triangle ABC,
Using Pythagoras theorem,
We get
AC2 = AB2 + BC2
BC2 = AC2 – BC2
BC2 = (13x)2 – (5x)2
BC2 = 169x2 – 25x2
BC2 = 144x2
BC = 12x
Sin A = 5/13
Cos A = base/ hypotenuse
= BC/AC
= 12x/ 13x
= 12/13
Tan A = perpendicular/ base
= AB/BC
= 5x/ 12x
= 5/ 12
Now,
(5 sin A – 2 cos A)/ tan A = [(5) (5/13) – (2) (12/13)]/ (5/12)
= (1/13)/ (5/12)
= 12/65
Hence (5 sin A – 2 cos A)/ tan A = 12/65
17. Given A is an acute angle and cosec A = √2, find the value of
(2 sin2 A + 3 cot2 A)/ (tan2 A – cos2 A).
Solution:
Let triangle ABC be a right-angled at B and A is a acute angle.
Given that cosec A = √2

Which implies,
AC/BC = √2/1
Let AC = √2x
Then BC = x
In right angled triangle ABC
By using Pythagoras theorem,
We get
AC2 = AB2 + BC2
(√2x)2 = AB2 + x2
AB2 = 2x2 – x2
AB = x
Sin A = perpendicular/ hypotenuse
= BC/AC
= 1/ √2
Cot A = base/ perpendicular
= x/x
= 1
Tan A = perpendicular/ base
= BC/AB
= x/x
= 1
Cos A = base/ hypotenuse
= AB/AC
= x/ √2x
= 1/√2
Substituting these values we get
2 sin2 A + 3 cot2 A/ (tan2 A – cos2 A) = 8
18. The diagonals AC and BD of a rhombus ABCD meet at O. If AC = 8 cm and BD = 6 cm, find sin ∠OCD.
Solution:
It is given that
Diagonals AC and BD of rhombus ABCD meet at O
AC = 8 cm and BD = 6 cm
O is the mid point of AC

We know that
AO = OC = AC/2 = 8/2 = 4 cm
O is the mid point of BD
BO = OD = BD/2 = 6/2 = 3 cm
In right angled ∆COD
CD2 = OC2 + OD2
Substituting the values
CD2 = 42 + 32
So we get
CD2 = 16 + 9 = 25
CD2 = 52
CD = 5 cm
In right angled ∆COD
sin ∠OCD = perpendicular/ hypotenuse
So we get
sin ∠OCD = OD/CD = 3/5
19. If tan θ = 5/12, find the value of (cos θ + sin θ)/ (cos θ – sin θ).
Solution:
Consider ∆ABC be right-angled at B and ∠ACB = θ
It is given that
tan θ = AB/BC = 5/12
Take AB = 5x then BC = 12x
In right-angled ∆ABC,
Using Pythagoras theorem
AC2 = AB2 + BC2
Substituting the values
AC2 = (5x)2 + (12x)2
By further calculation
AC2 = 25x2 + 144x2 = 169x2
So we get
AC2 = (13x)2
AC = 13x
In right-angled ∆ABC
cos θ = base/hypotenuse
cos θ = BC/AC
Substituting the values
cos θ = 12x/13x = 12/13
In right-angled ∆ABC
sin θ = perpendicular/hypotenuse
sin θ = AB/AC
Substituting the values
sin θ = 5x/13x = 5/13
Here
(cos θ + sin θ)/ (cos θ – sin θ) = [12/13 + 5/13]/ [12/13 – 5/13]
Taking LCM
= [(12 + 5)/ 13]/[(12 – 5)/ 13]
So we get
= 17/13/ 7/13
= 17/13 × 13/7
= 17/7
Therefore, (cos θ + sin θ)/ (cos θ – sin θ) = 17/7 = 2 3/7.
20. Given 5 cos A – 12 sin A = 0, find the value of (sin A + cos A)/ (2 cos A – sin A).
Solution:
It is given that
5 cos A – 12 sin A = 0
We can write it as
5 cos A = 12 sin A
So we get
sin A/ cos A = 5/12
We know that sin A/ cos A = tan A
tan A = 5/12

Consider ∆ABC right angled at B and ∠A is acute angle
Here
tan A = BC/AB = 5/12
Take BC = 5x then AB = 12x
In right-angled ∆ABC
Using Pythagoras theorem
AC2 = BC2 + AB2
Substituting the values
AC2 = (5x)2 + (12x)2
AC2 = 25x2 + 144x2 = 169x2
So we get
AC2 = (13x)2
AC = 13x
In right-angled ∆ABC
sin A = perpendicular/hypotenuse
So we get
sin A = BC/AC = 5x/13x = 5/13
In right-angled ∆ABC
cos A = base/hypotenuse
So we get
cos A = AB/AC = 12x/13x = 12/13
Here
(sin A + cos A)/ (2 cos A – sin A) = [5/13 + 12/13]/ [2 × 12/13 – 5/13]
By further calculation
= [(5 + 12)/13]/ [24/13 – 5/13]
So we get
= [(5 + 12)/13]/[(24 – 5)/13]
= 17/13/ 19/13
= 17/13 × 13/19
= 17/19
Therefore, (sin A + cos A)/ (2 cos A – sin A) = 17/19
21. If tan θ = p/q, find the value of (p sin θ – q cos θ)/ (p sin θ + q cos θ).
Solution:
It is given that
tan θ = p/q
Consider ∆ABC be right angled at B and ∠BCA = θ
tan θ = BC/AB = p/q
BC = px then AB = qx

In right angled ∆ABC
Using Pythagoras theorem
AC2 = BC2 + AB2
Substituting the values
AC2 = (px)2 + (qx)2
AC2 = p2x2 + q2x2
AC2 = x2 (p2 + q2)
So we get
AC = √x2 (p2 + q2)
AC = x(√p2 + q2)
In right-angled ∆ABC
sin θ = perpendicular/hypotenuse
sin θ = BC/AC
Substituting the values
sin θ = px/ x(√p2 + q2)
So we get
sin θ = p/ (√p2 + q2)
In right-angled ∆ABC
cos θ = base/hypotenuse
cos θ = AB/AC
Substituting the values
cos θ = qx/ x(√p2 + q2)
So we get
cos θ = q/ (√p2 + q2)
Here

22. If 3 cot θ = 4, find the value of (5 sin θ – 3 cos θ)/ (5 sin θ + 3 cos θ).
Solution:
It is given that
3 cot θ = 4
cot θ = 4/3

Consider ∆ABC to be right-angled at B and ∠ACB = θ
cot θ = BC/AB = 4/3
Take BC = 4x then AB = 3x
In right-angled ∆ABC
Using Pythagoras theorem
AC2 = AB2 + BC2
Substituting the values
AC2 = (3x)2 + (4x)2
AC2 = 9x2 + 16x2 = 25x2
So we get
AC2 = (5x)2
AC = 5x
In right angled ∆ABC
sin θ = perpendicular/hypotenuse
sin θ = AB/AC
Substituting the values
sin θ = 3x/5x = 3/5
In right-angled ∆ABC
cos θ = base/hypotenuse
cos θ = BC/AC
Substituting the values
cos θ = 4x/5x = 4/5


23. (i) If 5 cos θ – 12 sin θ = 0, find the value of (sin θ + cos θ)/(2 cos θ – sin θ).
(ii) If cosec θ = 13/12, find the value of (2 sin θ – 3 cos θ)/(4 sin θ – 9 cos θ).
Solution:
(i) It is given that
5 cos θ – 12 sin θ = 0
We can write it as
5 cos θ = 12 sin θ
sin θ/ cos θ = 5/12
tan θ = 5/12
Dividing both numerator and denominator by cos θ

(ii) It is given that
cosec θ = 13/12
We know that cosec θ = 1/ sin θ
1/sin θ = 13/12
sin θ = 12/13
Here cos2 θ = 1 – sin2 θ
Substituting the values
= 1 – (12/13)2
By further calculation
= 1 – 144/169
Taking LCM
= (169 – 144)/ 169
= 25/169
So we get
= (5/13)2
cos θ = 5/13
Here

24. If 5 sin θ = 3, find the value of (sec θ – tan θ)/ (sec θ + tan θ).
Solution:

Consider ∆ABC be right-angled at B and ∠ACB = θ
It is given that
5 sin θ = 3
sin θ = AB/AC = 3/5
Take AB = 3x then AC = 5x
In right-angled ∆ABC
Using Pythagoras theorem
AC2 = AB2 + BC2
BC2 = AC2 – AB2
Substituting the values
BC2 = (5x)2 – (3x)2
So we get
BC2 = 25x2 – 9x2 = 16x2
BC2 = (4x)2
BC = 4x
In right-angled ∆ABC
sec θ = hypotenuse/base
sec θ = AC/BC = 5x/4x = 5/4
In right-angled ∆ABC
tan θ = perpendicular/base
tan θ = AB/BC = 3x/4x = ¾


25. If θ is an acute angle and sin θ = cos θ, find the value of 2 tan2 θ + sin2 θ – 1.
Solution:

Consider ∆ABC be right angled at B and ∠ACB = θ
It is given that
sin θ = cos θ
sin θ/cos θ = 1
tan θ = AB/BC = 1
Take AB = x then BC = x
In right-angled ∆ABC
Using Pythagoras theorem
AC2 = AB2 + BC2
AC2 = x2 + x2 = 2x2
So we get
AC = √2x2
AC = (√2)x
In right-angled ∆ABC
sin θ = perpendicular/hypotenuse
sin θ = AB/AC = x/√2x = 1/√2
Here
2 tan2 θ + sin2 θ – 1 = 2 × (1)2 + (1/√2)2 – 1
By further calculation
= 2 × 1 + ½ – 1
= 2 + ½ – 1
= 1+ ½
Taking LCM
= (2 + 1)/2
= 3/2
Therefore, 2 tan2 θ + sin2 θ – 1 = 3/2.
26. Prove the following:
(i) cos θ tan θ = sin θ
(ii) sin θ cot θ = cos θ
(iii) sin2 θ/ cos θ + cos θ = 1/ cos θ.
Solution:
(i) cos θ tan θ = sin θ
LHS = cos θ tan θ
We know that tan θ = sin θ/cos θ
= cos θ (sin θ/cos θ)
So we get
= 1 × sin θ/1
= sin θ
= RHS
Therefore, LHS = RHS.
(ii) sin θ cot θ = cos θ
LHS = sin θ cot θ
We know that cot θ = cos θ/sin θ
= sin θ (cos θ/sin θ)
= 1 × cos θ/1
= cos θ
= RHS
Therefore, LHS = RHS.
(iii) sin2 θ/cos θ + cos θ = 1/cos θ
LHS = sin2 θ/cos θ + cos θ/1
Taking LCM
= (sin2 θ + cos2 θ)/cos θ
We know that sin2 θ + cos2 θ = 1
= 1/cos θ
= RHS
Therefore, LHS = RHS.
27. If in ∆ABC, ∠C = 900 and tan A = ¾, prove that sin A cos B + cos A sin B = 1.
Solution:
It is given that
tan A = BC/AC = ¾

Using Pythagoras theorem
AB2 = AC2 + BC2
Substituting the values
= 42 + 32
= 16 + 9
= 25
= 52
So we get AB = 5
Here
sin A = BC/AC = 3/5
cos A = AC/AB = 4/5
cos B = BC/AB = 3/5
sin B = AC/AB = 4/5
LHS = sin A cos B + cos A sin B
Substituting the values
= 3/5 × 3/5 + 4/5 × 4/5
By further calculation
= 9/25+ 16/25
= (9 + 16)/ 25
= 25/25
= 1
= RHS
Therefore, LHS = RHS.
28. (a) In figure (1) given below, ∆ABC is right-angled at B and ∆BRS is right-angled at R. If AB = 18 cm, BC = 7.5 cm, RS = 5 cm, ∠BSR = x0 and ∠SAB = y0, then find:
(i) tan x0
(ii) sin y0.
(b) In the figure (2) given below, ∆ABC is right angled at B and BD is perpendicular to AC. Find
(i) cos ∠CBD
(ii) cot ∠ABD.

Solution:
(a) ∆ABC is right-angled at B, ∆BSC is right-angled at S and ∆BRS is right-angled at R
It is given that
AB = 18 cm, BC = 7.5 cm, RS = 5 cm, ∠BSR = x0 and ∠SAB = y0
By Geometry ∆ARS and ∆ABC are similar
AR/AB = RS/BC
Substituting the values
AR/18 = 5/7.5
By further calculation
AR = (5 × 18)/7.5 = (1 × 18)/1.5
Multiply both the numerator and denominator by 10
AR = (18 × 10)/15
AR = (10 × 6)/5
AR = (2 × 6)/1 = 12
So we get
RB = AB – AR
RB = 18 – 12 = 6
In right-angled ∆ABC
Using Pythagoras theorem
AC2 = AB2 + BC2
Substituting the values
AC2 = 182 + 7.52
By further calculation
AC2 = 324 + 56.25 = 380.25
AC = √380.25 = 19.5 cm
(i) In right-angled ∆BSR
tan x0 = perpendicular/base
tan x0 = RB/RS = 6/5
(ii) In right-angled ∆ASR
sin y0 = perpendicular/hypotenuse
Using Pythagoras theorem
AS2 = 122 + 52
By further calculation
AS2 = 144 + 25 = 169
AS = √169 = 13 cm
So we get
sin y0 = RS/AS = 5/13
(b) We know that
∆ABC is right-angled at B and BD is perpendicular to AC
In right-angled ∆ABC
Using Pythagoras theorem
AC2 = AB2 + BC2
Substituting the values
AC2 = 122 + 52
By further calculation
AC2 = 144 + 25 = 169
So we get
AC2 = (13)2
AC = 13
By Geometry ∠CBD = ∠A and ∠ABD = ∠C
(i) cos ∠CBD = cos ∠A = base/hypotenuse
In right-angled ∆ABC
cos ∠CBD = cos ∠A = AB/AC = 12/13
(ii) cos ∠ABD = cos ∠C = base/perpendicular
In right-angled ∆ABC
cos ∠ABD = cos ∠C = BC/AB = 5/12
29. In the adjoining figure, ABCD is a rectangle. Its diagonal AC = 15 cm and ∠ACD = α. If cot α = 3/2, find the perimeter and the area of the rectangle.

Solution:
In right ∆ADC
cot α = CD/AD = 3/2
Take CD = 3x then AD = 2x
Using Pythagoras theorem
AC2 = CD2 + AD2
Substituting the values
(15)2 = (3x)2 + (2x)2
By further calculation
13x2 = 225
x2 = 225/13
So we get
x = √225/13 = 15/√13
Length of rectangle (l) = 3x = (3 × 15)/ √13 = 45/√13 cm
Breadth of rectangle (b) = 2x = (2 × 15)/ √13 = 30/√13 cm
(i) Perimeter of rectangle = 2 (l + b)
Substituting the values of l and b
= 2 (45/√13 + 30/√13)
So we get
= 2 × 75/√13
= 150/√13 cm
(ii) Area of rectangle = l × b
Substituting the values of l and b
= 45/√13 × 30/√13
So we get
= 1350/13
= 103 11/13 cm2
30. Using the measurements given in the figure alongside,
(a) Find the values of:
(i) sin ϕ
(ii) tan θ.
(b) Write an expression for AD in terms of θ.

Solution:
From the figure
BC = 12, BD = 13
In right-angled ∆BCD
Using Pythagoras theorem
BD2 = BC2 + CD2
It can be written as
CD2 = BD2 – BC2
Substituting the values
CD2 = (13)2 – (12)2
CD2 = 169 – 144 = 25
So we get
CD = √25 = 5

Construct BE perpendicular to AB
CD = BE = 5 and EA = AE = 14 – 5 = 9
(a) (i) sin ϕ = perpendicular/hypotenuse
In right-angled ∆BCD
sin ϕ = CD/BD = 5/13
(ii) tan θ = perpendicular/hypotenuse
In right-angled ∆AED
tan θ = ED/AE = BC/AE = 12/9 = 4/3 (Since ED = BC)
(b) In right-angled ∆AED
sin θ = perpendicular/hypotenuse
cos θ = base/perpendicular
We can write it as
sin θ = ED/AD or cos θ = AE/AD
AD = ED/sin θ or AD = AE/ cos θ
Substituting the values
AD = 12/sin θ or AD = 9/cos θ
Therefore, AD = 12/ sin θ or AD = 9/cos θ.
31. Prove the following:
(i) (sin A + cos A)2 + (sin A – cos A)2 = 2
(ii) cot2 A – 1/sin2 A + 1 = 0
(iii) 1/(1 + tan2 A) + 1/(1 + cot2 A) = 1
Solution:
(i) (sin A + cos A)2 + (sin A – cos A)2 = 2
LHS = (sin A + cos A)2 + (sin A – cos A)2
Using the formula
(a + b)2 = a2 + b2 + 2ab and (a – b)2 = a2 + b2 – 2ab
= [(sin A)2 + (cos A)2 + 2 sin A cos A] + [(sin A)2 + (cos A)2 – 2 sin A cos A]
By further calculation
= sin2 A + cos2 A + 2 sin A cos A + sin2 A + cos2 A – 2 sin A cos A
= sin2 A + cos2 A + sin2 A + cos2 A
= 2 sin2 A + 2 cos2 A
We know that sin2 A + cos2 A = 1
= 2 (sin2 A + cos2 A)
= 2 (1)
= 2
= RHS
Therefore, LHS = RHS.
(ii) cot2 A – 1/sin2 A + 1 = 0
LHS = cot2 A – 1/sin2 A + 1
We know that
1/sin A = cosec A
= cot2 A – cosec2 A + 1
= (1 + cot2 A) – cosec2 A
We know that 1 + cot2 A = cosec2 A
= cosec2 A – cosec2 A
= 0
= RHS
Therefore, LHS = RHS.
(iii) 1/(1 + tan2 A) + 1/(1 + cot2 A) = 1
LHS = 1/(1 + tan2 A) + 1/(1 + cot2 A)
We know that
sec2 A – tan2 A = 1
sec2 A = 1 + tan2 A
cosec2 A – cot2 A = 1
cosec2 A = 1 + cot2 A
So we get
= 1/sec2 A + 1/cosec2 A
Here 1/sec A = cos A and 1/cosec A = sin A
= cos2 A + sin2 A
= 1
= RHS
Therefore, LHS = RHS.
32. Simplify

Solution:

We know that 1 = sin2 θ + cos2 θ
= √cos2 θ/sin2 θ
= cos θ/ sin θ
Here cos θ/sin θ = cot θ
= cot θ
Therefore,
 = cot θ.
= cot θ.
33. If sin θ + cosec θ = 2, find the value of sin2 θ + cosec2 θ.
Solution:
It is given that
sin θ + cosec θ = 2
sin θ + 1/sin θ = 2
By further calculation
sin2 θ + 1 = 2 sin θ
sin2 θ – 2 sin θ + 1 = 0
So we get
(sin θ – 1)2 = 0
sin θ – 1 = 0
sin θ = 1
Here
sin2 θ + cosec2 θ = sin2 θ + 1/sin2 θ
Substituting the values
= 12 + 1/12
= 1 + 1/1
= 1 + 1
= 2
34. If x = a cos θ + b sin θ and y = a sin θ – b cos θ, prove that x2 + y2 = a2 + b2.
Solution:
It is given that
x = a cos θ + b sin θ …. (1)
y = a sin θ – b cos θ …. (2)
By squaring and adding both the equations
x2 + y2 = (a cos θ + b sin θ)2 + (a sin θ – b cos θ)2
Using the formula
(a + b)2 = a2 + b2 + 2ab and (a – b)2 = a2 + b2 – 2ab
= [(a cos θ)2 + (b sin θ)2 + 2 (a cos θ) (b sin θ)] + [(a sin θ)2 + (b cos θ)2 – 2 (a sin θ) (b cos θ)]
By further calculation
= a2 cos2 θ + b2 sin2 θ + 2 ab sin θ cos θ + a2 sin2 θ + b2 cos2 θ – 2 ab sin θ cos θ
= a2 cos2 θ + b2 sin2 θ + a2 sin2 θ + b2 cos2 θ
So we get
= a2 (cos2 θ + sin2 θ) + b2 (sin2 θ + cos2 θ)
Here sin2 θ + cos2 θ = 1
= a2 (1) + b2 (1)
= a2 + b2
Therefore, x2 + y2 = a2 + b2.
Chapter test
1. (a)From the figure (i) given below, calculate all the six t-ratios for both acute………
(b)From the figure (ii) given below, find the values of x and y in terms of t-ratios


Solution:
(a) From right-angled triangle ABC,
By Pythagoras theorem, we get
AC2 = AB2 + BC2
AB2 = AC2 – BC2
AB2 = (3)2 – (2)2
AB2 = 9 – 4
AB2 = 5
AB = √5
(i) sin A = perpendicular/ hypotenuse
= BC/AC
= 2/3
(ii) cos A = base/ hypotenuse
= AB/AC
= √5/3
(iii) tan A = perpendicular/ base
= BC/AB
= 2/ √5
(iv) cot A = base/perpendicular
= AB/ BC
= √5/2
(v) sec A = hypotenuse/ base
= AC/AB
= 3/ √5
(vi) cosec A = hypotenuse/perpendicular
= AC/BC
= 3/2
(b) From right angled triangle ABC,
∠BAC = θ
Then we know that,
Cot θ = base/ perpendicular
= AB/ BC
= x/ 10
x = 10 cot θ
also, cosec θ = hypotenuse/ perpendicular
= AC/ BC
= y/ 10
y = 10 cosec θ
Therefore, x = 10 cot θ and y = 10 cosec θ.
2. (a) From the figure (1) given below, find the values of:
(i) sin ∠ABC
(ii) tan x – cos x + 3 sin x.
(b) From the figure (2) given below, find the values of:
(i) 5 sin x
(ii) 7 tan x
(iii) 5 cos x – 17 sin y – tan x.

Solution:
(a) From the figure
BC = 12, CD = 9 and BC = 20
In right-angled ∆ABC,
Using Pythagoras theorem
AB2 = AC2 + BC2
It can be written as
AC2 = AB2 – BC2
Substituting the values
AC2 = (20)2 – (12)2
By further calculation
AC2 = 400 – 144 = 256
So we get
AC2 = (16)2
AC = 16
In right-angled ∆BCD
Using Pythagoras theorem
BD2 = BC2 + CD2
Substituting the values
BD2 = 122 + 92
By further calculation
BD2 = 144 + 81 = 225
So we get
BD2 = (15)2
BD = 15
(i) In right-angled ∆BCD
sin ∠ABC = perpendicular/hypotenuse
So we get
sin ∠ABC = AC/AB = 16/20 = 4/5
(ii) In right-angled ∆BCD
tan x = perpendicular/base
So we get
tan x = BC/CD = 12/9 = 4/3
In right-angled ∆BCD
cos x = base/hypotenuse
So we get
cos x = CD/BD = 9/15 = 3/5
In right-angled ∆BCD
sin x = perpendicular/hypotenuse
So we get
sin x = BC/BD = 12/15 = 4/5
tan x – cos x + 3 sin x = 4/3 – 3/5 + 3 × 4/5
By further calculation
= 4/3 – 3/5 + 12/5
Taking LCM
= (4 × 5 – 3 × 3 + 12 × 3)/ 15
So we get
= (20 – 9 + 36)/ 15
= (56 – 9)/ 15
= 27/15
= 3 2/15
Therefore, tan x – cos x + 3 sin x = 3 2/15.
(b) In the figure
AC = 17, AB = 25, AD = 15
In right-angled ∆ACD
Using Pythagoras theorem
AC2 = AD2 + CD2
Substituting the values
(17)2 = (15)2 + (CD)2
By further calculation
CD2 = (17)2 – (15)2
CD2 = 289 – 225 = 64
So we get
CD2 = 82
CD = 8
In right-angled ∆ABD
Using Pythagoras theorem
AB2 = AD2 + BD2
Substituting the values
(25)2 = (15)2 + BD2
By further calculation
BD2 = (25)2 – (15)2
BD2 = 625 – 225 = 400
So we get
BD2 = (20)2
BD = 20
(i) In right-angled ∆ABD
5 sin x = 5 (perpendicular/hypotenuse)
So we get
= 5 (AD/AB)
= 5 × 15/25
= 15/5
= 3
(ii) In right angled ∆ABD
7 tan x = 7 (perpendicular/base)
So we get
= 7 (AD/AB)
= 7 × 15/20
= 7 × ¾
= 21/4
= 5 ¼
(iii) In right-angled ∆ABD
cos x = base/hypotenuse
So we get
cos x = BD/AB = 20/25 = 4/5
In right-angled ∆ACD
sin y = perpendicular/hypotenuse
So we get
sin y = CD/AC = 8/17
In right-angled ∆ABD
tan x = perpendicular/base
So we get
tan x = AD/BD = 15/20 = ¾
5 cos x – 17 sin y – tan x = 5 × 4/5 – 17 × 8/17 – ¾
It can be written as
= 4/1 – 8/1 – ¾
Taking LCM
= (16 – 32 – 3)/4
= (16 – 35)/4
So we get
= – 19/4
= – 4 ¾
Therefore, 5 cos x – 17 sin y – tan x = – 4 ¾.
3. If q cos θ = p, find tan θ – cot θ in terms of p and q.
Solution:

Consider ABC as a triangle right angled at B and ∠ACB = θ
It is given that
q cos θ = p
cos θ = BC/AC = p/q
Take BC = px then AC = qx
In right-angled ∆ABC
Using Pythagoras theorem
AC2 = AB2 + BC2
It can be written as
AB2 = AC2 – BC2
Substituting the values
AB2 = (qx)2 – (px)2
AB2 = q2x2 – p2x2
Taking out the common terms
AB2 = (q2 – p2)x2
So we get
AB = √(q2 – p2) x2
AB = (√q2 – p2)x
In right-angled ∆ABC
tan θ = perpendicular/base
So we get
tan θ = AB/BC = [(√q2 – p2)x]/px
tan θ = (√q2 – p2)/p
In right-angled ∆ABC
cot θ = base/perpendicular
So we get
cot θ = BC/AB = px/[(√q2 – p2)x]
cot [(√q2 – p2)x] = p/(√q2 – p2)

4. Given 4 sin θ = 3 cos θ, find the values of:
(i) sin θ
(ii) cos θ
(iii) cot2 θ – cosec2 θ.
Solution:
It is given that
4 sin θ = 3 cos θ
sin θ/cos θ = ¾
tan θ = ¾

Consider ∆ABC right angled at B and ∠ACB = θ
tan θ = perpendicular/base
Substituting the values
¾ = AB/BC
AB/BC = ¾
Take AB = 3x then BC = 4x
In right-angled ∆ABC
Using Pythagoras theorem
AC2 = AB2 + BC2
Substituting the values
AC2 = (3x)2 + (4x)2
By further calculation
AC2 = 9x2 + 16x2 = 25x2
So we get
AC2 = (5x)2
AC = 5x
(i) In right-angled ∆ABC
sin θ = perpendicular/hypotenuse
So we get
sin θ = AB/AC = 3x/5x = 3/5
(ii) In right-angled ∆ABC
cos θ = base/hypotenuse
So we get
cos θ = BC/AC = 4x/5x = 4/5
(iii) In right-angled ∆ABC
cot θ = base/perpendicular
So we get
cot θ = BC/AB = 4x/3x = 4/3
In right-angled ∆ABC
cosec θ = hypotenuse/perpendicular
So we get
cosec θ = AC/AB = 5x/3x = 5/3
Here
cot2 θ – cosec2 θ = (4/3)2 – (5/3)2
By further calculation
= 16/9 – 25/9
= (16 – 25)/9
= -9/9
= – 1
Therefore, cot2 θ – cosec2 θ = -1.
5. If 2 cos θ = √3, prove that 3 sin θ – 4 sin3 θ = 1.
Solution:
It is given that
2 cos θ = √3
cos θ = √3/2
We know that
sin2 θ = 1 – cos2 θ
Substituting the values
= 1 – (√3/2)2
= 1 – ¾
= ¼
sin θ = √ ¼ = ½
Consider
LHS = 3 sin θ – 4 sin3 θ
It can be written as
= sin θ (3 – 4 sin2 θ)
Substituting the values
= ½ (3 – 4 × ¼)
= ½ (3 – 1)
= ½ × 1
= 1
= RHS
Therefore, proved.
6. If (sec θ – tan θ)/ (sec θ + tan θ) = ¼, find sin θ.
Solution:
We know that

By cross multiplication
4 – 4 sin θ = 1 + sin θ
We get
4 – 1 = sin θ + 4 sin θ
3 = 5 sin θ
sin θ = 3/5
7. If sin θ + cosec θ = 3 1/3, find the value of sin2 θ + cosec2 θ.
Solution:
It is given that
sin θ + cosec θ = 3 1/3 = 10/3
By squaring on both sides
(sin θ + cosec θ)2 = (10/3)2
Expanding using formula (a + b)2 = a2 + b2 + 2ab
sin2 θ + cosec2 θ + 2 sin θ cosec θ = 100/9
We know that sin θ = 1/cosec θ
sin2 θ + cosec2 θ + 2 sin θ × 1/ sin θ = 100/9
By further calculation
sin2 θ + cosec2 θ + 2 = 100/9
sin2 θ + cosec2 θ = 100/9 – 2
Taking LCM
sin2 θ + cosec2 θ = (100 – 18)/9 = 82/9
So we get
sin2 θ + cosec2 θ = 9 1/9
8. In the adjoining figure, AB = 4 m and ED = 3 m.
If sin α = 3/5 and cos β = 12/13, find the length of BD.

Solution:
It is given that
sin α = AB/AC = 3/5
AB = 3 and AC = 5
Using Pythagoras theorem
AC2 = AB2 + BC2
Substituting the values
52 = 32 + BC2
By further calculation
25 = 9 + BC2
BC2 = 25 – 9 = 16
So we get
BC2 = 42
BC = 4
We know that
tan α = AB/BC = 4/5
cos β = CD/CE = 12/13
CD = 12 and CE = 13
Using Pythagoras theorem
CE2 = CD2 + ED2
Substituting the values
132 = 122 + ED2
By further calculation
ED2 = 132 – 122
ED2 = 169 – 144 = 25
So we get
ED2 = (5)2
ED = 5
tan β = ED/CD = 5/12
From the figure
tan α = AB/BC = 4/BC
So we get
¾ = 4/BC
BC = (4 × 4)/3 = 16/3 m
tan β = ED/CD = 3/CD
5/12 = 3/CD
So we get
CD = (12 × 3)/5 = 36/5 m
Here
BD = BC + CD
Substituting the values
= 16/3 + 36/5
Taking LCM
= (80 + 108)/15
= 188/15 m
= 12 8/15 m