JEE Main 2021 March 16 – Shift 1 Maths Question Paper with Solutions

Section A

Question 1: Consider three observations a, b and c such that b = a+c. If the standard deviation of a+2, b+2, c+2 is d, then which of the following is true?

a. b² = a² + c² + 3d²
b. b² = 3 (a² + c²) – 9d²
c. b² = 3 (a² + c²) + 9d²
d. b² = 3 (a² + c² + d²)

Answer: (b)

For a, b, c mean =

S.D. of a, b, c = d

d² = [a² + b² + c²] / 3 – (4b² / 9)

b² = 3a² + 3c² – 9d²

Question 2: Let a vector

a. 1
b. 1 / 2
c. 1 / √2
d. 2 √2

Answer: (b)

(ɑ, β) = (2 cos 75^o, 2 sin 75^o)

Area = (1 / 2) (2 cos 75°)(2 sin 75°)

= sin (150°)

= 1 / 2 square unit

Question 3: If for a>0, the feet of perpendiculars from the points A (a, –2a, 3) and B (0, 4, 5) on the plane lx + my + nz = 0 are points C (0, –a, –1) and D respectively, then the length of line segment CD is equal to :

a. √41
b. √55
c. √31
d. √66

Answer: (d)

Direction cosines of plane = λ (direction cosines of line AC)

Direction cosines of plane = λa, – λa, 4λ

Hence equation plane is: ax – ay + 4z = 0

The point C lies on the plane

a (0) − a (− a)+ 4 (−1) = 0 ⇒ a = 2 (∵ a > 0)

So plane is 2x − 2y + 4z = 0, C ≡ (0,−2,−1)

So for coordinates of D,

D ≡ (- 1, 5, 3)

CD = √66 units

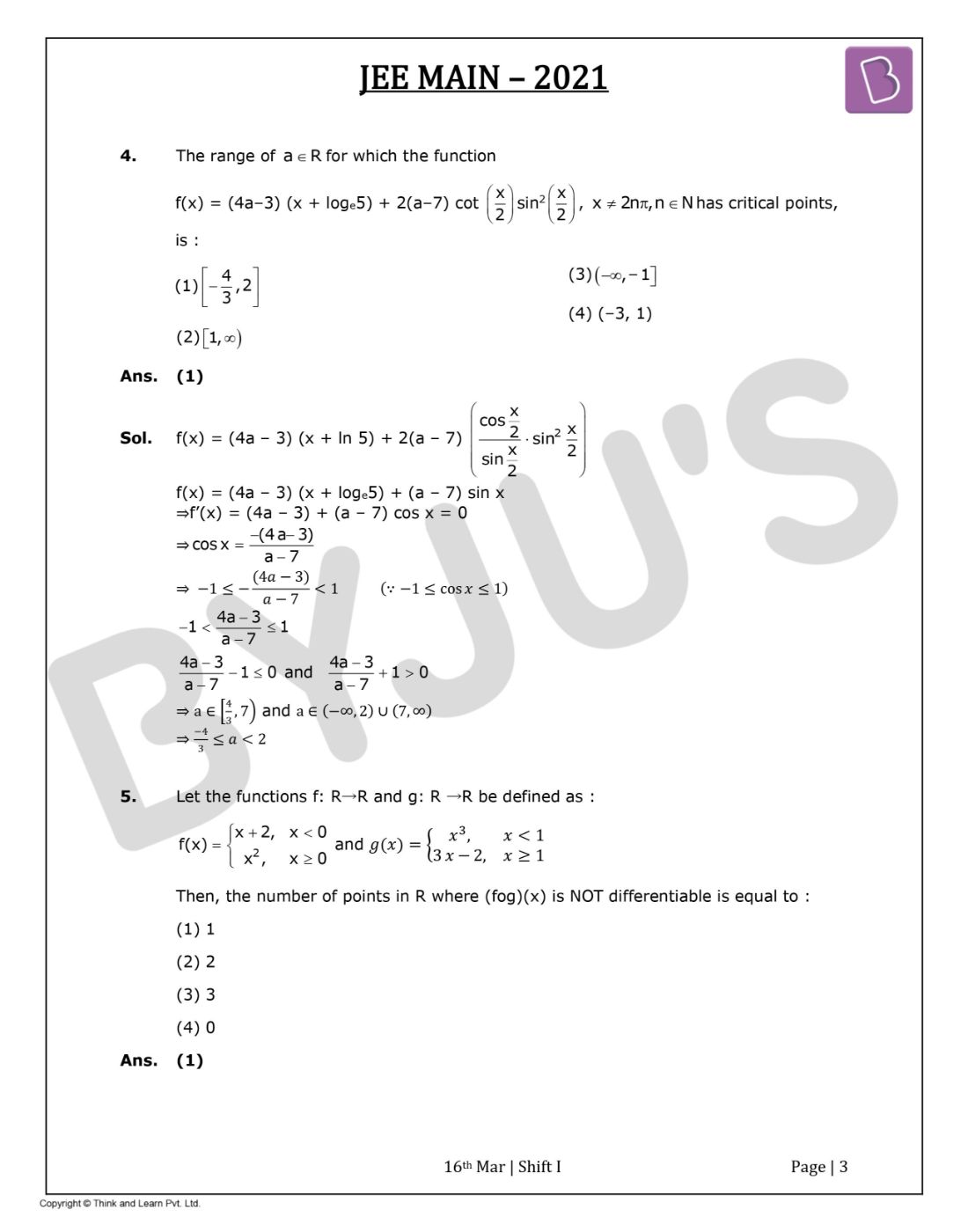
Question 4: The range of a ∈ R for which the function f (x) = (4a – 3) (x + log_e 5) + 2 (a – 7) cot (x / 2) sin² (x / 2), x ≠ 2nπ, n ∈ N has critical points, is

a. [- 4 / 3, 2]
b. [1, ∞)
c. (- ∞, – 1]
d. (– 3, 1)

Answer: (a)

f (x) = (4a – 3) (x + ln 5) + 2(a – 7) [(cos (x / 2) / sin (x / 2)) * sin² (x / 2)]

f (x) = (4a – 3) (x + log_e5) + (a – 7) sin x

f’ (x) = (4a – 3) + (a – 7) cos x = 0

cos x = – (4a – 3) / (a – 7)

– 1 ≤ – (4a – 3) / (a – 7) ≤ 1 (because – 1 ≤ cos x ≤ 1)

– 1 < [4a – 3] / [a – 7] ≤ 1

{[4a – 3] / [a – 7]} – 1 ≤ 0 and {[4a – 3] / [a – 7]} + 1 > 0

a.∈ [4 / 3, 7) and a ∈ (- ∞, 2) ⋃ (7, ∞)

(- 4 / 3) ≤ a < 2

Question 5: Let the functions f: R→R and g: R →R be defined as:

a. 1
b. 2
c. 3
d. 0

Answer: (a)

Clearly fog(x) is discontinuous at x = 0 then non-differentiable at x = 0

Now,

at x = 1

RHD = lim_h→0+ [f (1 + h) – f (1) ] / [h] = lim_h→0+ {[3 (1 + h) – 2]² – 1} / h = 6

LHD = lim_h→0- [f (1 – h) – f (1) ] / [- h] = lim_h→0- [(1 – h)⁶ – 1] / – h = 6

Number of points of non-differentiability = 1

Question 6:Let a complex number z, |z| ≠ 1, satisfy log_1/√2 [(|z| + 11) / (|z| – 1)²] ≤ 2. Then, the largest value of |z| is equal to ____

a. 5
b. 8
c. 6
d. 7

Answer: (d)

2|z| + 22 ≥ (|z| – 1)²

2|z| + 22 ≥ |z|² – 2|z| + 1

|z|² – 4|z| – 21 ≤ 0

(|z| – 7) (|z| + 3) ≤ 0

|z| ≤ 7

|z|_max = 7

Question 7: A pack of cards has one card missing. Two cards are drawn randomly and are found to be spades. The probability that the missing card is not a spade is:

a. 3 / 4
b. 52 / 867
c. 39 / 50
d. 22 / 425

Answer: (c)

= [1 – (13 / 52) * (13 / 51) (12 / 50)] / [(1 – (13 / 52) * {(13 / 51) (12 / 50) + (13 / 52) (12 / 51) * (11 / 50)}]

= 39 / 50

Question 8: If n is the number of irrational terms in the expansion of [3^1/4 + 5^1/8]⁶⁰, then (n – 1) is divisible by

a. 8
b. 26
c. 7
d. 30

Answer: (b)

T_r+1 = ⁶⁰C_r (3^1/4)^60-r (5^1/8)^r

It is rational if [60 – r] / 4, (r / 8), both are whole numbers, r ∈ {0,1,2,….60}

Common terms r ∈ {0,8,16,….56}

So 8 terms are rational

Then irrational terms = 61 – 8 = 53 = n

 ∴n – 1 = 52 = 13 × 2²

The factors are 1,2,4,13,26,52.

Question 9: Let the position vectors of two points P and Q be 3i – j + 2k and i + 2j – 4k, respectively. Let R and S be two points such that the direction ratios of lines PR and QS are (4, –1, 2) and (–2,1,–2) respectively. Let lines PR and QS intersect at T. If the vector TA is perpendicular to both PR and QS and the length of vector TA is √5 units, then the modulus of a position vector of A is:

a. √5
b. √171
c. √227
d. √482

Answer: (b)

P = 3i – j + 2k and Q = i + 2j – 4k

v_PR = (4, – 1, 2) and v_QS = (- 2 , 1, – 2)

L_PR = (3i – j + 2k) + λ (4i – 1j + 2k)

L_QS : r = (i + 2j – 4k) + 𝝁 (- 2i + 1j – 2k)

Now T on PR = (3 + 4λ, – 1 – λ, 2 + 2λ)

Similarly T on QS = (1 − 2𝝁, 2 + 𝝁,−4 − 2𝝁)

For λ and 𝝁:

3 + 4λ = 1 – 2𝝁 ⇒ 𝝁 + 2λ = – 1 ⇒ λ = 2

– 1 – λ = 2 + 𝝁 ⇒ 𝝁 + λ = – 3 ⇒ 𝝁 = – 5

And 2 + 2λ = – 4𝝁

T : (11, – 3, 6)

D.R. of TA = v_QS x v_PR

L_TA : r = (11i – 3j + 6k) + λ (- 4j – 2k)

Now A = (11,− 3 − 4λ, 6 − 2λ)

TA = √5

(4λ)² + (2λ)² = 5

16λ² + 4λ² = 5

λ = ± 1 / 2

A: (11, –5, 5) or A: (11, –1, 7)

|A| = √(121 + 25 + 25) or |A| = √(121 + 1 + 49)

= √171 or √171

Question 10: If the three normals drawn to the parabola, y² = 2x pass through the point (a, 0) a ≠ 0, then ‘a’ must be greater than

a. 1
b.1 / 2
c. – 1 / 2
d. –1

Answer: (1)

Let the equation of the normal be y = mx – 2am – am³.

Here 4a = 2

a = 1 / 2

y = mx – m – (1 / 2) m³

It passes through A (a, 0) then

0 = am – m – (1 / 2) m³

m = 0, m² – 2 (a – 1) = 0

For real values of m

2 (a – 1) > 0

a > 1

Question 11: Let S_k = ∑_r=1^k tan^-1[(6^r) / (2^r+1 + 3^2r+1)]. Then lim_k→∞ S_k =

a. tan^-1 (3 / 2)
b. cot^-1 (3 / 2)
c. π / 2
d. tan^–1(3)

Answer: (2)

Question 12: The number of roots of the equation, (81)^sin2x + (81)^cos2x = 30 in the interval [0, π] is equal to :

a. 3
b. 2
c. 4
d. 8

Answer: (3)

(81)^sin2x + (81)^cos2x = 30

(81)^sin2x + [81 / (81)^sin2x] = 30

Let (81)^sin2x = t

t + (81 / t) = 30 ⇒ t² + 81 = 30t

t² – 30t + 81 = 0

t² – 27t – 3t + 81 = 0

(t – 3) (t – 27) = 0

t = 3, 27

(81)^sin2x = 3, 3³

3^4sin2x = 3¹, 3³

4 sin² x = 1, 3

sin² x = (1 / 4), (3 / 4) in [0, π] sin x ≥ 0

sin x = 1 / 2, √3 / 2

x = π / 6, 5π / 6, π / 3, 2π / 3

Number of solutions = 4

Question 13: If y = y(x) is the solution of the differential equation, dy / dx + 2y tanx = sinx, y (π / 3) = 0, then the maximum value of the function y(x) over R is equal to :

a. 8
b. 1 / 2
c. – 15 / 4
d. 1 / 8

Answer: 4

dy / dx + 2y tanx = sinx

IF = e^{ln (sec2x)} = sec² x

y sec²x = ∫tanx secx dx = secx + c

Now, x = π / 3, y = 0, c = – 2

y = cosx – 2cos² x

y = – 2 [cos² x – (1 / 2) cosx] = – 2 [(cosx – (1 / 4))² – (1 / 16)]

y = (1 / 8) – 2 [cosx – (1 / 4)]²

y_max = 1 / 8

Question 14: Which of the following Boolean expression is a tautology?

a. (p ∧ q) ∧ (p → q)
b. (p ∧ q) ∨ (p ∨ q)
c. (p ∧ q) ∨ (p → q)
d. (p ∧ q) → (p → q)

Answer: (4)

Question 15: Let

a. No solution
b. Exactly two solutions
c. A unique solution
d. Infinitely many solutions

Answer: (1)

Question 16: If for x ∈ (0, π / 2), log₁₀sin x + log₁₀cos x = –1 and log₁₀(sin x+cos x) = 1 / 2 (log₁₀ n – 1), n>0, then the value of n is equal to:

a. 16
b. 20
c. 12
d. 9

Answer: (3)

log₁₀sin x + log₁₀cos x = –1

sinx * cosx = 1 / 10 — (1)

log₁₀ (sinx + cosx) = (1 / 2) (log₁₀ n – 1)

(sinx + cosx) = (n / 10)^½

sin² x + cos² x + 2 sinx cosx = n / 10 (squaring)

1 + 2 (1 / 10) = n / 10 [using equation (1)]

n / 10 = 12 / 10

n = 12

Question 17: The locus of the midpoints of the chord of the circle, x² + y² = 25 which is tangent to the hyperbola, x² / 9 – y² / 16 = 1 is :

a. (x² + y²)² – 16x² + 9y² = 0
b. (x² + y²)² – 9x² + 144y² = 0
c. (x² + y²)² – 9x² – 16y² = 0
d. (x² + y²)² – 9x² + 16y² = 0

Answer: (4)

The tangent of hyperbola y = mx ± √(9m² – 16) …(i) which is a chord of circle with mid-point (h, k).

So, the equation of chord T = S₁

hx + ky = h² + k²

y = – (hx / k) + (h² + k²) / k —- (ii)

By (i) and (ii)

m = – h / k and √9m² – 16 = [h² + k²] / k

9 (h² / k²) – 16 = (h² + k²)² / k²

The locus 9x² – 16y² = (x² + y²)².

Question 18: Let [x] denote the greatest integer less than or equal to x. If for n ∈ N, (1 – x + x³)ⁿ = ∑_j=0³ⁿ a_jx^j, then ∑_j=0^3n/2 a_2j + 4 ∑_j=0^(3n-1)/2 a_2j+1 =

a. 1
b. n
c. 2^n–1
d. 2

Answer: (1)

(1 – x + x³)ⁿ = ∑_j=0³ⁿ a_jx^j

(1–x + x³)ⁿ = a₀ + a₁x + a₂x² + ….. + a_3n x³ⁿ

Put x = 1

1 = a₀ + a₁ + a₂ + a₃ + a₄ + …… +a_3n …(1)

Put x = –1

1 = a₀ – a₁ + a₂ – a₃ + a₄ …… (–1)³ⁿa_3n …(2)

Add (1) + (2)

a₀ + a₂ + a₄ + a₆ + …….. = 1

Sub (1) – (2)

⇒ a₁ + a₃ + a₅ + a₇ + …….. = 0

Now ∑_j=0^3n/2 a_2j + 4 ∑_j=0^(3n-1)/2 a_2j+1 =

= (a₀ + a₂ + a₄ + …..) + 4(a₁ + a₃+ ….)

= 1 + 4 × 0

= 1

Question 19: Let P be a plane lx + my + nz = 0 containing the line, [1 – x] / 1 = [y + 4] / 2 = [z + 2] / 3. If plane P divides the line segment AB joining points A (–3, –6, 1) and B (2, 4, –3) in ratio k : 1 then the value of k is equal to :

a. 1.5
b. 2
c. 4
d. 3

Answer: (2)

Line lies on plane

– l + 2m + 3n = 0 …(1)

Point on line (1,– 4, – 2) lies on plane

l – 4m – 2n = 0 …(2)

From (1) & (2)

–2m + n = 0

2m = n

l = 3n + 2m

l = 4n

l : m : n :: 4n : (n / 2) : n

l : m : n :: 8n : (n) : 2n

l : m : n :: 8 : 1 : 2

Now equation of plane is 8x + y + 2z = 0

R divide AB is ratio k : 1

R : [(- 3 + 2k) / (k + 1), (- 6 + 4k) / (k + 1), (1 – 3k) / (k + 1)] lies on the plane

8 [(- 3 + 2k) / (k + 1) + (- 6 + 4k) / (k + 1) + 2 (1 – 3k) / (k + 1)] = 0

–24 + 16 k – 6+ 4k + 2 – 6k = 0

–28 + 14k = 0

k = 2

Question 20: The number of elements in the set x ∈ R : (|x| – 3) |x + 4| = 6) =

a. 2
b. 1
c. 3
d. 4

Answer: (1)

Case – 1: x ≤ – 4

(–x – 3)(–x – 4) = 6

(x + 3) (x + 4) = 6

x² + 7x + 6 = 0

x = –1 or – 6

But x ≤ – 4

x = – 6

Case – 2: x ∈ (– 4, 0)

(–x – 3)(x + 4) = 6

–x² – 7x – 12 – 6 = 0

x² + 7x + 18 = 0

D < 0 No solution

Case – 3: x ≥ 0

(x – 3)(x + 4) = 6

x² + x – 12 – 6 = 0

x² + x – 18 = 0

x = (- 1 ± √73) / 2

Only x = (√73 – 1) / 2

Hence, 2 elements only

Section – B

Question 21: Let f: (0, 2) → R be defined as f (x) = log₂ [1 + tan (πx / 4)]. Then, lim_n→∞ (2 / n) [f (1 / n) + f (2 / n) ……. f (1)] is equal to ______

Answer: (1)

Question 22: The total number of 3 × 3 matrices A having entries from the set {0, 1, 2, 3} such that the sum of all the diagonal entries of AA^T is 9, is equal to ____

Answer: (766)

Tr (AA^T) = x² + y² + z² + a² + b² + c² + d² + e² + f² = 9

all→1 =1

one 3, rest = 0 (9! / 8!) = 9

two 2 , one 1 & rest 0 (9!) / (2! 6!) = 63 × 4 = 252

one 2 , five 1, rest 0 (9!) / (5! 3!) = 63 × 8 = 504

Total = 766

Question 23: Let f:R→R be a continuous function such that f (x) + f (x + 1) = 2, for all x ∈ R. If I₁ = ∫₀⁸ f (x) dx and I₂ = ∫_-1³ f (x) dx, then the value of I₁ + 2I₂ is equal to ____

Answer: (16)

f (x) + f (x + 1) = 2 …..(i)

x→(x + 1)

f(x + 1) + f(x + 2) = 2 …..(ii)

By (i) & (ii)

f(x) – f(x + 2) = 0

f(x + 2) = f(x)

f(x) is periodic with T = 2

I₁ = ∫₀^2*4 f (x) dx = 4 ∫₀² f (x) dx

I₂ = ∫_-1³ f (x) dx = ∫₀⁴ f (x + 1) dx = ∫₀⁴ [2 – f (x)] dx

I₂ = 8 – 2 ∫₀² f (x) dx

I₁ + 2I₂ = 16

Question 24: Consider an arithmetic series and a geometric series having four initial terms from the set {11, 8, 21, 16, 26, 32, 4}. If the last terms of these series are the maximum possible four-digit numbers, then the number of common terms in these two series is equal to ____

Answer: (3)

By observation

A.P : 11, 16, 21, 26 …….

G.P : 4, 8, 16, 32 …….

So common terms are 16, 256, 4096.

Question 25: If the normal to the curve y (x) = ∫₀^x (2t² – 15t + 10) dt at a point (a, b) is parallel to the line x + 3y = – 5, a>1, then the value of |a + 6b| is equal to ____

Answer: (406)

y’ (x) = (2x² – 15x + 10)

At point (a, b) normal is

3 = (2a² – 15a + 10)

2a² – 15a + 7 = 0

2a² – 14a – a + 7 = 0

2a (a – 7) – 1 (a – 7) = 0

a = 1 / 2 or 7,

Given a > 1, a = 7

Also P lies on curve,

b = ∫₀^a (2t² – 15t + 10) dt

b = ∫₀⁷ (2t² – 15t + 10) dt

6b = – 413

|a + 6b| = 406

Question 26: If lim_x→0 [ae^x – b cosx + ce^-x] / [x sinx] = 2, then a + b + c is equal to ______

Answer: (4)

a – b + c = 0 & a – c = 0 & (a / 2) + (b / 2) + (c / 2) = 2

a + b + c = 4

Question 27: Let ABCD be a square of the side of unit length. Let a circle C₁ centred at A with a unit radius is drawn. Another circle C₂ which touches C₁ and the lines AD and AB is tangent to it, is also drawn. Let a tangent line from point C to the circle C₂ meet the side AB at E. If the length of EB is ɑ + √3β, where ɑ, β are integers, then ɑ + β is equal to ___

Answer: (1)

(i) √2r + r = 1

r = 1 / (√2 + 1)

r = √2 – 1

(ii) CC₂ = 2√2 – 2 = 2 (√2 – 1)

From △CC₂N = sin ɸ = (√2 – 1) / [2 (√2 – 1)]

ɸ = 30^o

(iii) In △ACE are sine law

AE / sin ɸ = AC / sin 105^o

AE = (1 / 2) * (√2 / (√3 + 1)) * 2√2

AE = 2 / (√3 + 1) = √3 – 1

EB = 1 – (√3 – 1)

ɑ = 2, β = – 1

ɑ + β = 1

Question 28: Let z and w be two complex numbers such that

Answer: (4)

Let z = x + iy

|z + i| = |z–3i|

y = 1

Now w = x² + y² – 2x – 2iy + 2

w = x² + 1 – 2x –2i + 2

Re(w) = x² – 2x + 3

Re(w) = (x–1)² + 2

Re(w)_min at x = 1

z = 1 + i

Now w = 1 + 1 –2 – 2i + 2

w = 2(1–i) = 2√2 e^i(-π/4)

wⁿ = 2√2 e^i(-π/4)

If wⁿ is real, n = 4

Question 29: Let

Answer: (36)

|P^-1AP – I|²

= |(P^-1AP – I) (P^-1AP – I)|

= |P^-1APP^-1 AP – 2P^-1AP + I|

= |P^-1A²P – 2P^-1AP + P^-1IP|

= |P^-1(A² – 2A + I) P|

= |P^-1(A – I)² P|

= |P^-1| |A – I|² |P|

= |A – I|²

=

= (1 (𝛚 (𝛚 + 1) + 𝛚) – 7𝛚 + 𝛚² . 𝛚)²

= (𝛚² + 2𝛚 – 7𝛚 + 1)²

= (𝛚² – 5𝛚 + 1)²

= (- 6𝛚)²

= 36𝛚² ⇒ ɑ = 36

Question 30: Let the curve y=y(x) be the solution of the differential equation, dy / dx = 2 (x + 1). If the numerical value of area bounded by the curve y = y(x) and the x-axis is 4√8 / 3, then the value of y(1) is equal to ___

Answer: (2)

y = x² + 2x + c

Area of rectangle ABCD = |(c – 1)√(1 – c)|

Area of parabola and x-axis = 2 (2 / 3 [(1 – c)^3/2]) = 4√8 / 3

1 – c = 2

c = –1

Equation of f (x) = x² + 2x – 1

f (1) = 1 + 2 – 1 = 2