Thermal expansion is the tendency of an object to change its dimension due to heat. The change can be either in length, area or volume. When the substance is heated it increases the kinetic energy of the molecules present in it. Depending on the type of expansion, thermal expansion is of 3 types:
Linear expansion
Area expansion
Volume expansion
Download JEE Advanced Previous Year Questions with Solutions on Thermal Expansion PDF
Question 1)When the temperature of a metal wire is increased from 00C to 100C, its length increases by 0.02%. The percentage change in its mass density will be closest to:
(A) 0.06
(B) 2.3
(C)0.008
(D) 0.8
Answer: (A) 0.06
Solution:
Change in the length of the metal wire (Δl) when its temperature is changed by ΔT is given by
Δl = lαΔT
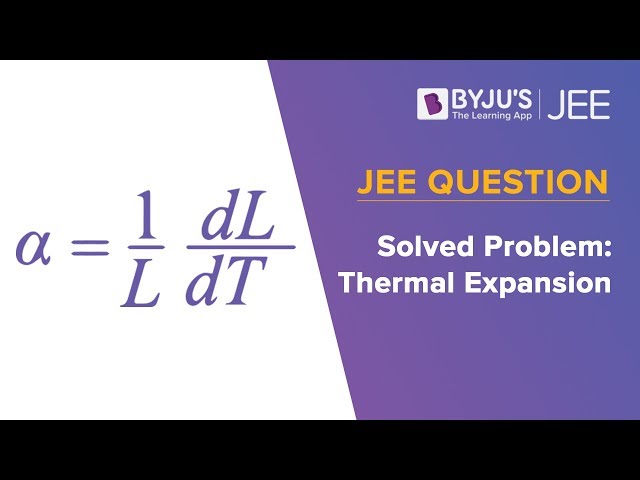
Here, α = coefficient of linear expansion
Δl = 0.02%, ΔT = 100C
Therefore, α = Δl/lΔT
= 0.02/ (100 x 10)
⇒ α = 2 x 10-5
Volume coefficient of expansion, γ = 3α = 6 x 10-5
Since ρ = M/V
(ΔV/V) x 100 = γΔT = (6 x 10-5 x 10 x 100) = 6 x 10-2
Volume increase by 0.06% therefore density decreases by 0.06%
Question 2) On a linear temperature scale Y, water freezes at -1600 Y and boils at -500Y. On this Y scale, a temperature of 340 K would be read as (water freezes at 273 K and boils at 373 K)
(A) -73.70 Y
(B) -233.70 Y
(C)-86.30 Y
(D) -106.30 Y
Answer:(C)-86.30 Y
Solution:
(Reading on any scale – LFP)/(UFP – LFP)
= constant for all scales
(340 – 273)/(373 – 273) = (Y – (-160))/(-50 – (-160))
⇒ (67/100) = (Y + 160)/110
Y = – 86.30 Y
Question 3) A metal ball immersed in alcohol weighs W1 at 00C and W2 at 500C. The coefficient of expansion of cubical metal is less than that of alcohol. Assuming that the density of the metal is large compared to that of alcohol, it can be shown that
(A)W1 > W2
(B) W1 = W2
(C)W1 < W2
(D) None of these
Answer: (C)W1 < W2
Solution:

Question 4) A non-isotropic solid metal cube has a coefficient of linear expansion as 5 x 10-5 /0C along the x-axis and 5 x 10-6 /0C along the Y and the Z-axis. If the coefficient of volume expansion of the solid is C x 10-6 /0C then the value of C is ________
Answer: 60
Solution:
Volume, V = lbh
γ = (ΔV/VΔt) = (Δl/lΔt) + (Δb/bΔt) + (Δh/hΔt)
( γ = coefficient of volume expansion)
⇒γ = 5 x 10-5 + 5 x 10-6 + 5 x 10-6
= 60 x 10-6 /0C
Therefore, value of C = 60.00
Question 5) A bakelite beaker has a volume capacity of 500 cc at 300 C. When it is partially filled with Vm volume (at 300 C) of mercury, it is found that the volume of the beaker remains constant as the temperature is varied. If γbeaker = 6 x 10-6 /0C and γmercury = 1.5 x 10-4 /0C, where γ is the coefficient of volume expansion, then Vm (in cc) is close to
Answer: 20
Solution:
Volume capacity of the beaker, V0 = 500 cc
Vb = V0 + V0γbeaker ΔT
When beaker is partially filled with Vm volume of mercury
Vb’ = Vm + VmγmΔT
Unfilled volume (V0 – Vm) = (Vb – Vm’)
⇒V0 γbeaker = Vmγm
Therefore, Vm = (V0 γbeaker) /γm
= (500 x 6 x 10-6)/(1.5 x 10-4)
= 20 cc
Question 6) Two rods A and B of identical dimensions are at temperature 300C. If A is heated upto 1800 C and B upto T0 C, then the new lengths are the same.If the ratio of the coefficient of linear expansion of A and B is 4:3, then the value of T is
(A) 2300 C
(B) 2700 C
(C) 2000 C
(D) 2500 C
Answer: (A) 2300 C
Solution:
Change in length in both rods are same
i.e △l1 = △l2
lα1△θ1 =lα2△θ2
(α1/α2) =(△θ2/△θ1)
(4/3) = (θ – 30)/(180 – 30)
θ = 2300 C
Question 7)A rod, of length L at room temperature and uniform area of cross-section A, is made of a metal having a coefficient of linear expansion α/0C. It is observed that an external compressive force F, is applied on each of its ends, prevents any change in the length of the rod, when its temperature rises by ΔT K. Young’s modulus, Y for this metal is
(A) F/Aα△T
(B) F/Aα(△T- 273)
(C) F/2Aα△T
(D) 2F/Aα△T
Answer:(A) F/Aα△T
Solution:
Young’s modulus Y = Stress/Strain = (F/A)/((△l/l))
Using coefficient of linear expansion
α = △l/l△T
⇒ △l/l =α △T
Therefore, Y = F/Aα△T
Question 8) At 400C, a brass wire of 1mm radius is hung from the ceiling. A small mass, M is hung from the free end of the wire. When the wire is cooled down from 400C to 200C it regains its original length of 0.2 m. The value of M is close to
(Coefficient of linear expansion and Young’s modulus of brass are 10-5/0C and 1011 N/m2, respectively; g = 10 m/s2)
(A) 9 Kg
(B) 0.5 Kg
(C) 1.5 kg
(D) 0.9 kg
Answer: 6.28 Kg (Bonus)
Solution:
Δtemp = Δload
and A = πr2 = π(10-3)2 = π x 10-6
LαΔT = FL/AY
0.2 x 10-5 x 20 = (F x 0.2/ (π x 10-6) x 1011
F = 20π N
Therefore,m = F/g = 2π= 6.28 Kg
Question 9) An external pressure P is applied on a cube at 00C so that it is equally compressed from all sides. K is the bulk modulus of the material of the cube and α is its coefficient of linear expansion. Suppose we want to bring the cube to its original size by heating it. The temperature should be raised by
(A) 3α/PK
(B) 3PKα
(C) P/ 3αK
(D) P/αK
Answer: (C) P/ 3αK
Solution:

Question 10) A compressive force, F is applied at the two ends of a long thin steel rod. It is heated, simultaneously, such that its temperature increases by ΔT. The net change in its length is zero. Let l be the length of the rod, A its area of cross-section, Y its Young’s modulus, and α its coefficient of linear expansion. Then, F is equal to:
(A) l2YαΔT
(B) lAYαΔT
(C)AYαΔT
(D) AY/αΔT
Answer: (C)AYαΔT
Solution:
Due to thermal expansion, change in length (Δl) = lαΔT
Young’s modulus (Y) = Normal Stress/Longitudinal Strain
Y = (F/A)/(Δl/l)
⇒ Δl = Fl/AY
Fl/AY = lαΔT
F = AYαΔT
Video Lesson – Thermal Expansion

Solved Problem on Thermal Expansion
Also Read:
JEE Main Thermal Expansion Previous Year Questions with Solutions
Comments