Elasticity

Table of Contents

What Is meant by Elasticity?
SI Unit of Elasticity
Types of Stress
Longitudinal Stress
Volume Stress or Bulk Stress
Tangential Stress or Shear Stress
What Is a Strain?
Longitudinal Strain
Volume Strain
Shearing Strain
Stress-Strain Curve
Elastic Hysteresis
Hooke’s Law
Young’s Modulus of Elasticity “y”
Bulk Modulus (B)
Rigidity Modulus
Poisson’s Ratio
Frequently Asked Questions – FAQs

What Is meant by Elasticity?

When an external force is applied to a rigid body, there is a change in its length, volume (or) shape. When external forces are removed, the body tends to regain its original shape and size. Such a property of a body by virtue of which a body tends to regain its original shape (or) size when external forces are removed is called elasticity.

SI Unit of Elasticity

The SI unit for elasticity is the pascal (Pa). It is defined as force per unit area. Typically, it is a measure of pressure, which in classical mechanics points to stress. The pascal has the dimension L⁻¹⋅M⋅T⁻².

Elastic Stress and Strain

What Is Stress?

When the body is deformed by the application of external forces, forces within the body are brought into play. Elastic bodies regain their original shape due to internal restoring forces. The internal forces and external forces are opposite in direction. If a force F is applied uniformly over a surface of area A, then the stress is defined as the force per unit area.

Stress = Force/Area

SI unit for stress is Nm^-2

Types of Stress

There are three types of stress:

Longitudinal stress
Volume stress or bulk stress
Tangential stress or shear stress

Longitudinal Stress

When the stress is normal to the surface area of the body, and there is a change in the length of the body, it is known as longitudinal stress.

Again it is classified into two types:

Tensile stress
Compressive stress.

Tensile stress: When longitudinal stress is produced due to an increase in the length of the object, it is known as tensile stress.

Compressive stress: Longitudinal stress produced due to the decrease in length of the object is known as compressive stress.

Volume Stress or Bulk Stress

If equal normal forces applied to the body causes a change in the volume of the body, the stress is called volume stress.

Tangential Stress or Shear Stress

When the stress is tangential or parallel to the surface of the body, it is known as tangential or shear stress. Due to this, the shape of the body changes (or) gets twisted.

What Is a Strain?

A body under stress gets deformed. The fractional change in the dimension of a body produced by the external stress acting on it is called strain. The ratio of charge of any dimension to its original dimension is called strain. Since strain is the ratio of two identical physical quantities, it is just a number. It has no unit or dimension.

\(\begin{array}{l}Strain=\frac{Change\,in\,dimension}{initial\,dimension}\end{array} \)

Strain is also classified into three types:

Longitudinal strain
Volume strain
Shearing strain or tangential strain

Longitudinal Strain

The strain under longitudinal stress is called longitudinal strain.

\(\begin{array}{l}Longitudinal\,strain=\frac{Change\,in\,length\,of\,the\,body}{initial\,length\,of\,the\,body}\end{array} \)

\(\begin{array}{l}=\frac{\Delta L}{L}\end{array} \)

Volume Strain

The strain caused by volume stress is called volume strain.

\(\begin{array}{l}Volume\,strain=\frac{Change\,in\,volume\,of\,the\,body}{\,Original\,volume\,of\,the\,body}\end{array} \)

\(\begin{array}{l}=\frac{\Delta V}{V}\end{array} \)

Shearing Strain

When a deforming force is applied to a body parallel to its surface, its shape (not size) changes, and this is known as shearing strain. The angle of shear Φ

\(\begin{array}{l}\tan \phi =\frac{\ell }{L}=\frac{displacement\,of\,upper\,face}{distance\,between\,two\,faces}\end{array} \)

Stress-Strain Curve

Elasticity Stress-Strain Curve

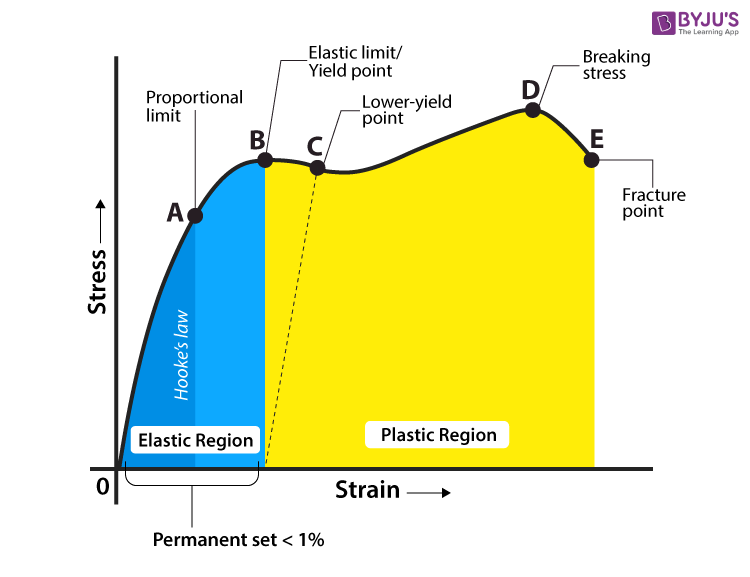
Proportion limit: The limit in which Hooke’s law is valid, and stress is directly proportional to strain.
Elastic limit: The maximum stress, which on removing the deforming force, makes the body recover its original state completely.
Lower yield point: The point beyond the elastic limit at which the length of the wire starts increasing with increasing stress. It is defined as the yield point.
Breaking point or fracture point: The point when the strain becomes so large that the wire breaks is called the breaking point.

Elastic Hysteresis

The strain persists even when the stress is removed, and this lagging behind of strain is called elastic hysteresis. This is why the values of strain for the same stress are different while increasing the load and decreasing the load.

Hooke’s Law

If deformation is small, the stress in a body is proportional to the corresponding strain; this fact is known as Hooke’s law.

Within elastic limit, stress & strain

\(\begin{array}{l}\Rightarrow \frac{Stress}{Strain}=Constant\end{array} \)

This constant is known as the modulus of elasticity (or) coefficient of elasticity. The elastic modulus has the same physical unit as stress. It only depends on the type of material used. It is independent of stress and strain. The modulus of elasticity is of three types:

Young’s modulus of elasticity “y”
The bulk modulus of elasticity “B”
Modulus of rigidity

Young’s Modulus of Elasticity “y”

Within the elastic limit, the ratio of longitudinal stress and longitudinal strain is called Young’s modulus of elasticity (y).

\(\begin{array}{l}y=\frac{Longitudinal\,stress}{Longitudinal\,strain}=\frac{\frac{F}{A}}{\frac{\ell }{L}}=\frac{FL}{A\ell }\end{array} \)

Within the elastic limit, the force acting upon a unit area of a wire by which the length of the wire becomes double is equivalent to Young’s modulus of elasticity of the material of the wire. If L is the length of the wire, r is the radius and is the increase in the length of the wire by suspending a weight (mg) at its one end, then Young’s modulus of elasticity of the wire becomes,

\(\begin{array}{l}y=\frac{F/A}{\ell /L}=\frac{FL}{A\ell }=\frac{mgL}{\pi {{r}^{2}}\ell }\end{array} \)

(a) The increment of the length of an object by its own weight:

Let a rope of mass M and length (L) be hanged vertically. As the tension of different points on the rope is different, similarly, the stress, as well as strain, will be different at different points.

Maximum stress at hanging point
Minimum stress at a lower point

Consider a dx element of rope at x distance from the lower end, then tension

\(\begin{array}{l}T=\left( \frac{M}{L} \right)\times g\end{array} \)

So, stress

\(\begin{array}{l}=\frac{T}{A}=\left( \frac{M}{L} \right)\frac{xg}{A}\end{array} \)

Let the increase in length of element dx be dy, then

\(\begin{array}{l}Strain=\frac{Change\,in\,length}{Original\,length}=\frac{\Delta y}{\Delta x}=\frac{dy}{dx}\end{array} \)

Now, we get stress and strain, then Young’s modulus of elasticity “y”

\(\begin{array}{l}y=\frac{Stress}{Strain}=\frac{\left( \frac{M}{L} \right)\frac{xg}{A}}{\frac{dy}{dx}}\Rightarrow \left( \frac{M}{L} \right)\frac{xg}{A}dx=\frac{1}{dy}\end{array} \)

The total change in length of the wire is

\(\begin{array}{l}\frac{Mg}{LA}\int\limits_{o}^{L}{x\,dx}=y\int\limits_{o}^{\Delta l}{dy}\end{array} \)

\(\begin{array}{l}\frac{Mg}{LA}\frac{{{L}^{2}}}{2}=y\Delta \ell\end{array} \)

\(\begin{array}{l}\frac{MgL}{2Ay}=\Delta \ell\end{array} \)

(b) Work done in stretching a wire

If we need to stretch a wire, we have to do work against its inter-atomic forces, which are stored in the form of elastic potential energy.

For a wire of length (L₀) stretched by a distance (x), the restoring elastic force is

\(\begin{array}{l}F=(Stress)(Area)=y\left[ \frac{x}{{{L}_{0}}} \right]A\end{array} \)

Work required for increasing an element length

\(\begin{array}{l}dW=Fdx=\frac{{{y}_{A}}}{{{L}_{0}}}x\,dx\end{array} \)

The total work required to stretch the wire is

\(\begin{array}{l}W=\int\limits_{0}^{\Delta \ell }{Fdx=\frac{{{y}_{A}}}{{{L}_{0}}}}\int\limits_{0}^{\Delta \ell }{x\,dx}\end{array} \)

\(\begin{array}{l}=\frac{{{y}_{A}}}{{{L}_{0}}}\left[ \frac{{{x}^{2}}}{2} \right]_{0}^{\Delta \ell }\end{array} \)

\(\begin{array}{l}=\frac{{{y}_{A}}{{\left( \Delta \ell \right)}^{2}}}{2{{L}_{0}}}\end{array} \)

(c) Analogy of rod as a spring

From the definition of Young’s modulus,

\(\begin{array}{l}y=\frac{Stress}{Strain}=\frac{FL}{A\,\Delta L}\end{array} \)

\(\begin{array}{l}F=\frac{{{y}_{A}}\Delta L}{L}\end{array} \)

This expression is an analogy of spring force

\(\begin{array}{l}F=kx\end{array} \)

\(\begin{array}{l}k=\frac{yA}{L}=\text{constant}\end{array} \)

Bulk Modulus (B)

Within the elastic limit, the ratio of the volume stress and the volume strain is called the bulk modulus of elasticity.

\(\begin{array}{l}B=\frac{Volume\,stress}{Volume\,strain}=\frac{\frac{F}{A}}{-\frac{\Delta V}{V}}=\frac{\Delta P}{\frac{-\Delta V}{V}}\end{array} \)

Rigidity Modulus

Within the elastic limit, the ratio of shearing stress (or) tangential stress and shearing strain (or) tangential strain is called the modulus of rigidity.

\(\begin{array}{l}\eta =\frac{Shearing\,stress}{Shearing\,strain}=\frac{\frac{{{F}_{tangential}}}{A}}{\phi }\end{array} \)

Φ – the angle of shear.

Poisson’s Ratio

Within the elastic limit, the ratio of lateral strain (or) transverse train and longitudinal strain is called Poisson’s ratio. In the case of a circular bar of material, the change in the diameter of the circular bar material to its diameter is due to deformation in the longitudinal direction.

\(\begin{array}{l}Poisson’s\,ratio(\sigma )=\frac{lateral\,strain}{longitudinal\,strain}=\frac{\beta }{\alpha }\end{array} \)

You might also be interested in the following:

Watch the video and learn about the concept of Hooke’s Law

Frequently Asked Questions on Elasticity

What is elasticity?

It is the ability of the deformed objects to regain their actual shape and size when the force causing the deformation is removed.

What are the types of modulus of elasticity?

There are three types of modulus of elasticity, namely Young’s modulus, shear modulus and bulk modulus.

What is the SI unit for the modulus of elasticity?

The SI unit for the modulus of elasticity is Pascal.

Define Hooke’s law.

Within the elastic limit of the material, the strain caused in the material is proportional to the applied stress.

Define Young’s modulus of elasticity.

Young’s modulus of elasticity is the ratio of the normal stress to the longitudinal strain.

Is stress a vector quantity?

No, stress is a scalar quantity.

Young’s modulus of steel is much more than that of rubber. For the same longitudinal strain, which one will have greater tensile stress?

Tensile stress = Young’s modulus x longitudinal strain.
Therefore, steel will have greater tensile stress.

What are ductile materials?

The materials whose plastic range is comparatively large.