What Is the Equilibrium Constant?
The equilibrium constant of a chemical reaction (usually denoted by the symbol K) provides insight into the relationship between the products and reactants when a chemical reaction reaches equilibrium. For example, the equilibrium constant of concentration (denoted by Kc) of a chemical reaction at equilibrium can be defined as the ratio of the concentration of products to the concentration of the reactants, each raised to their respective stoichiometric coefficients. It is important to note that there are different types of equilibrium constants that provide relationships between the products and the reactants of equilibrium reactions in terms of different units.
Download Complete Chapter Notes of Equilibrium
Download Now
For a chemical reaction, the equilibrium constant can be defined as the ratio between the amount of reactant and the amount of product which is used to determine chemical behaviour.
At equilibrium, the rate of the forward reaction = rate of the backward reaction
i.e., rf = rb Or, kf × α × [A]a[B]b = kb × α × [C]c [D]d
At a particular temperature, the rate constants are constant. The ratio of the rate constant of forward reaction to the rate constant of backward reaction should be a constant and is called an equilibrium constant (Kequ).
Table of Contents
- Formula
- Units
- △G, Qc and Kc
- kc vs kp
- Characteristics
- Applications
- Equilibrium Concentration
- Factors Affecting Equilibrium Constant
Equilibrium Constant Formula
Kequ = kf/kb = [C]c [D]d/[A]a [B]b = Kc
Where Kc indicates the equilibrium constant measured in moles per litre.
For reactions involving gases: The equilibrium constant formula, in terms of partial pressure, will be:
Kequ = kf/kb = [[pC]c [pD]d]/[[pA]a [pB]b] = Kp
Where Kp indicates the equilibrium constant formula in terms of partial pressures.
- Larger Kc/Kp values indicate higher product formation and higher percentage conversion.
- Lower Kc/Kp values indicate lower product formation and lower percentage conversion.
- Medium Kc/Kp values indicate optimum product formation.
Units of Equilibrium Constant
The equilibrium constant is the ratio of the concentrations raised to the stoichiometric coefficients. Therefore, the unit of the equilibrium constant = [Mole L-1]△n.
Where, ∆n = sum of stoichiometric coefficients of products – sum of stoichiometric coefficients of reactants.
⇒ Also Read:
Equilibrium Constant, Reaction Quotient and Gibbs Free Energy
K is the ratio of the relative amount of products to reactants at equilibrium, while Q is the ratio at any point in time of the reaction. The Q value can be compared to K to determine the direction of the reaction to take place. The spontaneity of the process is related to the free energy change. △G (Gibbs Free Energy), K (Equilibrium Constant), and Q (Reaction Quotient) are related, as detailed below:
- △G < 0 and Qc ˂ Kc or Kp at the start of the reaction: The reaction will proceed to form products.
- △G = 0 and Qc = Kc or Kp at equilibrium, and no more changes in the concentration of the mixture.
- △G > 0 and Qc > Kc or Kp after equilibrium: The reaction will proceed in the direction to form reactants.
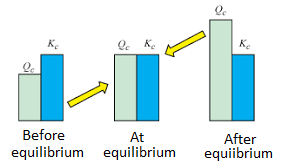
Equilibrium Constant vs Reaction Quotient
- Kc = Equilibrium constant measured in moles per litre.
- Kp = Equilibrium constant calculated from the partial pressures
Relationship between kc and kp
Consider the following reversible reaction: cC + dD ⇒ aA + bB
The equilibrium constant for the reaction is expressed in terms of the concentration (mole/litre):
If the equilibrium involves gaseous species, then the concentrations are replaced by partial pressures of the gaseous substances. The equilibrium constant in terms of partial pressures is:
Where pA, pB, pC and pD represent the partial pressures of the substance A, B, C and D, respectively. If gases are assumed to be ideal, then according to the ideal gas equation:
pV = nRT or p = nRT/V
Where,
- p is the pressure in Pa
- n is the number of moles of gas
- V is the volume in m3
- T is the temperature in Kelvin
- n/V = molar concentration = [C]
⇒ Check: Ideal Gas Equation
If C is in mol dm3 and p is in bar, then R = 0.0831 bar dm3 mol-1 K-1 or p = CRT
Substituting for pressure, in terms of concentration:
pA = [A] RT; pB = [B] RT; pC = [C] RT and pD = [D] RT
Substituting these values in expression for Kp:
Where, △n = (c+d) – (a+b), i.e. number of moles of gaseous products – number of moles of gaseous reactants in the balanced chemical reaction.
Characteristics of Equilibrium Constant
- It is reaction specific, and at a constant temperature, it is fixed.
- A catalyst changes the rate of forward and backward reactions equally, not to affect the value of the equilibrium constant.
- Changes in concentration, pressure, temperature, and inert gases may affect the equilibrium, favouring either forward or backward reaction but not the equilibrium constant.
- It is related to the standard free energy as, △G0 = -RT ln Kequ.
- For the same reversible reaction, Kequ has different values at different temperatures.
- The equilibrium constant of the reverse equilibrium is the reciprocal of the original equilibrium, i.e. Krev = 1/Kequ.
- If the equilibrium reaction stoichiometry is changed, the power of the equilibrium constant also gets changed by the same quantity.
- If the equilibrium constant for the reaction, A + B ⇌ C + D, is K, then, for equilibrium reaction 3A = 3B ⇒ 3C + 3D is K3.
- In case the stepwise multiple equilibria lead to the final products, the equilibrium constant of the net equilibrium = product of each stepwise equilibrium constant. Therefore, the net equilibrium constant K = K1 × K2 × K3.
- Simultaneous equilibrium reactions have a common product. The equilibrium constant of the reactions does not change. Due to the higher concentration of the common product, the product concentrations will be reduced.
Applications of Equilibrium Constant
Equilibrium Constant for Predicting the Extent of Reaction
The equilibrium constant (Kc) can be used to predict the extent of a reaction, i.e. the degree of the disappearance of the reactants. The magnitude of the equilibrium constant gives an idea of the relative amount of the reactants and the products.
Case 1: The larger value of the equilibrium constant (>103) shows that forward reaction is favoured, i.e. the concentration of products is much larger than that of the reactants at equilibrium.
For example,
- H2(g) + Br2(g)⇌ 2HBr(g) ⇒ Kc = 5.4×1018
- H2(g) + Cl2(g)⇌ 2HCl(g) ⇒ Kc = 4×1031
- H2(g) + 12O2(g) ⇌ H2O(g) ⇒ Kc = 2.4×1047
This shows that at equilibrium, the concentration of the products is very high, i.e. reaction goes almost to completion.
Case 2: Intermediate value of the equilibrium constant (10-3 to 103) show that the concentration of the reactants and products are comparable.
For example,
- Fe3 (aq) + SCN (aq) ⇌ [Fe(SCN)]2 (aq) ⇒ Kc = 138 at 298 K
- H2 (g) + I2 (g) ⇌ 2HI (g) ⇒ Kc = 57 at 700 K.
Case 3: Low value of equilibrium constant (<10-3) shows that backward reaction is favoured, i.e. concentration of reactants is much larger than that of products, i.e. the reaction proceeds to a very small extent in the forward direction.
For example,
- N2 (g) + O2 (g) ⇌ 2NO (g) ⇒ Kc =4.8 × 10-31 at 298K
- H2O (g) ⇌ H2 (g) + (1/2) O2 (g) ⇒ Kc = 4.1 × 10-48
Equilibrium Constant for Predicting the Direction of a Reaction
The equilibrium constant can be used to predict the direction of the reaction. We need a term, reaction quotient (Qc expressed in terms of concentrations or Qp in terms of partial pressures) similar to the equilibrium constant, except that the conditions are not at equilibrium.
For a balanced reaction, aA + bB ⇌ cC + dD
The reaction quotient (Qc or Qp) is given as:
Qc = [C]c[D]d/[A]a[B]b
Qp = pcC × pdD / paA × pbB
Comparison with Kc and the direction of reaction:
- If Q = Kc, the reaction is in equilibrium [Where, Kc = equilibrium constant]
- If Q > Kc, Q tends to decrease so as to become equal to K, the reaction will proceed in the backward direction.
- If Q < Kc, Q tends to increase so as to become equal to K, the reaction will proceed in the forward direction.
Calculating the Equilibrium Concentration
1. From Equilibrium Constant:
The degree of dissociation of an equilibrium involving gas can be calculated by knowing the equilibrium constant and the concentration of the gaseous reactant/product.
For example, in the decomposition of carbonate, the number of moles of carbon dioxide can be calculated from the equilibrium constant by assuming an ideal gas behaviour.
CaCO3 ⇌ CaO + CO2 Kp = [pco2]
The decomposition of ammonia gas is given below:
| Decomposition of Ammonia Reaction: 2NH3 ⇌ N2 + 3H2 | |||
| For initial moles | 1 | 0 | 0 |
| Moles at equilibrium | 1−2α | α | 3α |
| For C moles at equilibrium | C(1−2α) | Cα | 3Cα |
Number of moles at equilibrium = C(1 – 2α) + Cα + 3Cα = C(1 + 2α)
Partial pressure of ammonia = C(1 – 2α) /C(1 + 2α) = [(1 – 2α)/(1+2α)] Pt (Pt is the total presure)
Partial pressure of nitrogen = Cα/C(1 + 2α) = [α/(1 + 2α)]Pt
Partial pressure of hydrogen = 3Cα/C(1 + 2α) = [3α/(1+2α)]Pt
Kp = [pN2][pH2]3/[pNH3]2
Kp = [[pN2][pH2]3]/[pNH3]2
= [{α/[1+2α]} Pt {3α/[1+2α]}3]/ [{1−2α/[1+2α]Pt}2]
=27α4 Pt2 /[[1+2α]2[1−2α]2]
= 27α4 Pt2 /1 – 4α2
By knowing Kp and the total pressure, the degree of dissociation of ammonia can be calculated.
2. From Vapour Density Measurements
Vapour Density and Number of Moles:
For ideal gases, pV =nRT = [ω/M] × RT
M = [ωRT]/VP = ρ [RT]/P = ρ[RTV]/RTn = ρ V/n = 2 × Vapour Density
Vapour density = ρ × V/2n = α × 1/n
At equilibrium, V and ρ are constant, and Vapour Density is α × 1/n
Vapour Density and Equilibrium:
Vapour density at start/vapour density at equilibrium = D/d = M/m = Moles at equilibrium/Moles at start.
M = initial molecular weight and m = molecular weight at equilibrium
For example,
| Reaction: PCl5 ⇌ PCl3 + Cl2 | |||
| For initial moles | C | 0 | 0 |
| Moles at equilibrium | C(1 – α) | Cα | Cα |
Number of moles at equilibrium = C (1 – α) + Cα + Cα = C (1 + α)
C (1 + α) C = D/d : 1 + α
α = D/d – 1
Knowing D and d, or M and m, α can be calculated.
Factors Affecting Equilibrium Constant
Some factors that affect the equilibrium constant are as follows:
- Change in concentration of any product or reactant.
- Change in the pressure of the system.
- Change in temperature of the system.
- Adding inert gas.
- Adding catalyst.

Comments