NCERT Exemplar Solutions for Class 7 Maths Chapter 5 Lines and Angles

NCERT Exemplar Solutions for Class 7 Maths Chapter 5 Lines and Angles is an essential study material as it offers a wide range of questions that test students’ understanding of concepts. Our expert faculty have answered these questions in order to assist them with their annual exam preparation to attain good marks in the subject. Students who wish to score good marks in Maths should practise NCERT Exemplar Solutions for Class 7 Maths.

Chapter 5 – Lines and Angles solutions are available for download in PDF, which provides answers to all the questions present in the NCERT Exemplar Class 7 Maths textbook. Now, let us have a look at the concepts explained in this chapter.

• Related angles
  • Complementary Angles
  • Supplementary Angles
  • Adjacent Angles
  • Linear Pair
  • Vertically Opposite Angles
• Pairs of Lines
  • Intersecting Lines
  • Transversal
  • Angles made by a Transversal
  • Transversal of Parallel Lines

Download the PDF of NCERT Exemplar Solutions for Class 7 Maths Chapter 5 Lines and Angles

Access Answers to Maths NCERT Exemplar Solutions for Class 7 Chapter 5 Lines and Angles

Exercise Page: 128

In questions 1 to 41, there are four options, out of which one is correct. Write the correct one.

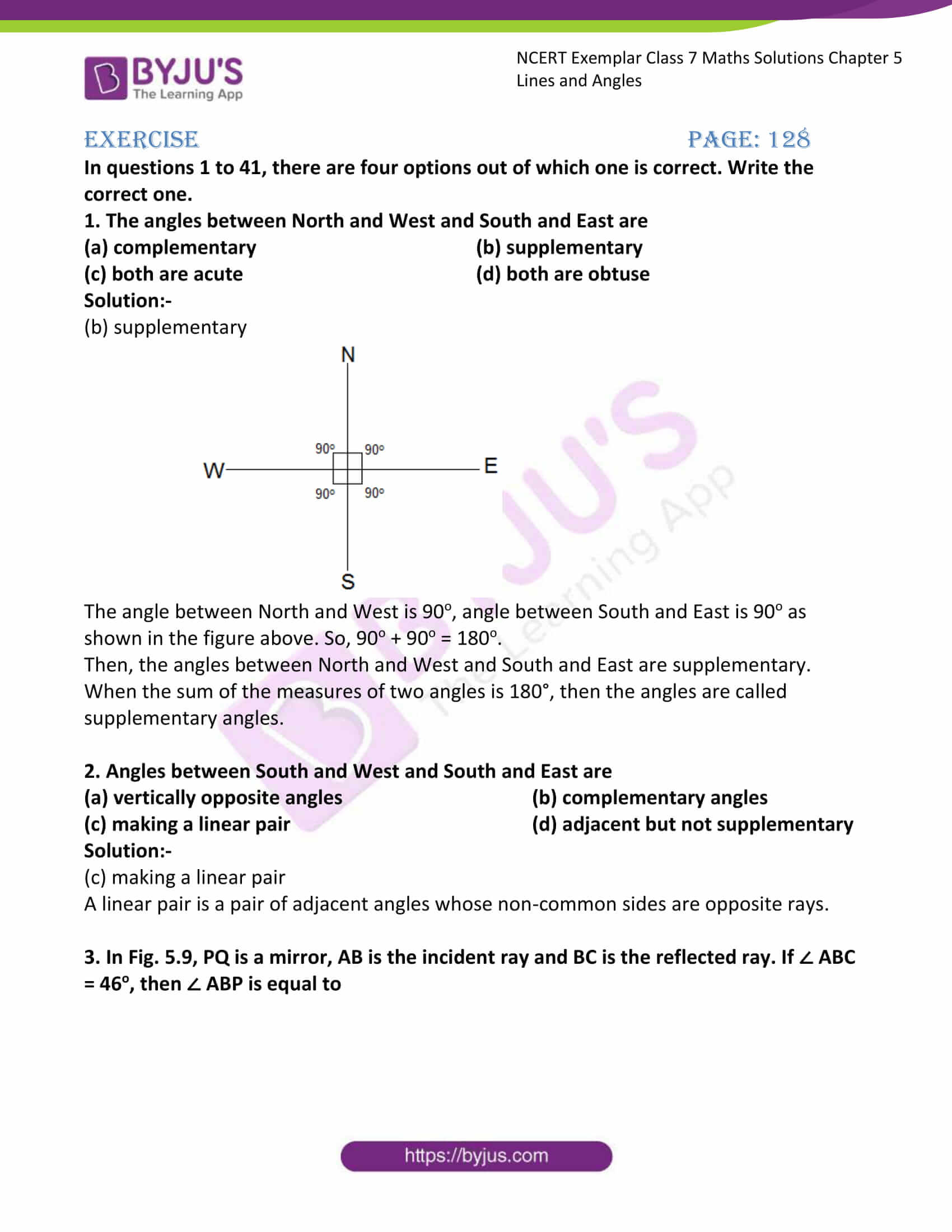
1. The angles between North and West and South and East are

(a) complementary (b) supplementary

(c) both are acute (d) both are obtuse

Solution:-

(b) supplementary

The angle between North and West is 90^o, the angle between South and East is 90^o, as shown in the figure above. So, 90^o + 90^o = 180^o.

Then, the angles between North and West and South and East are supplementary.

When the sum of the measures of two angles is 180°, then the angles are called supplementary angles.

2. Angles between South and West and South and East are

(a) vertically opposite angles (b) complementary angles

(c) making a linear pair (d) adjacent but not supplementary

Solution:-

(c) making a linear pair

A linear pair is a pair of adjacent angles whose non-common sides are opposite rays.

3. In Fig. 5.9, PQ is a mirror, AB is the incident ray and BC is the reflected ray. If ∠ ABC = 46^o, then ∠ ABP is equal to

(a) 44^o (b) 67^o (c) 13^o (d) 62^o

Solution:-

(b) 67^o

As we know that, the angle formed by the incident ray and angle formed by the reflected ray is equal.

From the given figure,

PQ is a straight line,

So, ∠ABP + ∠ABC + ∠CBQ = 180^o

Let us assume the ∠ABP = ∠CBQ = x

Then,

x + 46^o + x = 180^o

2x + 46^o = 180^o

2x = 180^o – 46^o

2x = 134^o

x = 134^o/2

x = 67^o

Therefore, the ∠ABP = ∠CBQ = 67^o

4. If the complement of an angle is 79^o, then the angle will be of

(a) 1^o (b) 11^o (c) 79^o (d) 101^o

Solution:-

(b) 11^o

When the sum of the measures of two angles is 90°, the angles are called complementary angles. Each of them is called complement of the other.

The given complement of an angle is 79^o

Let the measure of the angle be x^o.

Then,

x + 79^o = 90^o

x = 90^o – 79^o

x = 11^o

Hence, the measure of the angle is 11^o.

5. Angles which are both supplementary and vertically opposite are

(a) 95^o, 85^o (b) 90^o, 90^o (c) 100^o, 80^o (d) 45^o, 45^o

Solution:-

(b) 90^o, 90^o

When the sum of the measures of two angles is 180°, then the angles are called supplementary angles.

6. The angle which makes a linear pair with an angle of 61^o is of

(a) 29^o (b) 61^o (c) 122^o (d) 119^o

Solution:-

(d) 119^o

A linear pair is a pair of adjacent angles whose non-common sides are opposite rays.

We know that, measure of sum of adjacent angles is equal to 180^o.

Let the measure of other angle be x^o.

Then,

x + 61^o = 180^o

x = 180^o – 61^o

x = 119^o

7. The angles x and 90^o – x are

(a) supplementary (b) complementary

(c) vertically opposite (d) making a linear pair

Solution:-

(b) complementary

When the sum of the measures of two angles is 90^o, then the angles are called complementary angles.

x + 90^o – x = 90^o

90^o = 90^o

LHS = RHS

8. The angles x – 10^o and 190^o – x are

(a) interior angles on the same side of the transversal

(b) making a linear pair

(c) complementary

(d) supplementary

Solution:-

(d) supplementary

When the sum of the measures of two angles is 180^o, then the angles are called supplementary angles.

x – 10^o + 190^o – x = 180^o

190^o – 10 = 180^o

180^o = 180^o

LHS = RHS

9. In Fig. 5.10, the value of x is

(a) 110^o (b) 46^o (c) 64^o (d) 150^o

Solution:-

(d) 150^o

The sum of all angles about a point given in the figure is equal to 360^o.

Then, 100^o + 46^o + 64^o + x = 360^o

210^o + x = 360^o

x = 360^o – 210^o

x = 150^o

10. In Fig. 5.11, if AB || CD, ∠ APQ = 50^o and ∠PRD = 130^o, then ∠ QPR is

(a) 130^o (b) 50^o (c) 80^o (d) 30^o

Solution:-

(c) 80^o

We know that, ∠APR = ∠PRD … [because interior alternate angles]

∠APQ + ∠QPR = 130^o

50^o + ∠QPR = 130^o

∠QPR = 130^o – 50^o

∠QPR = 80^o

11. In Fig. 5.12, lines l and m intersect each other at a point. Which of the following is false?

(a) ∠a = ∠b (b) ∠d = ∠c

(c) ∠a + ∠d = 180^o (d) ∠a = ∠d

Solution:-

(d) ∠a = ∠d

∠a ≠ ∠d

∠a = ∠b [because vertically opposite angles]

∠d = ∠c [because vertically opposite angles]

∠a + ∠d = 180^o [Linear pair of angles]

12. If angle P and angle Q are supplementary and the measure of angle P is 60^o, then the measure of angle Q is

(a) 120^o (b) 60^o (c) 30^o (d) 20^o

Solution:-

(a) 120^o

When the sum of the measures of two angles is 180^o, then the angles are called supplementary angles.

P + Q = 180^o

60^o + Q = 180^o

Q = 180^o – 60^o

Q = 120^o

13. In Fig. 5.13, POR is a line. The value of a is

(a) 40^o (b) 45^o (c) 55^o (d) 60^o

Solution:-

(a) 40^o

We know that, when the sum of the measures of two angles is 180^o, then the angles are called supplementary angles.

(3a + 5)^o + (2a – 25)^o = 180^o

3a + 5 + 2a – 25 = 180^o

5a – 20 = 180^o

5a = 180^o + 20

5a = 200

a = 200/5

a = 40^o

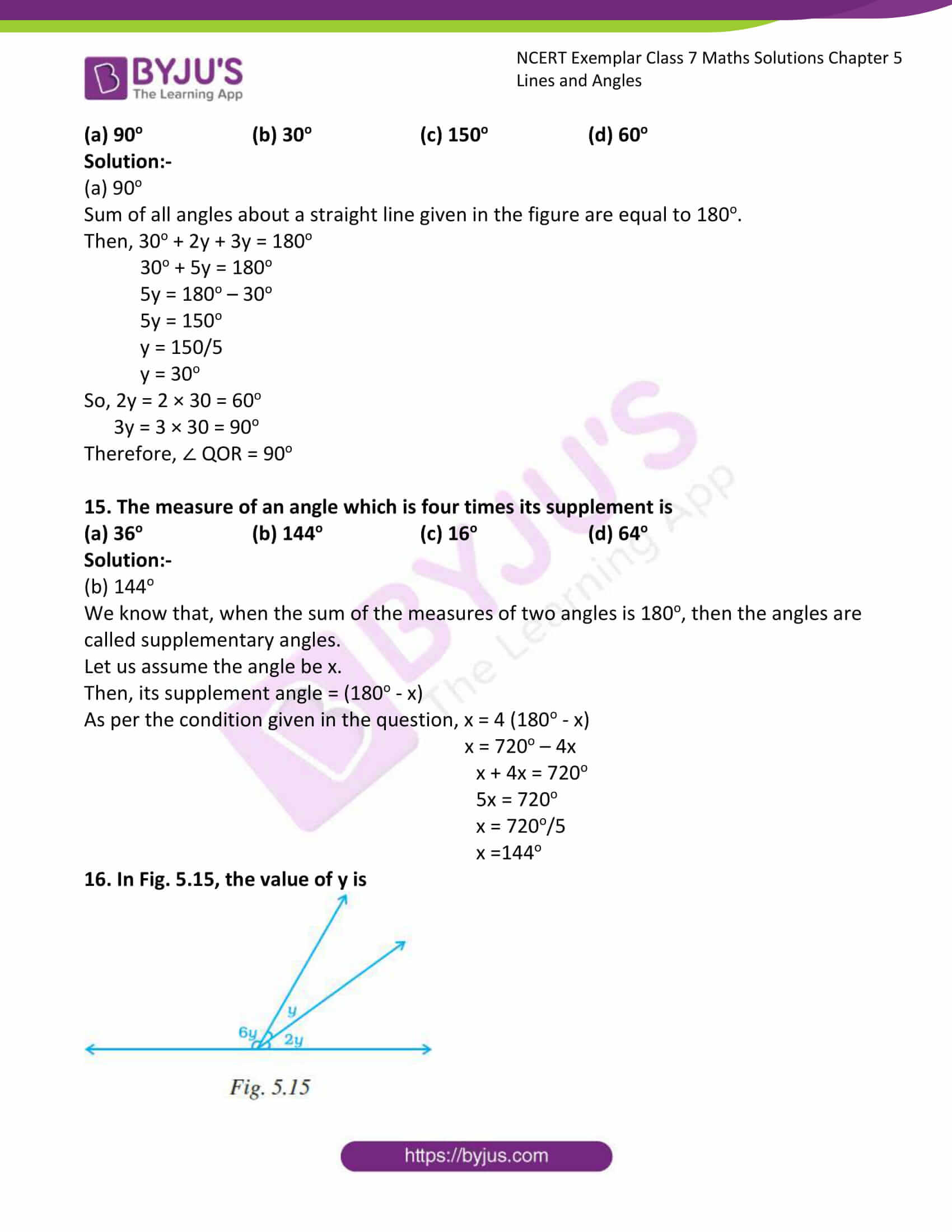
14. In Fig. 5.14, POQ is a line. If x = 30°, then ∠ QOR is

(a) 90^o (b) 30^o (c) 150^o (d) 60^o

Solution:-

(a) 90^o

Sum of all angles about a straight line given in the figure are equal to 180^o.

Then, 30^o + 2y + 3y = 180^o

30^o + 5y = 180^o

5y = 180^o – 30^o

5y = 150^o

y = 150/5

y = 30^o

So, 2y = 2 × 30 = 60^o

3y = 3 × 30 = 90^o

Therefore, ∠ QOR = 90^o

15. The measure of an angle which is four times its supplement is

(a) 36^o (b) 144^o (c) 16^o (d) 64^o

Solution:-

(b) 144^o

We know that, when the sum of the measures of two angles is 180^o, then the angles are called supplementary angles.

Let us assume the angle be x.

Then, its supplement angle = (180^o – x)

As per the condition given in the question, x = 4 (180^o – x)

x = 720^o – 4x

x + 4x = 720^o

5x = 720^o

x = 720^o/5

x =144^o

16. In Fig. 5.15, the value of y is

(a) 30^o (b) 15^o (c) 20^o (d) 22.5^o

Solution:-

(c) 20^o

The sum of all angles about a straight line given in the figure is equal to 180^o.

Then, 6y + y + 2y = 180^o

9y = 180^o

y = 180/9

y = 20^o

So, value of y is 20^o.

17. In Fig. 5.16, PA || BC || DT and AB || DC. Then, the values of a and b are respectively.

(a) 60^o, 120^o (b) 50^o,130^o (c) 70^o,110^o (d) 80^o,100^o

Solution:-

(b) 50^o,130^o

We know that, ∠PAB = ∠ABC = 50^o … [because interior alternate angles]

Given, AB || DC so consider it as parallelogram,

In a parallelogram, adjacent angles of the parallelogram are supplementary.

So, ∠ABC + ∠BCD = 180^o

50^o + ∠BCD = 180^o

∠BCD = 180^o – 50^o

∠BCD = 130^o

∠BCD = ∠CDT = 130^o  … [because interior alternate angles]

Therefore, a = 50^o and b = 130^o

18. The difference of two complementary angles is 30^o. Then, the angles are

(a) 60^o, 30^o (b) 70^o, 40^o (c) 20^o, 50^o (d) 105^o, 75^o

Solution:-

(a) 60^o, 30^o

When the sum of the measures of two angles is 90^o, then the angles are called complementary angles.

So, 60^o + 30^o = 90^o

As per the condition in the question, 60^o – 30^o = 30^o

19. In Fig. 5.17, PQ || SR and SP || RQ. Then, angles a and b are respectively

(a) 20^o, 50^o (b) 50^o, 20^o (c) 30^o, 50^o (d) 45^o, 35^o

Solution:-

(a) 20^o, 50^o

∠QRP = ∠RPS = 50^o  … [because interior alternate angles]

∠SRP = ∠RPQ = 20^o … [because interior alternate angles]

Therefore, angle a = 20^o and angle b = 50^o

20. In Fig. 5.18, a and b are

(a) alternate exterior angles

(b) corresponding angles

(c) alternate interior angles

(d) vertically opposite angles

Solution:-

(c) alternate interior angles

21. If two supplementary angles are in the ratio 1: 2, then the bigger angle is

(a) 120^o (b) 125^o (c) 110^o (d) 90^o

Solution:-

(a) 120^o

We know that, when the sum of the measures of two angles is 180^o, then the angles are called supplementary angles.

Let us assume two angles be 1x and 2x.

1x + 2x = 180^o

3x = 180^o

x = 180^o/3

x = 60^o

Then the bigger angle is 2x = 2 × 60^o = 120^o

22. In Fig. 5.19, ∠ROS is a right angle and ∠POR and ∠QOS are in the ratio 1: 5. Then, ∠QOS measures

(a) 150^o (b) 75^o (c) 45^o (d) 60^o

Solution:-

(b) 75^o

The sum of all angles about a straight line given in the figure is equal to 180^o.

Given, ∠ROS is a right angle = 90^o

Let us assume ∠POR = x and ∠QOS = 5x.

Then, ∠POR + ∠ROS + ∠QOS = 180^o

x + 90^o + 5x = 180^o

6x = 180^o – 90^o

6x = 90^o

x = 90^o/6

x = 15^o

So, ∠QOS measures = 5x = 5 × 15^o = 75^o

23. Statements a and b are as given below:

a: If two lines intersect, then the vertically opposite angles are equal.

b: If a transversal intersects two other lines, then the sum of two interior angles on the same side of the transversal is 180^o.

Then

(a) Both a and b are true (b) a is true and b is false

(c) a is false and b is true (d) both a and b are false

Solution:-

(b) a is true and b is false

24. For Fig. 5.20, statements p and q are given below:

p: a and b are forming a linear pair.

q: a and b are forming a pair of adjacent angles.

Then,

(a) both p and q are true

(b) p is true and q is false

(c) p is false and q is true

(d) both p and q are false

Solution:-

(a) both p and q are true

25. In Fig. 5.21, ∠AOC and ∠ BOC form a pair of

(a) vertically opposite angles

(b) complementary angles

(c) alternate interior angles

(d) supplementary angles

Solution:-

(d) supplementary angles

26. In Fig. 5.22, the value of a is

(a) 20^o (b) 15^o

(c) 5^o (d) 10^o

Solution:-

(d) 10^o

∠AOF = ∠COD = 90^o [because vertically opposite angles]

Sum of all angles about a straight line given in the figure are equal to 180^o.

Then, ∠BOC + ∠COD + ∠DOE = 180^o

40^o + 90^o + 5a = 180^o

130^o + 5a = 180^o

5a = 180^o – 130^o

5a = 50^o

a = 50/5

a = 10^o

27. In Fig. 5.23, if QP || SR, the value of a is

(a) 40^o (b) 30^o (c) 90^o (d) 80^o

Solution:-

(c) 90^o

To find out the value of ‘a’, draw a line XY, to cut at ‘a’.

So, XY || SR

∠XTS = ∠TSR = 30^o … [because interior alternate angles]

∠PQT = ∠QTX = 60^o … [because interior alternate angles]

Then, a = ∠XTS + ∠QTX

= 30^o + 60^o

= 90^o

28. In which of the following figures, a and b are forming a pair of adjacent angles?

Solution:-

In figure (d) a and b form a pair of adjacent angles.

29. In a pair of adjacent angles, (i) vertex is always common, (ii) one arm is always common, and (iii) uncommon arms are always opposite rays

Then

(a) All (i), (ii) and (iii) are true

(b) (iii) is false

(c) (i) is false but (ii) and (iii) are true

(d) (ii) is false

Solution:-

(b) (iii) is false

Two angles are called adjacent angles, if they have a common vertex and a common arm but no common interior points.

30. In Fig. 5.25, lines PQ and ST intersect at O. If ∠POR = 90° and x : y = 3 : 2, then z is equal to

(a) 126^o (b) 144^o (c) 136^o (d) 154^o

Solution:-

(b) 144^o

The sum of all angles about a straight line given in the figure is equal to 180^o.

PQ is a straight line.

Then, ∠POR + ∠ROT + ∠TOQ = 180^o

Given, x : y = 3 : 2

Let us assume x = 3a, y = 2a

90^o + 3a + 2a = 180^o

90^o + 5a = 180^o

5a = 180^o – 90^o

5a = 90^o

a = 90/5

a = 18^o

So, x = 3a = 3 × 18 = 54^o

y = 2a = 2 × 18 = 36^o

From the figure SOT is a straight line,

Then, z+ y = 180^o

z + 36^o = 180^o

z = 180^o – 36^o

z = 144^o

31. In Fig. 5.26, POQ is a line, then a is equal to

(a) 35^o (b) 100^o (c) 80^o (d) 135^o

Solution:-

(c) 80^o

From the figure POQ is a straight line,

Then, 100 + a = 180^o

a = 180^o – 100

a= 80^o

32. Vertically opposite angles are always

(a) supplementary (b) complementary

(c) adjacent (d) equal

Solution:-

(d) equal

33. In Fig. 5.27, a = 40^o. The value of b is

(a) 20^o (b) 24^o (c) 36^o (d) 120^o

Solution:-

(a) 20^o

Given, a = 40^o

Then, 2a = 2 × 40 = 80^o

From the figure, angles formed on the straight line are equal to 180^o,

Then, 5b + 2a = 180^o

5b + 80^o = 180^o

5b= 180^o – 80^o

5b = 100^o

b = 100/5

b = 20^o

34. If an angle is 60^o less than two times of its supplement, then the greater angle is

(a) 100^o (b) 80^o (c) 60^o (d) 120^o

Solution:-

(a) 100^o

Let us assume the angle be P.

Then, its supplement is 180^o – P

As per the condition in the question,

P = 2(180^o – P) – 60^o

P = 360^o – 2P – 60^o

P + 2P = 300^o

3P = 300^o

P = 300/3

P = 100^o

So, its supplement is 180^o – P = 180^o – 100^o = 80^o

Therefore, the greater angle is 100^o.

35. In Fig. 5.28, PQ || RS. If ∠1=(2a+b)^o and ∠6=(3a–b)^o, then the measure of ∠2 in terms of b is

(a) (2+b)^o (b) (3–b)^o (c) (108–b)^o (d) (180–b)^o

Solution: –

(c) (108–b)^o

From the question it is given that, ∠1 = (2a + b)^o and ∠6 = (3a – b)^o

Since ∠5 and ∠6 form a linear pair of angles

Then,

∠5 = (180-3a + b)^o … [equation 1]

∠5 = ∠1 = (180-3a + b)^o [Because Corresponding angles] …equation (2)

From equation (2) we get,

2a + b = 180-3a + b

5a = 180

a = 36⁰

Since ∠1 and ∠2 forms a linear pair so

∠2 = 180⁰– 2a-b

Substituting the value of a

∠2 = 180⁰– 72⁰ – b

∠2 = 108⁰– b

36. In Fig. 5.29, PQ||RS and a : b = 3 : 2. Then, f is equal to

(a) 36^o (b) 108^o (c) 72^o (d) 144^o

Solution: –

(b) 108^o

From the figure, PQ||RS.

From the question it is given that, a: b = 3: 2

So, let us assume a = 3m and b = 2m

We know that, sum of angles on the straight line is equal to 180^o

Then, ∠a + ∠b = 180^o

3m + 2m = 180^o

5m = 180^o

m = 180^o/5

m = 36^o

So, a = 3m = 3 × 36^o = 108^o

b = 2m = 2 × 36^o = 72

Therefore, ∠a = ∠f = 108^o [because corresponding angles]

37. In Fig. 5.30, line l intersects two parallel lines PQ and RS. Then, which one of the following is not true?

(a) ∠1 = ∠3 (b) ∠2 = ∠4

(c) ∠6 = ∠7 (d) ∠4 = ∠8

Solution:-

(d) ∠4 = ∠8

Because, ∠4 ≠ ∠8

38. In Fig. 5.30, which one of the following is not true?

(a) ∠1 + ∠5 = 180^o

(b) ∠2 + ∠5 = 180^o

(c) ∠3 + ∠8 = 180^o

(d) ∠2 + ∠3 = 180^o

Solution:-

(d) ∠2 + ∠3 = 180^o

We know that, interior opposite angles are equal

∠2 = ∠3

39. In Fig. 5.30, which of the following is true?

(a) ∠1 = ∠5 (b) ∠4 = ∠8 (c) ∠5 = ∠8 (d) ∠3 = ∠7

Solution:-

(c) ∠5 = ∠8

From the figure, PQ||RS

∠5 = ∠8 [interior alternate angles are equal]

40. In Fig. 5.31, PQ||ST. Then, the value of x + y is

(a) 125^o (b) 135^o (c) 145^o (d) 120^o

Solution: –

(b) 135^o

From the figure, PO is a straight line

We know that, sum of angles on the straight is equal to 180^o.

Then,

y + ∠ PQR = 180⁰

y + 130⁰ = 180⁰

y = 50^o

Then,

∠ QOS = ∠ TSO [Co-interior angle]

x = 85⁰

x + y = 135

41. In Fig. 5.32, if PQ||RS and QR||TS, then the value a is

(a) 95^o (b) 90^o (c) 85^o (d) 75^o

Solution:-

(a) 95^o

We know that, corresponding angles are equal

So,

∠ RQP = ∠ TSR = 85⁰ (Corresponding angles)

a + ∠ TSR = 180⁰

∠a = 95

In questions 42 to 56, fill in the blanks to make the statements true.

42. If sum of measures of two angles is 90^o, then the angles are _________.

Solution:-

If sum of measures of two angles is 90^o, then the angles are complementary.

43. If the sum of measures of two angles is 180^o, then they are _________.

Solution:-

If the sum of measures of two angles is 180^o, then they are supplementary.

44. A transversal intersects two or more than two lines at _________ points.

Solution:-

A transversal intersects two or more than two lines at distinct points.

If a transversal intersects two parallel lines, then (Q. 45 to 48).

45. The sum of interior angles on the same side of a transversal is 

Solution:-

The sum of interior angles on the same side of a transversal is 180^o.

46. Alternate interior angles have one common.

Solution:-

Alternate interior angles have one common arm.

47. Corresponding angles are on the side of the transversal.

Solution:-

Corresponding angles are on the same side of the transversal.

48. Alternate interior angles are on the side of the transversal.

Solution:-

Alternate interior angles are on the opposite side of the transversal

49. Two lines in a plane which do not meet at a point anywhere are called lines.

Solution:-

Two lines in a plane which do not meet at a point anywhere are called parallel lines.

50. Two angles forming a __________ pair are supplementary.

Solution:-

Two angles forming a linear pair are supplementary.

51. The supplement of an acute is always __________ angle.

Solution:-

The supplement of an acute is always an obtuse angle.

52. The supplement of a right angle is always _________ angle.

Solution:-

The supplement of a right angle is always right angle.

53. The supplement of an obtuse angle is always _________ angle.

Solution:-

The supplement of an obtuse angle is always an acute angle.

54. In a pair of complementary angles, each angle cannot be more than _________.

Solution:-

In a pair of complementary angles, each angle cannot be more than 90^o.

55. An angle is 45^o. Its complementary angle will be __________ .

Solution:-

An angle is 45^o. Its complementary angle will be 45^o.

56. An angle which is half of its supplement is of __________.

Solution:-

An angle which is half of its supplement is 60^o.

Let us assume the angle is p, and the supplement is 2p

p + 2p = 180⁰

3p = 180⁰

p = 60⁰