NCERT Solutions for Class 9 Maths Chapter 6 - Lines And Angles Exercise 6.3

Class 9 Maths introduces students to various new topics such as Euclid’s Geometry, Heron’s Formula, Surface Area and Volume and many more. So, it’s important that students should understand these new topics. Otherwise, they will start feeling that it’s a difficult subject and will not take an interest in it. Also, many times students byheart the steps of solving the questions in order to pass the exam as they haven’t understood the concepts. Thus, to help them, we have provided the NCERT Solutions step-by-step for Class 9 Maths Chapter 6 Lines and Angles – Exercise 6.3.

Students can go through these solutions to understand how the questions are being solved as per the latest syllabus. These NCERT Solutions of Class 9 Maths are provided by experienced teachers and contain step-by-step solutions, along with diagrams, wherever required. This will help students in their studies, and thus there is no need to byheart the Maths answers.

NCERT Solutions for Class 9 Maths Chapter 6 – Lines And Angles Exercise 6.3

Download PDF

Access Answers to NCERT Solutions for Class 9 Maths Chapter 6 – Lines And Angles Exercise 6.3

carouselExampleControls112

Previous



Next

Access other exercise Solutions of Class 9 Maths Chapter 6 – Lines and Angles

Students can have a look at the NCERT Solutions of the other exercises of this chapter by clicking the link below.

Exercise 6.1 Solutions 6 Questions (5 Short Answer Questions, 1 Long Answer Question)

Exercise 6.2 Solutions 6 Questions (3 Short Answer Questions, 3 Long Answer Questions)

Access answers to NCERT Solutions for Class 9 Maths Chapter 6 – Lines and Angles Exercise 6.3

1. In Fig. 6.39, sides QP and RQ of ΔPQR are produced to points S and T, respectively. If ∠SPR = 135° and ∠PQT = 110°, find ∠PRQ.

Solution:

It is given the TQR is a straight line, and so, the linear pairs (i.e. ∠TQP and ∠PQR) will add up to 180°

So, ∠TQP +∠PQR = 180°

Now, putting the value of ∠TQP = 110° we get,

∠PQR = 70°

Consider the ΔPQR,

Here, the side QP is extended to S, and so ∠SPR forms the exterior angle.

Thus, ∠SPR (∠SPR = 135°) is equal to the sum of interior opposite angles (Triangle property).

Or, ∠PQR +∠PRQ = 135°

Now, putting the value of ∠PQR = 70° we get,

∠PRQ = 135°-70°

Hence, ∠PRQ = 65°

2. In Fig. 6.40, ∠X = 62°, ∠XYZ = 54°. If YO and ZO are the bisectors of ∠XYZ and ∠XZY, respectively of Δ XYZ, find ∠OZY and ∠YOZ.

Solution:

We know the sum of the interior angles of the triangle.

So, ∠X +∠XYZ +∠XZY = 180°

Putting the values as given in the question, we get,

62°+54° +∠XZY = 180°

Or, ∠XZY = 64°

Now, we know that ZO is the bisector, so,

∠OZY = ½ ∠XZY

∴ ∠OZY = 32°

Similarly, YO is a bisector, and so,

∠OYZ = ½ ∠XYZ

Or, ∠OYZ = 27° (As ∠XYZ = 54°)

Now, as the sum of the interior angles of the triangle,

∠OZY +∠OYZ +∠O = 180°

Putting their respective values, we get,

∠O = 180°-32°-27°

Hence, ∠O = 121°

3. In Fig. 6.41, if AB || DE, ∠BAC = 35° and ∠CDE = 53°, find ∠DCE.

Solution:

We know that AE is a transversal since AB || DE

Here ∠BAC and ∠AED are alternate interior angles.

Hence, ∠BAC = ∠AED

It is given that ∠BAC = 35°

∠AED = 35°

Now, consider the triangle CDE. We know that the sum of the interior angles of a triangle is 180°.

∴ ∠DCE+∠CED+∠CDE = 180°

Putting the values, we get

∠DCE+35°+53° = 180°

Hence, ∠DCE = 92°

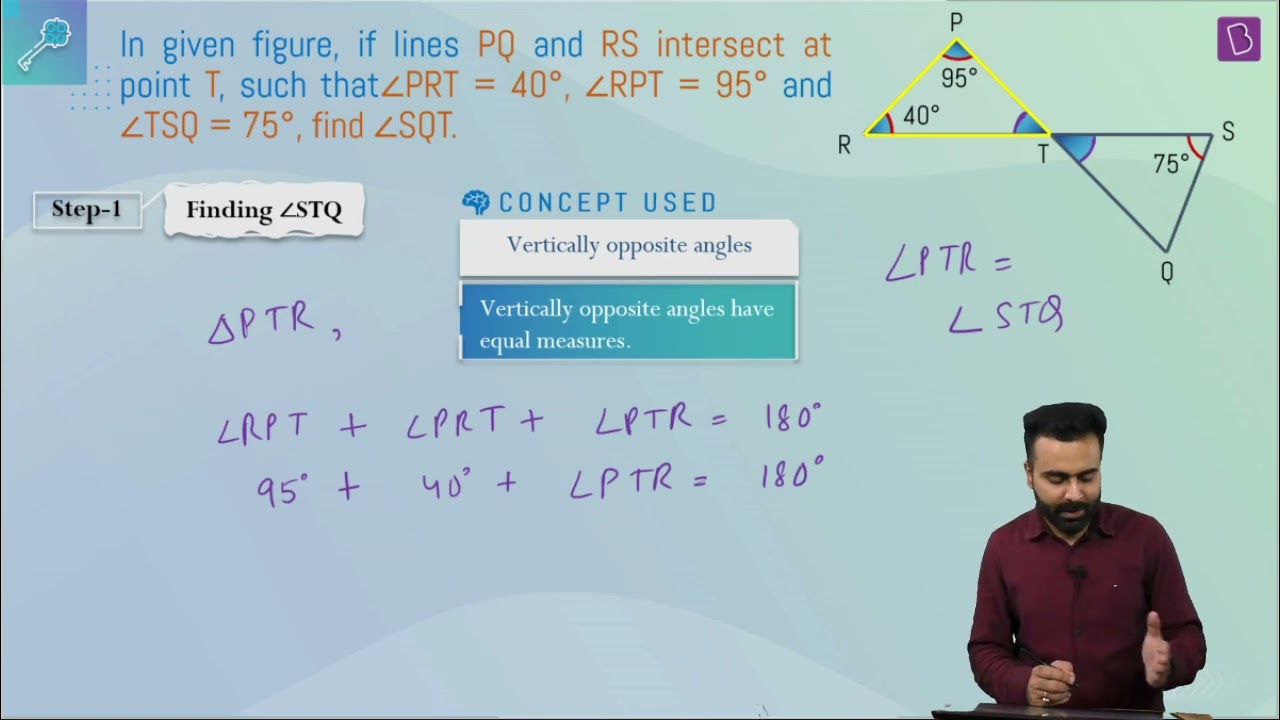
4. In Fig. 6.42, if lines PQ and RS intersect at point T, such that ∠PRT = 40°, ∠RPT = 95°, and ∠TSQ = 75°, find ∠SQT.

Solution:

Consider triangle PRT.

∠PRT +∠RPT + ∠PTR = 180°

So, ∠PTR = 45°

Now ∠PTR will be equal to ∠STQ as they are vertically opposite angles.

So, ∠PTR = ∠STQ = 45°

Again, in triangle STQ,

∠TSQ +∠PTR + ∠SQT = 180°

Solving this, we get,

74° + 45° + ∠SQT = 180°

∠SQT = 60°

5. In Fig. 6.43, if PQ ⊥ PS, PQ || SR, ∠SQR = 28° and ∠QRT = 65°, then find the values of x and y.

Solution:

x +∠SQR = ∠QRT (As they are alternate angles since QR is transversal)

So, x+28° = 65°

∴ x = 37°

It is also known that alternate interior angles are the same, and so,

∠QSR = x = 37°

Also, now,

∠QRS +∠QRT = 180° (As they are a Linear pair)

Or, ∠QRS+65° = 180°

So, ∠QRS = 115°

Using the angle sum property in Δ SPQ,

∠SPQ + x + y = 180°

Putting their respective values, we get,

90°+37° + y = 180°

y = 180⁰ – 127⁰ = 53⁰

Hence, y = 53°

6. In Fig. 6.44, the side QR of ΔPQR is produced to a point S. If the bisectors of ∠PQR and ∠PRS meet at point T, then prove that ∠QTR = ½ ∠QPR.

Solution:

Consider the ΔPQR. ∠PRS is the exterior angle, and ∠QPR and ∠PQR are the interior angles.

So, ∠PRS = ∠QPR+∠PQR (According to triangle property)

Or, ∠PRS -∠PQR = ∠QPR ———–(i)

Now, consider the ΔQRT,

∠TRS = ∠TQR+∠QTR

Or, ∠QTR = ∠TRS-∠TQR

We know that QT and RT bisect ∠PQR and ∠PRS, respectively.

So, ∠PRS = 2 ∠TRS and ∠PQR = 2∠TQR

Now, ∠QTR = ½ ∠PRS – ½∠PQR

Or, ∠QTR = ½ (∠PRS -∠PQR)

From (I), we know that ∠PRS -∠PQR = ∠QPR

So, ∠QTR = ½ ∠QPR (hence proved).

The application of Lines and Angles can be found on flooring, ceilings, farming, construction, surveying of property, engineering and many other areas. Here, in Exercise 6.3 of Chapter 6, Class 9 Maths, students will learn the “Angle Sum Property of a Triangle” and the Theorem associated with it. The angle sum property explains that the sum of all the interior angles of a triangle is 180^o. Also, there are some solved examples that are given in the NCERT textbook for students’ better understanding of this concept. After going through those examples, students can solve the exercise 6.3 questions. Try to solve it on their own first. In case they get stuck somewhere, then go through the NCERT Solutions for Class 9 Maths Chapter 6 Lines and Angles Exercise 6.3 PDF.

We hope this information on “NCERT Solution for Class 9 Maths Chapter 6 Lines and Angles Exercise 6.3” is useful for students. Stay tuned for further updates on CBSE and other competitive exams. For more interactive Maths and Science videos, download the BYJU’S App and subscribe to YouTube Channel.