NCERT Solutions Class 9 Science Chapter 11 – CBSE Free PDF Download
*According to the CBSE Syllabus 2023-24, this chapter has been renumbered as Chapter 10.
NCERT Solutions for Class 9 Science Chapter 11 Work and Energy help you lay a good foundation for your CBSE exam preparation. Students who refer to NCERT Solutions regularly are benefited from the comprehensive methodology and the detailed step-by-step procedure, which will fetch them good marks in their board examinations.
Download Exclusively Curated Chapter Notes for Class 9 Science Chapter – 11 Work and Energy
Download Most Important Questions for Class 9 Science Chapter – 11 Work and Energy
NCERT Solutions for Class 9 Science Chapter 11: Work and Energy help students to practise and gain more confidence in their preparations. All the NCERT Class 9 solutions are thoroughly prepared by our team of subject experts, and it includes objectives, diagram-based explanations, and short and long-type questions, which can be useful for both CBSE exams and other competitive exams.
NCERT Solutions for Class 9 Science Chapter 11: Work and Energy
Access Answers of Science NCERT Class 9 Chapter 11: Work and Energy (All intext and exercise questions solved)
Exercise-11.1 Page: 148
1. A force of 7 N acts on an object. The displacement is, say 8 m, in the direction of the force. Let us take it that the force acts on the object through the displacement. What is the work done in this case?
Solution:
When a force F acts on an object to move it in its direction through a distance S, work is done.
The work on the body is done by force.
Work done = Force × Displacement
W = F × S
Where,
F = 7 N S = 8 m
So, work done,
W = 7 × 8
W = 56 Nm
W = 56 J
Exercise-11.2 Page: 149
1. When do we say that work is done?
Solution:
Work is completed whenever the given conditions are satisfied:
(i) A force acts on the body.
(ii) There’s a displacement of the body by applying force in or opposite to the direction of the force.
2. Write an expression for the work done when a force is acting on an object in the direction of its displacement.
Solution:
When a force F displaces a body through a distance S within the direction of the applied force, then the work done W on the body is given by the expression:
W = F × S
3. Define 1 J of work.
Solution:
1 J is the amount of work done on an object when a force of 1 N displaces it by 1 m along the line of action of the force.
4. A pair of bullocks exerts a force of 140 N on a plough. The field being ploughed is 15 m long.
How much work is done in ploughing the length of the field?
Solution:
Work done by the bullocks is given by the expression:
W= F × d
Where,
Applied force, F = 140 N
Displacement, d = 15 m
W = 140 x 15 = 2100 J
Therefore, 2100 J of work is done in ploughing the length of the field.
Exercise-11.3 Page: 152
1. What is the kinetic energy of an object?
Solution:
The energy possessed by a body by virtue of its motion is termed mechanical energy or kinetic energy. Every moving object possesses mechanical energy. A body uses mechanical energy to try to work. The kinetic energy of the hammer is employed in driving a nail into a log of wood, the mechanical energy of air is employed to run wind mills, etc.
2. Write an expression for the kinetic energy of an object.
Solution:
If a body of mass m is moving with a speed v, then its K.E. Ek is given by the expression,
Ek = 1/2 m v2
Its SI unit is Joule (J).
3. The kinetic energy of an object of mass, m moving with a velocity of 5 ms-1 is 25 J. What will be its kinetic energy when its velocity is doubled? What will be its kinetic energy when its velocity is increased three times?
Solution:
Given
K.E. of the object = 25J
Velocity of the object (v) = 5 m/s
K.E. = (½) mv2
25 = (½) m (5)2
50 = 25 x m
m = 50/25
m = 2 kg
Now, when velocity is doubled
v = 10 m/s
m = 2 kg
K.E. = (½) x 2 x (10)2
K.E. = 102
K.E. = 100 J
When velocity is increased three times, then
v = 15 m/s
m = 2 kg
K.E. = (½) x 2 x (15)2
K.E. = (15)2
K.E. = 225 J
Exercise-11.4 Page: 156
1. What is power?
Solution:
Power is defined as the rate of doing work or the rate of transfer of energy. If an agent does a work W in time t, then power is given by:

P = W/T
It is expressed in watt (W).
2. Define 1 watt of power.
Solution:
A body is claimed to possess power of one watt if it works at the speed of 1 joule in 1 s.
That is,
One W = 1 J/1 S
3. A lamp consumes 1000 J of electrical energy in 10 s. What is its power?
Solution:
Power = Work/Time
P = W/T
Time = 10 s
Work done = Energy consumed by the lamp = 1000 J
Power = 1000/10 = 100 Js-1 =100 W
Hence, the power of the lamp is 100 W
4. Define average power.
Solution:
Average power is defined as the ratio of total work done by the body to the total time taken by the body.

Exercises – 11.5 Page: 158
1. Look at the activities listed below. Reason out whether or not work is done in the light of your understanding of the term ‘work’.
(a) Suma is swimming in a pond.
(b)A donkey is carrying a load on its back.
(c) A wind-mill is lifting water from a well.
(d) A green plant is carrying out photosynthesis.
(e) An engine is pulling a train.
(f) Food grains are getting dried in the sun.
(g) A sailboat is moving due to wind energy.
Solution:
Work is finished whenever the given 2 conditions are satisfied:
(i) A force acts on the body.
(ii) There’s a displacement of the body by applying force in or opposite to the direction of the force.
(a) While swimming, Suma applies a force to push the water backwards. Therefore, Suma swims in the forward direction caused by the forward reaction of water. Here, the force causes a displacement. Hence, the work is done by Seema while swimming.
(b) While carrying a load, the donkey has to apply a force in the upward direction. But, displacement of the load is in the forward direction. Since displacement is perpendicular to force, the work done is zero.
(c) A windmill works against gravity to elevate water. The windmill lift water by applying a force in an upward direction, and thus the water is moving in the same upward direction itself. Hence, work is done by the windmill to lift water from the well.
(d) No force is required when a green plant is carrying out photosynthesis. The plant does not exert any force to move. Since there is no displacement or force. Hence, no work is done.
(e) When an engine is pulling a train, it is applying a force in the forward direction. So, it is moving in the forward direction. Since displacement and force are in the same direction. Hence, work is done by the engine.
(f) There is no force involved in the process of drying food grains in the sun and the grains do not move. Since there is no force or displacement. Hence, no work is done.
(g) When a sailboat is moving due to wind energy, it is applying force in the forward direction. So, it is moving in the forward direction. Since displacement and force are in the same direction. Hence, work is done.
2. An object thrown at a certain angle to the ground moves in a curved path and falls back to the ground. The initial and the final points of the path of the object lie on the same horizontal line. What is the work done by the force of gravity on the object?
Solution:
Work done by the force of gravity on an object depends solely on vertical displacement. Vertical displacement is given by the distinction in the initial and final positions/heights of the object which is zero.
Work done by gravity is given by the expression,
W = m × g × h
Where,
h= Vertical displacement = 0
W = m g × 0 = 0 J
Hence, the work done by the gravity on the given object is zero joule.
3. A battery lights a bulb. Describe the energy changes involved in the process.
Solution:
When a bulb is connected to a battery, then the energy of the battery is transferred into voltage. Once the bulb receives this voltage, then it converts it into light and heat energy. Hence, the transformation of energy in the given situation can be shown as:
Chemical Energy → Electrical Energy → Light Energy + Heat Energy.
4. Certain force acting on a 20 kg mass changes its velocity from 5 m s-1 to 2 m s-1. Calculate the work done by the force.
Solution:
Given
Initial velocity u = 5 m/s
Mass of the body = 20kg
Final velocity v = 2 m/s
The initial kinetic energy
Ei = (1/2) mu2 = (1/2) × 20 × (5)2
= 10 × 25
= 250 J
Final kinetic energy
Ef = (1/2) mv2 = (1/2) × 20 × (2)2
= 10 × 4
= 40 J
Therefore,
Work done = Change in kinetic energy
Work done = Ef – Ei
Work done = 40 J – 250 J
Work done = -210 J
5. A mass of 10 kg is at a point A on a table. It is moved to a point B. If the line joining A and B is horizontal, what is the work done on the object by the gravitational force? Explain your answer.
Solution:
Work done by gravity depends solely on the vertical displacement of the body. It doesn’t rely on the trail of the body. Therefore, work done by gravity is given by the expression,
W= m g h
Where,
Vertical displacement, h = 0
∴ W= m × g × zero = 0
Therefore the work done on the object by gravity is zero.
6. The potential energy of a freely falling object decreases progressively. Does this violate the law of conservation of energy? Why?
Solution:
No, the method doesn’t violate the law of conservation of energy. This is because when the body falls from a height, its potential energy changes into kinetic energy progressively. A decrease in the potential energy is equal to an increase in the kinetic energy of the body. Throughout the method, the total mechanical energy of the body remains conserved. Therefore, the law of conservation of energy isn’t desecrated.
7. What are the various energy transformations that occur when you are riding a bicycle?
Solution:
During riding a bicycle, the muscular energy of the rider regenerates into heat and mechanical energy. Kinetic energy provides a rate to the bicycle, and warmth energy heats our body.
Muscular energy → mechanical energy + heat
8. Does the transfer of energy take place when you push a huge rock with all your might and fail to move it? Where is the energy you spend going?
Solution:
When we push a huge rock, there’s no transfer of muscular energy to the stationary rock. Also, there’s no loss of energy since muscular energy is transferred into heat energy, which causes our body to become hot.
9. A certain household has consumed 250 units of energy during a month. How much energy is this in joules?
Solution:
1 unit of energy = 1kWh
Given
Energy (E) = 250 units
1 unit = 1 kWh
1 kWh = 3.6 x 106 J
Therefore, 250 units of energy = 250 × 3.6 × 106
= 9 × 108 J.
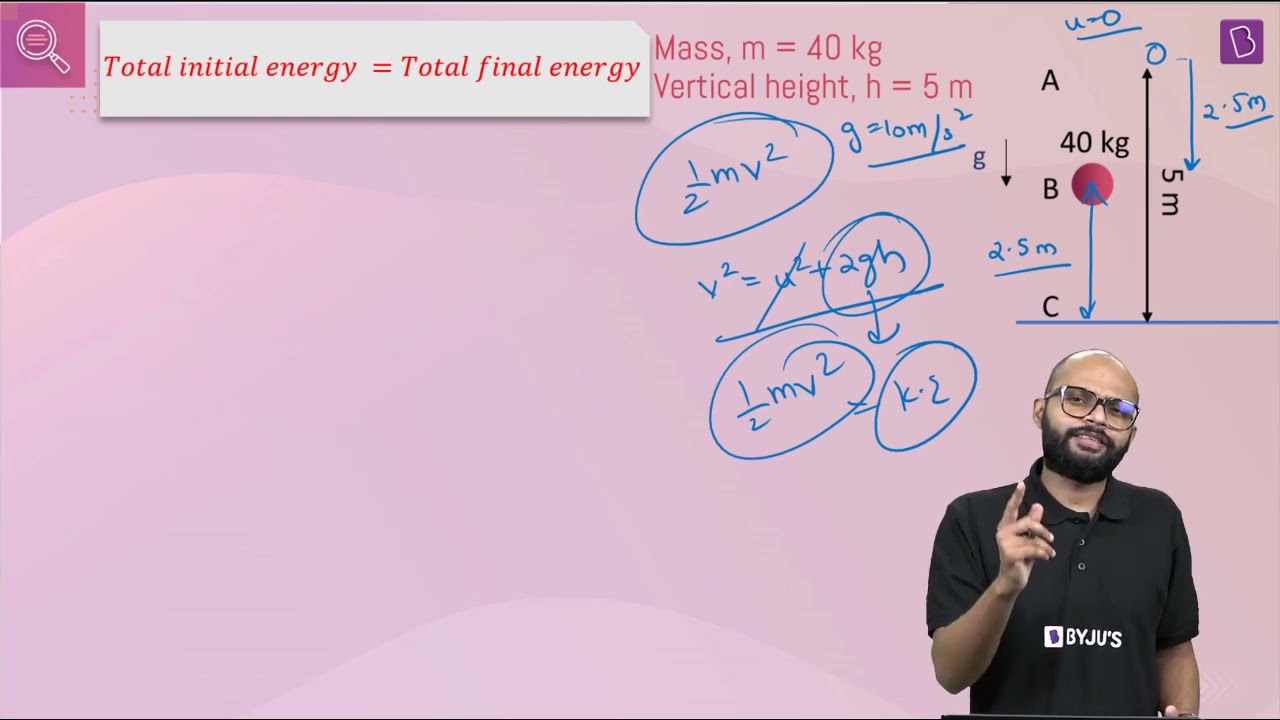
10. An object of mass 40 kg is raised to a height of 5 m above the ground. What is its potential energy? If the object is allowed to fall, find its kinetic energy when it is half-way down.
Solution:
Given Mass (m) = 40 kg
Acceleration due to gravity (g)= 10m/s²
Height (h)= 5m
Potential energy= m × g× h
P.E= 40 × 10 × 5 = 2000J
Potential energy = 2000J ( 2000 joules)
At a height of 5 metres, the object has a potential energy of 2000 J.
When this object is allowed to fall and it is halfway down, its height above the ground will be half of 5 m= 5/2= 2.5m.
P.E at Halfway down= m× g×h
P.E= 40× 10 × 2.5= 1000J
[h= 2.5 m]
Potential Energy halfway down= 1000 joules.
According to the law of conservation of energy:
Total potential energy= potential energy halfway down+ kinetic energy halfway down
2000 = 1000 + K.E halfway down
K.E at halfway down= 2000- 1000= 1000 J
Kinetic energy at halfway down= 1000 joules.
11. What is the work done by the force of gravity on a satellite moving round the earth? Justify your answer.
Solution:
Work is completed whenever the given two conditions are satisfied:
(i) A force acts on the body.
(ii) There’s a displacement of the body by applying force in or opposite to the direction of the force.
If the direction of force is perpendicular to displacement, then the work done is zero. When a satellite moves around the Earth, then the force of gravity on the satellite is perpendicular to its displacement. Therefore, the work done on the satellite by the Earth is zero.
12. Can there be displacement of an object in the absence of any force acting on it? Think. Discuss this question with your friends and teacher
Solution:
Yes, there can be displacement of an object in the absence of any force acting on it. If a single force acts on an object, the object accelerates. If an object accelerates, a force is acting on it.
Assume an object is moving with constant velocity. The net force acting on it is zero. But, there is a displacement along with the motion of the object. Therefore, there can be a displacement without a force.
13. A person holds a bundle of hay over his head for 30 minutes and gets tired. Has he done some work or not? Justify your answer.
Solution:
Work is completed whenever the given 2 conditions are satisfied.
(i) A force acts on the body.
(ii) There’s a displacement of the body by applying force in or opposite to the direction of the force.
When an individual holds a bundle of hay over his head, there is no displacement in the hay bundle. Although the force of gravity is acting on the bundle, the person isn’t applying any force on it. Therefore, in the absence of force, work done by the person on the bundle is zero.
14. An electric heater is rated 1500 W. How much energy does it use in 10 hours?
Solution:
Given,
Power of the heater = 1500 W = 1.5 kW
Time taken = 10 hours
Energy consumed by an electric heater can be obtained with the help of the expression,
Power = Energy consumed / Time taken
Hence,
Energy consumed = Power x Time taken
Energy consumed = 1.5 x 10
Energy consumed = 15 kWh
Therefore, the energy consumed by the heater in 10 hours is 15 kWh.
15. Illustrate the law of conservation of energy by discussing the energy changes which occur when we draw a pendulum bob to one side and allow it to oscillate. Why does the bob eventually come to rest? What happens to its energy eventually? Is it a violation of the law of conservation of energy?
Solution:
Consider the case of an oscillation pendulum.

When an apparatus moves from its mean position P to either of its extreme positions A or B, it rises through a height h on top of the mean level P. At this time, the K.E. of the bob changes fully into P.E. The K.E. becomes zero, and also, the bob possesses P.E. solely. Because it moves towards purpose P, its P.E. decreases increasingly. Consequently, the K.E. will increase. Because the bob reaches purpose P, its P.E. becomes zero, and also, the bob possesses K.E. solely. This method is perennial as long as the apparatus oscillates.
The bob doesn’t oscillate forever. It involves rest as a result of air resistance resisting its motion. The apparatus loses its K.E. to beat this friction and stops once in a while. The law of conservation of energy isn’t desecrated because the energy lost by the apparatus to beat friction is gained by its surroundings. Hence, the overall energy of the apparatus and also the encompassing system stay preserved.
16. An object of mass, m is moving with a constant velocity, v. How much work should be done on the object in order to bring the object to rest?
Solution:
The kinetic energy of an object of mass m, moving with a velocity, v, is given by the expression,
K.E = 1/2 mv2
In order to bring it to rest, its velocity has to be reduced to zero, and in order to accomplish that, the kinetic energy has to be drained off and sent somewhere else.
An external force has to absorb energy from the object, i.e. do negative work on it, equal to its kinetic energy, or
– 1/2 mv2.
17. Calculate the work required to be done to stop a car of 1500 kg moving at a velocity of 60 km/h.
Solution:
Given data:
The mass of the body = 1500kg
Velocity v = 60km/hr

The work required to stop the moving car = change in kinetic energy
18. In each of the following a force, F is acting on an object of mass, m. The direction of displacement is from west to east shown by the longer arrow. Observe the diagrams carefully and state whether the work done by the force is negative, positive or zero.

Solution:
Case I
In this case, the direction of force functioning on the block is perpendicular to the displacement. Therefore, work done by force on the block will be zero.
Case II
In this case, the direction of force functioning on the block is in the direction of displacement. Therefore, work done by force on the block will be positive.
Case III
In this case, the direction of force functioning on the block is contrary to the direction of displacement. Therefore, work done by force on the block will be negative.
19. Soni says that the acceleration in an object could be zero even when several forces are acting on it. Do you agree with her? Why?
Solution:
Acceleration in an object could be zero even when many forces work on it. This happens when all the forces get rid of one another, i.e., the online force working on the object is zero. For a uniformly moving object, the online force working on the it is zero. Hence, the acceleration of the thing is zero. Hence, Soni is correct.
20. Find the energy in kW h consumed in 10 hours by four devices of power 500 W each.
Solution:
Given,
Power rating of the device (P) = 500 W = 0.50 kW
Time for which the device runs (T) = 10 h
Energy consumed by an electric device can be obtained by the expression
Power = Energy consumed/Time taken
∴ Energy consumed = Power × Time
Energy consumed = 0.50 × 10
Energy consumed = 5 kWh
Thus, the energy consumed by four equal rating devices in 10 h will be
⇒ 4 × 5 kWh
= 20 kWh
21. A freely falling object eventually stops on reaching the ground. What happens to its kinetic energy?
Solution:
When an object falls freely towards the ground, its potential energy decreases, and kinetic energy increases; as the object touches the ground, all its potential energy becomes kinetic energy. Since the object hits the ground, all its kinetic energy becomes heat energy and sound energy. It can also deform the ground depending upon the ground’s nature and the amount of kinetic energy possessed by the object.
| Also Access |
| NCERT Exemplar Solutions for Class 9 Science Chapter 11 |
| CBSE Notes for Class 9 Science Chapter 11 |
NCERT Solutions for Class 9 Science Chapter 11: Work and Energy
Work and Energy is one of the important topics in the Class 9 Science curriculum, and the expected weightage is 27. Every student should practise these NCERT Solutions as there are more solved numericals which are repetitively asked in the exams. Apart from the solved examples, these solutions also include key notes and important terminologies from the exam point of view.
Topic covered in NCERT Solutions
- Work – 5 Questions (3 long, 2 short)
- Power -5 Questions (1 long, 4 short)
- Energy 1 Question (1 short)
- Unit of Energy 1 Question (1 MCQ)
- Forms of Energy 1 Question (1 MCQ)
- Kinetic Energy and its expression 1 Question (1 short)
- Potential Energy and its expression 1 Question (1 short)
- Conservation of Energy 1 Question (1 short)
NCERT Solutions for Class 9 Science Chapter 11: Work and Energy
Class 9 Chapter 11: Work and Energy is an important topic that provides a foundation for all your future studies. Work and Energy are closely related terms which are quite often used in our daily lives. Here, in this topic, students learn more in detail about work, power, force, energy, and how they are interrelated to each other. NCERT Solutions for Class 9 Science Chapter 11: Work and Energy include more solved problems and other daily basis examples, which help students to learn the topic effectively.
Key Features of NCERT Solutions for Class 9 Science Chapter 11: Work and Energy
- Provides detailed explanations for all complex topics.
- Provides completely solved solutions to all the questions in the Class 9 Science NCERT textbooks.
- These solutions include important questions at the end of every chapter
- The language used in NCERT Solutions for Class 9 Science is easy and simple to understand for the students.
- All these solutions are prepared by the subject experts after extensive research on every topic in order to provide appropriate and genuine information to the students.
Disclaimer:
Dropped Topics – 11.3.1 Commercial Unit of Energy.
Frequently Asked Questions on NCERT Solutions for Class 9 Science Chapter 11
What are the topics covered under Chapter 11 of NCERT Solutions for Class 9 Science?
Work – 5 Questions (3 long, 2 short)
Power – 5 Questions (1 long, 4 short)
Energy – 1 Question (short)
Unit of Energy – 1 Question (MCQ)
Forms of Energy – 1 Question (MCQ)
Kinetic Energy and its expression – 1 Question (short)
Potential Energy and its expression – 1 Question (short)
Conservation of Energy – 1 Question (short)














Good Solutions very nice keep it up 👍👍🤓👌👌