NCERT Solutions for Class 10 Maths Chapter 10 – Circles Exercise 10.1 gives students a detailed answer to the questions provided in the NCERT Class 10 Maths Chapter 10. Students are advised to study these exercise solutions to clear all their doubts about Circles. The NCERT solution covers basic topics on circles and numerical problems on a tangent to a circle. Solving these exercise questions, students can understand the theorem and can solve the question on a tangent to a circle easily.
Topics Covered in Exercise 10.1
- Introduction
- Tangent to a circle
- Solved examples
How Are They Helpful?
- NCERT Class 10 Maths Solutions make students understand the theorem thoroughly.
- Help them memorising the derivations and important formulas.
- Assists students in solving different varieties of questions.
NCERT Solutions for Class 10 Maths Chapter 10 Circles Exercise 10.1
Access Other Exercise Solutions of Class 10 Maths Chapter 10 – Circles
Students can access the other exercise wise solutions of NCERT Class 10 Maths Chapter 10 from the links below.
Exercise 10.1- 4 Questions (1 short answer question, 1 fill in the blanks question and 2 long answer questions)
Exercise 10.2- 13 Questions (10 long answer questions, 4 descriptive type questions and 2 short answer questions)
Access Answers to NCERT Class 10 Maths Chapter 10 – Circles Exercise 10.1
1. How many tangents can a circle have?
Answer:
There can be infinite tangents to a circle. A circle is made up of infinite points which are at an equal distance from a point. Since there are infinite points on the circumference of a circle, infinite tangents can be drawn from them.
2. Fill in the blanks.
(i) A tangent to a circle intersects it in …………… point(s).
(ii) A line intersecting a circle in two points is called a ………….
(iii) A circle can have …………… parallel tangents at the most.
(iv) The common point of a tangent to a circle and the circle is called …………
Answer:
(i) A tangent to a circle intersects it in one point(s).
(ii) A line intersecting a circle in two points is called a secant.
(iii) A circle can have two parallel tangents at the most.
(iv) The common point of a tangent to a circle and the circle is called the point of contact.
3. A tangent PQ at a point P of a circle of radius 5 cm meets a line through the centre O at
a point Q so that OQ = 12 cm. Length PQ is
(A) 12 cm
(B) 13 cm
(C) 8.5 cm
(D) √119 cm
Answer:
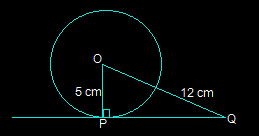
In the above figure, the line that is drawn from the centre of the given circle to the tangent PQ is perpendicular to PQ.
And so, OP ⊥ PQ
Using Pythagoras’ theorem in triangle ΔOPQ, we get
OQ2 = OP2+PQ2
(12)2 = 52+PQ2
PQ2 = 144-25
PQ2 = 119
PQ = √119 cm
So, option D, i.e., √119 cm, is the length of PQ.
4. Draw a circle and two lines parallel to a given line such that one is a tangent and the
other, a secant to the circle.
Answer:

In the above figure, XY and AB are two parallel lines. Line segment AB is the tangent at point C, while line segment XY is the secant.


Thankyou