RD Sharma Solutions for Class 7 Maths Exercise 14.1 of Chapter 14 Lines And Angles in PDF is available here. Students can refer and download it from the available links. This exercise has thirty-two questions. RD Sharma Solutions for Class 7 provide solutions for all topics covered in this chapter. Exercise 14.1 mainly deals with a pair of angles and their properties. Let us have a look at some of the important topics present in this exercise.
- Pairs of angles
- Definition and meaning of adjacent angles
- Definition and meaning of linear pair
- Vertically opposite angles
- Definition of angles at a point
- Concept of complementary angles
- Concept of supplementary angles
RD Sharma Solutions for Class 7 Maths Chapter 14 – Lines and Angles Exercise 14.1
Access answers to Maths RD Sharma Solutions For Class 7 Chapter 14 – Lines And Angles Exercise 14.1
Exercise 14.1 Page No: 14.6
1. Write down each pair of adjacent angles shown in fig. 13.

Solution:
The angles that have common vertex and a common arm are known as adjacent angles
Therefore the adjacent angles in given figure are:
∠DOC and ∠BOC
∠COB and ∠BOA
2. In Fig. 14, name all the pairs of adjacent angles.

Solution:
The angles that have common vertex and a common arm are known as adjacent angles.
In fig (i), the adjacent angles are
∠EBA and ∠ABC
∠ACB and ∠BCF
∠BAC and ∠CAD
In fig (ii), the adjacent angles are
∠BAD and ∠DAC
∠BDA and ∠CDA
3. In fig. 15, write down
(i) Each linear pair
(ii) Each pair of vertically opposite angles.

Solution:
(i) The two adjacent angles are said to form a linear pair of angles if their non – common arms are two opposite rays.
∠1 and ∠3
∠1 and ∠2
∠4 and ∠3
∠4 and ∠2
∠5 and ∠6
∠5 and ∠7
∠6 and ∠8
∠7 and ∠8
(ii) The two angles formed by two intersecting lines and have no common arms are called vertically opposite angles.
∠1 and ∠4
∠2 and ∠3
∠5 and ∠8
∠6 and ∠7
4. Are the angles 1 and 2 given in Fig. 16 adjacent angles?

Solution:
No, because they don’t have common vertex.
5. Find the complement of each of the following angles:
(i) 35o
(ii) 72o
(iii) 45o
(iv) 85o
Solution:
(i) The two angles are said to be complementary angles if the sum of those angles is 90o
Complementary angle for given angle is
90o – 35o = 55o
(ii) The two angles are said to be complementary angles if the sum of those angles is 90o
Complementary angle for given angle is
900 – 72o = 18o
(iii) The two angles are said to be complementary angles if the sum of those angles is 90o
Complementary angle for given angle is
90o – 45o = 45o
(iv) The two angles are said to be complementary angles if the sum of those angles is 90o
Complementary angle for given angle is
90o – 85o = 5o
6. Find the supplement of each of the following angles:
(i) 70o
(ii) 120o
(iii) 135o
(iv) 90o
Solution:
(i) The two angles are said to be supplementary angles if the sum of those angles is 180o
Therefore supplementary angle for the given angle is
180o – 70o = 110o
(ii) The two angles are said to be supplementary angles if the sum of those angles is 180o
Therefore supplementary angle for the given angle is
180o – 120o = 60o
(iii) The two angles are said to be supplementary angles if the sum of those angles is 180o
Therefore supplementary angle for the given angle is
180o – 135o = 45o
(iv) The two angles are said to be supplementary angles if the sum of those angles is 180o
Therefore supplementary angle for the given angle is
180o – 90o = 90o
7. Identify the complementary and supplementary pairs of angles from the following pairs:
(i) 25o, 65o
(ii) 120o, 60o
(iii) 63o, 27o
(iv) 100o, 80o
Solution:
(i) 25o + 65o = 90o so, this is a complementary pair of angle.
(ii) 120o + 60o = 180o so, this is a supplementary pair of angle.
(iii) 63o + 27o = 90o so, this is a complementary pair of angle.
(iv) 100o + 80o = 180o so, this is a supplementary pair of angle.
8. Can two obtuse angles be supplementary, if both of them be
(i) Obtuse?
(ii) Right?
(iii) Acute?
Solution:
(i) No, two obtuse angles cannot be supplementary
Because, the sum of two angles is greater than 90o so their sum will be greater than 180o
(ii) Yes, two right angles can be supplementary
Because, 90o + 90o = 180o
(iii) No, two acute angle cannot be supplementary
Because, the sum of two angles is less than 90o so their sum will also be less than 90o
9. Name the four pairs of supplementary angles shown in Fig.17.

Solution:
The two angles are said to be supplementary angles if the sum of those angles is 180o
The supplementary angles are
∠AOC and ∠COB
∠BOC and ∠DOB
∠BOD and ∠DOA
∠AOC and ∠DOA
10. In Fig. 18, A, B, C are collinear points and ∠DBA = ∠EBA.
(i) Name two linear pairs.
(ii) Name two pairs of supplementary angles.

Solution:
(i) Two adjacent angles are said to be form a linear pair of angles, if their non-common arms are two opposite rays.
Therefore linear pairs are
∠ABD and ∠DBC
∠ABE and ∠EBC
(ii) We know that every linear pair forms supplementary angles, these angles are
∠ABD and ∠DBC
∠ABE and ∠EBC
11. If two supplementary angles have equal measure, what is the measure of each angle?
Solution:
Let p and q be the two supplementary angles that are equal
The two angles are said to be supplementary angles if the sum of those angles is 180o
∠p = ∠q
So,
∠p + ∠q = 180o
∠p + ∠p = 180o
2∠p = 180o
∠p = 180o/2
∠p = 90o
Therefore, ∠p = ∠q = 90o
12. If the complement of an angle is 28o, then find the supplement of the angle.
Solution:
Given complement of an angle is 28o
Here, let x be the complement of the given angle 28o
Therefore, ∠x + 28o = 90o
∠x = 90o – 28o
= 62o
So, the supplement of the angle = 180o – 62o
= 118o
13. In Fig. 19, name each linear pair and each pair of vertically opposite angles:

Solution:
Two adjacent angles are said to be linear pair of angles, if their non-common arms are two opposite rays.
Therefore linear pairs are listed below:
∠1 and ∠2
∠2 and ∠3
∠3 and ∠4
∠1 and ∠4
∠5 and ∠6
∠6 and ∠7
∠7 and ∠8
∠8 and ∠5
∠9 and ∠10
∠10 and ∠11
∠11 and ∠12
∠12 and ∠9
The two angles are said to be vertically opposite angles if the two intersecting lines have no common arms.
Therefore supplement of the angle are listed below:
∠1 and ∠3
∠4 and ∠2
∠5 and ∠7
∠6 and ∠8
∠9 and ∠11
∠10 and ∠12
14. In Fig. 20, OE is the bisector of ∠BOD. If ∠1 = 70o, find the magnitude of ∠2, ∠3 and ∠4.

Solution:
Given, ∠1 = 70o
∠3 = 2(∠1)
= 2(70o)
∠3 = 140o
∠3 = ∠4
As, OE is the angle bisector,
∠DOB = 2(∠1)
= 2(70o)
= 140o
∠DOB + ∠AOC + ∠COB +∠AOD = 360o [sum of the angle of circle = 360o]
140o + 140o + 2(∠COB) = 360o
Since, ∠COB = ∠AOD
2(∠COB) = 360o – 280o
2(∠COB) = 80o
∠COB = 80o/2
∠COB = 40o
Therefore, ∠COB = ∠AOD = 40o
The angles are, ∠1 = 70o, ∠2 = 40o, ∠3 = 140o and ∠4 = 40o
15. One of the angles forming a linear pair is a right angle. What can you say about its other angle?
Solution:
Given one of the angle of a linear pair is the right angle that is 90o
We know that linear pair angle is 180o
Therefore, the other angle is
180o – 90o = 90o
16. One of the angles forming a linear pair is an obtuse angle. What kind of angle is the other?
Solution:
Given one of the angles of a linear pair is obtuse, then the other angle should be acute, because only then their sum will be 180o.
17. One of the angles forming a linear pair is an acute angle. What kind of angle is the other?
Solution:
Given one of the Angles of a linear pair is acute, then the other angle should be obtuse, only then their sum will be 180o.
18. Can two acute angles form a linear pair?
Solution:
No, two acute angles cannot form a linear pair because their sum is always less than 180o.
19. If the supplement of an angle is 65o, then find its complement.
Solution:
Let x be the required angle
So, x + 65o = 180o
x = 180o – 65o
x = 115o
The two angles are said to be complementary angles if the sum of those angles is 90o here it is more than 90o therefore the complement of the angle cannot be determined.
20. Find the value of x in each of the following figures.

Solution:
(i) We know that ∠BOA + ∠BOC = 180o
[Linear pair: The two adjacent angles are said to form a linear pair of angles if their non–common arms are two opposite rays and sum of the angle is 180o]60o + xo = 180o
xo = 180o – 60o
xo = 120o
(ii) We know that ∠POQ + ∠QOR = 180o
[Linear pair: The two adjacent angles are said to form a linear pair of angles if their non–common arms are two opposite rays and sum of the angle is 180o]3xo + 2xo = 180o
5xo = 180o
xo = 180o/5
xo = 36o
(iii) We know that ∠LOP + ∠PON + ∠NOM = 180o
[Linear pair: The two adjacent angles are said to form a linear pair of angles if their non–common arms are two opposite rays and sum of the angle is 180o]Since, 35o + xo + 60o = 180o
xo = 180o – 35o – 60o
xo = 180o – 95o
xo = 85o
(iv) We know that ∠DOC + ∠DOE + ∠EOA + ∠AOB+ ∠BOC = 360o
83o + 92o + 47o + 75o + xo = 360o
xo + 297o = 360o
xo = 360o – 297o
xo = 63o
(v) We know that ∠ROS + ∠ROQ + ∠QOP + ∠POS = 360o
3xo + 2xo + xo + 2xo = 360o
8xo = 360o
xo = 360o/8
xo = 45o
(vi) Linear pair: The two adjacent angles are said to form a linear pair of angles if their non–common arms are two opposite rays and sum of the angle is 180o
Therefore 3xo = 105o
xo = 105o/3
xo = 35o
21. In Fig. 22, it being given that ∠1 = 65o, find all other angles.

Solution:
Given from the figure 22, ∠1 = ∠3 are the vertically opposite angles
Therefore, ∠3 = 65o
Here, ∠1 + ∠2 = 180° are the linear pair [The two adjacent angles are said to form a linear pair of angles if their non–common arms are two opposite rays and sum of the angle is 180o]
Therefore, ∠2 = 180o – 65o
= 115o
∠2 = ∠4 are the vertically opposite angles [from the figure]
Therefore, ∠2 = ∠4 = 115o
And ∠3 = 65o
22. In Fig. 23, OA and OB are opposite rays:
(i) If x = 25o, what is the value of y?
(ii) If y = 35o, what is the value of x?

Solution:
(i) ∠AOC + ∠BOC = 180o [The two adjacent angles are said to form a linear pair of angles if their non–common arms are two opposite rays and sum of the angle is 180o]
2y + 50 + 3x = 180o
3x + 2y = 175o
Given If x = 25o, then
3(25o) + 2y = 175o
75o + 2y = 175o
2y = 175o – 75o
2y = 100o
y = 100o/2
y = 50o
(ii) ∠AOC + ∠BOC = 180o [The two adjacent angles are said to form a linear pair of angles if their non–common arms are two opposite rays and sum of the angle is 180o]
2y + 5 + 3x = 180o
3x + 2y = 175o
Given If y = 35o, then
3x + 2(35o) = 175o
3x + 70o = 175o
3x = 1750 – 70o
3x = 105o
x = 105o/3
x = 35o
23. In Fig. 24, write all pairs of adjacent angles and all the liner pairs.

Solution:
Pairs of adjacent angles are:
∠DOA and ∠DOC
∠BOC and ∠COD
∠AOD and ∠BOD
∠AOC and ∠BOC
Linear pairs: [The two adjacent angles are said to form a linear pair of angles if their non–common arms are two opposite rays and sum of the angle is 180o]
∠AOD and ∠BOD
∠AOC and ∠BOC
24. In Fig. 25, find ∠x. Further find ∠BOC, ∠COD and ∠AOD.

Solution:
(x + 10)o + xo + (x + 20)o = 180o[linear pair]
On rearranging we get
3xo + 30o = 180o
3xo = 180o – 30o
3xo = 150o
xo = 150o/3
xo = 50o
Also given that
∠BOC = (x + 20)o
= (50 + 20)o
= 70o
∠COD = 50o
∠AOD = (x + 10)o
= (50 + 10)o
= 60o
25. How many pairs of adjacent angles are formed when two lines intersect in a point?
Solution:
If the two lines intersect at a point, then four adjacent pairs are formed and those are linear.
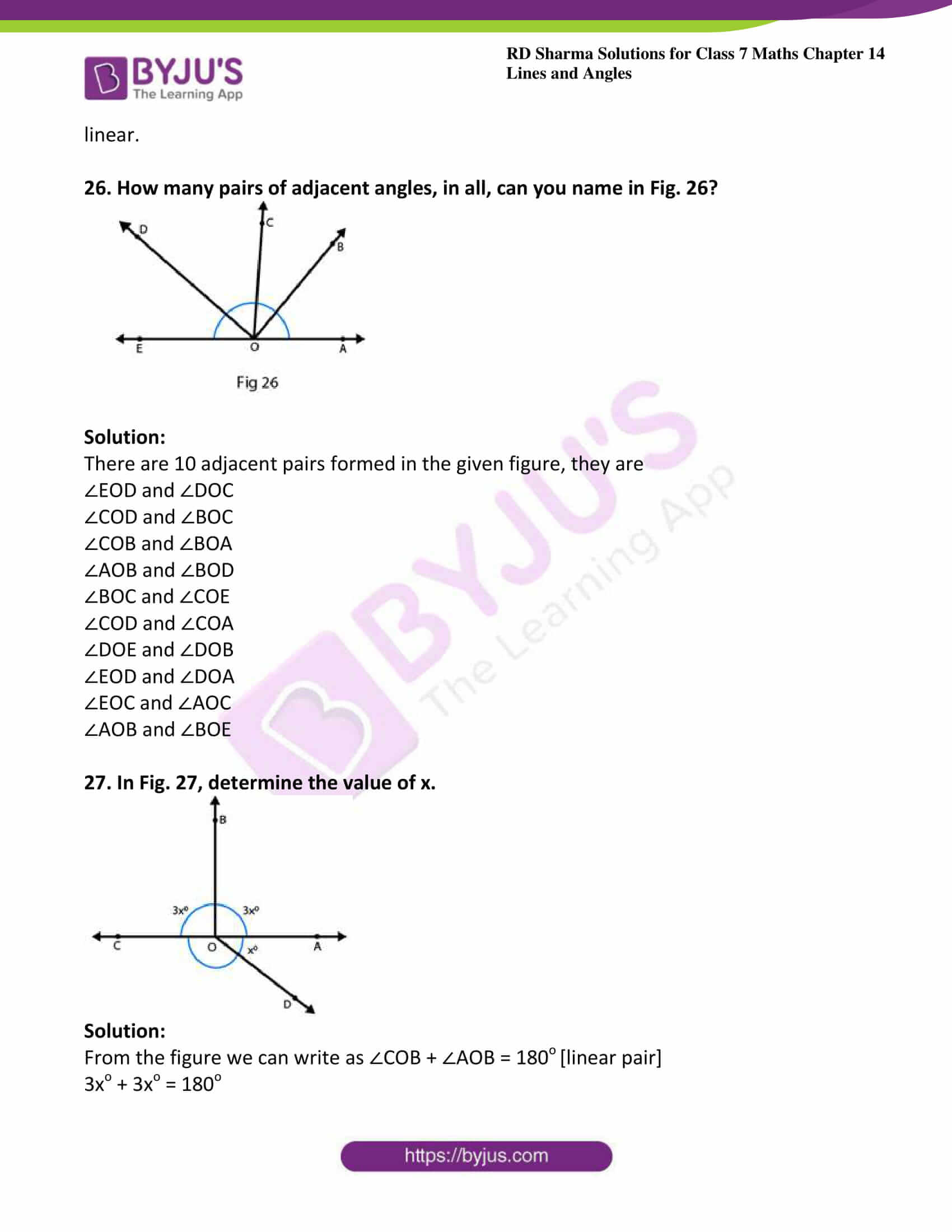
26. How many pairs of adjacent angles, in all, can you name in Fig. 26?

Solution:
There are 10 adjacent pairs formed in the given figure, they are
∠EOD and ∠DOC
∠COD and ∠BOC
∠COB and ∠BOA
∠AOB and ∠BOD
∠BOC and ∠COE
∠COD and ∠COA
∠DOE and ∠DOB
∠EOD and ∠DOA
∠EOC and ∠AOC
∠AOB and ∠BOE
27. In Fig. 27, determine the value of x.

Solution:
From the figure we can write as ∠COB + ∠AOB = 180o [linear pair]
3xo + 3xo = 180o
6xo = 180o
xo = 180o/6
xo = 30o
28. In Fig.28, AOC is a line, find x.

Solution:
From the figure we can write as
∠AOB + ∠BOC = 180o [linear pair]
Linear pair
2x + 70o = 180o
2x = 180o – 70o
2x = 110o
x = 110o/2
x = 55o
29. In Fig. 29, POS is a line, find x.

Solution:
From the figure we can write as angles of a straight line,
∠QOP + ∠QOR + ∠ROS = 180o
60o + 4x + 40o = 180o
On rearranging we get, 100o + 4x = 180o
4x = 180o – 100o
4x = 80o
x = 80o/4
x = 20o
30. In Fig. 30, lines l1 and l2 intersect at O, forming angles as shown in the figure. If x = 45o, find the values of y, z and u.

Solution:
Given that, ∠x = 45o
From the figure we can write as
∠x = ∠z = 45o
Also from the figure, we have
∠y = ∠u
From the property of linear pair we can write as
∠x + ∠y + ∠z + ∠u = 360o
45o + 45o + ∠y + ∠u = 360o
90o + ∠y + ∠u = 360o
∠y + ∠u = 360o – 90o
∠y + ∠u = 270o (vertically opposite angles ∠y = ∠u)
2∠y = 270o
∠y = 135o
Therefore, ∠y = ∠u = 135o
So, ∠x = 45o, ∠y = 135o, ∠z = 45o and ∠u = 135o
31. In Fig. 31, three coplanar lines intersect at a point O, forming angles as shown in the figure. Find the values of x, y, z and u

Solution:
Given that, ∠x + ∠y + ∠z+ ∠u + 50o + 90o = 360o
Linear pair, ∠x + 50o + 90o = 180o
∠x + 140o = 180o
On rearranging we get
∠x = 180o – 140o
∠x = 40o
From the figure we can write as
∠x = ∠u = 40o are vertically opposite angles
∠z = 90o is a vertically opposite angle
∠y = 50o is a vertically opposite angle
Therefore, ∠x = 40o, ∠y = 50o, ∠z = 90o and ∠u = 40o
32. In Fig. 32, find the values of x, y and z.

Solution:
∠y = 25o vertically opposite angle
From the figure we can write as
∠x = ∠z are vertically opposite angles
∠x + ∠y + ∠z + 25o = 360o
∠x + ∠z + 25o + 25o = 360o
On rearranging we get,
∠x + ∠z + 50o = 360o
∠x + ∠z = 360o – 50o [∠x = ∠z]
2∠x = 310o
∠x = 155o
And, ∠x = ∠z = 155o
Therefore, ∠x = 155o, ∠y = 25o and ∠z = 155o


















Very nice