RD Sharma Solutions for Class 10 Maths Chapter 6 Trigonometric Identities Exercise 6.1

In this exercise, students will be proving useful trigonometric identities using some basic trigonometric identities. The RD Sharma Solutions Class 10 is the most accurate resource for students to clarify doubts and prepare confidently for their exams. For detailed step-by-step solutions to exercise questions, students can download the RD Sharma Solutions for Class 10 Maths Chapter 6 Trigonometric Identities Exercise 6.1 PDF below.

RD Sharma Solutions for Class 10 Maths Chapter 6 Trigonometric Identities Exercise 6.1

Download PDF

carouselExampleControls111

Previous



Next

Access RD Sharma Solutions for Class 10 Maths Chapter 6 Trigonometric Identities Exercise 6.1

Prove the following trigonometric identities:

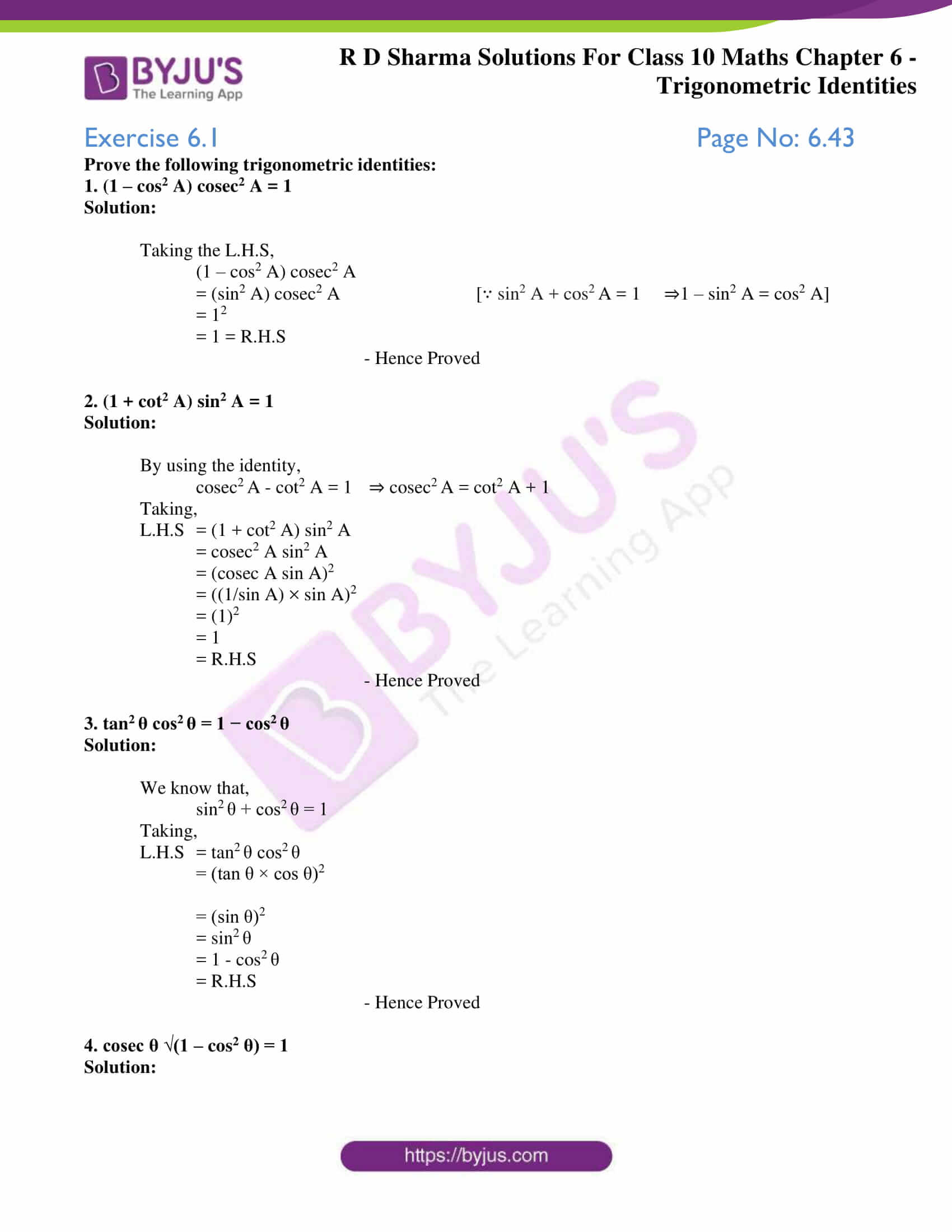
1. (1 – cos² A) cosec² A = 1

Solution:

Taking the L.H.S,

(1 – cos² A) cosec² A

= (sin² A) cosec² A [∵ sin² A + cos² A = 1 ⇒1 – sin² A = cos² A]

= 1²

= 1 = R.H.S

– Hence Proved

2. (1 + cot² A) sin² A = 1 

Solution: 

By using the identity,

cosec² A – cot² A = 1 ⇒ cosec² A = cot² A + 1

Taking,

L.H.S = (1 + cot² A) sin² A

= cosec² A sin² A

= (cosec A sin A)²

= ((1/sin A) × sin A)²

= (1)²

= 1

= R.H.S

– Hence Proved

3. tan² θ cos² θ = 1 − cos² θ 

Solution: 

We know that,

sin² θ + cos² θ = 1

Taking,

L.H.S = tan² θ cos² θ

= (tan θ × cos θ)²

= (sin θ)²

= sin² θ

= 1 – cos² θ

= R.H.S

– Hence Proved

4. cosec θ √(1 – cos² θ) = 1

Solution:

Using identity,

sin² θ + cos² θ = 1  ⇒ sin² θ = 1 – cos² θ

Taking L.H.S,

L.H.S = cosec θ √(1 – cos² θ)

= cosec θ √( sin² θ)

= cosec θ x sin θ

= 1

= R.H.S

– Hence Proved

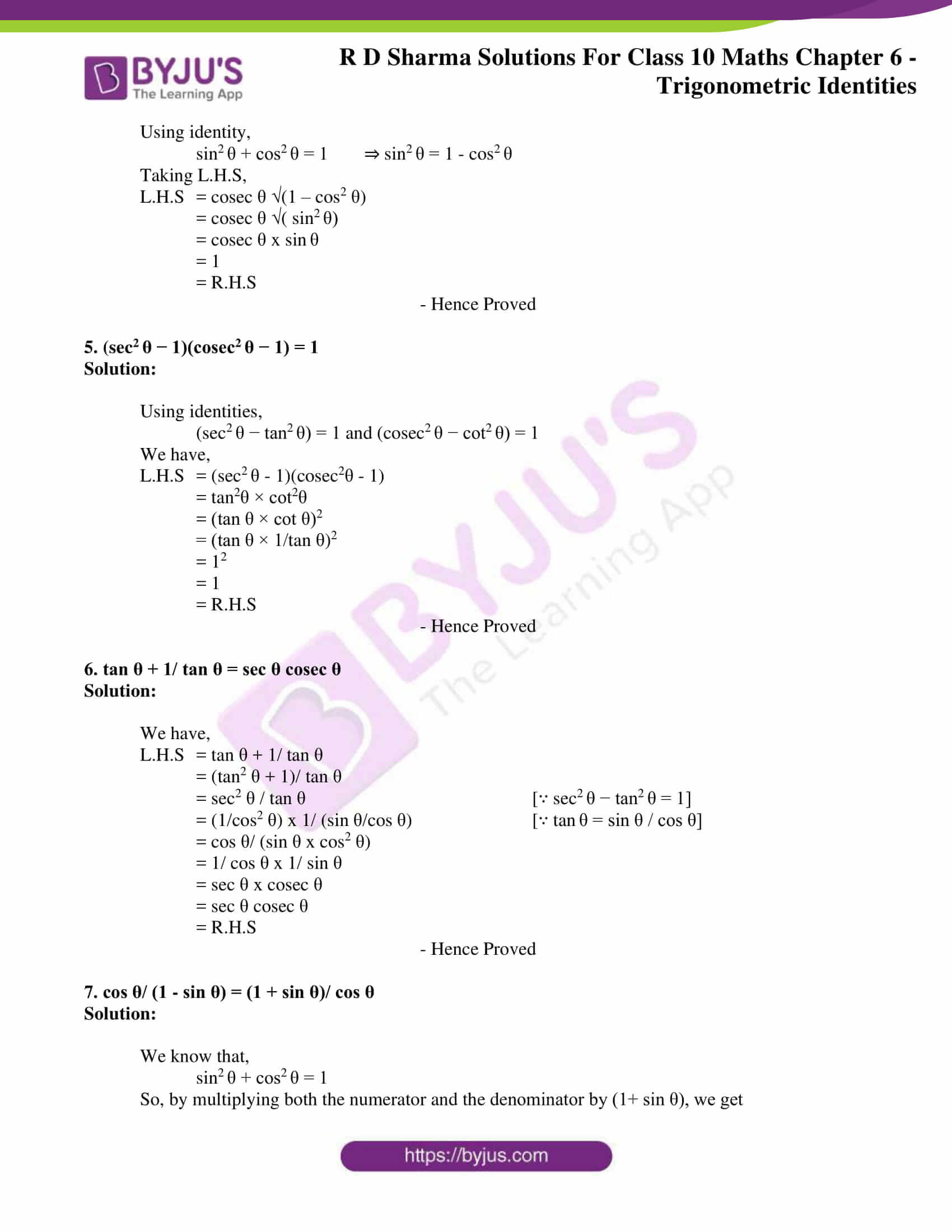
5. (sec² θ − 1)(cosec² θ − 1) = 1 

Solution:

Using identities,

(sec² θ − tan² θ) = 1 and (cosec² θ − cot² θ) = 1

We have,

L.H.S = (sec² θ – 1)(cosec²θ – 1)

= tan²θ × cot²θ

= (tan θ × cot θ)²

= (tan θ × 1/tan θ)²

= 1²

= 1

= R.H.S

– Hence Proved

6. tan θ + 1/ tan θ = sec θ cosec θ

Solution:

We have,

L.H.S = tan θ + 1/ tan θ

= (tan² θ + 1)/ tan θ

= sec² θ / tan θ [∵ sec² θ − tan² θ = 1]

= (1/cos² θ) x 1/ (sin θ/cos θ) [∵ tan θ = sin θ / cos θ]

= cos θ/ (sin θ x cos² θ)

= 1/ cos θ x 1/ sin θ

= sec θ x cosec θ

= sec θ cosec θ

= R.H.S

– Hence Proved

7. cos θ/ (1 – sin θ) = (1 + sin θ)/ cos θ

Solution:

We know that,

sin² θ + cos² θ = 1

So, by multiplying both the numerator and the denominator by (1+ sin θ), we get

L.H.S =

= R.H.S

– Hence Proved

8. cos θ/ (1 + sin θ) = (1 – sin θ)/ cos θ

Solution:

We know that,

sin² θ + cos² θ = 1

So, by multiplying both the numerator and the denominator by (1- sin θ), we get

L.H.S =

= R.H.S

– Hence Proved

9. cos² θ + 1/(1 + cot² θ) = 1

Solution:

We already know that,

cosec² θ − cot² θ = 1 and sin² θ + cos² θ = 1

Taking L.H.S,

= cos² A + sin² A

= 1

= R.H.S

– Hence Proved

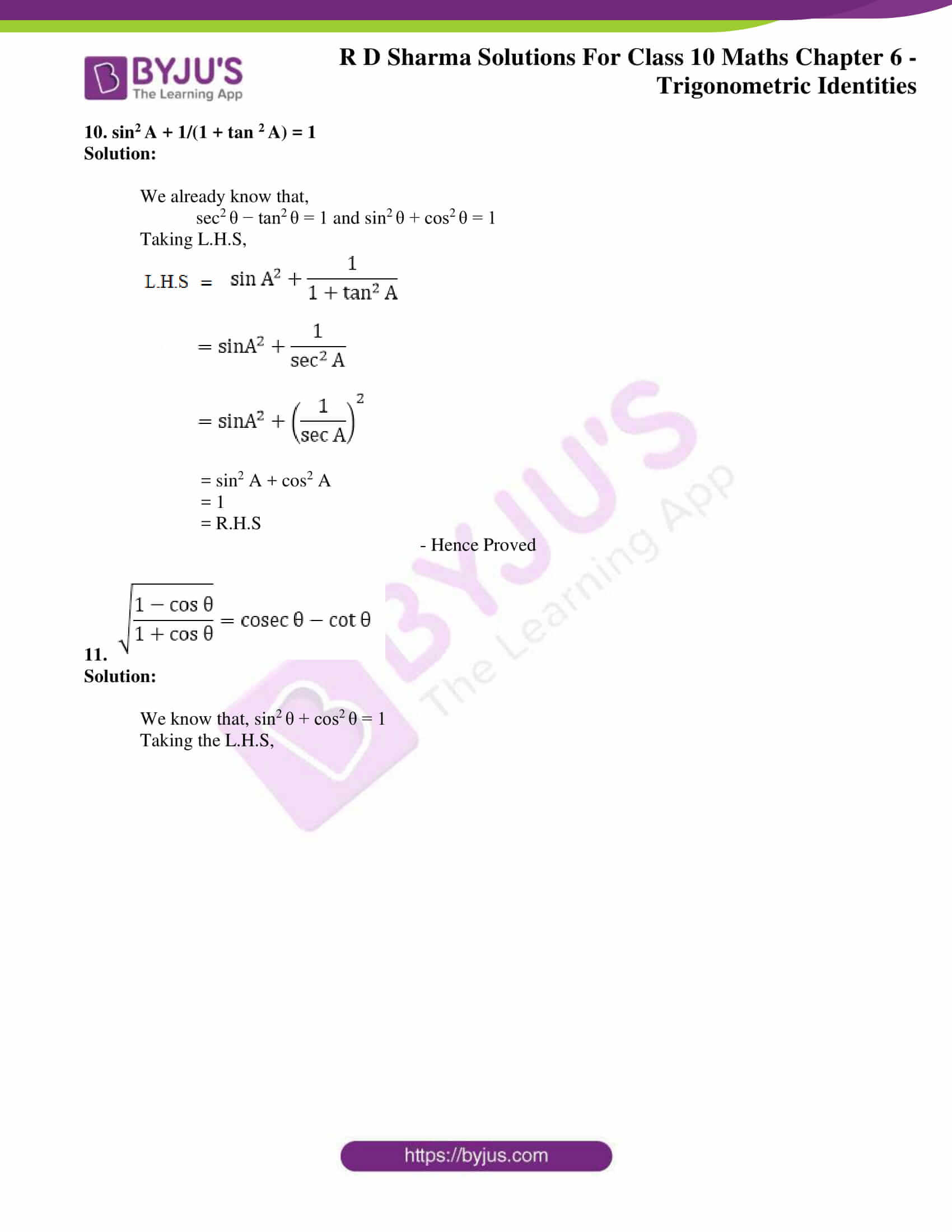
10. sin² A + 1/(1 + tan ² A) = 1

Solution:

We already know that,

sec² θ − tan² θ = 1 and sin² θ + cos² θ = 1

Taking L.H.S,

= sin² A + cos² A

= 1

= R.H.S

– Hence Proved

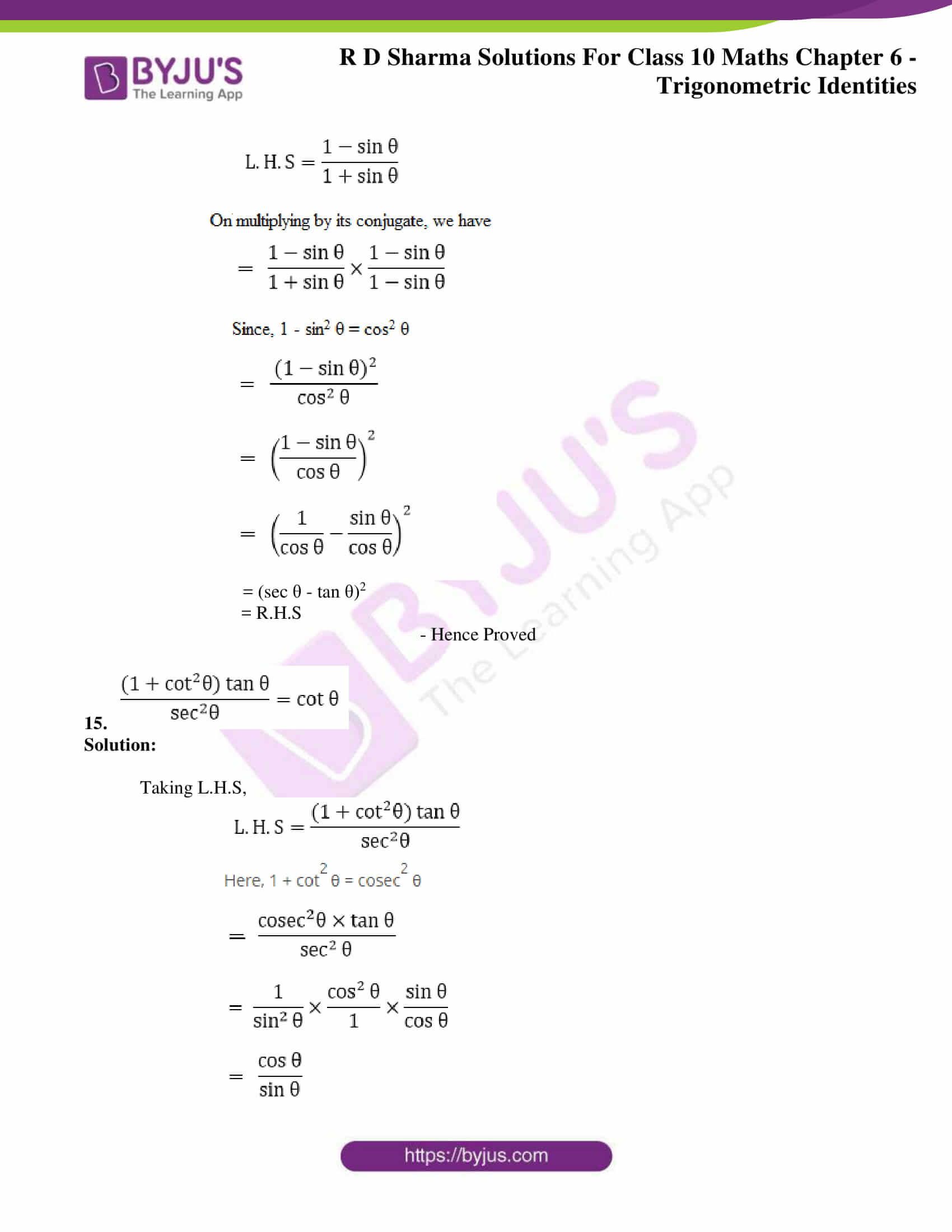
11.

Solution:

We know that, sin² θ + cos² θ = 1

Taking the L.H.S,

= cosec θ – cot θ

= R.H.S

– Hence Proved

12. 1 – cos θ/ sin θ = sin θ/ 1 + cos θ

Solution:

We know that,

sin² θ + cos² θ = 1

So, by multiplying both the numerator and the denominator by (1+ cos θ), we get

= R.H.S

– Hence Proved

13. sin θ/ (1 – cos θ) = cosec θ + cot θ

Solution:

Taking L.H.S,

= cosec θ + cot θ

= R.H.S

– Hence Proved

14. (1 – sin θ) / (1 + sin θ) = (sec θ – tan θ)²

Solution:

Taking the L.H.S,

= (sec θ – tan θ)²

= R.H.S

– Hence Proved

15.

Solution:

Taking L.H.S,

= cot θ

= R.H.S

– Hence Proved

16. tan² θ − sin² θ = tan² θ sin² θ 

Solution:

Taking L.H.S,

L.H.S = tan² θ − sin² θ 

= tan² θ sin² θ

= R.H.S

– Hence Proved

17. (cosec θ + sin θ)(cosec θ – sin θ) = cot²θ + cos²θ 

Solution:

Taking L.H.S = (cosec θ + sin θ)(cosec θ – sin θ)

On multiplying, we get,

= cosec² θ – sin² θ

= (1 + cot² θ) – (1 – cos² θ) [Using cosec² θ − cot² θ = 1 and sin² θ + cos² θ = 1]

= 1 + cot² θ – 1 + cos² θ

= cot² θ + cos² θ

= R.H.S

– Hence Proved

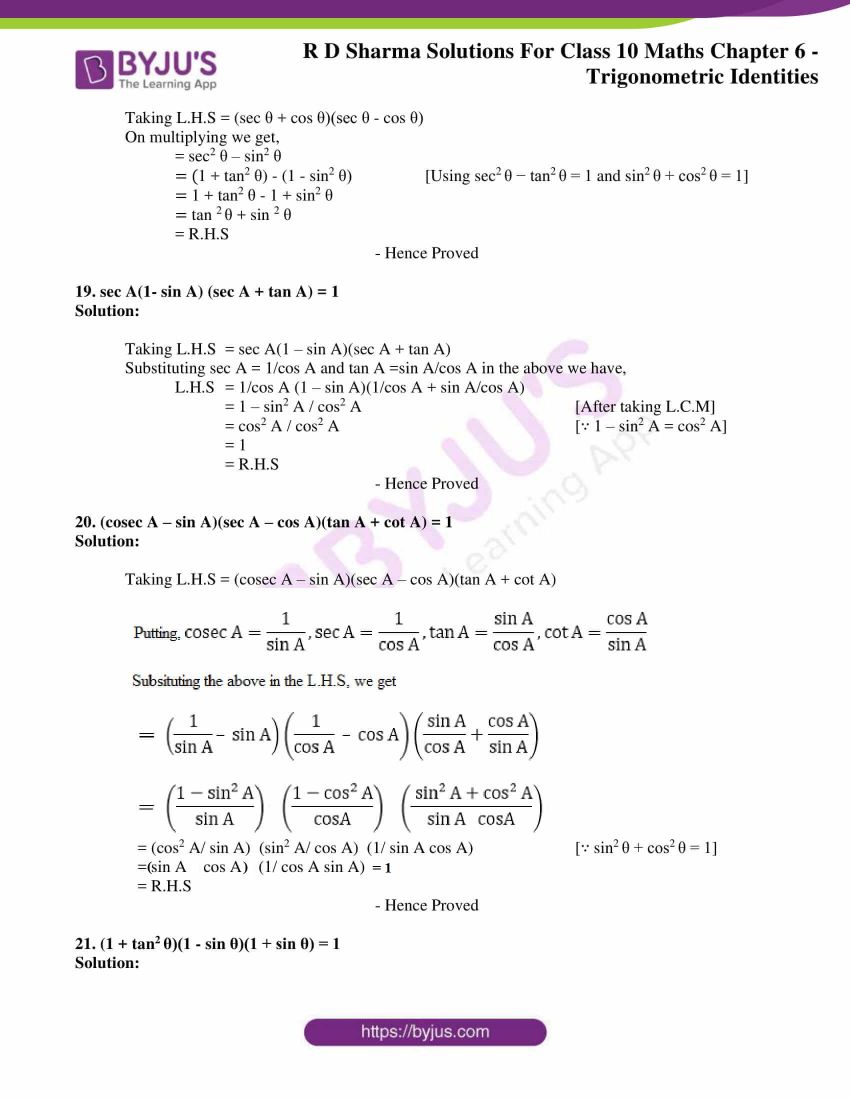
18. (sec θ + cos θ) (sec θ – cos θ) = tan² θ + sin² θ 

Solution:

Taking L.H.S = (sec θ + cos θ)(sec θ – cos θ)

On multiplying, we get,

= sec² θ – sin² θ

= (1 + tan² θ) – (1 – sin² θ) [Using sec² θ − tan² θ = 1 and sin² θ + cos² θ = 1]

= 1 + tan² θ – 1 + sin² θ

= tan ² θ + sin ² θ

= R.H.S

– Hence Proved

19. sec A(1- sin A) (sec A + tan A) = 1

Solution:

Taking L.H.S = sec A(1 – sin A)(sec A + tan A)

Substituting sec A = 1/cos A and tan A =sin A/cos A in the above, we have,

L.H.S = 1/cos A (1 – sin A)(1/cos A + sin A/cos A)

= 1 – sin² A / cos² A [After taking L.C.M]

= cos² A / cos² A [∵ 1 – sin² A = cos² A]

= 1

= R.H.S

– Hence Proved

20. (cosec A – sin A)(sec A – cos A)(tan A + cot A) = 1 

Solution:

Taking L.H.S = (cosec A – sin A)(sec A – cos A)(tan A + cot A)

= (cos² A/ sin A) (sin² A/ cos A) (1/ sin A cos A) [∵ sin² θ + cos² θ = 1]

= (sin A  cos A ) (1/ cos A sin A)

= 1

= R.H.S

– Hence Proved

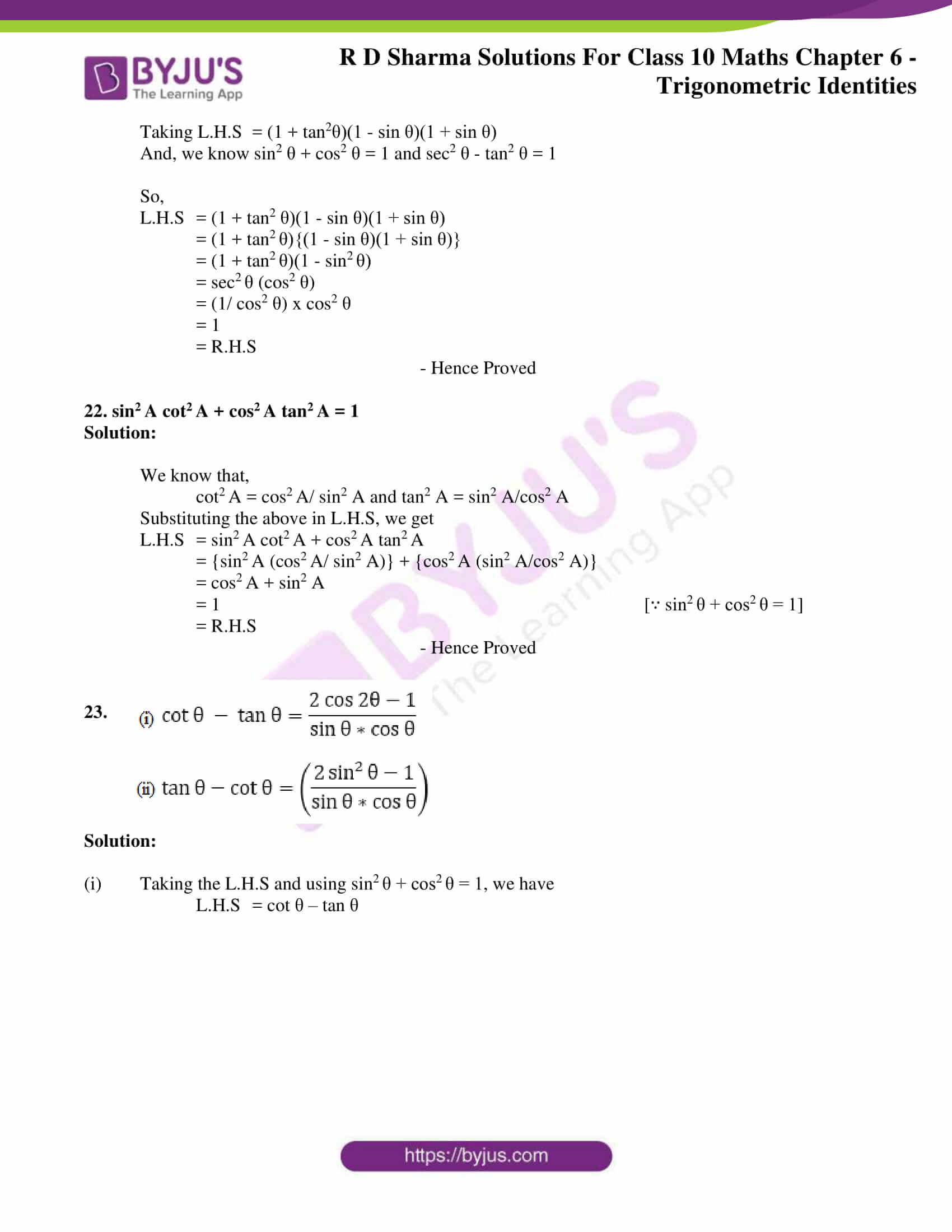
21. (1 + tan² θ)(1 – sin θ)(1 + sin θ) = 1

Solution:

Taking L.H.S = (1 + tan²θ)(1 – sin θ)(1 + sin θ)

And, we know sin² θ + cos² θ = 1 and sec² θ – tan² θ = 1

So,

L.H.S = (1 + tan² θ)(1 – sin θ)(1 + sin θ)

= (1 + tan² θ){(1 – sin θ)(1 + sin θ)}

= (1 + tan² θ)(1 – sin² θ)

= sec² θ (cos² θ)

= (1/ cos² θ) x cos² θ

= 1

= R.H.S

– Hence Proved

22. sin² A cot² A + cos² A tan² A = 1

Solution:

We know that,

cot² A = cos² A/ sin² A and tan² A = sin² A/cos² A

Substituting the above in L.H.S, we get

L.H.S = sin² A cot² A + cos² A tan² A

= {sin² A (cos² A/ sin² A)} + {cos² A (sin² A/cos² A)}

= cos² A + sin² A

= 1 [∵ sin² θ + cos² θ = 1]

= R.H.S

– Hence Proved

23.

Solution:

(i) Taking the L.H.S and using sin² θ + cos² θ = 1, we have

L.H.S = cot θ – tan θ

= R.H.S

– Hence Proved

(ii) Taking the L.H.S and using sin² θ + cos² θ = 1, we have

L.H.S = tan θ – cot θ

= R.H.S

– Hence Proved

24. (cos² θ/ sin θ) – cosec θ + sin θ = 0

Solution:

Taking L.H.S and using sin² θ + cos² θ = 1, we have

= – sin θ + sin θ

= 0

= R.H.S

• Hence proved

25.

Solution:

Taking L.H.S,

= 2 sec² A

= R.H.S

• Hence proved

26.

Solution:

Taking the LHS and using sin² θ + cos² θ = 1, we have

= 2/ cos θ

= 2 sec θ

= R.H.S

• Hence proved

27.

Solution:

Taking the LHS and using sin² θ + cos² θ = 1, we have

= R.H.S

• Hence proved

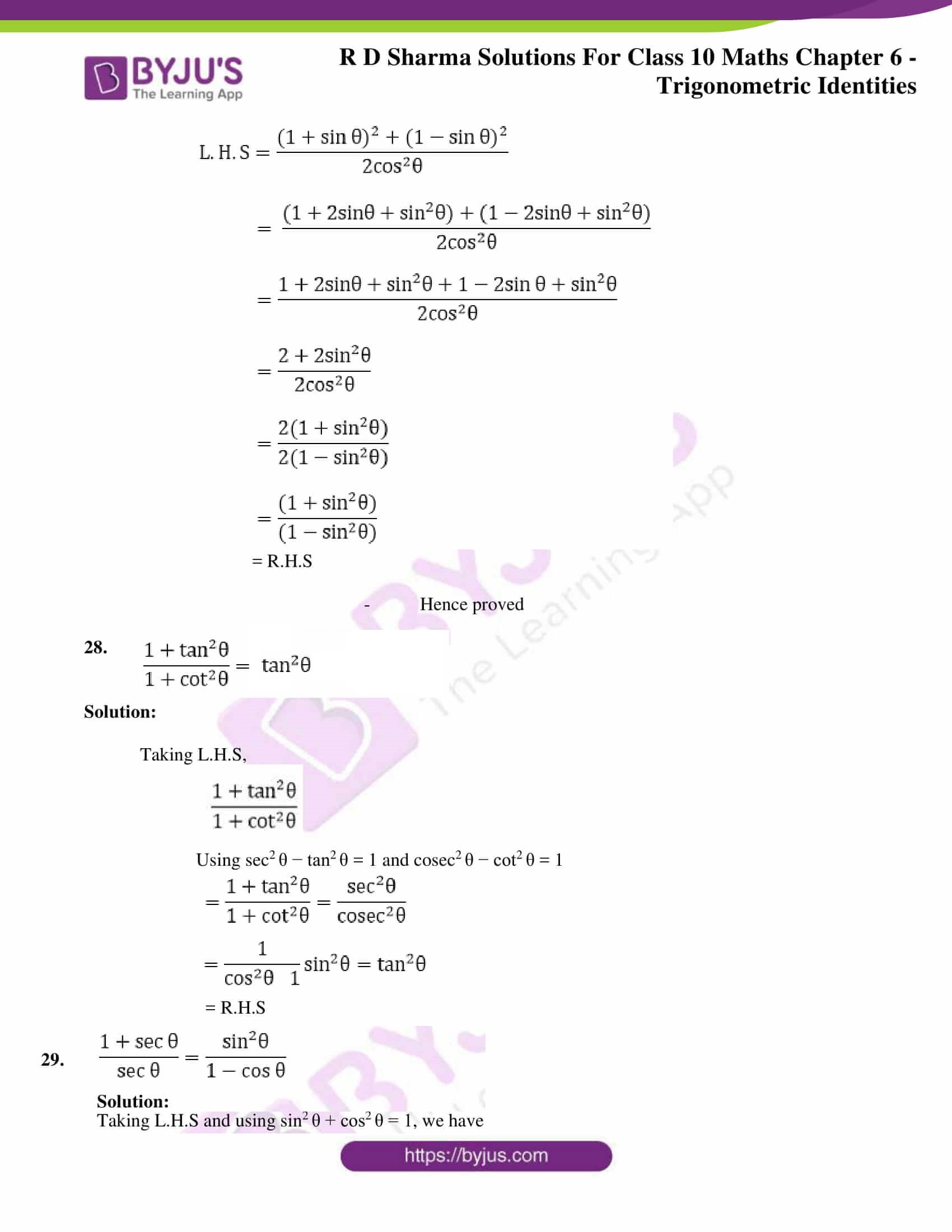
28.

Solution:

Taking L.H.S,

Using sec² θ − tan² θ = 1 and cosec² θ − cot² θ = 1

= R.H.S

29.

Solution:

Taking L.H.S and using sin² θ + cos² θ = 1, we have

= R.H.S

• Hence proved

30.

Solution:

Taking LHS, we have

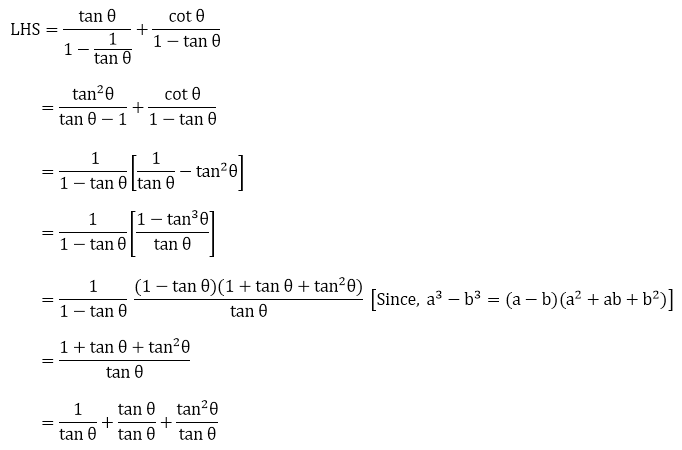
= 1 + tan θ + cot θ

= R.H.S

• Hence proved

31. sec⁶ θ = tan⁶ θ + 3 tan² θ sec² θ + 1

Solution: 

From trig. Identities we have,

sec² θ − tan² θ = 1

On cubing both sides,

(sec²θ − tan²θ)³ = 1

sec⁶ θ − tan⁶ θ − 3sec² θ tan² θ(sec² θ − tan² θ) = 1

sec⁶ θ − tan⁶ θ − 3sec² θ tan² θ = 1

⇒ sec⁶ θ = tan⁶ θ + 3sec² θ tan² θ + 1

Hence, L.H.S = R.H.S

• Hence proved

32. cosec⁶ θ = cot⁶ θ + 3cot² θ cosec² θ + 1

Solution:

From trig. Identities we have,

cosec² θ − cot² θ = 1

On cubing both sides,

(cosec² θ − cot² θ)³ = 1

cosec⁶ θ − cot⁶ θ − 3cosec² θ cot² θ (cosec² θ − cot² θ) = 1

cosec⁶ θ − cot⁶ θ − 3cosec² θ cot² θ = 1

⇒ cosec⁶ θ = cot⁶ θ + 3 cosec² θ cot² θ + 1

Hence, L.H.S = R.H.S

• Hence proved

33.

Solution:

Taking L.H.S and using sec² θ − tan² θ = 1 ⇒ 1 + tan² θ = sec² θ

= R.H.S

• Hence proved

34.

Solution:

Taking L.H.S and using the identity sin²A + cos²A = 1, we get

sin²A = 1 − cos²A

⇒ sin²A = (1 – cos A)(1 + cos A)

• Hence proved

35.

Solution:

We have,

Rationalizing the denominator and numerator with (sec A + tan A) and using sec² θ − tan² θ = 1 we get,

= R.H.S

• Hence proved

36.

Solution:

We have,

On multiplying the numerator and denominator by (1 – cos A), we get

= R.H.S

• Hence proved

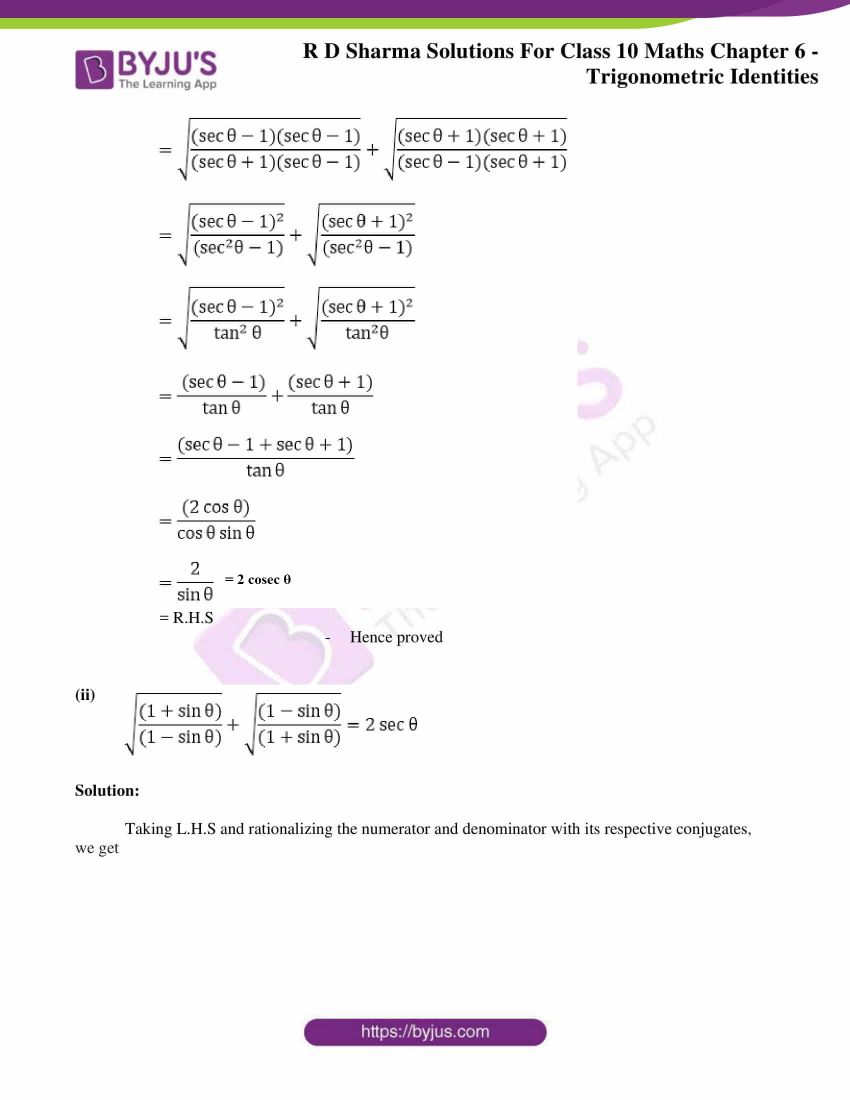
37. (i)

Solution:

Taking L.H.S and rationalizing the numerator and denominator with √(1 + sin A), we get

= R.H.S

• Hence proved

(ii)

Solution:

Taking L.H.S and rationalizing the numerator and denominator with its respective conjugates, we get

= 2 cosec A

= R.H.S

• Hence proved

38. Prove that:

(i)

Solution:

Taking L.H.S and rationalizing the numerator and denominator with its respective conjugates, we get

= 2 cosec θ

= R.H.S

• Hence proved

(ii)

Solution:

Taking L.H.S and rationalizing the numerator and denominator with its respective conjugates, we get

= R.H.S

• Hence proved

(iii)

Solution:

Taking L.H.S and rationalizing the numerator and denominator with its respective conjugates, we get

= 2 cosec θ

= R.H.S

• Hence proved

(iv)

Solution:

Taking L.H.S, we have

= R.H.S

• Hence proved

39.

Solution:

Taking LHS = (sec A – tan A)², we have

= R.H.S

• Hence proved

40.

Solution:

Taking L.H.S and rationalizing the numerator and denominator with (1 – cos A), we get

= (cosec A – cot A)²

= (cot A – cosec A)²

= R.H.S

• Hence proved

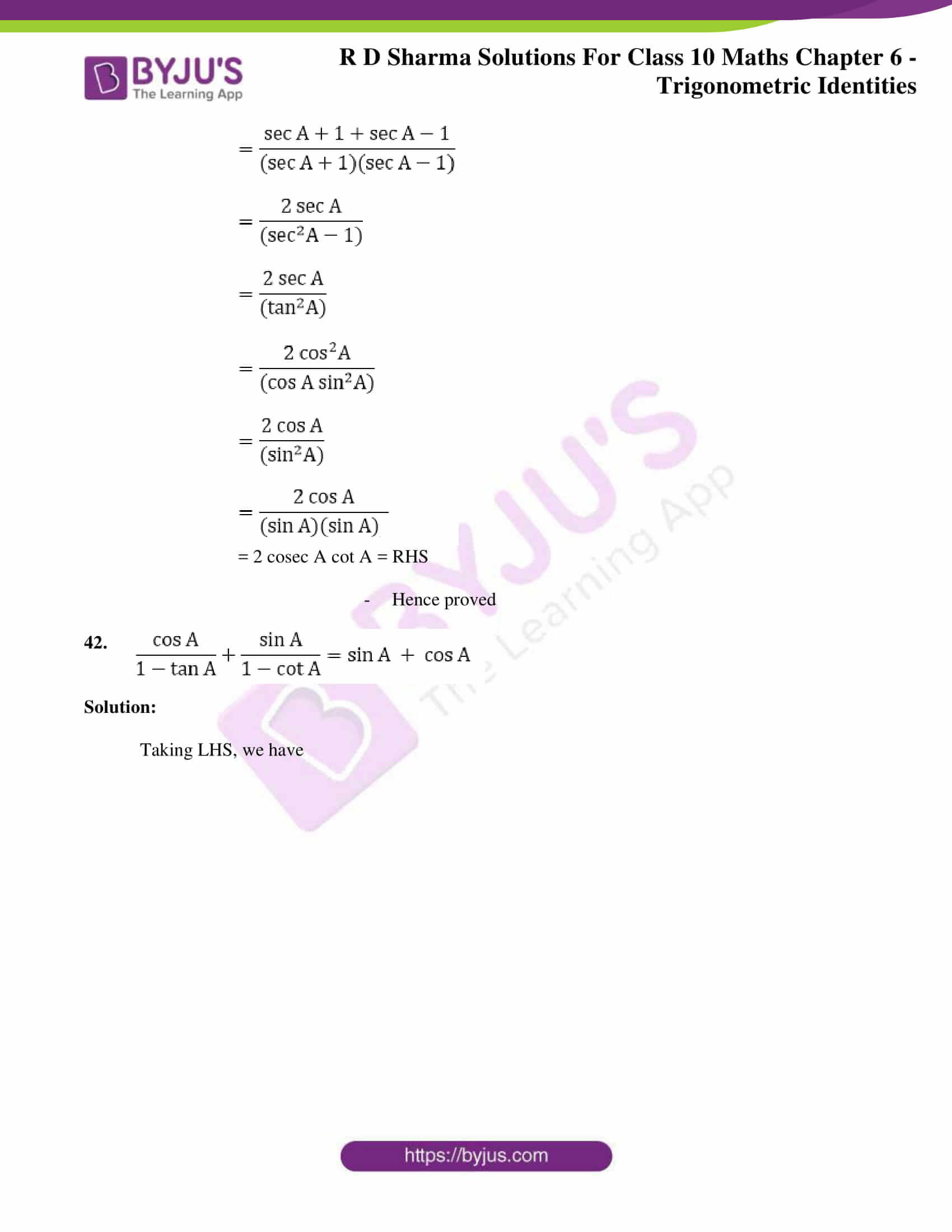
41.

Solution:

Considering L.H.S and taking L.C.M and simplifying, we have,

= 2 cosec A cot A = RHS

• Hence proved

42.

Solution:

Taking LHS, we have

= cos A + sin A

= RHS

• Hence proved

43.

Solution:

Considering L.H.S and taking L.C.M and simplifying, we have,

= 2 sec² A

= RHS

• Hence proved