Students can easily download the RD Sharma Solutions for Exercise 3.7 of Class 8 Maths Chapter 3, Squares and Square Roots, from the link accessible here. BYJU’S subject experts have answered all the questions in Exercise 3.7 in Chapter 3 Maths in an understandable manner, which will help students in solving this exercise. Our main intention is to offer better clarity about the concepts covered in this chapter to students. The answers are designed elaborately in a step-wise format based on the latest CBSE syllabus and evaluation process. Exercise 3.7 of Class 8 is about finding square roots of rational numbers in decimal form. By practising the RD Sharma Class 8 Solutions, students can attain good scores in the annual exams.
RD Sharma Solutions for Class 8 Maths Chapter 3 Squares and Square Roots Exercise 3.7
Access Answers to RD Sharma Solutions for Class 8 Maths Exercise 3.7 Chapter 3 Squares and Square Roots
EXERCISE 3.7 PAGE NO: 3.52
Find the square root of the following numbers in decimal form:
1. 84.8241
Solution:
By using the long division method

∴ the square root of 84.8241
√84.8241 = 9.21
2. 0.7225
Solution:
By using the long division method

∴ the square root of 0.7225
√0.7225 = 0.85
3. 0.813604
Solution:
By using the long division method

∴ the square root of 0.813604
√0.813604 = 0.902
4. 0.00002025
Solution:
By using the long division method

∴ the square root of 0.00002025
√0.00002025 = 0.0045
5. 150.0625
Solution:
By using the long division method

∴ the square root of 150.0625
√150.0625 = 12.25
6. 225.6004
Solution:
By using the long division method

∴ the square root of 225.6004
√225.6004 = 15.02
7. 3600.720036
Solution:
By using the long division method

∴ the square root of 3600.720036
√3600.720036 = 60.006
8. 236.144689
Solution:
By using the long division method

∴ the square root of 236.144689
√236.144689 = 15.367
9. 0.00059049
Solution:
By using the long division method

∴ the square root of 0.00059049
√0.00059049 = 0.0243
10. 176.252176
Solution:
By using the long division method

∴ the square root of 176.252176
√176.252176 = 13.276
11. 9998.0001
Solution:
By using the long division method

∴ the square root of 9998.0001
√9998.0001 = 99.99
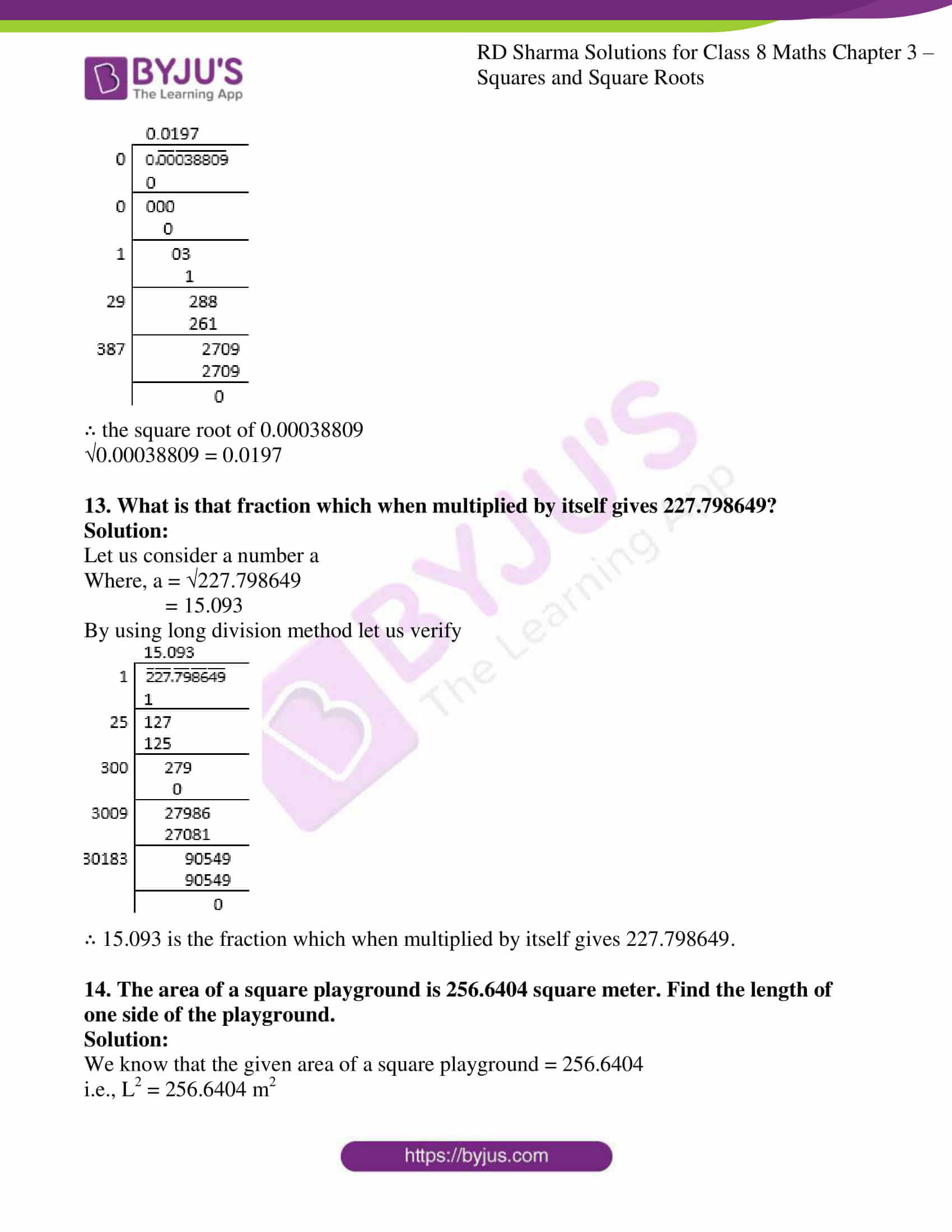
12. 0.00038809
Solution:
By using the long division method

∴ the square root of 0.00038809
√0.00038809 = 0.0197
13. What is that fraction which, when multiplied by itself, gives 227.798649?
Solution:
Let us consider a number a
Where, a = √227.798649
= 15.093
By using the long division method, let us verify

∴ 15.093 is the fraction which, when multiplied by itself, gives 227.798649.
14. The area of a square playground is 256.6404 square metres. Find the length of one side of the playground.
Solution:
We know that the given area of a square playground = 256.6404
i.e., L2 = 256.6404 m2
L = √256.6404
= 16.02m
By using the long division method, let us verify

∴ the length of one side of the playground is 16.02m.
15. What is the fraction which, when multiplied by itself, gives 0.00053361?
Solution:
Let us consider a number a
Where, a = √0.00053361
= 0.0231
By using the long division method, let us verify

∴ 0.0231 is the fraction which, when multiplied by itself, gives 0.00053361.
16. Simplify:
(i) (√59.29 – √5.29)/ (√59.29 + √5.29)
(ii) (√0.2304 + √0.1764)/ (√0.2304 – √0.1764)
Solution:
(i) (√59.29 – √5.29)/ (√59.29 + √5.29)
Firstly, let us find the square root √59.29 and √5.29
√59.29 = √5929/ √100
= 77/10
= 7.7
√5.29 = √5.29/ √100
= 23/10
= 2.3
So, (7.7 – 2.3)/ (7.7 + 2.3)
= 54/10
= 0.54
(ii) (√0.2304 + √0.1764)/ (√0.2304 – √0.1764)
Firstly let us find the square root √0.2304 and √0.1764
√0.2304 = √2304/ √10000
= 48/100
= 0.48
√0.1764 = √1764/ √10000
= 42/100
= 0.42
So, (0.48 + 0.42)/ (0.48 – 0.42)
= 0.9/0.06
= 15
17. Evaluate √50625 and hence find the value of √506.25 + √5.0625
Solution:
By using the long division method, let us find the √50625

So now, √506.25 = √50625/ √100
= 225/10
= 22.5
√5.0625 = √50625/ √10000
= 225/100
= 2.25
So equating in the above equation we get,
√506.25 + √5.0625 = 22.5 + 2.25
= 24.75
18. Find the value of √103.0225 and hence find the value of
(i) √10302.25
(ii) √1.030225
Solution:
By using the long division method, let us find the
√103.0225 = √(1030225/10000) = √1030225/√10000

So now, (i)√10302.25 = √(1030225/ 100)
= 1015/ 10
= 101.5
(ii)√1.030225 = √1030225/ √1000000
= 1015/1000
= 1.015
RD Sharma Solutions for Class 8 Maths Exercise 3.7 Chapter 3 – Squares and Square Roots
Exercise 3.7 of RD Sharma Solutions for Chapter 3 Squares and Square Roots, mainly deals with the concepts related to the square roots of rational numbers in decimal form. RD Sharma Solutions can help the students practise and learn each and every concept, as it provides solutions to all the questions in the RD Sharma textbook. Very lengthy questions that require many steps can be easily remembered by the students by using RD Sharma Solutions. Those students who aim to secure high marks in Class 8 Maths are advised to practise all the questions in the textbook.








Comments