NCERT Exemplar Solutions Class 10 Maths Chapter 3 – Free PDF Download
NCERT Exemplar Class 10 Maths Chapter 3, Pair of Linear Equations in Two Variables, is provided here in PDF. Students can easily download it and prepare well for the board exam. These solutions are formulated by our subject experts as per the latest CBSE syllabus (2023-2024) and NCERT guidelines. It will help students to do last-minute revisions during the board exams.
Chapter 3 helps students with learning how to solve the linear equation algebraically or graphically. They will study different methods of solving linear equations and will be introduced to methods like the elimination method, substitution method and cross-multiplication method. To help students understand and solve the exercise problems in the textbook, NCERT Exemplars for Chapter 3, Pair of Linear Equations in Two Variables, are given here. Click here to get Exemplars for all chapters.
The topics covered in Chapter 3 for Exemplar problems and solutions are listed below:
- Algebraic and geometrical representation of Pair of Linear Equations in Two Variables
- Graphical methods of solving a pair of linear equations
- Algebraic methods of solving a pair of linear equations such as substitution method, elimination method, cross-multiplication method
- Reducing the pair of equations into a linear form
Access and download the Class 10 Maths Chapter 3 NCERT Exemplar PDF from the link below.
NCERT Exemplar Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables
Access Answers to NCERT Exemplar Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables
Exercise 3.1
Choose the correct answer from the given four options:
1. Graphically, the pair of equations
6x – 3y + 10 = 0
2x – y + 9 = 0
represents two lines which are
(A) intersecting at exactly one point (B) intersecting at exactly two points
(C) coincident (D) parallel.
Solution:
(D) Parallel
Explanation:
The given equations are,
6x-3y+10 = 0
dividing by 3
⇒ 2x-y+ 10/3= 0… (i)
And 2x-y+9=0…(ii)
Table for 2x-y+ 10/3 = 0,
| x | 0 | -5/3 |
| y | 10/3 | 0 |
Table for 2x-y+9=0
| x | 0 | -9/2 |
| y | 9 | 0 |
 Hence, the pair of equations represents two parallel lines.
Hence, the pair of equations represents two parallel lines.
2. The pair of equations x + 2y + 5 = 0 and –3x – 6y + 1 = 0 have
(A) a unique solution (B) exactly two solutions
(C) infinitely many solutions (D) no solution
Solution:
(D) no solution
Explanation:
The equations are:
x + 2y + 5 = 0
–3x – 6y + 1 = 0
a1 = 1; b1 = 2; c1 = 5
a2 = -3; b2 = -6; c2 = 1
a1/a2 = -1/3
b1/b2 = -2/6 = -1/3
c1/c2 = 5/1 = 5
Here,
a1/a2 = b1/b2 ≠ c1/c2
Therefore, the pair of equations has no solution.
3. If a pair of linear equations is consistent, then the lines will be
(A) parallel (B) always coincident
(C) intersecting or coincident (D) always intersecting
Solution:
(C) intersecting or coincident
Explanation:
Conditions for a pair of linear equations to be consistent are:
Intersecting lines, having a unique solution,
a1/a2 ≠ b1/b2
Coincident or dependent
a1/a2 = b1/b2 = c1/c2
4. The pair of equations y = 0 and y = –7 has
(A) one solution (B) two solutions
(C) infinitely many solutions (D) no solution
Solution:
(D) no solution
Explanation:
The given pair of equations are y = 0 and y = – 7.

Graphically, both lines are parallel and have no solution
5. The pair of equations x = a and y = b graphically represents lines which are
(A) parallel (B) intersecting at (b, a)
(C) coincident (D) intersecting at (a, b)
Solution:
(D) intersecting at (a, b)
Explanation:
Graphically in every condition,
a, b>>0
a, b< 0
a>0, b< 0
a<0, b>0 but a = b≠ 0.
The pair of equations x = a and y = b graphically represents lines which are intersecting
at (a, b).
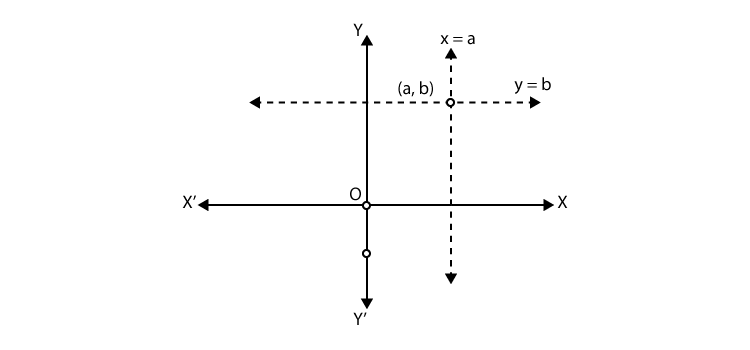
Hence, in this case, two lines intersect at (a, b).
Exercise 3.2
1. Do the following pair of linear equations have no solution? Justify your answer.
(i) 2x + 4y = 3
12y + 6x = 6
(ii) x = 2y
y = 2x
(iii) 3x + y – 3 = 0
2x + 2/3y = 2
Solution:
The Condition for no solution = a1/a2 = b1/b2 ≠ c1/c2 (parallel lines)
(i) Yes.
Given pair of equations are,
2x+4y – 3 = 0 and 6x + 12y – 6 = 0
Comparing the equations with ax+ by +c = 0;
We get,
a1 = 2, b1 = 4, c1 = – 3;
a2 = 6, b2 = 12, c2 = – 6;
a1 /a2 = 2/6 = 1/3
b1 /b2 = 4/12 = 1/3
c1 /c2 = – 3/ – 6 = ½
Here, a1/a2 = b1/b2 ≠ c1/c2, i.e parallel lines
Hence, the given pair of linear equations has no solution.
(ii) No.
Given pair of equations,
x = 2y or x – 2y = 0
y = 2x or 2x – y = 0;
Comparing the equations with ax+ by +c = 0;
We get,
a1 = 1, b1 = – 2, c1 = 0;
a2 = 2, b2 = – 1, c2 = 0;
a1 /a2 = ½
b1 /b2 = -2/-1 = 2
Here, a1/a2 ≠ b1/b2.
Hence, the given pair of linear equations has a unique solution.
(iii) No.
Given pair of equations,
3x + y – 3 = 0
2x + 2/3 y = 2
Comparing the equations with ax+ by +c = 0;
We get,
a1 = 3, b1 = 1, c1 = – 3;
a2 = 2, b2 = 2/3, c2 = – 2;
a1 /a2 = 2/6 = 3/2
b1 /b2 = 4/12 = 3/2
c1 /c2 = – 3/-2 = 3/2
Here, a1/a2 = b1/b2 = c1/c2, i.e coincident lines
2. Do the following equations represent a pair of coincident lines? Justify your answer.
(i) 3x + 1/7y = 3
7x + 3y = 7
(ii) –2x – 3y = 1
6y + 4x = – 2
(iii) x/2 + y + 2/5 = 0
4x + 8y + 5/16 = 0
Solution:
Condition for coincident lines,
a1/a2 = b1/b2 = c1/c2;
(i) No.
Given pair of linear equations are:
3x + 1/7y = 3
7x + 3y = 7
Comparing the above equations with ax + by + c = 0;
Here, a1 = 3, b1 = 1/7, c1 = – 3;
And a2 = 7, b2 = 3, c2 = – 7;
a1 /a2 = 3/7
b1 /b2 = 1/21
c1 /c2 = – 3/ – 7 = 3/7
Here, a1/a2 ≠ b1/b2.
Hence, the given pair of linear equations has a unique solution.
(ii) Yes.
Given pair of linear equations.
– 2x – 3y – 1 = 0 and 4x + 6y + 2 = 0;
Comparing the above equations with ax + by + c = 0;
Here, a1 = – 2, b1 = – 3, c1 = – 1;
And a2 = 4, b2 = 6, c2 = 2;
a1 /a2 = – 2/4 = – ½
b1 /b2 = – 3/6 = – ½
c1 /c2 = – ½
Here, a1/a2 = b1/b2 = c1/c2, i.e. coincident lines
Hence, the given pair of linear equations is coincident.
(iii) No.
Given pair of linear equations are
x/2 + y + 2/5 = 0
4x + 8y + 5/16 = 0
Comparing the above equations with ax + by + c = 0;
Here, a1 = ½, b1 = 1, c1 = 2/5;
And a2 = 4, b2 = 8, c2 = 5/16;
a1 /a2 = 1/8
b1 /b2 = 1/8
c1 /c2 = 32/25
Here, a1/a2 = b1/b2 ≠ c1/c2, i.e. parallel lines
Hence, the given pair of linear equations has no solution.
3. Are the following pair of linear equations consistent? Justify your answer.
(i) –3x– 4y = 12
4y + 3x = 12
(ii) (3/5)x – y = ½
(1/5)x – 3y= 1/6
(iii) 2ax + by = a
- ax + 2by – 2a = 0; a, b ≠ 0
(iv) x + 3y = 11
2 (2x + 6y) = 22
Solution:
Conditions for pair of linear equations to be consistent are:
a1/a2 ≠ b1/b2. [unique solution]
a1/a2 = b1/b2 = c1/c2 [coincident or infinitely many solutions]
(i) No.
The given pair of linear equations
– 3x – 4y – 12 = 0 and 4y + 3x – 12 = 0
Comparing the above equations with ax + by + c = 0;
We get,
a1 = – 3, b1 = – 4, c1 = – 12;
a2 = 3, b2 = 4, c2 = – 12;
a1 /a2 = – 3/3 = – 1
b1 /b2 = – 4/4 = – 1
c1 /c2 = – 12/ – 12 = 1
Here, a1/a2 = b1/b2 ≠ c1/c2
Hence, the pair of linear equations has no solution, i.e., inconsistent.
(ii) Yes.
The given pair of linear equations
(3/5)x – y = ½
(1/5)x – 3y= 1/6
Comparing the above equations with ax + by + c = 0;
We get,
a1 = 3/5, b1 = – 1, c1 = – ½;
a2 = 1/5, b2 = 3, c2 = – 1/6;
a1 /a2 = 3
b1 /b2 = – 1/ – 3 = 1/3
c1 /c2 = 3
Here, a1/a2 ≠ b1/b2.
Hence, the given pair of linear equations has a unique solution, i.e., consistent.
(iii) Yes.
The given pair of linear equations –
2ax + by –a = 0 and 4ax + 2by – 2a = 0
Comparing the above equations with ax + by + c = 0;
We get,
a1 = 2a, b1 = b, c1 = – a;
a2 = 4a, b2 = 2b, c2 = – 2a;
a1 /a2 = ½
b1 /b2 = ½
c1 /c2 = ½
Here, a1/a2 = b1/b2 = c1/c2
Hence, the given pair of linear equations has infinitely many solutions, i.e., consistent
(iv) No.
The given pair of linear equations
x + 3y = 11 and 2x + 6y = 11
Comparing the above equations with ax + by + c = 0;
We get,
a1 = 1, b1 = 3, c1 = 11
a2 = 2, b2 = 6, c2 = 11
a1 /a2 = ½
b1 /b2 = ½
c1 /c2 = 1
Here, a1/a2 = b1/b2 ≠ c1/c2.
Hence, the given pair of linear equations has no solution.
Exercise 3.3
1. For which value(s) of λ , do the pair of linear equations
λx + y = λ2 and x + λy = 1 have
(i) no solution?
(ii) infinitely many solutions?
(iii) a unique solution?
Solution:
The given pair of linear equations is
λx + y = λ2 and x + λy = 1
a1 = λ, b1= 1, c1 = – λ2
a2 =1, b2=λ, c2=-1
The given equations are;
λ x + y – λ2 = 0
x + λ y – 1 = 0
Comparing the above equations with ax + by + c = 0;
We get,
a1 = λ, b1 = 1, c1 = – λ 2;
a2 = 1, b2 = λ, c2 = – 1;
a1 /a2 = λ/1
b1 /b2 = 1/λ
c1 /c2 = λ2
(i) For no solution,
a1/a2 = b1/b2≠ c1/c2
i.e. λ = 1/ λ ≠ λ2
so, λ 2 = 1;
and λ 2 ≠ λ
Here, we take only λ = – 1,
Since the system of linear equations has no solution.
(ii) For infinitely many solutions,
a1/a2 = b1/b2 = c1/c2
i.e. λ = 1/ λ = λ2
so λ = 1/ λ gives λ = + 1;
λ = λ 2 gives λ = 1,0;
Hence satisfying both the equations
λ = 1 is the answer.
(iii) For a unique solution,
a1/a2 ≠ b1/b2
so λ ≠1/ λ
hence, λ2 ≠ 1;
λ ≠ + 1;
So, all real values of λ except +1.
2. For which value(s) of k will the pair of equations
kx + 3y = k – 3
12x + ky = k
have no solution?
Solution:
The given pair of linear equations is
kx + 3y = k – 3 …(i)
12x + ky = k …(ii)
On comparing the equations (i) and (ii) with ax + by = c = 0,
We get,
a1 = k, b1 = 3, c1 = -(k – 3)
a2 = 12, b2 = k, c2 = – k
Then,
a1 /a2 = k/12
b1 /b2 = 3/k
c1 /c2 = (k-3)/k
For no solution of the pair of linear equations,
a1/a2 = b1/b2≠ c1/c2
k/12 = 3/k ≠ (k-3)/k
Taking the first two parts, we get
k/12 = 3/k
k2 = 36
k = + 6
Taking the last two parts, we get
3/k ≠ (k-3)/k
3k ≠ k(k – 3)
k2 – 6k ≠ 0
so, k ≠ 0,6
Therefore, the value of k for which the given pair of linear equations has no solution is k = – 6.
3. For which values of a and b will the following pair of linear equations have infinitely many solutions?
x + 2y = 1
(a – b)x + (a + b)y = a + b – 2
Solution:
The given pair of linear equations are:
x + 2y = 1 …(i)
(a-b)x + (a + b)y = a + b – 2 …(ii)
On comparing with ax + by = c = 0 we get
a1 = 1, b1 = 2, c1 = – 1
a2 = (a – b), b2 = (a + b), c2 = – (a + b – 2)
a1 /a2 = 1/(a-b)
b1 /b2 = 2/(a+b)
c1 /c2 = 1/(a+b-2)
For infinitely many solutions of the pair of linear equations,
a1/a2 = b1/b2=c1/c2(coincident lines)
so, 1/(a-b) = 2/ (a+b) = 1/(a+b-2)
Taking the first two parts,
1/(a-b) = 2/ (a+b)
a + b = 2(a – b)
a = 3b …(iii)
Taking the last two parts,
2/ (a+b) = 1/(a+b-2)
2(a + b – 2) = (a + b)
a + b = 4 …(iv)
Now, put the value of a from Eq. (iii) in Eq. (iv), and we get
3b + b = 4
4b = 4
b = 1
Put the value of b in Eq. (iii), and we get
a = 3
So, the values (a,b) = (3,1) satisfy all the parts. Hence, the required values of a and b are 3 and 1, respectively, for which the given pair of linear equations has infinitely many solutions.
4. Find the value(s) of p in (i) to (iv) and p and q in (v) for the following pair of equations:
(i) 3x – y – 5 = 0 and 6x – 2y – p = 0, if the lines represented by these equations are parallel.
Solution:
Given pair of linear equations is
3x – y – 5 = 0 …(i)
6x – 2y – p = 0 …(ii)
On comparing with ax + by + c = 0 we get
We get,
a1 = 3, b1 = – 1, c1 = – 5;
a2 = 6, b2 = – 2, c2 = – p;
a1 /a2 = 3/6 = ½
b1 /b2 = ½
c1 /c2 = 5/p
Since the lines represented by these equations are parallel, then
a1/a2 = b1/b2 ≠ c1/c2
Taking the last two parts, we get ½ ≠ 5/p
So, p ≠ 10
Hence, the given pair of linear equations are parallel for all real values of p except 10.
(ii) – x + py = 1 and px – y = 1, if the pair of equations has no solution.
Solution:
Given pair of linear equations is
– x + py = 1 …(i)
px – y – 1 = 0 …(ii)
On comparing with ax + by + c = 0,
We get,
a1 = -1, b1 = p, c1 =- 1;
a2 = p, b2 = – 1, c2 =- 1;
a1 /a2 = -1/p
b1 /b2 = – p
c1 /c2 = 1
Since the lines equations have no solution, i.e., both lines are parallel to each other,
a1/a2 = b1/b2≠ c1/c2
-1/p = – p ≠ 1
Taking the last two parts, we get
p ≠ -1
Taking the first two parts, we get
p2 = 1
p = + 1
Hence, the given pair of linear equations has no solution for p = 1.
(iii) – 3x + 5y = 7 and 2px – 3y = 1, if the lines represented by these equations are intersecting at a unique point.
Solution:
Given, pair of linear equations is
– 3x + 5y = 7
2px – 3y = 1
On comparing with ax + by + c = 0, we get
Here, a1 = -3, b1 = 5, c1 = – 7;
And a2 = 2p, b2 = – 3, c2 = – 1;
a1 /a2 = -3/ 2p
b1 /b2 = – 5/3
c1 /c2 = 7
Since the lines intersect at a unique point, i.e., it has a unique solution
a1/a2 ≠ b1/b2
-3/2p ≠ -5/3
p ≠ 9/10
Hence, the lines represented by these equations intersect at a unique point for all real values of p except 9/10.
(iv) 2x + 3y – 5 = 0 and px – 6y – 8 = 0, if the pair of equations has a unique solution.
Solution:
Given, pair of linear equations is
2x + 3y – 5 = 0
px – 6y – 8 = 0
On comparing with ax + by + c = 0 we get
Here, a1 = 2, b1 = 3, c1 = – 5;
And a2 = p, b2 = – 6, c2 = – 8;
a1 /a2 = 2/p
b1 /b2 = – 3/6 = – ½
c1 /c2 = 5/8
Since the pair of linear equations has a unique solution,
a1/a2 ≠ b1/b2
so 2/p ≠ – ½
p ≠ – 4
Hence, the pair of linear equations has a unique solution for all values of p except – 4.
(v) 2x + 3y = 7 and 2px + py = 28 – qy, if the pair of equations has infinitely many solutions.
Solution:
Given pair of linear equations is
2x + 3y = 7
2px + py = 28 – qy
or 2px + (p + q)y – 28 = 0
On comparing with ax + by + c = 0,
We get,
Here, a1 = 2, b1 = 3, c1 = – 7;
And a2 = 2p, b2 = (p + q), c2 = – 28;
a1/a2 = 2/2p
b1/b2 = 3/ (p+q)
c1/c2 = ¼
Since the pair of equations has infinitely many solutions i.e., both lines are coincident.
a1/a2 = b1/b2 = c1/c2
1/p = 3/(p+q) = ¼
Taking the first and third parts, we get
p = 4
Again, taking the last two parts, we get
3/(p+q) = ¼
p + q = 12
Since p = 4
So, q = 8
Here, we see that the values of p = 4 and q = 8 satisfy all three parts.
Hence, the pair of equations has infinitely many solutions for all values of p = 4 and q = 8.
5. Two straight paths are represented by the equations x – 3y = 2 and –2x + 6y = 5.Check whether the paths cross each other or not.
Solution:
Given linear equations are
x – 3y – 2 = 0 …(i)
-2x + 6y – 5 = 0 …(ii)
On comparing with ax + by c=0,
We get
a1 =1, b1 =-3, c1 =- 2;
a2 = -2, b2 =6, c2 =- 5;
a1/a2 = – ½
b1/b2 = – 3/6 = – ½
c1/c2 = 2/5
i.e., a1/a2 = b1/b2 ≠ c1/c2 [parallel lines]
Hence, two straight paths represented by the given equations never cross each other because they are parallel to each other.
6. Write a pair of linear equations which has the unique solution x = – 1, y =3. How many such pairs can you write?
Solution:
Condition for the pair of system to have a unique solution
a1/a2 ≠ b1/b2
Let the equations be,
a1x + b1y + c1 = 0
a2x + b2y + c2 = 0
Since, x = – 1 and y = 3 is the unique solution of these two equations, then
It must satisfy the equations –
a1(-1) + b1(3) + c1 = 0
– a1 + 3b1 + c1 = 0 …(i)
and a2(- 1) + b2(3) + c2 = 0
– a2 + 3b2 + c2 = 0 …(ii)
Since for the different values of a1, b1, c1 and a2, b2, c2 satisfy the Eqs. (i) and (ii),

Hence, infinitely many pairs of linear equations are possible.
7. If 2x + y = 23 and 4x – y = 19, find the values of 5y – 2x and y/x – 2.
Solution:
Given equations are
2x + y = 23 …(i)
4x – y = 19 …(ii)
On adding both equations, we get
6x = 42
So, x = 7
Put the value of x in Eq. (i), and we get
2(7) + y = 23
y = 23 – 14
so, y = 9
Hence 5y – 2x = 5(9) – 2(7) = 45 – 14 = 31
y/x – 2 = 9/7 -2 = -5/7
Hence, the values of (5y – 2x) and y/x – 2 are 31 and -5/7 respectively.
8. Find the values of x and y in the following rectangle [see Fig. 3.2].

Solution:
Using the property of a rectangle,
We know that,
Lengths are equal,
i.e., CD = AB
Hence, x + 3y = 13 …(i)
Breadth are equal,
i.e., AD = BC
Hence, 3x + y = 7 …(ii)
On multiplying Eq. (ii) by 3 and then subtracting Eq. (i),
We get,
8x = 8
So, x = 1
On substituting x = 1 in Eq. (i),
We get,
y = 4
Therefore, the required values of x and y are 1 and 4, respectively.
Exercise 3.4
1. Graphically, solve the following pair of equations:
2x + y = 6
2x – y + 2 = 0
Find the ratio of the areas of the two triangles formed by the lines representing these equations with the x-axis and the lines with the y-axis.
Solution:
Given equations are 2x + y = 6 and 2x – y + 2 = 0
Table for equation 2x + y – 6 = 0, for x = 0, y = 6, for y = 0, x = 3.
| x | 0 | 3 |
| y | 6 | 0 |
Table for equation 2x – y + 2 = 0, for x = 0, y = 2, for y = 0,x = – 1
| x | 0 | -1 |
| y | 2 | 0 |
Let A1 and A2 represent the areas of triangles ACE and BDE, respectively.

Let the area of triangle formed with x-axis = T1
T1 = Area of △ACE = ½ × AC × PE
T1 = ½ × 4 × 4 = 8
And area of triangle formed with y-axis = T2
T1 = Area of △BDE = ½ × BD × QE
T1 = ½ × 4 × 1 = 2
T1:T2 = 8:2 = 4:1
Hence, the pair of equations intersect graphically at point E(1,4)
i.e., x = 1 and y = 4.
2. Determine, graphically, the vertices of the triangle formed by the lines
y = x, 3y = x, x + y = 8
Solution:
Given linear equations are
y = x …(i)
3y = x …(ii)
and x + y = 8 …(iii)
Table for line y = x,
| x | 0 | 1 | 2 |
| y | 0 | 1 | 2 |
Table for line x = 3y,
| x | 0 | 3 | 6 |
| y | 0 | 1 | 2 |
Table for line x + y = 8
| x | 0 | 4 | 8 |
| y | 8 | 4 | 0 |
Plotting the points A (1, 1), B(2,2), C (3, 1), D (6, 2), we get the straight lines AB and CD.
Similarly, plotting the points P (0, 8), Q(4, 4) and R(8, 0), we get the straight line PQR.
AB and CD intersect the line PR on Q and D, respectively.

So, △OQD is formed by these lines. Hence, the vertices of the △OQD formed by the given lines are O(0, 0), Q(4, 4) and D(6,2).
3. Draw the graphs of the equations x = 3, x = 5 and 2x – y – 4 = 0. Also, find the area of the quadrilateral formed by the lines and the x–axis.
Solution:
Given equation of lines x = 3, x = 5 and 2x-y-4 = 0.
Table for line 2x – y – 4 = 0
| x | 0 | 2 |
| y | -4 | 0 |
Plotting the graph, we get,

From the graph, we get,
AB = OB-OA = 5-3 = 2
AD = 2
BC = 6
Thus, quadrilateral ABCD is a trapezium, then,
Area of quadrilateral ABCD = ½ × (distance between parallel lines) = ½ × (AB) × (AD + BC)
= 8 sq units
4. The cost of 4 pens and 4 pencil boxes is Rs. 100. Three times the cost of a pen is Rs. 15 more than the cost of a pencil box. Form the pair of linear equations for the above situation. Find the cost of a pen and a pencil box.
Solution:
Let the cost of a pen and a pencil box be Rs x and Rs y, respectively.
According to the question,
4x + 4y = 100
Or x + y = 25 …(i)
3x = y + 15
Or 3x-y = 15 …(ii)
On adding Equation (i) and (ii), we get,
4x = 40
So, x = 10
Substituting x = 10, in Eq. (i), we get
y = 25-10 = 15
Hence, the cost of a pen = Rs. 10
The cost of a pencil box = Rs. 15
5. Determine, algebraically, the vertices of the triangle formed by the lines
3x – y = 3
2x – 3y = 2
x + 2y = 8
Solution:
3x – y = 2 …(i)
2x -3y = 2 …(ii)
x + 2y = 8 …(iii)
Let the equations of the line (i), (ii) and (iii) represent the side of a ∆ABC.
On solving (i) and (ii),
We get,
[First, multiply Eq. (i) by 3 in Eq. (i) and then subtract](9x-3y)-(2x-3y) = 9-2
7x = 7
x = 1
Substituting x=1 in Eq. (i), we get
3×1-y = 3
y = 0
So, the coordinate of point B is (1, 0)
On solving lines (ii) and (iii),
We get,
[First, multiply Eq. (iii) by 2 and then subtract](2x + 4y)-(2x-3y) = 16-2
7y = 14
y = 2
Substituting y=2 in Eq. (iii), we get
x + 2 × 2 = 8
x + 4 = 8
x = 4
Hence, the coordinate of point C is (4, 2).
On solving lines (iii) and (i),
We get,
[First, multiply in Eq. (i) by 2 and then add](6x-2y) + (x + 2y) = 6 + 8
7x = 14
x = 2
Substituting x=2 in Eq. (i), we get
3 × 2 – y = 3
y = 3
So, the coordinate of point A is (2, 3).
Hence, the vertices of the ∆ABC formed by the given lines are as follows,
A (2, 3), B (1, 0) and C (4, 2).
6. Ankita travels 14 km to her home partly by rickshaw and partly by bus. She takes half an hour if she travels 2 km by rickshaw and the remaining distance by bus. On the other hand, if she travels 4 km by rickshaw and the remaining distance by bus, she takes 9 minutes longer. Find the speed of the rickshaw and of the bus.
Solution:
Let the speed of the rickshaw and the bus be x and y km/h, respectively.
Now, she has taken time to travel 2 km by rickshaw, t1 = (2/x) hr
Speed = distance/ time
She has taken time to travel the remaining distance i.e., (14 – 2) = 12km
By bus t2 = (12/y) hr
By the first condition,
t1 + t2 = ½ = (2/x) + (12/y) … (i)
Now, she has taken time to travel 4 km by rickshaw, t3 = (4/x) hr
and she has taken time to travel the remaining distance i.e., (14 – 4) = 10km, by bus = t4 = (10/y) hr
By the second condition,
t3 + t4 = ½ + 9/60 = ½ + 3/20
(4/x) + (10/y) = (13/20) …(ii)
Let (1/x) = u and (1/y) = v
Then equations (i) and (ii) become
2u + 12v = ½ …(iii)
4u + 10v = 13/20…(iv)
[First, multiply Eq. (iii) by 2 and then subtract](4u + 24v) – (4u + 10v) = 1–13/20
14v = 7/20
v = 1/40
Substituting the value of v in Eq. (iii),
2u + 12(1/40) = ½
2u = 2/10
u = 1/10
x = 1/u = 10km/hr
y = 1/v = 40km/hr
Hence, the speed of rickshaw = 10 km/h
And the speed of the bus = 40 km/h.
Also, BYJU’S provides Class 10 Maths NCERT Solutions, notes, exemplar books, question papers and some preparation tips and tricks so that students can score good marks in Maths subject. They are also suggested to solve sample papers and previous years’ question papers to get a clear idea of the question pattern and marking scheme for the chapter, Pair of Linear Equations in Two Variables.
The exemplar comes with detailed explanations to help students grasp the core concepts and get the right insight into how the problems should be handled. With the exemplar solutions, students will be able to study smartly, develop better Maths skills and ultimately be prepared to solve any type of question that may be asked in the board exams. Get updated study materials to study well, and also download BYJU’S – The Learning App to experience a new method of learning with the help of educational videos clearing the concepts of Maths topics in a visual way.
Frequently Asked Questions on NCERT Exemplar Solutions for Class 10 Maths Chapter 3
What are the main topics covered in NCERT Exemplar Solutions for Class 10 Maths Chapter 3?
1. Algebraic and geometrical representation of Pair of Linear Equations in Two Variables
2. Graphical methods of solving a pair of linear equations
3. Algebraic methods of solving a pair of linear equations such as substitution method, elimination method, cross-multiplication method
4. Reducing the pair of equations into a linear form
These topics are explained in simple language to help students score well in the board exams, irrespective of their intelligence levels.
What is the substitution method according to NCERT Exemplar Solutions for Class 10 Maths Chapter 3?
Does NCERT Exemplar Solutions for Class 10 Maths Chapter 3 help students score well in the board exams?
| Also Access |
| NCERT Solutions for Class 10 Maths Chapter 3 |
| CBSE Notes for Class 10 Maths Chapter 3 |
Also, Read

























Comments