RD Sharma Solutions for Class 11 Maths Chapter 13 Complex Numbers

RD Sharma Solutions Class 11 Maths Chapter 13 – Get Free PDF (for 2023 – 2024)

RD Sharma Solutions for Class 11 Maths Chapter 13 – Complex Numbers are provided here for students to prepare and score good marks in the board exams. The combination of a real number and an imaginary number is termed a complex number. All the concepts related to complex numbers are explained in this chapter with suitable examples. The RD Sharma Solutions for Class 11 Maths are formulated by experts at BYJU’S after conducting vast research on each concept. 

Chapter 13 – Complex Numbers contains four exercises, and the RD Sharma Solutions provide precise solutions to the questions present in each exercise. Students who find difficulty in solving problems can quickly download the RD Sharma Class 11 Maths Solutions in PDF format from the links given below and can start practising offline for good results. Now, let us have a look at the concepts discussed in this chapter.

• Integral Powers of IOTA (i)
• Imaginary quantities
• Complex numbers
• Equality of complex numbers
• Addition of complex numbers
  • Properties of addition of complex numbers
• Subtraction of complex numbers
• Multiplication of complex numbers
  • Properties of multiplication
• Division of complex numbers
• Conjugate of complex numbers
• Modulus of a complex number
  • Properties of modulus
• Reciprocal of a complex number
• Square roots of a complex number
• Representation of a complex number
  • Geometrical representation of a complex number
  • Argument or amplitude of a complex number for different signs of real and imaginary parts
  • Vectorial representation of a complex number
  • Polar or trigonometrical form of a complex number
  • Multiplication of a complex number by IOTA
  • The polar form of a complex number for different signs of real and imaginary parts

Download the PDF of RD Sharma Solutions for Class 11 Maths Chapter 13 – Complex Numbers

Access answers to RD Sharma Solutions for Class 11 Maths Chapter 13 – Complex Numbers

EXERCISE 13.1 PAGE NO: 13.3

1. Evaluate the following:

(i) i ⁴⁵⁷

(ii) i ⁵²⁸

(iii) 1/ i⁵⁸

(iv) i ³⁷ + 1/i ⁶⁷

(v) [i ⁴¹ + 1/i ²⁵⁷]

(vi) (i ⁷⁷ + i ⁷⁰ + i ⁸⁷ + i ⁴¹⁴)³

(vii) i ³⁰ + i ⁴⁰ + i ⁶⁰

(viii) i ⁴⁹ + i ⁶⁸ + i ⁸⁹ + i ¹¹⁰

Solution:

(i) i ⁴⁵⁷

Let us simplify, and we get,

i⁴⁵⁷ = i ^{(456 + 1)}

= i ⁴⁽¹¹⁴⁾ × i

= (1)¹¹⁴ × i

= i [since i⁴ = 1]

(ii) i ⁵²⁸

Let us simplify, and we get,

i ⁵²⁸ = i ⁴⁽¹³²⁾

= (1)¹³²

= 1 [since i⁴ = 1]

(iii) 1/ i⁵⁸

Let us simplify, and we get,

1/ i⁵⁸ = 1/ i ⁵⁶⁺²

= 1/ i ⁵⁶ × i²

= 1/ (i⁴)¹⁴ × i²

= 1/ i² [since, i⁴ = 1]

= 1/-1 [since, i² = -1]

= -1

(iv) i ³⁷ + 1/i ⁶⁷

Let us simplify, and we get,

i ³⁷ + 1/i ⁶⁷ = i³⁶⁺¹ + 1/ i⁶⁴⁺³

= i + 1/i³ [since, i⁴ = 1]

= i + i/i⁴

= i + i

= 2i

(v) [i ⁴¹ + 1/i ²⁵⁷]

Let us simplify, and we get,

= [i + 1/i]⁹ [since, 1/i = -1]

= [i – i]

= 0

(vi) (i ⁷⁷ + i ⁷⁰ + i ⁸⁷ + i ⁴¹⁴)³

Let us simplify, and we get,

(i ⁷⁷ + i ⁷⁰ + i ⁸⁷ + i ⁴¹⁴)³ = (i^{(76 + 1)} + i^{(68 + 2)} + i^{(84 + 3)} + i^{(412 + 2)} ) ³

= (i + i² + i³ + i²)³ [since i³ = – i, i² = – 1]

= (i + (– 1) + (– i) + (– 1)) ³

= (– 2)³

= – 8

(vii) i ³⁰ + i ⁴⁰ + i ⁶⁰

Let us simplify, and we get,

i ³⁰ + i ⁴⁰ + i ⁶⁰ = i^{(28 + 2) +} i⁴⁰ + i⁶⁰

= (i⁴)⁷ i² + (i⁴)¹⁰ + (i⁴)¹⁵

= i² + 1¹⁰ + 1¹⁵

= – 1 + 1 + 1

= 1

(viii) i ⁴⁹ + i ⁶⁸ + i ⁸⁹ + i ¹¹⁰

Let us simplify, and we get,

i ⁴⁹ + i ⁶⁸ + i ⁸⁹ + i ¹¹⁰ = i^{(48 + 1)} + i⁶⁸ + i^{(88 + 1)} + i^{(116 + 2)}

= (i⁴)¹²×i + (i⁴)¹⁷ + (i⁴)²²×i + (i⁴)²⁹×i²

= i + 1 + i – 1

= 2i

2. Show that 1 + i¹⁰ + i²⁰ + i³⁰ is a real number.

Solution:

Given:

1 + i¹⁰ + i²⁰ + i³⁰ = 1 + i^{(8 + 2) +} i²⁰ + i^{(28 + 2)}

= 1 + (i⁴)² × i² + (i⁴)⁵ + (i⁴)⁷ × i²

= 1 – 1 + 1 – 1 [since, i⁴ = 1, i² = – 1]

= 0

Hence, 1 + i¹⁰ + i²⁰ + i³⁰ is a real number.

3. Find the values of the following expressions:

(i) i⁴⁹ + i⁶⁸ + i⁸⁹ + i¹¹⁰

(ii) i³⁰ + i⁸⁰ + i¹²⁰

(iii) i + i² + i³ + i⁴

(iv) i⁵ + i¹⁰ + i¹⁵

(v) [i⁵⁹² + i⁵⁹⁰ + i⁵⁸⁸ + i⁵⁸⁶ + i⁵⁸⁴] / [i⁵⁸² + i⁵⁸⁰ + i⁵⁷⁸ + i⁵⁷⁶ + i⁵⁷⁴]

(vi) 1 + i² + i⁴ + i⁶ + i⁸ + … + i²⁰

(vii) (1 + i)⁶ + (1 – i)³

Solution:

(i) i⁴⁹ + i⁶⁸ + i⁸⁹ + i¹¹⁰

Let us simplify, and we get,

i⁴⁹ + i⁶⁸ + i⁸⁹ + i¹¹⁰ = i ^{(48 + 1)} + i⁶⁸ + i^{(88 + 1)} + i^{(108 + 2)}

= (i⁴)¹² × i + (i⁴)¹⁷ + (i⁴)²² × i + (i⁴)²⁷ × i²

= i + 1 + i – 1 [since i⁴ = 1, i² = – 1]

= 2i

∴ i⁴⁹ + i⁶⁸ + i⁸⁹ + i¹¹⁰ = 2i

(ii) i³⁰ + i⁸⁰ + i¹²⁰

Let us simplify, and we get,

i³⁰ + i⁸⁰ + i¹²⁰ = i^{(28 + 2)} + i⁸⁰ + i¹²⁰

= (i⁴)⁷ × i² + (i⁴)²⁰ + (i⁴)³⁰

= – 1 + 1 + 1 [since i⁴ = 1, i² = – 1]

= 1

∴ i³⁰ + i⁸⁰ + i¹²⁰ = 1

(iii) i + i² + i³ + i⁴

Let us simplify, and we get,

i + i² + i³ + i⁴ = i + i² + i²×i + i⁴

= i – 1 + (– 1) × i + 1 [since i⁴ = 1, i² = – 1]

= i – 1 – i + 1

= 0

∴ i + i² + i³ + i⁴ = 0

(iv) i⁵ + i¹⁰ + i¹⁵

Let us simplify, and we get,

i⁵ + i¹⁰ + i¹⁵ = i^{(4 + 1)} + i^{(8 + 2)} + i^{(12 + 3)}

= (i⁴)¹×i + (i⁴)²×i² + (i⁴)³×i³

= (i⁴)¹×i + (i⁴)²×i² + (i⁴)³×i²×i

= 1×i + 1 × (– 1) + 1 × (– 1)×i

= i – 1 – i

= – 1

∴ i⁵ + i¹⁰ + i¹⁵ = -1

(v) [i⁵⁹² + i⁵⁹⁰ + i⁵⁸⁸ + i⁵⁸⁶ + i⁵⁸⁴] / [i⁵⁸² + i⁵⁸⁰ + i⁵⁷⁸ + i⁵⁷⁶ + i⁵⁷⁴]

Let us simplify, and we get,

= [i¹⁰ (i⁵⁸² + i⁵⁸⁰ + i⁵⁷⁸ + i⁵⁷⁶ + i⁵⁷⁴) / (i⁵⁸² + i⁵⁸⁰ + i⁵⁷⁸ + i⁵⁷⁶ + i⁵⁷⁴)]

= i¹⁰

= i⁸ i²

= (i⁴)² i²

= (1)² (-1) [since i⁴ = 1, i² = -1]

= -1 

∴ [i⁵⁹² + i⁵⁹⁰ + i⁵⁸⁸ + i⁵⁸⁶ + i⁵⁸⁴] / [i⁵⁸² + i⁵⁸⁰ + i⁵⁷⁸ + i⁵⁷⁶ + i⁵⁷⁴] = -1

(vi) 1 + i² + i⁴ + i⁶ + i⁸ + … + i²⁰

Let us simplify, and we get,

1 + i² + i⁴ + i⁶ + i⁸ + … + i²⁰ = 1 + (– 1) + 1 + (– 1) + 1 + … + 1

= 1

∴ 1 + i² + i⁴ + i⁶ + i⁸ + … + i²⁰ = 1

(vii) (1 + i)⁶ + (1 – i)³

Let us simplify, and we get,

(1 + i)⁶ + (1 – i)³ = {(1 + i)² }³ + (1 – i)² (1 – i)

= {1 + i² + 2i}³ + (1 + i^{2 –} 2i)(1 – i)

= {1 – 1 + 2i}³ + (1 – 1 – 2i)(1 – i)

= (2i)³ + (– 2i)(1 – i)

= 8i³ + (– 2i) + 2i²

= – 8i – 2i – 2 [since i³ = – i, i² = – 1]

= – 10 i – 2

= – 2(1 + 5i)

= – 2 – 10i

∴ (1 + i)⁶ + (1 – i)³ = – 2 – 10i

EXERCISE 13.2 PAGE NO: 13.31

1. Express the following complex numbers in the standard form a + ib.

(i) (1 + i) (1 + 2i)

(ii) (3 + 2i) / (-2 + i)

(iii) 1/(2 + i)²

(iv) (1 – i) / (1 + i)

(v) (2 + i)³ / (2 + 3i)

(vi) [(1 + i) (1 +√3i)] / (1 – i)

(vii) (2 + 3i) / (4 + 5i)

(viii) (1 – i)³ / (1 – i³)

(ix) (1 + 2i)^-3

(x) (3 – 4i) / [(4 – 2i) (1 + i)]

(xi)

(xii) (5 +√2i) / (1-√2i)

Solution:

(i) (1 + i) (1 + 2i)

Let us simplify and express in the standard form of (a + ib).

(1 + i) (1 + 2i) = (1+i)(1+2i)

= 1(1+2i)+i(1+2i)

= 1+2i+i+2i²

= 1+3i+2(-1) [since, i² = -1]

= 1+3i-2

= -1+3i

∴ The values of a and b are -1 and 3.

(ii) (3 + 2i) / (-2 + i)

Let us simplify and express in the standard form of (a + ib).

(3 + 2i) / (-2 + i) = [(3 + 2i) / (-2 + i)] × (-2-i) / (-2-i) [multiply and divide with (-2-i)]

= [3(-2-i) + 2i (-2-i)] / [(-2)² – (i)²]

= [-6 -3i – 4i -2i²] / (4-i²)

= [-6 -7i -2(-1)] / (4 – (-1)) [since, i² = -1]

= [-4 -7i] / 5

∴ The values of a, b are -4/5, -7/5

(iii) 1/(2 + i)²

Let us simplify and express in the standard form of (a + ib).

1/(2 + i)² = 1/(2² + i² + 2(2) (i))

= 1/ (4 – 1 + 4i) [since, i² = -1]

= 1/(3 + 4i) [multiply and divide with (3 – 4i)]

= 1/(3 + 4i) × (3 – 4i)/ (3 – 4i)]

= (3-4i)/ (3² – (4i)²)

= (3-4i)/ (9 – 16i²)

= (3-4i)/ (9 – 16(-1)) [since, i² = -1]

= (3-4i)/25

∴ The values of a and b are 3/25 and -4/25

(iv) (1 – i) / (1 + i)

Let us simplify and express in the standard form of (a + ib).

(1 – i) / (1 + i) = (1 – i) / (1 + i) × (1-i)/(1-i) [multiply and divide with (1-i)]

= (1² + i² – 2(1)(i)) / (1² – i²)

= (1 + (-1) -2i) / (1 – (-1))

= -2i/2

= -i

∴ The values of a and b are 0 and -1

(v) (2 + i)³ / (2 + 3i)

Let us simplify and express in the standard form of (a + ib).

(2 + i)³ / (2 + 3i) = (2³ + i³ + 3(2)²(i) + 3(i)²(2)) / (2 + 3i)

= (8 + (i².i) + 3(4)(i) + 6i²) / (2 + 3i)

= (8 + (-1)i + 12i + 6(-1)) / (2 + 3i)

= (2 + 11i) / (2 + 3i)

= (2 + 11i)/(2 + 3i) × (2-3i)/(2-3i)

= [2(2-3i) + 11i(2-3i)] / (2² – (3i)²)

= (4 – 6i + 22i – 33i²) / (4 – 9i²)

= (4 + 16i – 33(-1)) / (4 – 9(-1)) [since, i² = -1]

= (37 + 16i) / 13

∴ The values of a and b are 37/13 and 16/13

(vi) [(1 + i) (1 +√3i)] / (1 – i)

Let us simplify and express in the standard form of (a + ib).

= (1 + √3i + i + √3i²) / (1 – i)

= (1 + (√3+1)i + √3(-1)) / (1-i) [since, i² = -1]

= [(1-√3) + (1+√3)i] / (1-i)

= [(1-√3) + (1+√3)i] / (1-i) × (1+i)/(1+i)

= [(1-√3) (1+i) + (1+√3)i(1+i)] / (1² – i²)

= [1-√3+ (1-√3)i + (1+√3)i + (1+√3)i²] / (1-(-1)) [since, i² = -1]

= [(1-√3)+(1-√3+1+√3)i+(1+√3)(-1)] / 2

= (-2√3 + 2i) / 2

= -√3 + i

∴ The values of a and b are -√3 and 1

(vii) (2 + 3i) / (4 + 5i)

Let us simplify and express in the standard form of (a + ib).

(2 + 3i) / (4 + 5i) = [multiply and divide with (4-5i)]

= (2 + 3i) / (4 + 5i) × (4-5i)/(4-5i)

= [2(4-5i) + 3i(4-5i)] / (4² – (5i)²)

= [8 – 10i + 12i – 15i²] / (16 – 25i²)

= [8+2i-15(-1)] / (16 – 25(-1)) [since, i² = -1]

= (23 + 2i) / 41

∴ The values of a and b are 23/41 and 2/41

(viii) (1 – i)³ / (1 – i³)

Let us simplify and express in the standard form of (a + ib).

(1 – i)³ / (1 – i³) = [1³ – 3(1)²i + 3(1)(i)² – i³] / (1-i².i)

= [1 – 3i + 3(-1)-i².i] / (1 – (-1)i) [since, i² = -1]

= [-2 – 3i – (-1)i] / (1+i)

= [-2-4i] / (1+i)

= [-2-4i] / (1+i) × (1-i)/(1-i)

= [-2(1-i)-4i(1-i)] / (1² – i²)

= [-2+2i-4i+4i²] / (1 – (-1))

= [-2-2i+4(-1)] /2

= (-6-2i)/2

= -3 – i

∴ The values of a and b are -3 and -1

(ix) (1 + 2i)^-3

Let us simplify and express in the standard form of (a + ib).

(1 + 2i)^-3 = 1/(1 + 2i)³

= 1/(1³+3(1)² (2i)+2(1)(2i)² + (2i)³)

= 1/(1+6i+4i²+8i³)

= 1/(1+6i+4(-1)+8i².i) [since, i² = -1]

= 1/(-3+6i+8(-1)i) [since, i² = -1]

= 1/(-3-2i)

= -1/(3+2i)

= -1/(3+2i) × (3-2i)/(3-2i)

= (-3+2i)/(3² – (2i)²)

= (-3+2i) / (9-4i²)

= (-3+2i) / (9-4(-1))

= (-3+2i) /13

∴ The values of a and b are -3/13 and 2/13

(x) (3 – 4i) / [(4 – 2i) (1 + i)]

Let us simplify and express in the standard form of (a + ib).

(3 – 4i) / [(4 – 2i) (1 + i)] = (3-4i)/ [4(1+i)-2i(1+i)]

= (3-4i)/ [4+4i-2i-2i²]

= (3-4i)/ [4+2i-2(-1)] [since, i² = -1]

= (3-4i)/ (6+2i)

= (3-4i)/ (6+2i) × (6-2i)/(6-2i)

= [3(6-2i)-4i(6-2i)] / (6² – (2i)²)

= [18 – 6i – 24i + 8i²] / (36 – 4i²)

= [18 – 30i + 8 (-1)] / (36 – 4 (-1)) [since, i² = -1]

= [10-30i] / 40

= (1 – 3i) / 4

∴ The values of a and b are 1/4 and -3/4

(xi)

(xii) (5 +√2i) / (1-√2i)

Let us simplify and express in the standard form of (a + ib).

(5 +√2i) / (1-√2i) = [Multiply and divide with (1+√2i)]

= (5 +√2i) / (1-√2i) × (1+√2i)/(1+√2i)

= [5(1+√2i) + √2i(1+√2i)] / (1² – (√2)²)

= [5+5√2i + √2i + 2i²] / (1 – 2i²)

= [5 + 6√2i + 2(-1)] / (1-2(-1)) [since, i² = -1]

= [3+6√2i]/3

= 1+ 2√2i

∴ The values of a and b are 1 and 2√2

2. Find the real values of x and y, if

(i) (x + iy) (2 – 3i) = 4 + i

(ii) (3x – 2i y) (2 + i)² = 10(1 + i)

(iv) (1 + i) (x + iy) = 2 – 5i

Solution:

(i) (x + iy) (2 – 3i) = 4 + i

Given:

(x + iy) (2 – 3i) = 4 + i

Let us simplify the expression, and we get,

x(2 – 3i) + iy(2 – 3i) = 4 + i

2x – 3xi + 2yi – 3yi² = 4 + i

2x + (-3x+2y)i – 3y (-1) = 4 + i [since, i² = -1]

2x + (-3x+2y)i + 3y = 4 + i [since, i² = -1]

(2x+3y) + i(-3x+2y) = 4 + i

Equating Real and Imaginary parts on both sides, we get

2x+3y = 4… (i)

And -3x+2y = 1… (ii)

Multiply (i) by 3 and (ii) by 2 and add

On solving, we get,

6x – 6x – 9y + 4y = 12 + 2

13y = 14

y = 14/13

Substitute the value of y in (i), and we get,

2x+3y = 4

2x + 3(14/13) = 4

2x = 4 – (42/13)

= (52-42)/13

2x = 10/13

x = 5/13

x = 5/13, y = 14/13

∴ The real values of x and y are 5/13 and 14/13

(ii) (3x – 2i y) (2 + i)² = 10(1 + i)

Given:

(3x – 2iy) (2+i)² = 10(1+i)

(3x – 2yi) (2²+i²+2(2)(i)) = 10+10i

(3x – 2yi) (4 + (-1)+4i) = 10+10i [since, i² = -1]

(3x – 2yi) (3+4i) = 10+10i

Let us divide with 3+4i in both sides, and we get,

(3x – 2yi) = (10+10i)/(3+4i)

= Now multiply and divide with (3-4i)

= [10(3-4i) + 10i(3-4i)] / (3² – (4i)²)

= [30-40i+30i-40i²] / (9 – 16i²)

= [30-10i-40(-1)] / (9-16(-1))

= [70-10i]/25

Now, equating Real and Imaginary parts on both sides, we get

3x = 70/25 and -2y = -10/25

x = 70/75 and y = 1/5

x = 14/15 and y = 1/5

∴ The real values of x and y are 14/15 and 1/5

(4+2i) x-3i-3 + (9-7i)y = 10i

(4x+9y-3) + i(2x-7y-3) = 10i

Now, equating Real and Imaginary parts on both sides, we get,

4x+9y-3 = 0 … (i)

And 2x-7y-3 = 10

2x-7y = 13 … (ii)

Multiply (i) by 7 and (ii) by 9 and add.

On solving these equations, we get

28x + 18x + 63y – 63y = 117 + 21

46x = 117 + 21

46x = 138

x = 138/46

= 3

Substitute the value of x in (i), and we get,

4x+9y-3 = 0

9y = -9

y = -9/9

= -1

x = 3 and y = -1

∴ The real values of x and y are 3 and -1

(iv) (1 + i) (x + iy) = 2 – 5i

Given:

(1 + i) (x + iy) = 2 – 5i

Divide with (1+i) on both sides, and we get,

(x + iy) = (2 – 5i)/(1+i)

Multiply and divide by (1-i).

= (2 – 5i)/(1+i) × (1-i)/(1-i)

= [2(1-i) – 5i (1-i)] / (1² – i²)

= [2 – 7i + 5(-1)] / 2 [since, i² = -1]

= (-3-7i)/2

Now, equating Real and Imaginary parts on both sides, and we get

x = -3/2 and y = -7/2

∴ The real values of x and y are -3/2, -7/2

3. Find the conjugates of the following complex numbers:

(i) 4 – 5i

(ii) 1 / (3 + 5i)

(iii) 1 / (1 + i)

(iv) (3 – i)² / (2 + i)

(v) [(1 + i) (2 + i)] / (3 + i)

(vi) [(3 – 2i) (2 + 3i)] / [(1 + 2i) (2 – i)]

Solution:

(i) 4 – 5i

Given:

4 – 5i

We know the conjugate of a complex number (a + ib) is (a – ib).

So,

∴ The conjugate of (4 – 5i) is (4 + 5i)

(ii) 1 / (3 + 5i)

Given:

1 / (3 + 5i)

Since the given complex number is not in the standard form of (a + ib),

Let us convert to standard form by multiplying and dividing with (3 – 5i).

We get,

We know the conjugate of a complex number (a + ib) is (a – ib).

So,

∴ The conjugate of (3 – 5i)/34 is (3 + 5i)/34

(iii) 1 / (1 + i)

Given:

1 / (1 + i)

Since the given complex number is not in the standard form of (a + ib),

Let us convert to standard form by multiplying and dividing with (1 – i).

We get,

We know the conjugate of a complex number (a + ib) is (a – ib).

So,

∴ The conjugate of (1-i)/2 is (1+i)/2

(iv) (3 – i)² / (2 + i)

Given:

(3 – i)² / (2 + i)

Since the given complex number is not in the standard form of (a + ib),

Let us convert to standard form.

We know the conjugate of a complex number (a + ib) is (a – ib).

So,

∴ The conjugate of (2 – 4i) is (2 + 4i)

(v) [(1 + i) (2 + i)] / (3 + i)

Given:

Since the given complex number is not in the standard form of (a + ib),

Let us convert to standard form.

We know the conjugate of a complex number (a + ib) is (a – ib).

So,

∴ The conjugate of (3 + 4i)/5 is (3 – 4i)/5

(vi) [(3 – 2i) (2 + 3i)] / [(1 + 2i) (2 – i)]

Given:

Since the given complex number is not in the standard form of (a + ib),

Let us convert to standard form.

We know the conjugate of a complex number (a + ib) is (a – ib).

So,

∴ The conjugate of (63 – 16i)/25 is (63 + 16i)/25

4. Find the multiplicative inverse of the following complex numbers:

(i) 1 – i

(ii) (1 + i √3)²

(iii) 4 – 3i

(iv) √5 + 3i

Solution:

(i) 1 – i

Given:

1 – i

We know the multiplicative inverse of a complex number (Z) is Z^-1 or 1/Z

So,

∴ The multiplicative inverse of (1 – i) is (1 + i)/2

(ii) (1 + i √3)²

Given:

(1 + i √3)²

Z = (1 + i √3)²

= 1² + (i √3)² + 2 (1) (i√3)

= 1 + 3i² + 2 i√3

= 1 + 3(-1) + 2 i√3 [since, i² = -1]

= 1 – 3 + 2 i√3

= -2 + 2 i√3

We know the multiplicative inverse of a complex number (Z) is Z^-1 or 1/Z

So,

Z = -2 + 2 i√3

∴ The multiplicative inverse of (1 + i√3)² is (-1-i√3)/8

(iii) 4 – 3i

Given:

4 – 3i

We know the multiplicative inverse of a complex number (Z) is Z^-1 or 1/Z

So,

Z = 4 – 3i

∴ The multiplicative inverse of (4 – 3i) is (4 + 3i)/25

(iv) √5 + 3i

Given:

√5 + 3i

We know the multiplicative inverse of a complex number (Z) is Z^-1 or 1/Z

So,

Z = √5 + 3i

∴ The multiplicative inverse of (√5 + 3i) is (√5 – 3i)/14

6. If z₁ = (2 – i), z₂ = (-2 + i), find

Solution:

Given:

z₁ = (2 – i) and z₂ = (-2 + i)

7. Find the modulus of [(1 + i)/(1 – i)] – [(1 – i)/(1 + i)]

Solution:

Given:

So,

Z = [(1 + i)/(1 – i)] – [(1 – i)/(1 + i)]

Let us simplify, and we get

= [(1+i) (1+i) – (1-i) (1-i)] / (1² – i²)

= [1² + i² + 2(1)(i) – (1² + i² – 2(1)(i))] / (1 – (-1)) [Since, i² = -1]

= 4i/2

= 2i

We know that for a complex number Z = (a+ib) it’s magnitude is given by |z| = √(a² + b²)

So,

|Z| = √(0² + 2²)

= 2

∴ The modulus of [(1 + i)/(1 – i)] – [(1 – i)/(1 + i)] is 2.

8. If x + iy = (a+ib)/(a-ib), prove that x² + y² = 1

Solution:

Given:

x + iy = (a+ib)/(a-ib)

We know that for a complex number Z = (a+ib) it’s magnitude is given by |z| = √(a² + b²)

So,

|a/b| is |a| / |b|

Applying Modulus on both sides, we get,

9. Find the least positive integral value of n for which [(1+i)/(1-i)]ⁿ is real.

Solution:

Given:

Z = [(1+i)/(1-i)]ⁿ

Now, let us multiply and divide by (1+i), and we get

= i [which is not real]

For n = 2, we have

= -1 [which is real]

So, the smallest positive integral ‘n’ that can make [(1+i)/(1-i)]ⁿ real is 2.

∴ The smallest positive integral value of ‘n’ is 2.

10. Find the real values of θ for which the complex number (1 + i cos θ) / (1 – 2i cos θ) is purely real.

Solution:

Given:

(1 + i cos θ) / (1 – 2i cos θ)

Z = (1 + i cos θ) / (1 – 2i cos θ)

Let us multiply and divide by (1 + 2i cos θ)

For a complex number to be purely real, the imaginary part should be equal to zero.

So,

3cos θ = 0 (since, 1 + 4cos²θ ≥ 1)

cos θ = 0

cos θ = cos π/2

θ = [(2n+1)π] / 2, for n ∈ Z

= 2nπ ± π/2, for n ∈ Z

∴ The values of θ to get the complex number to be purely real is 2nπ ± π/2, for n ∈ Z

11. Find the smallest positive integer value of n for which (1+i) ⁿ / (1-i) ^n-2 is a real number.

Solution:

Given:

(1+i) ⁿ / (1-i) ^n-2

Z = (1+i) ⁿ / (1-i) ^n-2

Let us multiply and divide by (1 – i)²

For n = 1,

Z = -2i¹⁺¹

= -2i²

= 2, which is a real number.

∴ The smallest positive integer value of n is 1.

12. If [(1+i)/(1-i)]³ – [(1-i)/(1+i)]³ = x + iy, find (x, y)

Solution:

Given:

Let us rationalise the denominator, we get

i³–(-i)³ = x + iy

2i³ = x + iy

2i².i = x + iy

2(-1)I = x + iy

-2i = x + iy

Equating Real and Imaginary parts on both sides, we get

x = 0 and y = -2

∴ The values of x and y are 0 and -2.

13. If (1+i)² / (2-i) = x + iy, find x + y

Solution:

Given:

(1+i)² / (2-i) = x + iy

Upon expansion, we get,

Let us equate real and imaginary parts on both sides we get,

x = -2/5 and y = 4/5

so,

x + y = -2/5 + 4/5

= (-2+4)/5

= 2/5

∴ The value of (x + y) is 2/5

EXERCISE 13.3 PAGE NO: 13.39

1. Find the square root of the following complex numbers.

(i) – 5 + 12i

(ii) -7 – 24i

(iii) 1 – i

(iv) – 8 – 6i

(v) 8 – 15i

(vi) -11 – 60√-1

(vii) 1 + 4√-3

(viii) 4i

(ix) -i

Solution:

(i) – 5 + 12i

Given:

– 5 + 12i

We know, Z = a + ib

So, √(a + ib) = √(-5+12i)

Here, b > 0

Let us simplify now,

∴ Square root of (– 5 + 12i) is ±[2 + 3i]

(ii) -7 – 24i

Given:

-7 – 24i

We know, Z = -7 – 24i

So, √(a + ib) = √(-7 – 24i)

Here, b < 0

Let us simplify now,

∴ Square root of (-7 – 24i) is ± [3 – 4i]

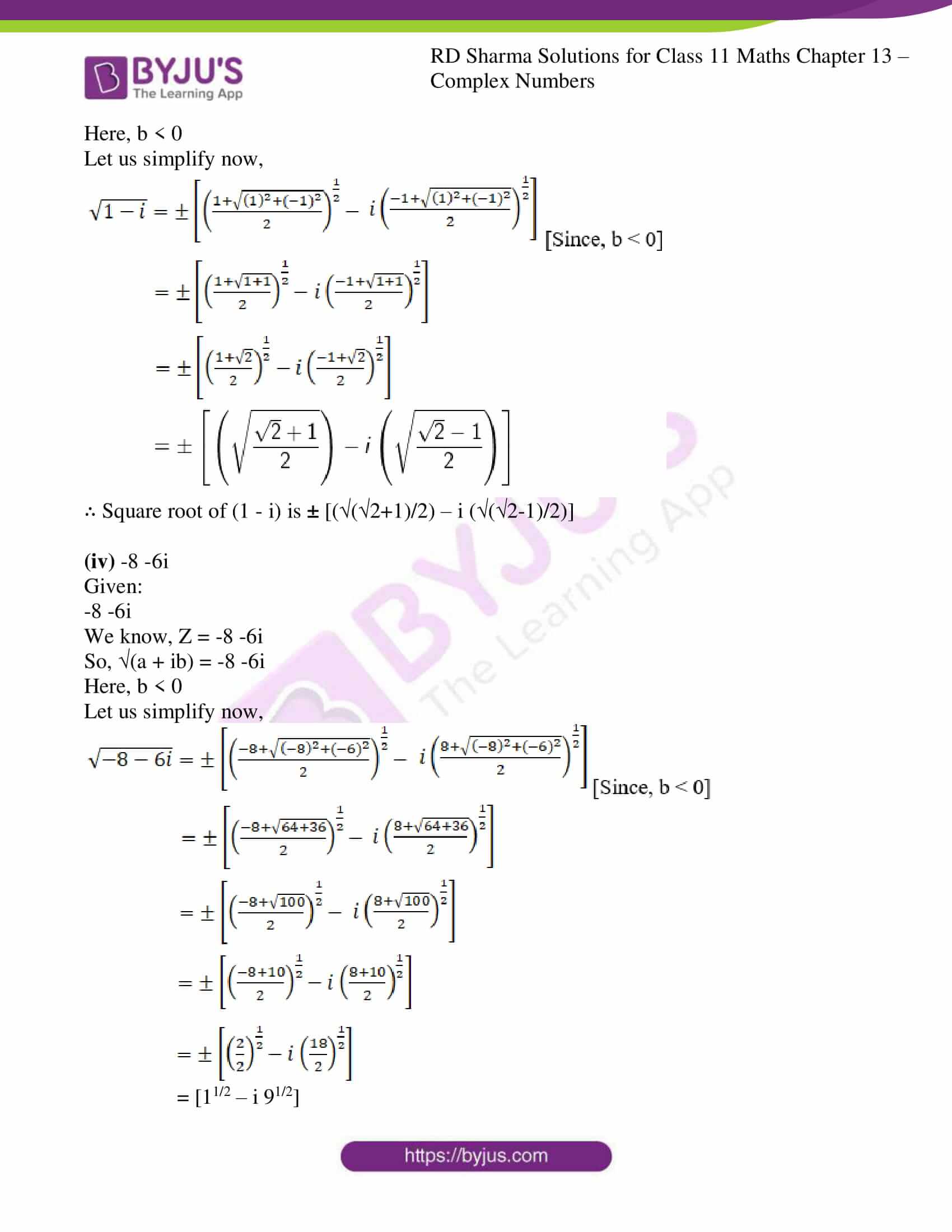
(iii) 1 – i

Given:

1 – i

We know, Z = (1 – i)

So, √(a + ib) = √(1 – i)

Here, b < 0

Let us simplify now.

∴ The square root of (1 – i) is ± [(√(√2+1)/2) – i (√(√2-1)/2)]

(iv) -8 -6i

Given:

-8 -6i

We know, Z = -8 -6i

So, √(a + ib) = -8 -6i

Here, b < 0

Let us simplify now.

= [1^1/2 – i 9^1/2]

= ± [1 – 3i]

∴ The square root of (-8 -6i) is ± [1 – 3i]

(v) 8 – 15i

Given:

8 – 15i

We know, Z = 8 – 15i

So, √(a + ib) = 8 – 15i

Here, b < 0

Let us simplify now.

∴ The square root of (8 – 15i) is ± 1/√2 (5 – 3i)

(vi) -11 – 60√-1

Given:

-11 – 60√-1

We know, Z = -11 – 60√-1

So, √(a + ib) = -11 – 60√-1

= -11 – 60i

Here, b < 0

Let us simplify now.

∴ The square root of (-11 – 60√-1) is ± (5 – 6i)

(vii) 1 + 4√-3

Given:

1 + 4√-3

We know, Z = 1 + 4√-3

So, √(a + ib) = 1 + 4√-3

= 1 + 4(√3) (√-1)

= 1 + 4√3i

Here, b > 0

Let us simplify now.

∴ The square root of (1 + 4√-3) is ± (2 + √3i)

(viii) 4i

Given:

4i

We know, Z = 4i

So, √(a + ib) = 4i

Here, b > 0

Let us simplify now.

∴ The square root of 4i is ± √2 (1 + i)

(ix) –i

Given:

-i

We know, Z = -i

So, √(a + ib) = -i

Here, b < 0

Let us simplify now.

∴ The square root of –i is ± 1/√2 (1 – i)

EXERCISE 13.4 PAGE NO: 13.57

1. Find the modulus and arguments of the following complex numbers and hence express each of them in the polar form.

(i) 1 + i

(ii) √3 + i

(iii) 1 – i

(iv) (1 – i) / (1 + i)

(v) 1/(1 + i)

(vi) (1 + 2i) / (1 – 3i)

(vii) sin 120^o – i cos 120^o

(viii) -16 / (1 + i√3)

Solution:

We know that the polar form of a complex number Z = x + iy is given by Z = |Z| (cos θ + i sin θ)

Where,

|Z| = modulus of complex number = √(x² + y²)

θ = arg (z) = argument of complex number = tan^-1 (|y| / |x|)

(i) 1 + i

Given: Z = 1 + i

So now,

|Z| = √(x² + y²)

= √(1² + 1²)

= √(1 + 1)

= √2

θ = tan^-1 (|y| / |x|)

= tan^-1 (1 / 1)

= tan^-1 1

Since x > 0, y > 0 complex number lies in 1^st quadrant and the value of θ is 0⁰≤θ≤90⁰.

θ = π/4

Z = √2 (cos (π/4) + i sin (π/4))

∴ The polar form of (1 + i) is √2 (cos (π/4) + i sin (π/4))

(ii) √3 + i

Given: Z = √3 + i

So now,

|Z| = √(x² + y²)

= √((√3)² + 1²)

= √(3 + 1)

= √4

= 2

θ = tan^-1 (|y| / |x|)

= tan^-1 (1 / √3)

Since x > 0, y > 0 complex number lies in 1^st quadrant and the value of θ is 0⁰≤θ≤90⁰.

θ = π/6

Z = 2 (cos (π/6) + i sin (π/6))

∴ The polar form of (√3 + i) is 2 (cos (π/6) + i sin (π/6))

(iii) 1 – i

Given: Z = 1 – i

So now,

|Z| = √(x² + y²)

= √(1² + (-1)²)

= √(1 + 1)

= √2

θ = tan^-1 (|y| / |x|)

= tan^-1 (1 / 1)

= tan^-1 1

Since x > 0, y < 0 complex number lies in the 4^th quadrant, and the value of θ is -90⁰≤θ≤0⁰.

θ = -π/4

Z = √2 (cos (-π/4) + i sin (-π/4))

= √2 (cos (π/4) – i sin (π/4))

∴ The polar form of (1 – i) is √2 (cos (π/4) – i sin (π/4))

(iv) (1 – i) / (1 + i)

Given: Z = (1 – i) / (1 + i)

Let us multiply and divide by (1 – i), and we get

= 0 – i

So now,

|Z| = √(x² + y²)

= √(0² + (-1)²)

= √(0 + 1)

= √1

θ = tan^-1 (|y| / |x|)

= tan^-1 (1 / 0)

= tan^-1 ∞

Since x ≥ 0, y < 0 complex number lies in the 4^th quadrant, and the value of θ is -90⁰≤θ≤0⁰.

θ = -π/2

Z = 1 (cos (-π/2) + i sin (-π/2))

= 1 (cos (π/2) – i sin (π/2))

∴ The polar form of (1 – i) / (1 + i) is 1 (cos (π/2) – i sin (π/2))

(v) 1/(1 + i)

Given: Z = 1 / (1 + i)

Let us multiply and divide by (1 – i), and we get

So now,

|Z| = √(x² + y²)

= √((1/2)² + (-1/2)²)

= √(1/4 + 1/4)

= √(2/4)

= 1/√2

θ = tan^-1 (|y| / |x|)

= tan^-1 ((1/2) / (1/2))

= tan^-1 1

Since x > 0, y < 0 complex number lies in the 4^th quadrant, and the value of θ is -90⁰≤θ≤0⁰.

θ = -π/4

Z = 1/√2 (cos (-π/4) + i sin (-π/4))

= 1/√2 (cos (π/4) – i sin (π/4))

∴ Polar form of 1/(1 + i) is 1/√2 (cos (π/4) – i sin (π/4))

(vi) (1 + 2i) / (1 – 3i)

Given: Z = (1 + 2i) / (1 – 3i)

Let us multiply and divide by (1 + 3i), and we get

So now,

|Z| = √(x² + y²)

= √((-1/2)² + (1/2)²)

= √(1/4 + 1/4)

= √(2/4)

= 1/√2

θ = tan^-1 (|y| / |x|)

= tan^-1 ((1/2) / (1/2))

= tan^-1 1

Since x < 0, y > 0 complex number lies in the 2^nd quadrant, and the value of θ is 90⁰≤θ≤180⁰.

θ = 3π/4

Z = 1/√2 (cos (3π/4) + i sin (3π/4))

∴ Polar form of (1 + 2i) / (1 – 3i) is 1/√2 (cos (3π/4) + i sin (3π/4))

(vii) sin 120^o – i cos 120^o

Given: Z = sin 120^o – i cos 120^o

= √3/2 – i (-1/2)

= √3/2 + i (1/2)

So now,

|Z| = √(x² + y²)

= √((√3/2)² + (1/2)²)

= √(3/4 + 1/4)

= √(4/4)

= √1

= 1

θ = tan^-1 (|y| / |x|)

= tan^-1 ((1/2) / (√3/2))

= tan^-1 (1/√3)

Since x > 0, y > 0 complex number lies in 1^st quadrant and the value of θ is 0⁰≤θ≤90⁰.

θ = π/6

Z = 1 (cos (π/6) + i sin (π/6))

∴ The polar form of √3/2 + i (1/2) is 1 (cos (π/6) + i sin (π/6))

(viii) -16 / (1 + i√3)

Given: Z = -16 / (1 + i√3)

Let us multiply and divide by (1 – i√3), and we get

So now,

|Z| = √(x² + y²)

= √((-4)² + (4√3)²)

= √(16 + 48)

= √(64)

= 8

θ = tan^-1 (|y| / |x|)

= tan^-1 ((4√3) / 4)

= tan^-1 (√3)

Since x < 0, y > 0 complex number lies in the 2^nd quadrant, and the value of θ is 90⁰≤θ≤180⁰.

θ = 2π/3

Z = 8 (cos (2π/3) + i sin (2π/3))

∴ The polar form of -16 / (1 + i√3) is 8 (cos (2π/3) + i sin (2π/3))

2. Write (i²⁵)³ in polar form.

Solution:

Given: Z = (i²⁵)³

= i⁷⁵

= i⁷⁴. i

= (i²)³⁷. i

= (-1)³⁷. i

= (-1). i

= – i

= 0 – i

So now,

|Z| = √(x² + y²)

= √(0² + (-1)²)

= √(0 + 1)

= √1

θ = tan^-1 (|y| / |x|)

= tan^-1 (1 / 0)

= tan^-1 ∞

Since x ≥ 0, y < 0 complex number lies in the 4^th quadrant, and the value of θ is -90⁰≤θ≤0⁰.

θ = -π/2

Z = 1 (cos (-π/2) + i sin (-π/2))

= 1 (cos (π/2) – i sin (π/2))

∴ The polar form of (i²⁵)³ is 1 (cos (π/2) – i sin (π/2))

3. Express the following complex numbers in the form r (cos θ + i sin θ):

(i) 1 + i tan α

(ii) tan α – i

(iii) 1 – sin α + i cos α

(iv) (1 – i) / (cos π/3 + i sin π/3)

Solution:

(i) 1 + i tan α

Given: Z = 1 + i tan α

We know that the polar form of a complex number Z = x + iy is given by Z = |Z| (cos θ + i sin θ)

Where,

|Z| = modulus of complex number = √(x² + y²)

θ = arg (z) = argument of complex number = tan^-1 (|y| / |x|)

We also know that tan α is a periodic function with period π.

So α is lying in the interval [0, π/2) ∪ (π/2, π].

Let us consider case 1:

α ∈ [0, π/2)

So now,

|Z| = r = √(x² + y²)

= √(1² + tan² α)

= √( sec² α)

= |sec α| since sec α is positive in the interval [0, π/2)

θ = tan^-1 (|y| / |x|)

= tan^-1 (tan α / 1)

= tan^-1 (tan α)

= α since tan α is positive in the interval [0, π/2)

∴ The polar form is Z = sec α (cos α + i sin α)

Let us consider case 2:

α ∈ (π/2, π]

So now,

|Z| = r = √(x² + y²)

= √(1² + tan² α)

= √( sec² α)

= |sec α|

= – sec α since sec α is negative in the interval (π/2, π]

θ = tan^-1 (|y| / |x|)

= tan^-1 (tan α / 1)

= tan^-1 (tan α)

= -π + α since tan α is negative in the interval (π/2, π]

θ = -π + α [since, θ lies in 4^th quadrant]

Z = -sec α (cos (α – π) + i sin (α – π))

∴ The polar form is Z = -sec α (cos (α – π) + i sin (α – π))

(ii) tan α – i

Given: Z = tan α – i

We know that the polar form of a complex number Z = x + iy is given by Z = |Z| (cos θ + i sin θ)

Where,

|Z| = modulus of complex number = √(x² + y²)

θ = arg (z) = argument of complex number = tan^-1 (|y| / |x|)

We also know that tan α is a periodic function with period π.

So α is lying in the interval [0, π/2) ∪ (π/2, π].

Let us consider case 1:

α ∈ [0, π/2)

So now,

|Z| = r = √(x² + y²)

= √(tan² α + 1²)

= √( sec² α)

= |sec α| since sec α is positive in the interval [0, π/2)

= sec α

θ = tan^-1 (|y| / |x|)

= tan^-1 (1/tan α)

= tan^-1 (cot α) since cot α is positive in the interval [0, π/2)

= α – π/2 [since, θ lies in 4^th quadrant]

Z = sec α (cos (α – π/2) + i sin (α – π/2))

∴ Polar form is Z = sec α (cos (α – π/2) + i sin (α – π/2))

Let us consider case 2:

α ∈ (π/2, π]

So now,

|Z| = r = √(x² + y²)

= √(tan² α + 1²)

= √( sec² α)

= |sec α|

= – sec α since sec α is negative in the interval (π/2, π]

θ = tan^-1 (|y| / |x|)

= tan^-1 (1/tan α)

= tan^-1 (cot α)

= π/2 + α since cot α is negative in the interval (π/2, π]

θ = π/2 + α [since, θ lies in 3^th quadrant]

Z = -sec α (cos (π/2 + α) + i sin (π/2 + α))

∴ The polar form is Z = -sec α (cos (π/2 + α) + i sin (π/2 + α))

(iii) 1 – sin α + i cos α

Given: Z = 1 – sin α + i cos α

By using the formulas,

Sin² θ + cos² θ = 1

Sin 2θ = 2 sin θ cos θ

Cos 2θ = cos² θ – sin² θ

So,

z= (sin²(α/2) + cos²(α/2) – 2 sin(α/2) cos(α/2)) + i (cos²(α/2) – sin²(α/2))

= (cos(α/2) – sin(α/2))² + i (cos²(α/2) – sin²(α/2))

We know that the polar form of a complex number Z = x + iy is given by Z = |Z| (cos θ + i sin θ)

Where,

|Z| = modulus of complex number = √(x² + y²)

θ = arg (z) = argument of complex number = tan^-1 (|y| / |x|)

Now,

We know that sine and cosine functions are periodic with period 2π.

Here, we have 3 intervals:

0 ≤ α ≤ π/2

π/2 ≤ α ≤ 3π/2

3π/2 ≤ α ≤ 2π

Let us consider case 1:

In the interval 0 ≤ α ≤ π/2

Cos (α/2) > sin (α/2) and also 0 < π/4 + α/2 < π/2

So,

∴ The polar form is Z = √2 (cos (α/2) – sin (α/2)) (cos (π/4 + α/2) + i sin (π/4 + α/2))

Let us consider case 2:

In the interval π/2 ≤ α ≤ 3π/2

Cos (α/2) < sin (α/2) and also π/2 < π/4 + α/2 < π

So,

Since (1 – sin α) > 0 and cos α < 0 [Z lies in the 4^th quadrant]

= α/2 – 3π/4

∴ Polar form is Z = –√2 (cos (α/2) – sin (α/2)) (cos (α/2 – 3π/4) + i sin (α/2 – 3π/4))

Let us consider case 3:

In the interval 3π/2 ≤ α ≤ 2π

Cos (α/2) < sin (α/2) and also π < π/4 + α/2 < 5π/4

So,

θ = tan^-1 (tan (π/4 + α/2))

= π – (π/4 + α/2) [since, θ lies in 1st quadrant and tan’s period is π]

= α/2 – 3π/4

∴ The polar form is Z = –√2 (cos (α/2) – sin (α/2)) (cos (α/2 – 3π/4) + i sin (α/2 – 3π/4))

(iv) (1 – i) / (cos π/3 + i sin π/3)

Given: Z = (1 – i) / (cos π/3 + i sin π/3)

Let us multiply and divide by (1 – i√3), and we get

We know that the polar form of a complex number Z = x + iy is given by Z = |Z| (cos θ + i sin θ)

Where,

|Z| = modulus of complex number = √(x² + y²)

θ = arg (z) = argument of complex number = tan^-1 (|y| / |x|)

Now,

Since x < 0, y < 0 complex number lies in the 3^rd quadrant, and the value of θ is 180⁰≤θ≤-90⁰.

= tan^-1 (2 + √3)

= -7π/12

Z = √2 (cos (-7π/12) + i sin (-7π/12))

= √2 (cos (7π/12) – i sin (7π/12))

∴ Polar form of (1 – i) / (cos π/3 + i sin π/3) is √2 (cos (7π/12) – i sin (7π/12))

4. If z₁ and z₂ are two complex number such that |z₁| = |z₂| and arg (z₁) + arg (z₂) = π, then show that

Solution:

Given:

|z₁| = |z₂| and arg (z₁) + arg (z₂) = π

Let us assume arg (z₁) = θ

arg (z₂) = π – θ

We know that in the polar form, z = |z| (cos θ + i sin θ)

z₁ = |z₁| (cos θ + i sin θ) …………. (i)

z₂ = |z₂| (cos (π – θ) + i sin (π – θ))

= |z₂| (-cos θ + i sin θ)

= – |z₂| (cos θ – i sin θ)

Now let us find the conjugate of

= – |z₂| (cos θ + i sin θ) …… (ii) (since,

Now,

z₁ /
= [|z₁| (cos θ + i sin θ)] / [-|z₂| (cos θ + i sin θ)]

= – |z₁| / |z₂| [since, |z₁| = |z₂|]

= -1

When we cross multiply, we get,

z₁ = –

Hence, proved.

5. If z₁, z₂ and z₃, z₄ are two pairs of conjugate complex numbers, prove that arg (z₁/z₄) + arg (z₂/z₃) = 0

Solution:

Given:

Hence, proved.

6. Express sin π/5 + i (1 – cos π/5) in polar form.

Solution:

Given:

Z = sin π/5 + i (1 – cos π/5)

By using the formula,

sin 2θ = 2 sin θ cos θ

1- cos 2θ = 2 sin² θ

So,

Z = 2 sin π/10 cos π/10 + i (2 sin² π/10)

= 2 sin π/10 (cos π/10 + i sin π/10)

∴ The polar form of sin π/5 + i (1 – cos π/5) is 2 sin π/10 (cos π/10 + i sin π/10)

Also, access exercises of RD Sharma Solutions for Class 11 Maths Chapter 13 – Complex Numbers

Exercise 13.1 Solutions

Exercise 13.2 Solutions

Exercise 13.3 Solutions

Exercise 13.4 Solutions