RD Sharma Solutions for Class 11 Maths Chapter 30 Derivatives

RD Sharma Solutions Class 11 Maths Chapter 30 – Download Free PDF Updated Session for 2023-24

RD Sharma Solutions for Class 11 Maths Chapter 30 – Derivatives are provided here for students to score good marks in the final exam. Chapter 30 of Class 11 RD Sharma Solutions has explanatory answers to exercise-wise problems in a comprehensive manner. This chapter provides students with the knowledge of derivatives at a point. The PDF of RD Sharma Solutions is available, both online and offline, to help students ace the examination.

Chapter 30 – Derivatives contains five exercises, and RD Sharma Solutions provide descriptive answers to the questions present in each exercise. Students who aim to perform well in the final exam are advised to solve problems using the solutions PDF. The main aim of creating solutions is to help students clear their doubts instantly and improve conceptual knowledge. RD Sharma Class 11 Maths Solutions free PDF links are available below. Now, let us have a look at the concepts discussed in this chapter.

• Derivative at a point
  • Physical interpretation of derivative at a point
  • Geometrical interpretation of derivative at a point
• Derivative of a function
  • Derivative as a rate measurer
• Differentiation from first principles
• Fundamental rules for differentiation
  • Product rule for differentiation
  • Quotient rule for differentiation

RD Sharma Solutions for Class 11 Maths Chapter 30 – Derivatives

Download PDF

carouselExampleControls111

Previous



Next

Access answers to RD Sharma Solutions for Class 11 Maths Chapter 30 – Derivatives

EXERCISE 30.1 PAGE NO: 30.3

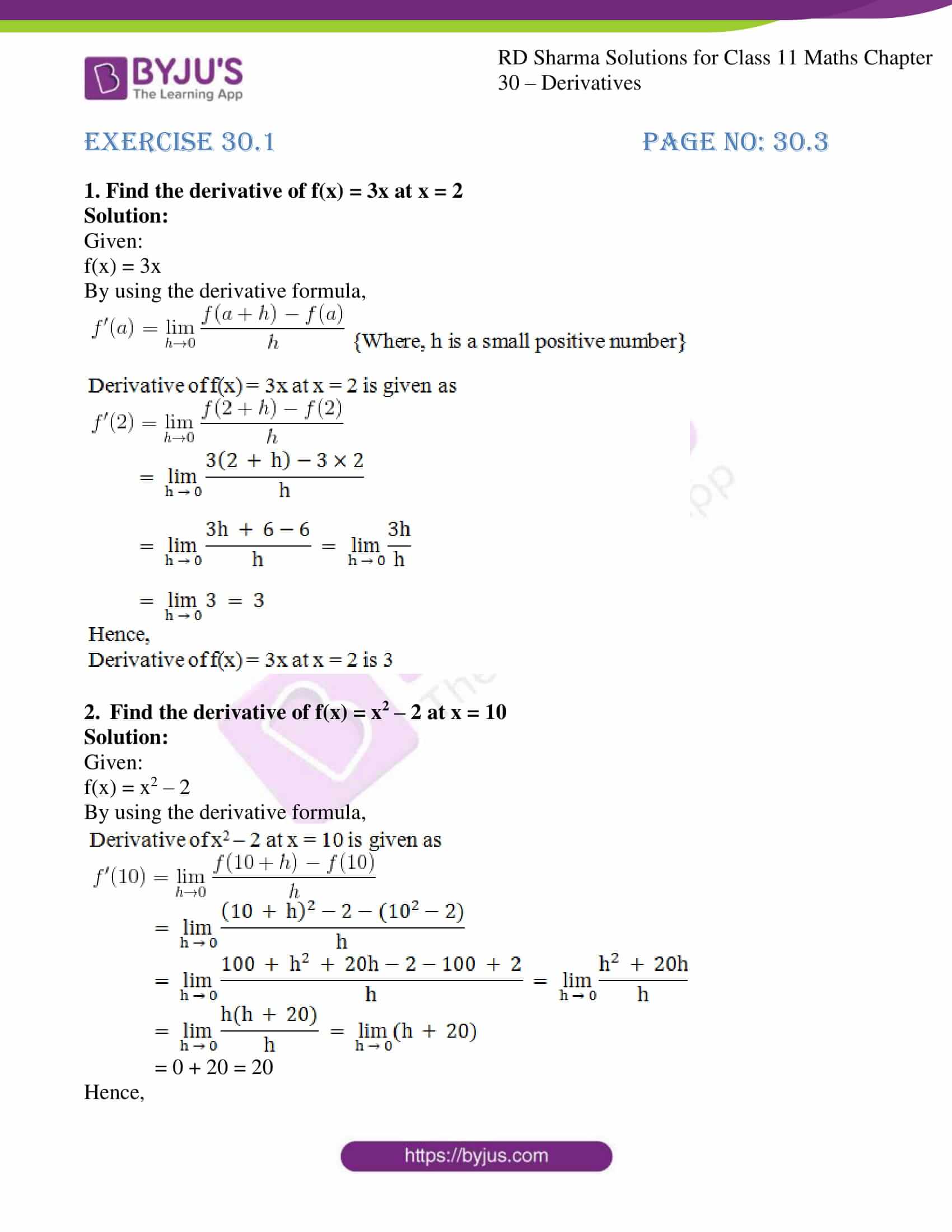
1. Find the derivative of f(x) = 3x at x = 2

Solution:

Given:

f(x) = 3x

By using the derivative formula,

2. Find the derivative of f(x) = x² – 2 at x = 10

Solution:

Given:

f(x) = x² – 2

By using the derivative formula,

= 0 + 20 = 20

Hence,

Derivative of f(x) = x² – 2 at x = 10 is 20

3. Find the derivative of f(x) = 99x at x = 100.

Solution:

Given:

f(x) = 99x

By using the derivative formula,

4. Find the derivative of f(x) = x at x = 1

Solution:

Given:

f(x) = x

By using the derivative formula,

5. Find the derivative of f(x) = cos x at x = 0

Solution:

Given:

f(x) = cos x

By using the derivative formula,

6. Find the derivative of f(x) = tan x at x = 0

Solution:

Given:

f(x) = tan x

By using the derivative formula,

7. Find the derivatives of the following functions at the indicated points:
(i) sin x at x = π/2
(ii) x at x = 1

(iii) 2 cos x at x = π/2

(iv) sin 2xat x = π/2

Solution:

(i) sin x at x = π/2

Given:

f (x) = sin x

By using the derivative formula,

We know that 1 – cos x = 2 sin²(x/2)

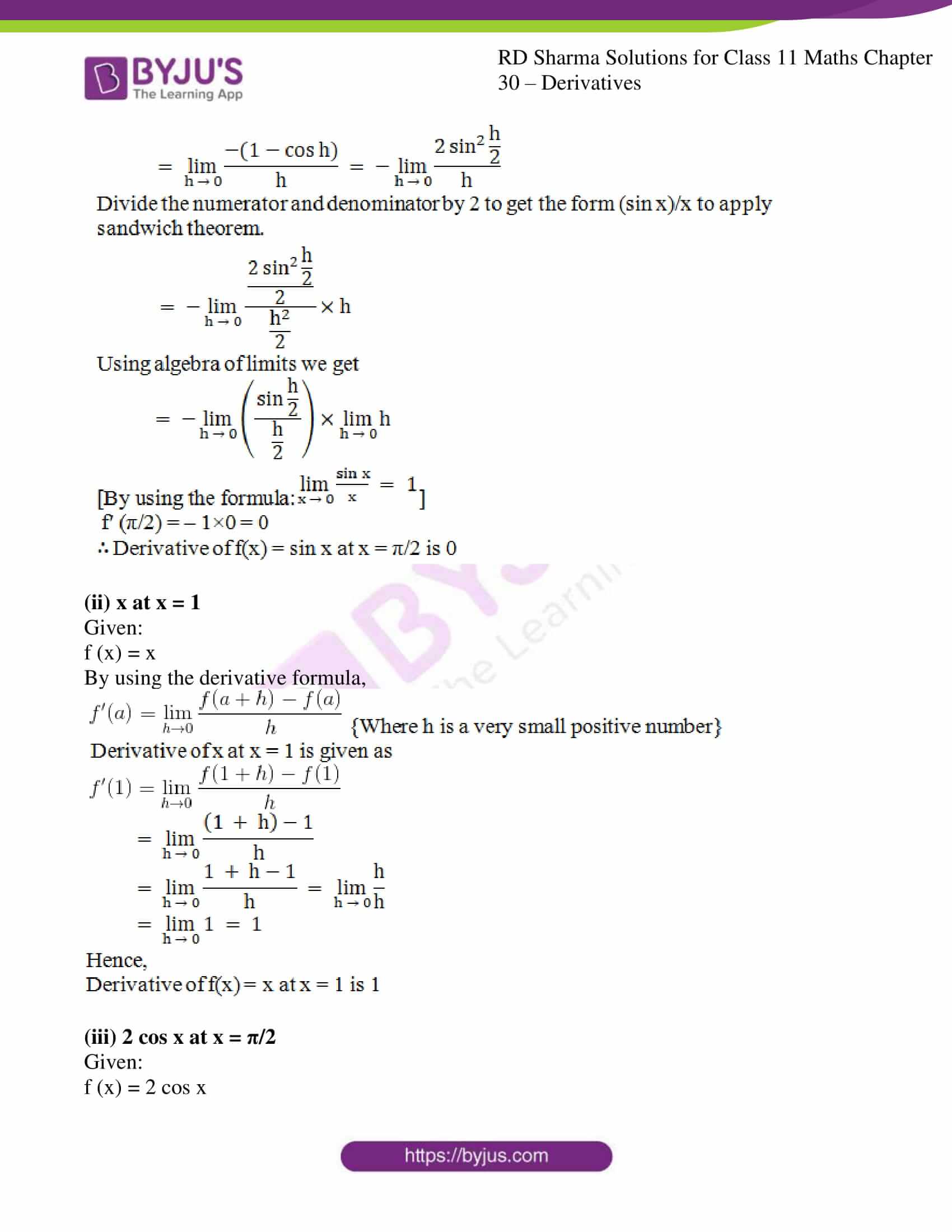
(ii) x at x = 1

Given:

f (x) = x

By using the derivative formula,

(iii) 2 cos x at x = π/2

Given:

f (x) = 2 cos x

By using the derivative formula,

(iv) sin 2xat x = π/2

Solution:

Given:

f (x) = sin 2x

By using the derivative formula,

Now, multiply the numerator and denominator by 2, and we get

EXERCISE 30.2 PAGE NO: 30.25

1. Differentiate each of the following from the first principles:
(i) 2/x
(ii) 1/√x

(iii) 1/x³

(iv) [x² + 1]/ x

(v) [x² – 1] / x

Solution:

(i) 2/x

Given:

f (x) = 2/x

By using the formula,

∴ Derivative of f(x) = 2/x is -2x^-2

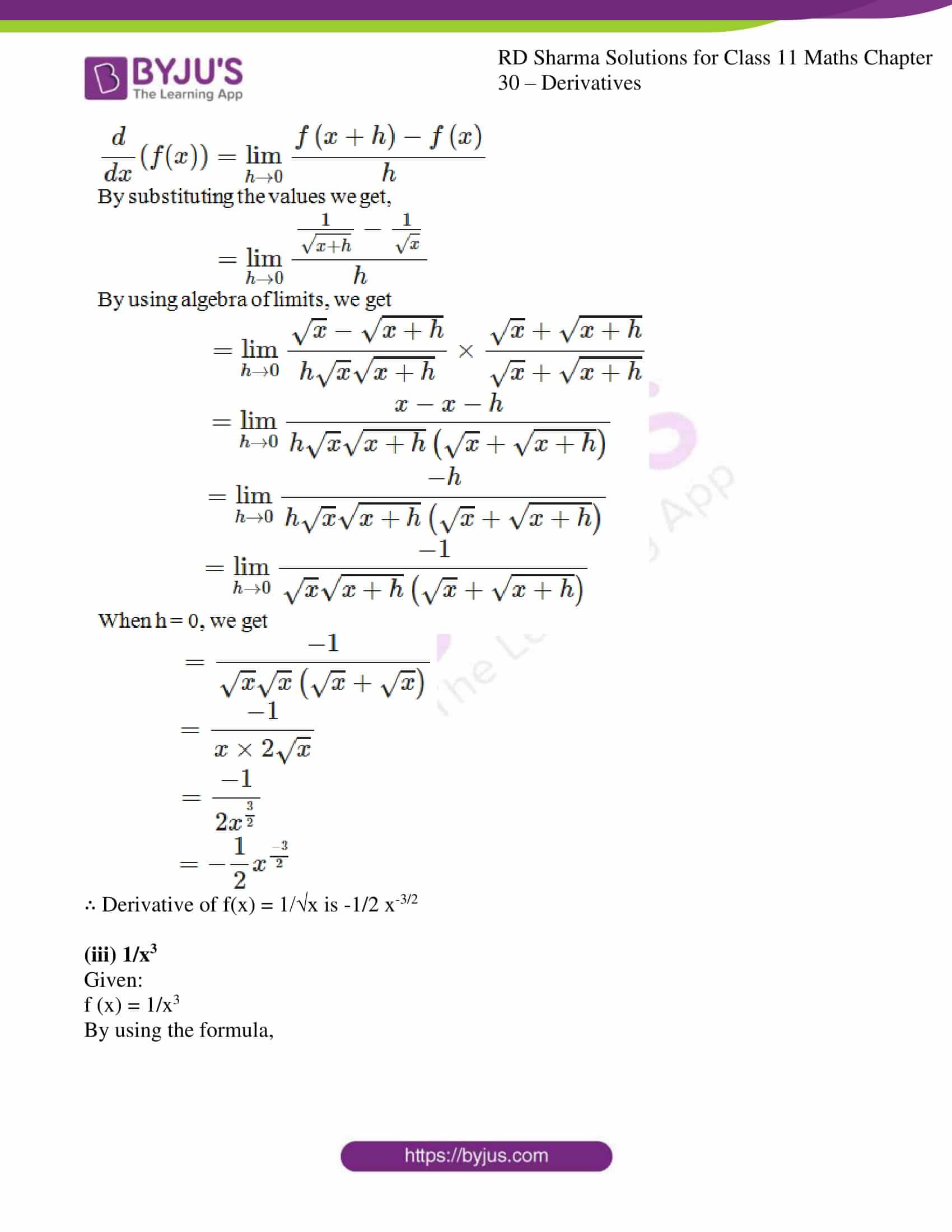
(ii) 1/√x

Given:

f (x) = 1/√x

By using the formula,

∴ Derivative of f(x) = 1/√x is -1/2 x^-3/2

(iii) 1/x³

Given:

f (x) = 1/x³

By using the formula,

∴ Derivative of f(x) = 1/x³ is -3x^-4

(iv) [x² + 1]/ x

Given:

f (x) = [x² + 1]/ x

By using the formula,

= 1 – 1/x²

∴ Derivative of f(x) = 1 – 1/x²

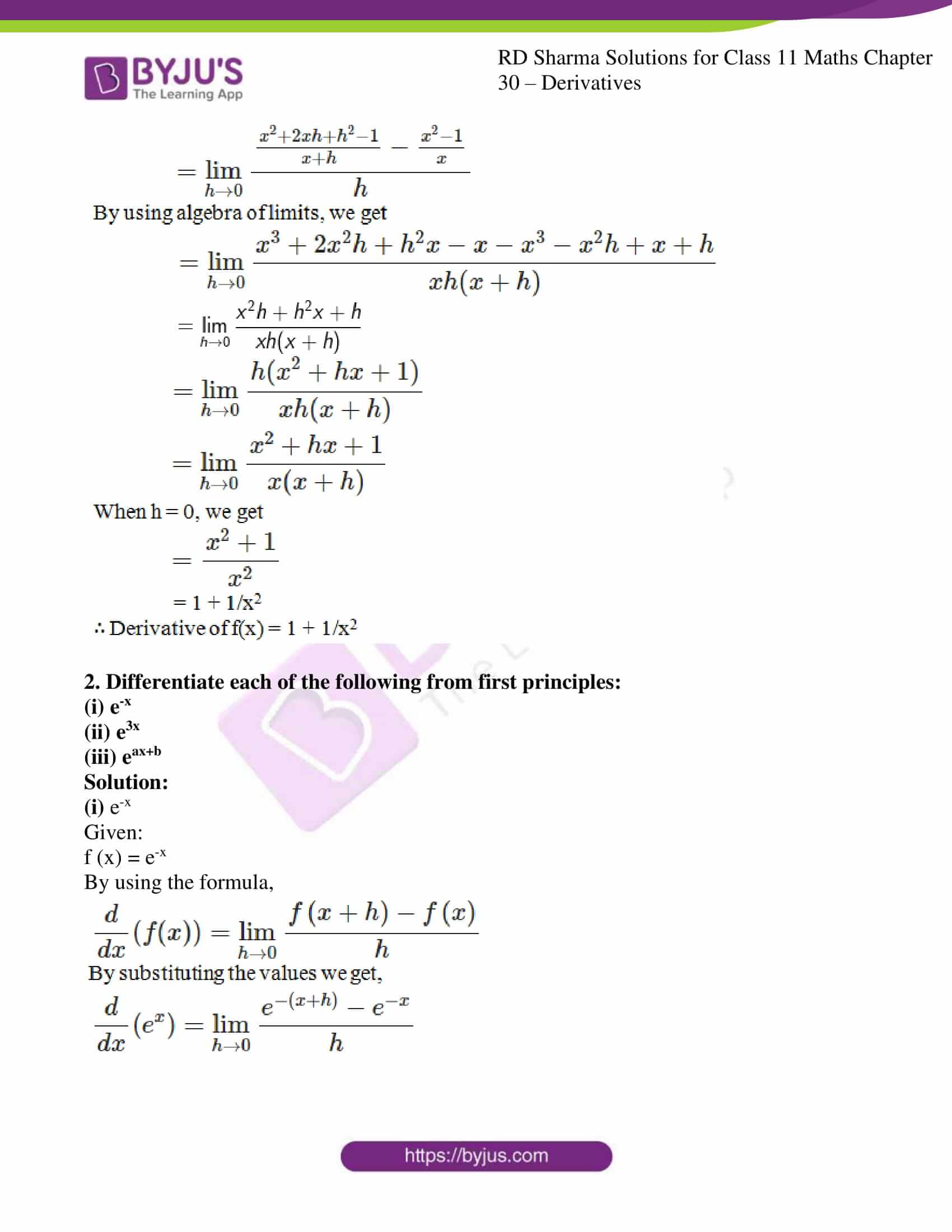
(v) [x² – 1] / x

Given:

f (x) = [x² – 1]/ x

By using the formula,

2. Differentiate each of the following from the first principles:

(i) e^-x

(ii) e^3x

(iii) e^ax+b

Solution:

(i) e^-x

Given:

f (x) = e^-x

By using the formula,

(ii) e^3x

Given:

f (x) = e^3x

By using the formula,

(iii) e^ax+b

Given:

f (x) = e^ax+b

By using the formula,

3. Differentiate each of the following from the first principles:

(i) √(sin 2x)

(ii) sin x/x

Solution:

(i) √(sin 2x)

Given:

f (x) = √(sin 2x)

By using the formula,

(ii) sin x/x

Given:

f (x) = sin x/x

By using the formula,

4. Differentiate the following from the first principles:

(i) tan² x

(ii) tan (2x + 1)

Solution:

(i) tan² x

Given:

f (x) = tan² x

By using the formula,

(ii) tan (2x + 1)

Given:

f (x) = tan (2x + 1)

By using the formula,

5. Differentiate the following from the first principles:

(i) sin √2x

(ii) cos √x

Solution:

(i) sin √2x

Given:

f (x) = sin √2x

f (x + h) = sin √2(x+h)

By using the formula,

(ii) cos √x

Given:

f (x) = cos √x

f (x + h) = cos √(x+h)

By using the formula,

EXERCISE 30.3 PAGE NO: 30.33

Differentiate the following with respect to x:

1. x⁴ – 2sin x + 3 cos x

Solution:

Given:

f (x) = x⁴ – 2sin x + 3 cos x

Differentiate on both sides with respect to x, and we get

2. 3^x + x³ + 3³

Solution:

Given:

f (x) = 3^x + x³ + 3³

Differentiate on both sides with respect to x, and we get

Solution:

Given:

Differentiate on both sides with respect to x, and we get

4. e^{x log a} + e^{a log x} + e^{a log a}

Solution:

Given:

f (x) = e^{x log a} + e^{a log x} + e^{a log a}

We know that,

e^{log f(x)} = f(x)

So,

f(x) = a^x + x^a + a^a

Differentiate on both sides with respect to x, and we get

5. (2x² + 1) (3x + 2)

Solution:

Given:

f (x) = (2x² + 1) (3x + 2)

= 6x³ + 4x² + 3x + 2

Differentiate on both sides with respect to x, and we get

EXERCISE 30.4 PAGE NO: 30.39

Differentiate the following functions with respect to x:

1. x³ sin x

Solution:

Let us consider y = x³ sin x

We need to find dy/dx

We know that y is a product of two functions, say u and v, where,

u = x³ and v = sin x

∴ y = uv

Now let us apply the product rule of differentiation.

By using the product rule, we get

2. x³ e^x

Solution:

Let us consider y = x³ e^x

We need to find dy/dx

We know that y is a product of two functions, say u and v, where,

u = x³ and v = e^x

∴ y = uv

Now let us apply the product rule of differentiation.

By using the product rule, we get

3. x² e^x log x

Solution:

Let us consider y = x² e^x log x

We need to find dy/dx

We know that y is a product of two functions, say u and v, where,

u = x² and v = e^x

∴ y = uv

Now let us apply the product rule of differentiation.

By using the product rule, we get

4. xⁿ tan x

Solution:

Let us consider y = xⁿ tan x

We need to find dy/dx

We know that y is a product of two functions, say u and v, where,

u = xⁿ and v = tan x

∴ y = uv

Now let us apply the product rule of differentiation.

By using the product rule, we get

5. xⁿ log_a x

Solution:

Let us consider y = xⁿ log_a x

We need to find dy/dx

We know that y is a product of two functions, say u and v, where,

u = xⁿ and v = log_a x

∴ y = uv

Now let us apply the product rule of differentiation.

By using the product rule, we get

EXERCISE 30.5 PAGE NO: 30.44

Differentiate the following functions with respect to x:

Solution:

Let us consider

y =

We need to find dy/dx

We know that y is a fraction of two functions, say u and v, where,

u = x² + 1 and v = x + 1

∴ y = u/v

Now let us apply the quotient rule of differentiation.

By using the quotient rule, we get

Solution:

Let us consider

y =

We need to find dy/dx

We know that y is a fraction of two functions, say u and v, where,

u = 2x – 1 and v = x² + 1

∴ y = u/v

Now let us apply the quotient rule of differentiation.

By using the quotient rule, we get

Solution:

Let us consider

y =

We need to find dy/dx

We know that y is a fraction of two functions, say u and v, where,

u = x + e^x and v = 1 + log x

∴ y = u/v

Now let us apply the quotient rule of differentiation.

By using the quotient rule, we get

Solution:

Let us consider

y =

We need to find dy/dx

We know that y is a fraction of two functions, say u and v, where,

u = e^x – tan x and v = cot x – xⁿ

∴ y = u/v

Now let us apply the quotient rule of differentiation.

By using the quotient rule, we get

Solution:

Let us consider

y =

We need to find dy/dx

We know that y is a fraction of two functions, say u and v, where,

u = ax² + bx + c and v = px² + qx + r

∴ y = u/v

Now let us apply the quotient rule of differentiation.

By using the quotient rule, we get

Also, access exercises of RD Sharma Solutions for Class 11 Maths Chapter 30 – Derivatives

Exercise 30.1 Solutions

Exercise 30.2 Solutions

Exercise 30.3 Solutions

Exercise 30.4 Solutions

Exercise 30.5 Solutions