Here, in this exercise, we shall discuss concepts related to the derivative at a point. The answers are explained with suitable examples for students to understand clearly. Students can make use of RD Sharma Class 11 Maths Solutions to obtain good marks in the board examination. Subject experts have designed the solutions in a step-by-step manner to help students understand the concepts and score well in the exams. The PDF of RD Sharma Solutions is provided in the links given below. It can be downloaded easily and can be used for future reference as well.
RD Sharma Solutions for Class 11 Maths Exercise 30.1 Chapter 30 – Derivatives
Also, access other exercises of RD Sharma Solutions for Class 11 Maths Chapter 30 – Derivatives
Access answers to RD Sharma Solutions for Class 11 Maths Exercise 30.1 Chapter 30 – Derivatives
EXERCISE 30.1 PAGE NO: 30.3
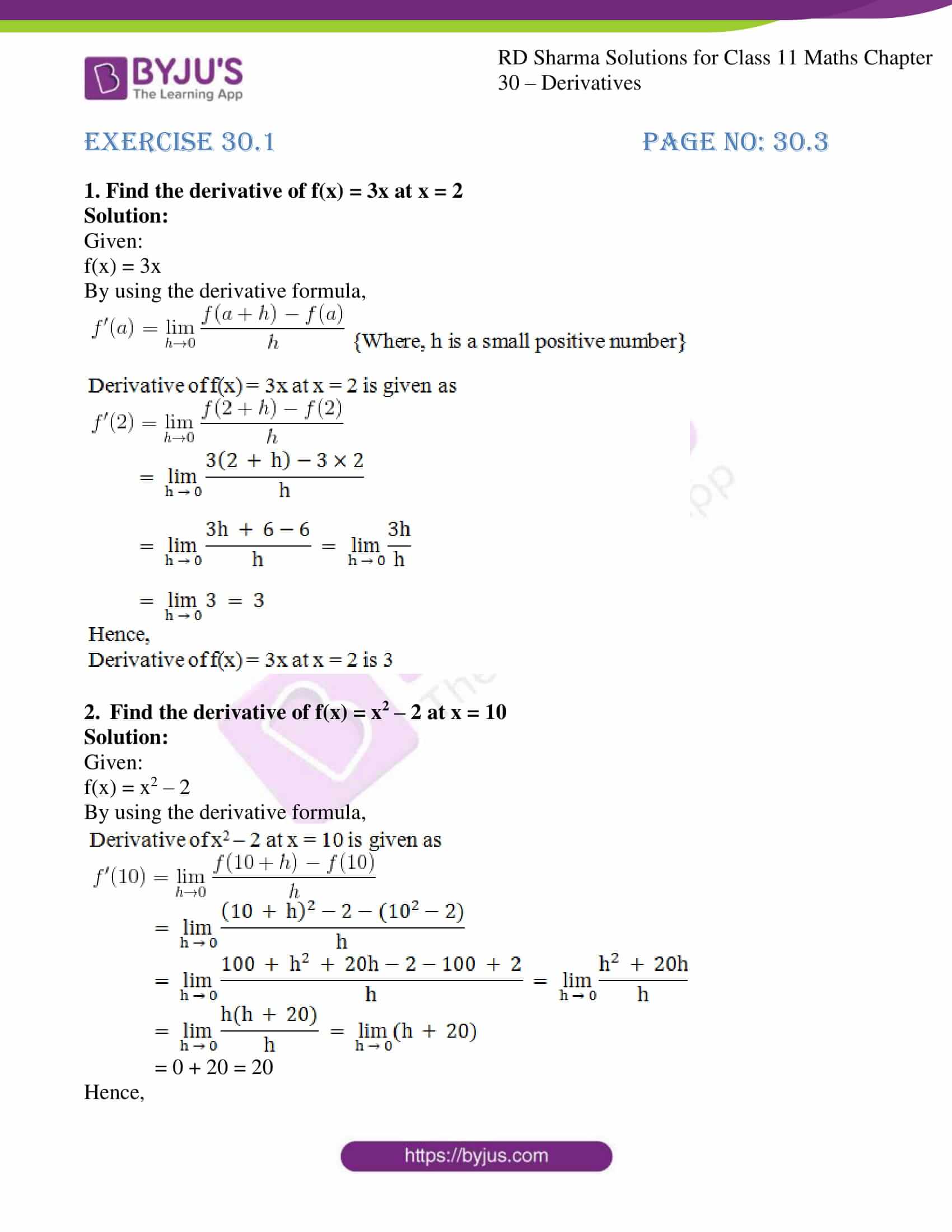
1. Find the derivative of f(x) = 3x at x = 2
Solution:
Given:
f(x) = 3x
By using the derivative formula,

2. Find the derivative of f(x) = x2 – 2 at x = 10
Solution:
Given:
f(x) = x2 – 2
By using the derivative formula,

= 0 + 20 = 20
Hence,
Derivative of f(x) = x2 – 2 at x = 10 is 20
3. Find the derivative of f(x) = 99x at x = 100.
Solution:
Given:
f(x) = 99x
By using the derivative formula,

4. Find the derivative of f(x) = x at x = 1
Solution:
Given:
f(x) = x
By using the derivative formula,

5. Find the derivative of f(x) = cos x at x = 0
Solution:
Given:
f(x) = cos x
By using the derivative formula,


6. Find the derivative of f(x) = tan x at x = 0
Solution:
Given:
f(x) = tan x
By using the derivative formula,

7. Find the derivatives of the following functions at the indicated points:
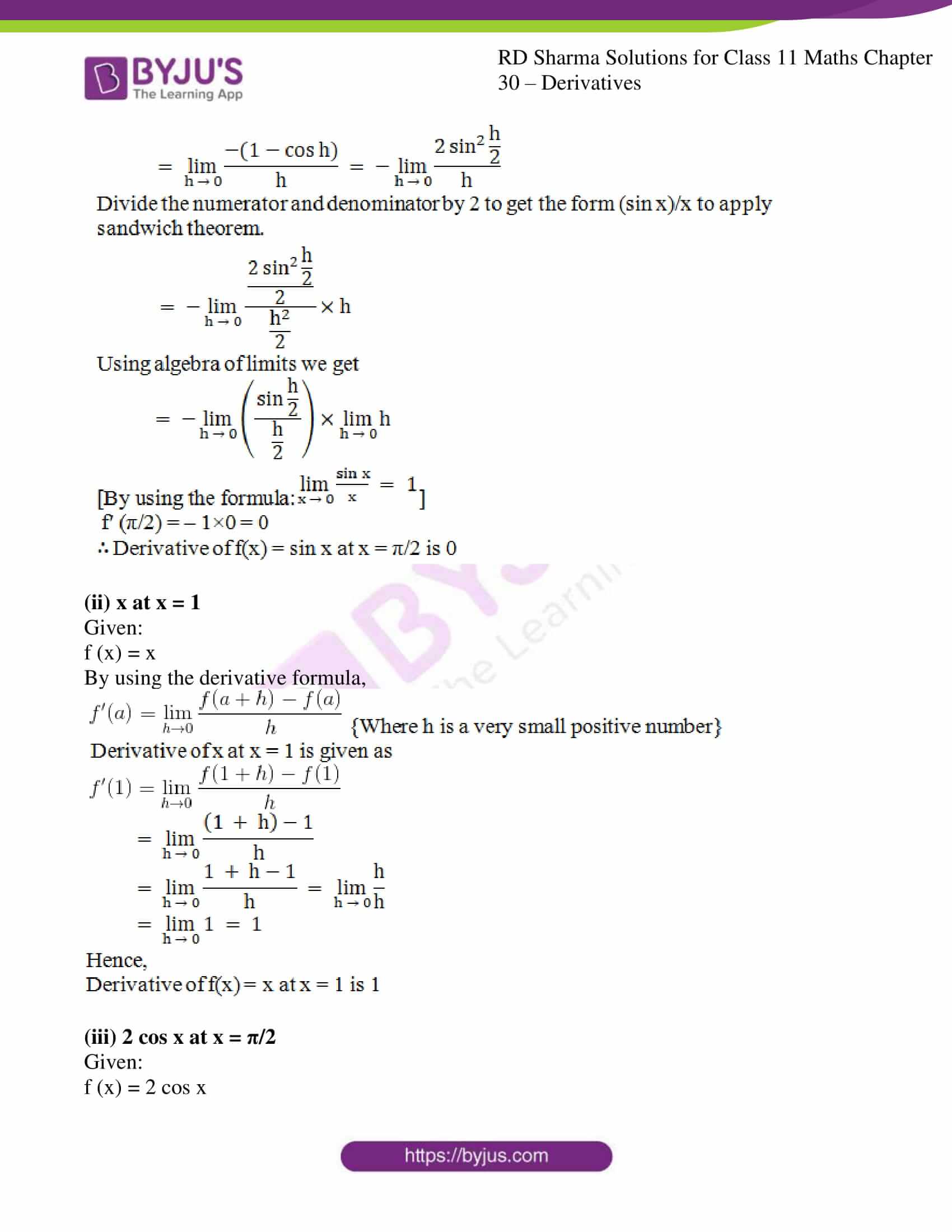
(i) sin x at x = π/2
(ii) x at x = 1
(iii) 2 cos x at x = π/2
(iv) sin 2xat x = π/2
Solution:
(i) sin x at x = π/2
Given:
f (x) = sin x
By using the derivative formula,

We know that 1 – cos x = 2 sin2(x/2)

(ii) x at x = 1
Given:
f (x) = x
By using the derivative formula,

(iii) 2 cos x at x = π/2
Given:
f (x) = 2 cos x
By using the derivative formula,

(iv) sin 2xat x = π/2
Solution:
Given:
f (x) = sin 2x
By using the derivative formula,

Now, multiply the numerator and denominator by 2, we get






Comments