In Exercise 5.2 of Chapter 5, we shall discuss problems based on signs of trigonometric functions and variations in values of trigonometric functions in different quadrants. Students can refer to RD Sharma Class 11 Solutions which is prepared by our expert tutors to help students clarify their doubts pertaining to this chapter. With regular practice, students can speed up solving problems by using short-cut tips to secure high marks in their examinations. Students can download the PDF easily from the links given below.
RD Sharma Solutions for Class 11 Maths Exercise 5.2 Chapter 5 – Trigonometric Functions
Also, access other exercises of RD Sharma Solutions for Class 11 Maths Chapter 5 – Trigonometric Functions
Access answers to RD Sharma Solutions for Class 11 Maths Exercise 5.2 Chapter 5 – Trigonometric Functions
1. Find the values of the other five trigonometric functions in each of the following:
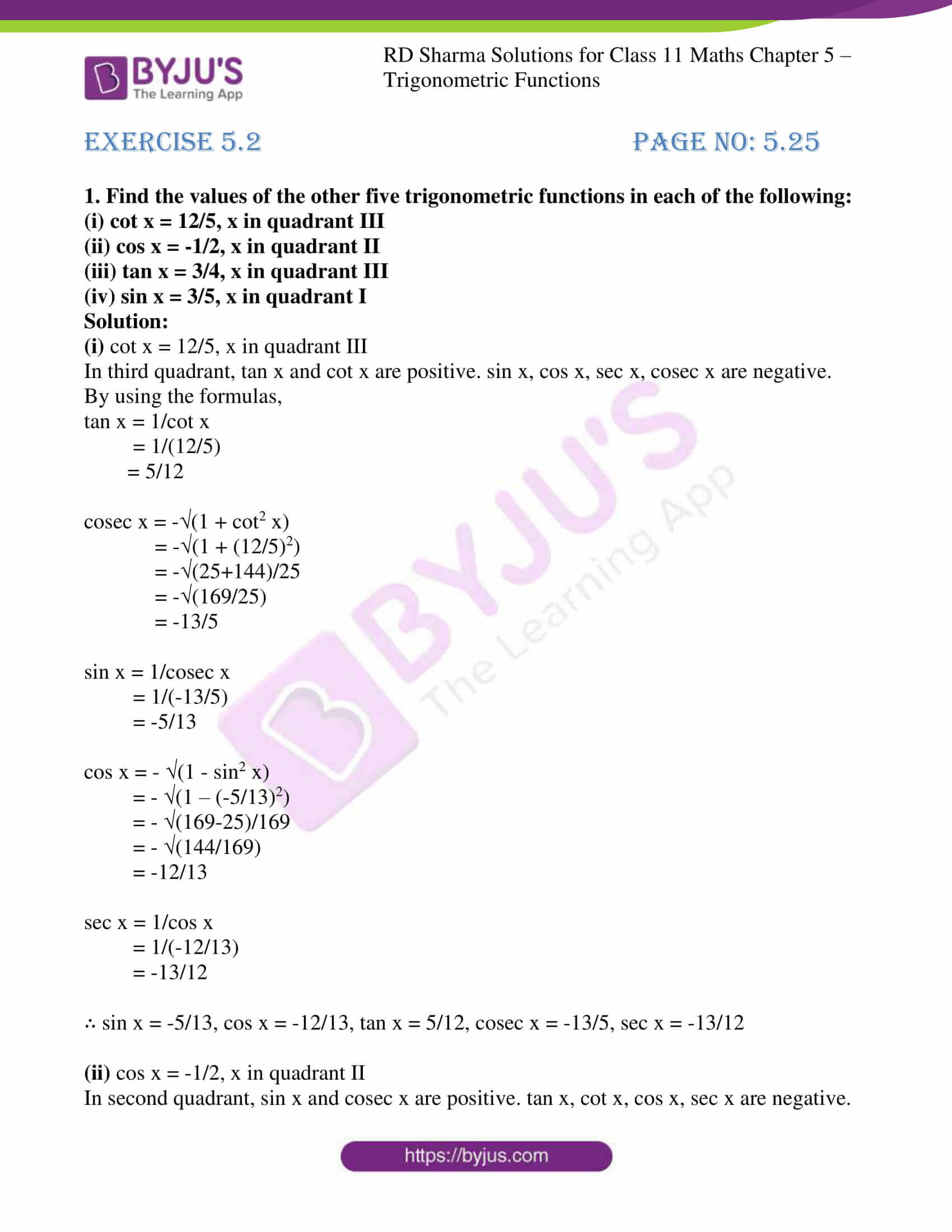
(i) cot x = 12/5, x in quadrant III
(ii) cos x = -1/2, x in quadrant II
(iii) tan x = 3/4, x in quadrant III
(iv) sin x = 3/5, x in quadrant I
Solution:
(i) cot x = 12/5, x in quadrant III
In the third quadrant, tan x and cot x is positive. sin x, cos x, sec x, cosec x are negative.
By using the formulas,
tan x = 1/cot x
= 1/(12/5)
= 5/12
cosec x = –√(1 + cot2 x)
= –√(1 + (12/5)2)
= –√(25+144)/25
= –√(169/25)
= -13/5
sin x = 1/cosec x
= 1/(-13/5)
= -5/13
cos x = – √(1 – sin2 x)
= – √(1 – (-5/13)2)
= – √(169-25)/169
= – √(144/169)
= -12/13
sec x = 1/cos x
= 1/(-12/13)
= -13/12
∴ sin x = -5/13, cos x = -12/13, tan x = 5/12, cosec x = -13/5, sec x = -13/12
(ii) cos x = -1/2, x in quadrant II
In second quadrant, sin x and cosec x are positive. tan x, cot x, cos x, sec x are negative.
By using the formulas,
sin x = √(1 – cos2 x)
= √(1 – (-1/2)2)
= √(4-1)/4
= √(3/4)
= √3/2
tan x = sin x/cos x
= (√3/2)/(-1/2)
= -√3
cot x = 1/tan x
= 1/-√3
= -1/√3
cosec x = 1/sin x
= 1/(√3/2)
= 2/√3
sec x = 1/cos x
= 1/(-1/2)
= -2
∴ sin x = √3/2, tan x = -√3, cosec x = 2/√3, cot x = -1/√3 sec x = -2
(iii) tan x = 3/4, x in quadrant III
In the third quadrant, tan x and cot x is positive. sin x, cos x, sec x, cosec x are negative.
By using the formulas,
sin x = √(1 – cos2 x)
= – √(1-(-4/5)2)
= – √(25-16)/25
= – √(9/25)
= – 3/5
cos x = 1/sec x
= 1/(-5/4)
= -4/5
cot x = 1/tan x
= 1/(3/4)
= 4/3
cosec x = 1/sin x
= 1/(-3/5)
= -5/3
sec x = -√(1 + tan2 x)
= – √(1+(3/4)2)
= – √(16+9)/16
= – √ (25/16)
= -5/4
∴ sin x = -3/5, cos x = -4/5, cosec x = -5/3, sec x = -5/4, cot x = 4/3
(iv) sin x = 3/5, x in quadrant I
In the first quadrant, all trigonometric ratios are positive.
So, by using the formulas,
tan x = sin x/cos x
= (3/5)/(4/5)
= 3/4
cosec x = 1/sin x
= 1/(3/5)
= 5/3
cos x = √(1-sin2 x)
= √(1 – (-3/5)2)
= √(25-9)/25
= √(16/25)
= 4/5
sec x = 1/cos x
= 1/(4/5)
= 5/4
cot x = 1/tan x
= 1/(3/4)
= 4/3
∴ cos x = 4/5, tan x = 3/4, cosec x = 5/3, sec x = 5/4, cot x = 4/3
2. If sin x = 12/13 and lies in the second quadrant, find the value of sec x + tan x.
Solution:
Given:
Sin x = 12/13, and x lie in the second quadrant.
We know, in the second quadrant, sin x and cosec x are positive, and all other ratios are negative.
By using the formulas,
Cos x = √(1-sin2 x)
= – √(1-(12/13)2)
= – √(1- (144/169))
= – √(169-144)/169
= -√(25/169)
= – 5/13
We know,
tan x = sin x/cos x
sec x = 1/cos x
Now,
tan x = (12/13)/(-5/13)
= -12/5
sec x = 1/(-5/13)
= -13/5
Sec x + tan x = -13/5 + (-12/5)
= (-13-12)/5
= -25/5
= -5
∴ Sec x + tan x = -5
3. If sin x = 3/5, tan y = 1/2 and π/2 < x< π< y< 3π/2 find the value of 8 tan x -√5 sec y.
Solution:
Given:
sin x = 3/5, tan y = 1/2 and π/2 < x< π< y< 3π/2
We know that x is in the second quadrant and y is in the third quadrant.
In the second quadrant, cos x and tan x are negative.
In the third quadrant, sec y is negative.
By using the formula,
cos x = – √(1-sin2 x)
tan x = sin x/cos x
Now,
cos x = – √(1-sin2 x)
= – √(1 – (3/5)2)
= – √(1 – 9/25)
= – √((25-9)/25)
= – √(16/25)
= – 4/5
tan x = sin x/cos x
= (3/5)/(-4/5)
= 3/5 × -5/4
= -3/4
We know that sec y = – √(1+tan2 y)
= – √(1 + (1/2)2)
= – √(1 + 1/4)
= – √((4+1)/4)
= – √(5/4)
= – √5/2
Now, 8 tan x – √5 sec y = 8(-3/4) – √5(-√5/2)
= -6 + 5/2
= (-12+5)/2
= -7/2
∴ 8 tan x – √5 sec y = -7/2
4. If sin x + cos x = 0 and x lies in the fourth quadrant, find sin x and cos x.
Solution:
Given:
Sin x + cos x = 0 and x lies in the fourth quadrant.
Sin x = -cos x
Sin x/cos x = -1
So, tan x = -1 (since, tan x = sin x/cos x)
We know that, in the fourth quadrant, cos x and sec x are positive, and all other ratios are negative.
By using the formulas,
Sec x = √(1 + tan2 x)
Cos x = 1/sec x
Sin x = – √(1- cos2 x)
Now,
Sec x = √(1 + tan2 x)
= √(1 + (-1)2)
= √2
Cos x = 1/sec x
= 1/√2
Sin x = – √(1 – cos2 x)
= – √(1 – (1/√2)2)
= – √(1 – (1/2))
= – √((2-1)/2)
= – √(1/2)
= -1/√2
∴ sin x = -1/√2 and cos x = 1/√2
5. If cos x = -3/5 and π<x<3π/2 find the values of other five trigonometric functions and hence evaluate 
Solution:
Given:
cos x= -3/5 and π <x < 3π/2
We know that in the third quadrant, tan x and cot x are positive, and all other rations are negative.
By using the formulas,
Sin x = – √(1-cos2 x)
Tan x = sin x/cos x
Cot x = 1/tan x
Sec x = 1/cos x
Cosec x = 1/sin x
Now,
Sin x = – √(1-cos2 x)
= – √(1-(-3/5)2)
= – √(1-9/25)
= – √((25-9)/25)
= – √(16/25)
= – 4/5
Tan x = sin x/cos x
= (-4/5)/(-3/5)
= -4/5 × -5/3
= 4/3
Cot x = 1/tan x
= 1/(4/3)
= 3/4
Sec x = 1/cos x
= 1/(-3/5)
= -5/3
Cosec x = 1/sin x
= 1/(-4/5)
= -5/4
∴ 
= [(-5/4) + (3/4)] / [(-5/3) – (4/3)]
= [(-5+3)/4] / [(-5-4)/3]
= [-2/4] / [-9/3]
= [-1/2] / [-3]
= 1/6






Comments