RD Sharma Solutions for Class 11 Chapter 9 - Values of Trigonometric Functions at Multiples and Submultiples of an Angle Exercise 9.1

RD Sharma Solutions for Chapter 9 Exercise 9.1 are given here. In this exercise, we shall discuss problems based on the values of trigonometric functions at ‘2x’, ‘x’ and ‘x/2’ in terms of values at ‘x’, ‘x/2’ and ‘cos x’. The solutions for this exercise are prepared by experts at BYJU’S in the best possible ways to make them easily understandable for the students. RD Sharma Class 11 Solutions can be used as the best reference material for students to score high marks in their exams. Solutions to this exercise are readily accessible and are available in PDF format. Students can easily download the PDF from the links provided below.

RD Sharma Solutions for Class 11 Maths Exercise 9.1 Chapter 9 – Values of Trigonometric Functions at Multiples and Submultiples of an Angle

Download PDF

carouselExampleControls112

Previous Next

Also, access other exercises of RD Sharma Solutions for Class 11 Maths Chapter 9 – Values of Trigonometric Functions at Multiples and Submultiples of an Angle

Exercise 9.2 Solutions

Exercise 9.3 solutions

Access answers to RD Sharma Solutions for Class 11 Maths Exercise 9.1 Chapter 9 – Values of Trigonometric Functions at Multiples and Submultiples of an Angle

Prove the following identities:

1. √[(1 – cos 2x) / (1 + cos 2x)] = tan x

Solution:

Let us consider LHS:

√[(1 – cos 2x) / (1 + cos 2x)]

We know that cos 2x = 1 – 2 sin² x

= 2 cos² x – 1

So,

√[(1 – cos 2x) / (1 + cos 2x)] = √[(1 – (1 – 2sin² x)) / (1 + (2cos²x – 1))]

= √[(1 – 1 + 2sin² x) / (1 + 2cos² x – 1)]

= √[2 sin² x / 2 cos² x]

= sin x/cos x

= tan x

= RHS

Hence proved.

2. sin 2x / (1 – cos 2x) = cot x

Solution:

Let us consider LHS:

sin 2x / (1 – cos 2x)

We know that cos 2x = 1 – 2 sin² x

Sin 2x = 2 sin x cos x

So,

sin 2x / (1 – cos 2x) = (2 sin x cos x) / (1 – (1 – 2sin² x))

= (2 sin x cos x) / (1 – 1 + 2sin² x)]

= [2 sin x cos x / 2 sin² x]

= cos x/sin x

= cot x

= RHS

Hence proved.

3. sin 2x / (1 + cos 2x) = tan x

Solution:

Let us consider LHS:

sin 2x / (1 + cos 2x)

We know that cos 2x = 1 – 2 sin² x

= 2 cos² x – 1

Sin 2x = 2 sin x cos x

So,

sin 2x / (1 + cos 2x) = [2 sin x cos x / (1 + (2cos²x – 1))]

= [2 sin x cos x / (1 + 2cos² x – 1)]

= [2 sin x cos x / 2 cos² x]

= sin x/cos x

= tan x

= RHS

Hence proved.

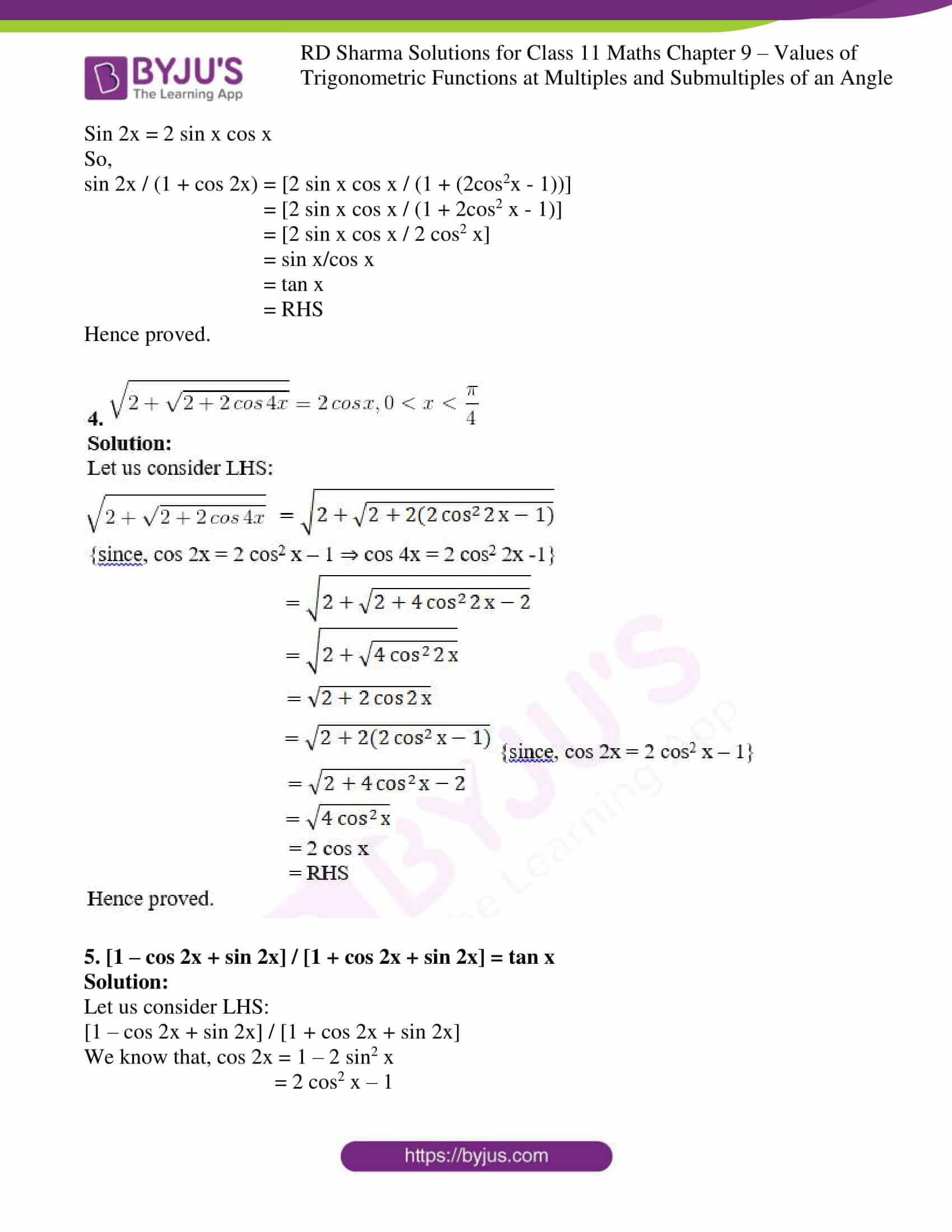
5. [1 – cos 2x + sin 2x] / [1 + cos 2x + sin 2x] = tan x

Solution:

Let us consider LHS:

We know that, cos 2x = 1 – 2 sin² x

= 2 cos² x – 1

Sin 2x = 2 sin x cos x

So,

6. [sin x + sin 2x] / [1 + cos x + cos 2x] = tan x

Solution:

Let us consider LHS:

We know that, cos 2x = cos² x – sin² x

Sin 2x = 2 sin x cos x

So,

= RHS

Hence proved.

7. cos 2x / (1 + sin 2x) = tan (π/4 – x)

Solution:

Let us consider LHS:

cos 2x / (1 + sin 2x)

We know that, cos 2x = cos² x – sin² x

Sin 2x = 2 sin x cos x

So,

8. cos x / (1 – sin x) = tan (π/4 + x/2)

Solution:

Let us consider LHS:

cos x / (1 – sin x)

We know that, cos 2x = cos² x – sin² x

Cos x = cos² x/2 – sin² x/2

Sin 2x = 2 sin x cos x

Sin x = 2 sin x/2 cos x/2

So,

11. (cos α + cos β) ² + (sin α + sin β) ² = 4 cos² (α – β)/2

Solution:

Let us consider LHS:

(cos α + cos β)² + (sin α + sin β)²

Upon expansion, we get,

(cos α + cos β)² + (sin α + sin β)² =

= cos² α + cos² β + 2 cos α cos β + sin² α + sin² β + 2 sin α sin β

= 2 + 2 cos α cos β + 2 sin α sin β

= 2 (1 + cos α cos β + sin α sin β)

= 2 (1 + cos (α – β)) [since, cos (A – B) = cos A cos B + sin A sin B]

= 2 (1 + 2 cos² (α – β)/2 – 1) [since, cos2x = 2cos² x – 1]

= 2 (2 cos² (α – β)/2)

= 4 cos² (α – β)/2

= RHS

Hence Proved.

12. sin² (π/8 + x/2) – sin² (π/8 – x/2) = 1/√2 sin x

Solution:

Let us consider LHS:

sin² (π/8 + x/2) – sin² (π/8 – x/2)

we know, sin² A – sin² B = sin (A+B) sin (A-B)

so,

sin² (π/8 + x/2) – sin² (π/8 – x/2) = sin (π/8 + x/2 + π/8 – x/2) sin (π/8 + x/2 – (π/8 – x/2))

= sin (π/8 + π/8) sin (π/8 + x/2 – π/8 + x/2)

= sin π/4 sin x

= 1/√2 sin x [since, since π/4 = 1/√2]

= RHS

Hence proved.

13. 1 + cos² 2x = 2 (cos⁴ x + sin⁴ x)

Solution:

Let us consider LHS:

1 + cos² 2x

We know, cos2x = cos² x – sin² x

cos² x + sin² x = 1

so,

1 + cos² 2x = (cos² x + sin² x) ² + (cos² x – sin² x) ²

= (cos⁴ x + sin⁴ x + 2 cos² x sin² x) + (cos⁴ x + sin⁴ x – 2 cos² x sin² x)

= cos⁴ x + sin⁴ x + cos⁴ x + sin⁴ x

= 2 cos⁴ x + 2 sin⁴ x

= 2 (cos⁴ x + sin⁴ x)

= RHS

Hence proved.

14. cos³ 2x + 3 cos 2x = 4 (cos⁶ x – sin⁶ x)

Solution:

Let us consider RHS:

4 (cos⁶ x – sin⁶ x)

Upon expansion, we get,

4 (cos⁶ x – sin⁶ x) = 4 [(cos² x)³ – (sin² x)³]

= 4 (cos² x – sin² x) (cos⁴ x + sin⁴ x + cos² x sin² x)

By using the formula,

a³ – b³ = (a-b) (a² + b² + ab)

= 4 cos 2x (cos⁴ x + sin⁴ x + cos² x sin² x + cos² x sin² x – cos² x sin

We know, cos 2x = cos² x – sin² x

So,

= 4 cos 2x (cos⁴ x + sin⁴ x + 2 cos² x sin² x – cos² x sin² x)

= 4 cos 2x [(cos² x)² + (sin² x)² + 2 cos² x sin² x – cos² x sin² x]

We know, a² + b² + 2ab = (a + b)²

= 4 cos 2x [(1)² – 1/4 (4 cos² x sin² x)]

= 4 cos 2x [(1)² – 1/4 (2 cos x sin x)²]

We know, sin 2x = 2sin x cos x

= 4 cos 2x [(1²) – 1/4 (sin 2x)²]

= 4 cos 2x (1 – 1/4 sin² 2x)

We know, sin² x = 1 – cos² x

= 4 cos 2x [1 – 1/4 (1 – cos² 2x)]

= 4 cos 2x [1 – 1/4 + 1/4 cos² 2x]

= 4 cos 2x [3/4 + 1/4 cos² 2x]

= 4 (3/4 cos 2x + 1/4 cos³ 2x)

= 3 cos 2x + cos³ 2x

= cos³ 2x + 3 cos 2x

= LHS

Hence proved.

15. (sin 3x + sin x) sin x + (cos 3x – cos x) cos x = 0

Solution:

Let us consider LHS:

(sin 3x + sin x) sin x + (cos 3x – cos x) cos x

= (sin 3x) (sin x) + sin² x + (cos 3x) (cos x) – cos² x

= [(sin 3x) (sin x) + (cos 3x) (cos x)] + (sin² x – cos² x)

= [(sin 3x) (sin x) + (cos 3x) (cos x)] – (cos² x – sin² x)

= cos (3x – x) – cos 2x

We know, cos 2x = cos² x – sin² x

cos A cos B + sin A sin B = cos(A – B)

So,

= cos 2x – cos 2x

= 0

= RHS

Hence Proved.

16. cos² (π/4 – x) – sin² (π/4 – x) = sin 2x

Solution:

Let us consider LHS:

cos² (π/4 – x) – sin² (π/4 – x)

We know, cos² A – sin² A = cos 2A

So,

cos² (π/4 – x) – sin² (π/4 – x) = cos 2 (π/4 – x)

= cos (π/2 – 2x)

= sin 2x [since, cos (π/2 – A) = sin A]

= RHS

Hence proved.

17. cos 4x = 1 – 8 cos² x + 8 cos⁴ x

Solution:

Let us consider LHS:

cos 4x

We know, cos 2x = 2 cos² x – 1

So,

cos 4x = 2 cos² 2x – 1

= 2(2 cos² 2x – 1)² – 1

= 2[(2 cos² 2x) ² + 1² – 2×2 cos² x] – 1

= 2(4 cos⁴ 2x + 1 – 4 cos² x) – 1

= 8 cos⁴ 2x + 2 – 8 cos² x – 1

= 8 cos⁴ 2x + 1 – 8 cos² x

= RHS

Hence Proved.

18. sin 4x = 4 sin x cos³ x – 4 cos x sin³ x

Solution:

Let us consider LHS:

sin 4x

We know, sin 2x = 2 sin x cos x

cos 2x = cos² x – sin² x

So,

sin 4x = 2 sin 2x cos 2x

= 2 (2 sin x cos x) (cos² x – sin² x)

= 4 sin x cos x (cos² x – sin² x)

= 4 sin x cos³ x – 4 sin³ x cos x

= RHS

Hence proved.

19. 3(sin x – cos x) ⁴ + 6 (sin x + cos x) ² + 4 (sin⁶ x + cos⁶ x) = 13

Solution:

Let us consider LHS:

3(sin x – cos x) ⁴ + 6 (sin x + cos x) ² + 4 (sin⁶ x + cos⁶ x)

We know, (a + b)² = a² + b² + 2ab

(a – b)² = a² + b² – 2ab

a³ + b³ = (a + b) (a² + b² – ab)

So,

3(sin x – cos x) ⁴ + 6 (sin x + cos x) ² + 4 (sin⁶ x + cos⁶ x) = 3{(sin x – cos x) ²}² + 6 {(sin x)² + (cos x)² + 2 sin x cos x)} + 4 {(sin² x)³ + (cos² x)³}

= 3{(sin x) ² + (cos x)² – 2 sin x cos x)}² + 6 (sin² x + cos² x + 2 sin x cos x) + 4{(sin² x + cos² x) (sin⁴ x + cos⁴ x – sin² x cos² x)}

= 3(1 – 2 sin x cos x) ² + 6 (1 + 2 sin x cos x) + 4{(1) (sin⁴ x + cos⁴ x – sin² x cos² x)}

We know, sin² x + cos² x = 1

So,

= 3{1² + (2 sin x cos x) ² – 4 sin x cos x} + 6 (1 + 2 sin x cos x) + 4{(sin² x)² + (cos² x)² + 2 sin² x cos² x – 3 sin² x cos² x)}

= 3{1 + 4 sin² x cos² x – 4 sin x cos x} + 6 (1 + 2 sin x cos x) + 4{(sin² x + cos² x) ² – 3 sin² x cos² x)}

= 3 + 12 sin² x cos² x – 12 sin x cos x + 6 + 12 sin x cos x + 4{(1)² – 3 sin² x cos² x)}

= 9 + 12 sin² x cos² x + 4(1 – 3 sin² x cos² x)

= 9 + 12 sin² x cos² x + 4 – 12 sin² x cos² x

= 13

= RHS

Hence proved.

20. 2(sin⁶ x + cos⁶ x) – 3(sin⁴ x + cos⁴ x) + 1 = 0

Solution:

Let us consider LHS:

2(sin⁶ x + cos⁶ x) – 3(sin⁴ x + cos⁴ x) + 1

We know, (a + b)² = a² + b² + 2ab

a³ + b³ = (a + b) (a² + b² – ab)

So,

2(sin⁶ x + cos⁶ x) – 3(sin⁴ x + cos⁴ x) + 1 = 2{(sin² x) ³ + (cos² x) ³} – 3{(sin² x) ² + (cos² x) ²} + 1

= 2{(sin² x + cos² x) (sin⁴ x + cos⁴ x – sin² x cos² x} – 3{(sin² x) ² + (cos² x) ² + 2sin² x cos² x – 2sin² x cos² x} + 1

= 2{(1) (sin⁴ x + cos⁴ x + 2 sin² x cos² x – 3 sin² x cos² x} – 3{(sin² x + cos² x) ² – 2sin² x cos² x} + 1

We know, sin² x + cos² x = 1

= 2{(sin² x + cos² x) ² – 3 sin² x cos² x} – 3{(1)² – 2sin² x cos² x} + 1

= 2{(1)² – 3 sin² x cos² x} – 3(1 – 2sin² x cos² x) + 1

= 2(1 – 3 sin² x cos² x) – 3 + 6 sin² x cos² x + 1

= 2 – 6 sin² x cos² x – 2 + 6 sin² x cos² x

= 0

= RHS

Hence proved.

21. cos⁶ x – sin⁶ x = cos 2x (1 – 1/4 sin² 2x)

Solution:

Let us consider LHS:

cos⁶ x – sin⁶ x

We know, (a + b) ² = a² + b² + 2ab

a³ – b³ = (a – b) (a² + b² + ab)

So,

cos⁶ x – sin⁶ x = (cos² x)³ – (sin² x)³

= (cos² x – sin² x) (cos⁴ x + sin⁴ x + cos² x sin² x)

We know, cos 2x = cos² x – sin² x

So,

= cos 2x [(cos² x) ² + (sin² x) ² + 2 cos² x sin² x – cos² x sin² x]

= cos 2x [(cos² x) ² + (sin² x) ² – 1/4 × 4 cos² x sin² x]

We know, sin² x + cos² x = 1

So,

= cos 2x [(1)² – 1/4 × (2 cos x sin x) ²]

We know, sin 2x = 2 sin x cos x

So,

= cos 2x [1 – 1/4 × (sin 2x) ²]

= cos 2x [1 – 1/4 × sin² 2x]

= RHS

Hence proved.

22. tan (π/4 + x) + tan (π/4 – x) = 2 sec 2x

Solution:

Let us consider LHS:

tan (π/4 + x) + tan (π/4 – x)

We know,

tan (A+B) = (tan A + tan B)/(1- tan A tan B)

tan (A-B) = (tan A – tan B)/(1+ tan A tan B)

So,