RD Sharma Solutions for Class 8 Maths Chapter 8 Division of Algebraic Expressions

Students can refer to RD Sharma Solutions for Class 8 Maths Chapter 8 Division of Algebraic Expressions, which is the best study material for them to excel in the annual exams. Subject experts at BYJU’S have formulated RD Sharma Class 8 Maths Solutions, which include all the questions providing a clear understanding of important concepts by giving detailed explanations for the problems. The PDFs of this chapter are available here, and students can download them for free from the links given below. Chapter 8 – Division of Algebraic Expressions contains six exercises, and the RD Sharma Solutions available on this page provide solutions to the questions present in each exercise. Now, let us have a look at the concepts covered in this chapter.

• Polynomials and degree of a polynomial in two variables.
• Division of a monomial by a monomial.
• Division of polynomials in one variable.
• Division of a polynomial by a monomial.
• Division of a polynomial by a binomial by using the long division method.
• Division of polynomials by using factorization.

RD Sharma Solutions for Class 8 Maths Chapter 8 Division of Algebraic Expressions

Download PDF

carouselExampleControls111

Previous



Next

Access Answers to Maths RD Sharma Solutions for Class 8 Chapter 8 Division of Algebraic Expressions

EXERCISE 8.1 PAGE NO: 8.2

1. Write the degree of each of the following polynomials:

(i) 2x³ + 5x² – 7

(ii) 5x² – 3x + 2

(iii) 2x + x² – 8

(iv) 1/2y⁷ – 12y⁶ + 48y⁵ – 10

(v) 3x³ + 1

(vi) 5

(vii) 20x³ + 12x²y² – 10y² + 20

Solution:

(i) 2x³ + 5x² – 7

We know that in a polynomial, the degree is the highest power of the variable.

The degree of the polynomial, 2x³ + 5x² – 7, is 3.

(ii) 5x² – 3x + 2

The degree of the polynomial, 5x² – 3x + 2, is 2.

(iii) 2x + x² – 8

The degree of the polynomial, 2x + x² – 8, is 2.

(iv) 1/2y⁷ – 12y⁶ + 48y⁵ – 10

The degree of the polynomial, 1/2y⁷ – 12y⁶ + 48y⁵ – 10, is 7.

(v) 3x³ + 1

The degree of the polynomial, 3x³ + 1, is 3

(vi) 5

The degree of the polynomial 5 is 0 (since 5 is a constant number).

(vii) 20x³ + 12x²y² – 10y² + 20

The degree of the polynomial, 20x³ + 12x²y² – 10y² + 20, is 4.

2. Which of the following expressions are not polynomials?

(i) x² + 2x^-2

(ii) √(ax) + x² – x³

(iii) 3y³ – √5y + 9

(iv) ax^1/2 + ax + 9x² + 4

(v) 3x^-3 + 2x^-1 + 4x + 5

Solution:

(i) x² + 2x^-2

The given expression is not a polynomial.

Because a polynomial does not contain any negative powers or fractions.

(ii) √(ax) + x² – x³

The given expression is not a polynomial.

Because the polynomial has 1/2 as the power of variable x, which is not a non-negative integer.

(iii) 3y³ – √5y + 9

The given expression is a polynomial.

Because the polynomial has positive powers.

(iv) ax^1/2 + ax + 9x² + 4

The given expression is not a polynomial.

Because a polynomial does not contain any negative powers or fractions.

(v) 3x^-3 + 2x^-1 + 4x + 5

The given expression is not a polynomial.

Because a polynomial does not contain any negative powers or fractions.

3. Write each of the following polynomials in the standard form. Also, write their degree.

(i) x² + 3 + 6x + 5x⁴

(ii) a² + 4 + 5a⁶

(iii) (x³ – 1) (x³ – 4)

(iv) (y³ – 2) (y³ + 11)

(v) (a³ – 3/8) (a³ + 16/17)

(vi) (a + 3/4) (a + 4/3)

Solution:

(i) x² + 3 + 6x + 5x⁴

The standard form of the polynomial is written in either increasing or decreasing order of their powers.

3 + 6x + x² + 5x⁴ or 5x⁴ + x² + 6x + 3

The degree of the given polynomial is 4.

(ii) a² + 4 + 5a⁶

The standard form of the polynomial is written in either increasing or decreasing order of their powers.

4 + a² + 5a⁶ or 5a⁶ + a² + 4

The degree of the given polynomial is 6.

(iii) (x³ – 1) (x³ – 4)

x⁶ – 4x³ – x³ + 4

x⁶ – 5x³ + 4

The standard form of the polynomial is written in either increasing or decreasing order of their powers.

x⁶ – 5x³ + 4 or 4 – 5x³ + x⁶

The degree of the given polynomial is 6.

(iv) (y³ – 2) (y³ + 11)

y⁶ + 11y³ – 2y³ – 22

y⁶ + 9y³ – 22

The standard form of the polynomial is written in either increasing or decreasing order of their powers.

y⁶ + 9y³ – 22 or -22 + 9y³ + y⁶

The degree of the given polynomial is 6.

(v) (a³ – 3/8) (a³ + 16/17)

a⁶ + 16a³/17 – 3a³/8 – 6/17

a⁶ + 77/136a³ – 48/136

The standard form of the polynomial is written in either increasing or decreasing order of their powers.

a⁶ + 77/136a³ – 48/136 or -48/136 + 77/136a³ + a⁶

The degree of the given polynomial is 6.

(vi) (a + 3/4) (a + 4/3)

a² + 4a/3 + 3a/4 + 1

a² + 25a/12 + 1

The standard form of the polynomial is written in either increasing or decreasing order of their powers.

a² + 25a/12 + 1 or 1 + 25a/12 + a²

The degree of the given polynomial is 2.

EXERCISE 8.2 PAGE NO: 8.4

Divide:

1. 6x³y²z² by 3x²yz

Solution:

We have,

6x³y²z² / 3x²yz

By using the formula aⁿ / a^m = a^n-m

6/3 x^3-2 y^2-1 z^2-1

2xyz

2. 15m²n³ by 5m²n²

Solution:

We have,

15m²n³ / 5m²n²

By using the formula aⁿ / a^m = a^n-m

15/5 m^2-2 n^3-2

3n

3. 24a³b³ by -8ab

Solution:

We have,

24a³b³ / -8ab

By using the formula aⁿ / a^m = a^n-m

24/-8 a^3-1 b^3-1

-3a²b²

4. -21abc² by 7abc

Solution:

We have,

-21abc² / 7abc

By using the formula aⁿ / a^m = a^n-m

-21/7 a^1-1 b^1-1 c^2-1

-3c

5. 72xyz² by -9xz

Solution:

We have,

72xyz² / -9xz

By using the formula aⁿ / a^m = a^n-m

72/-9 x^1-1 y z^2-1

-8yz

6. -72a⁴b⁵c⁸ by -9a²b²c³

Solution:

We have,

-72a⁴b⁵c⁸ / -9a²b²c³

By using the formula aⁿ / a^m = a^n-m

-72/-9 a^4-2 b^5-2 c^8-3

8a²b³c⁵

Simplify:

7. 16m³y² / 4m²y

Solution:

We have,

16m³y² / 4m²y

By using the formula aⁿ / a^m = a^n-m

16/4 m^3-2 y^2-1

4my

8. 32m²n³p² / 4mnp

Solution:

We have,

32m²n³p² / 4mnp

By using the formula aⁿ / a^m = a^n-m

32/4 m^2-1 n^3-1 p^2-1

8mn²p

EXERCISE 8.3 PAGE NO: 8.6

Divide:

1. x + 2x² + 3x⁴ – x⁵ by 2x

Solution:

We have,

(x + 2x² + 3x⁴ – x⁵) / 2x

x/2x + 2x²/2x + 3x⁴/2x – x⁵/2x

By using the formula aⁿ / a^m = a^n-m

1/2 x^1-1 + x^2-1 + 3/2 x^4-1 – 1/2 x^5-1

1/2 + x + 3/2 x³ – 1/2 x⁴

2. y⁴ – 3y³ + 1/2y² by 3y

Solution:

We have,

(y⁴ – 3y³ + 1/2y²)/ 3y

y⁴/3y – 3y³/3y + (½)y²/3y

By using the formula aⁿ / a^m = a^n-m

1/3 y^4-1 – y^3-1 + 1/6 y^2-1

1/3y³ – y² + 1/6y

3. -4a³ + 4a² + a by 2a

Solution:

We have,

(-4a³ + 4a² + a) / 2a

-4a³/2a + 4a²/2a + a/2a

By using the formula aⁿ / a^m = a^n-m

-2a^3-1 + 2a^2-1 + 1/2 a^1-1

-2a² + 2a + ½

4. –x⁶ + 2x⁴ + 4x³ + 2x² by √2x²

Solution:

We have,

(–x⁶ + 2x⁴ + 4x³ + 2x²) / √2x²

-x⁶/√2x² + 2x⁴/√2x² + 4x³/√2x² + 2x²/√2x²

By using the formula aⁿ / a^m = a^n-m

-1/√2 x^6-2 + 2/√2 x^4-2 + 4/√2 x^3-2 + 2/√2 x^2-2

-1/√2 x⁴ + √2x² + 2√2x + √2

5. -4a³ + 4a² + a by 2a

Solution:

We have,

(-4a³ + 4a² + a) / 2a

-4a³/2a + 4a²/2a + a/2a

By using the formula aⁿ / a^m = a^n-m

-2a^3-1 + 2a^2-1 + 1/2a^1-1

-2a² + 2a + ½

6. √3a⁴ + 2√3a³ + 3a² – 6a by 3a

Solution:

We have,

(√3a⁴ + 2√3a³ + 3a² – 6a) / 3a

√3a⁴/3a + 2√3a³/3a + 3a²/3a – 6a/3a

By using the formula aⁿ / a^m = a^n-m

√3/3 a^4-1 + 2√3/3 a^3-1 + a^2-1 – 2a^1-1

1/√3 a³ + 2/√3 a² + a – 2

EXERCISE 8.4 PAGE NO: 8.11

Divide:

1. 5x³ – 15x² + 25x by 5x

Solution:

We have,

(5x³ – 15x² + 25x) / 5x

5x³/5x – 15x²/5x + 25x/5x

By using the formula aⁿ / a^m = a^n-m

5/5 x^3-1 – 15/5 x^2-1 + 25/5 x^1-1

x² – 3x + 5

2. 4z³ + 6z² – z by -1/2z

Solution:

We have,

(4z³ + 6z² – z) / -1/2z

4z³/(-1/2z) + 6z²/(-1/2z) – z/(-1/2z)

By using the formula aⁿ / a^m = a^n-m

-8 z^3-1 – 12z^2-1 + 2 z^1-1

-8z² – 12z + 2

3. 9x²y – 6xy + 12xy² by -3/2xy

Solution:

We have,

(9x²y – 6xy + 12xy²) / -3/2xy

9x²y/(-3/2xy) – 6xy/(-3/2xy) + 12xy²/(-3/2xy)

By using the formula aⁿ / a^m = a^n-m

(-9×2)/3 x^2-1y^1-1 – (-6×2)/3 x^1-1y^1-1 + (-12×2)/3 x^1-1y^2-1

-6x + 4 – 8y

4. 3x³y² + 2x²y + 15xy by 3xy

Solution:

We have,

(3x³y² + 2x²y + 15xy) / 3xy

3x³y²/3xy + 2x²y/3xy + 15xy/3xy

By using the formula aⁿ / a^m = a^n-m

3/3 x^3-1y^2-1 + 2/3 x^2-1y^1-1 + 15/3 x^1-1y^1-1

x²y + 2/3x + 5

5. x² + 7x + 12 by x + 4

Solution:

We have,

(x² + 7x + 12) / (x + 4)

By using the long division method

∴ (x² + 7x + 12) / (x + 4) = x + 3

6. 4y² + 3y + 1/2 by 2y + 1

Solution:

We have,

4y² + 3y + 1/2 by (2y + 1)

By using the long division method

∴ (4y² + 3y + 1/2) / (2y + 1) = 2y + 1/2

7. 3x³ + 4x² + 5x + 18 by x + 2

Solution:

We have,

(3x³ + 4x² + 5x + 18) / (x + 2)

By using the long division method

∴ (3x³ + 4x² + 5x + 18) / (x + 2) = 3x² – 2x + 9

8. 14x² – 53x + 45 by 7x – 9

Solution:

We have,

(14x² – 53x + 45) / (7x – 9)

By using the long division method

∴ (14x² – 53x + 45) / (7x – 9) = 2x – 5

9. -21 + 71x – 31x² – 24x³ by 3 – 8x

Solution:

We have,

-21 + 71x – 31x² – 24x³ by 3 – 8x

(-24x³ – 31x² + 71x – 21) / (3 – 8x)

By using the long division method

∴ (-24x³ – 31x² + 71x – 21) / (3 – 8x) = 3x² + 5x – 7

10. 3y⁴ – 3y³ – 4y² – 4y by y² – 2y

Solution:

We have,

(3y⁴ – 3y³ – 4y² – 4y) / (y² – 2y)

By using the long division method

∴ (3y⁴ – 3y³ – 4y² – 4y) / (y² – 2y) = 3y² + 3y + 2

11. 2y⁵ + 10y⁴ + 6y³ + y² + 5y + 3 by 2y³ + 1

Solution:

We have,

(2y⁵ + 10y⁴ + 6y³ + y² + 5y + 3) / (2y³ + 1)

By using the long division method

∴ (2y⁵ + 10y⁴ + 6y³ + y² + 5y + 3) / (2y³ + 1) = y² + 5y + 3

12. x⁴ – 2x³ + 2x² + x + 4 by x² + x + 1

Solution:

We have,

(x⁴ – 2x³ + 2x² + x + 4) / (x² + x + 1)

By using the long division method

∴ (x⁴ – 2x³ + 2x² + x + 4) / (x² + x + 1) = x² – 3x + 4

13. m³ – 14m² + 37m – 26 by m² – 12m + 13

Solution:

We have,

(m³ – 14m² + 37m – 26) / (m² – 12m + 13)

By using the long division method

∴ (m³ – 14m² + 37m – 26) / (m² – 12m + 13) = m – 2

14. x⁴ + x² + 1 by x² + x + 1

Solution:

We have,

(x⁴ + x² + 1) / (x² + x + 1)

By using the long division method

∴ (x⁴ + x² + 1) / (x² + x + 1) = x² – x + 1

15. x⁵ + x⁴ + x³ + x² + x + 1 by x³ + 1

Solution:

We have,

(x⁵ + x⁴ + x³ + x² + x + 1) / (x³ + 1)

By using the long division method

∴ (x⁵ + x⁴ + x³ + x² + x + 1) / (x³ + 1) = x² + x + 1

Divide each of the following and find the quotient and remainder:

16. 14x³ – 5x² + 9x – 1 by 2x – 1

Solution:

We have,

(14x³ – 5x² + 9x – 1) / (2x – 1)

By using the long division method

∴ Quotient is 7x² + x + 5 and the Remainder is 4.

17. 6x³ – x² – 10x – 3 by 2x – 3

Solution:

We have,

(6x³ – x² – 10x – 3) / (2x – 3)

By using the long division method

∴ Quotient is 3x² + 4x + 1 and the Remainder is 0.

18. 6x³ + 11x² – 39x – 65 by 3x² + 13x + 13

Solution:

We have,

(6x³ + 11x² – 39x – 65) / (3x² + 13x + 13)

By using the long division method

∴ Quotient is 2x – 5 and the Remainder is 0.

19. 30x⁴ + 11x³ – 82x² – 12x + 48 by 3x² + 2x – 4

Solution:

We have,

(30x⁴ + 11x³ – 82x² – 12x + 48) / (3x² + 2x – 4)

By using the long division method

∴ Quotient is 10x² – 3x – 12 and the Remainder is 0.

20. 9x⁴ – 4x² + 4 by 3x² – 4x + 2

Solution:

We have,

(9x⁴ – 4x² + 4) / (3x² – 4x + 2)

By using the long division method

∴ Quotient is 3x² + 4x + 2 and the Remainder is 0.

21. Verify division algorithm i.e. Dividend = Divisor × Quotient + Remainder, in each of the following. Also, write the quotient and remainder:

Dividend divisor

(i) 14x² + 13x – 15 7x – 4

(ii) 15z³ – 20z² + 13z – 12 3z – 6

(iii) 6y⁵ – 28y³ + 3y² + 30y – 9 2x² – 6

(iv) 34x – 22x³ – 12x⁴ – 10x² – 75 3x + 7

(v) 15y⁴ – 16y³ + 9y² – 10/3y + 6 3y – 2

(vi) 4y³ + 8y + 8y² + 7 2y² – y + 1

(vii) 6y⁴ + 4y⁴ + 4y³ + 7y² + 27y + 6 2y³ + 1

Solution:

(i) Dividend divisor

14x² + 13x – 15 7x – 4

By using the long division method

Let us verify, Dividend = Divisor × Quotient + Remainder

14x² + 13x – 15 = (7x – 4) × (2x + 3) + (-3)

= 14x² + 21x – 8x -12 -3

= 14x² + 13x – 15

Hence, verified.

∴ The quotient is 2x + 3 and the Remainder is -3.

(ii) Dividend divisor

15z³ – 20z² + 13z – 12 3z – 6

By using the long division method

Let us verify, Dividend = Divisor × Quotient + Remainder

15z³ – 20z² + 13z – 12 = (3z – 6) × (5z² + 10z/3 + 11) + 54

= 15z³ + 10z² + 33z – 30z² – 20z + 54

= 15z² – 20z² + 13z – 12

Hence, verified.

∴ The quotient is 5z² + 10z/3 + 11 and the Remainder is 54.

(iii) Dividend divisor

6y⁵ – 28y³ + 3y² + 30y – 9 2x² – 6

By using the long division method

Let us verify, Dividend = Divisor × Quotient + Remainder

6y⁵ – 28y³ + 3y² + 30y – 9 = (2x² – 6) × (3y³ – 5y + 3/2) + 0

= 6y⁵ – 10y³ + 3y² – 18y³ + 30y – 9

= 6y⁵ – 28y³ + 3y² + 30y – 9

Hence, verified.

∴ The quotient is 3y³ – 5y + 3/2 and the Remainder is 0.

(iv) Dividend divisor

34x – 22x³ – 12x⁴ – 10x² – 75 3x + 7

-12x⁴ – 22x³ – 10x² + 34x – 75

By using the long division method

Let us verify, Dividend = Divisor × Quotient + Remainder

-12x⁴ – 22x³ – 10x² + 34x – 75 = (3x + 7) × (-4x³ + 2x² – 8x + 30) – 285

= -12x⁴ + 6x³ – 24x² – 28x³ + 14x² + 90x – 56x + 210 -285

= -12x⁴ – 22x³ – 10x² + 34x – 75

Hence, verified.

∴ The quotient is -4x³ + 2x² – 8x + 30 and the Remainder is -285.

(v) Dividend divisor

15y⁴ – 16y³ + 9y² – 10/3y + 6 3y – 2

By using the long division method

Let us verify, Dividend = Divisor × Quotient + Remainder

15y⁴ – 16y³ + 9y² – 10/3y + 6 = (3y – 2) × (5y³ – 2y² + 5y/3) + 6

= 15y⁴ – 6y³ + 5y² – 10y³ + 4y² – 10y/3 + 6

= 15y⁴ – 16y³ + 9y² – 10/3y + 6

Hence, verified.

∴ The quotient is 5y³ – 2y² + 5y/3 and the Remainder is 6.

(vi) Dividend divisor

4y³ + 8y + 8y² + 7 2y² – y + 1

4y³ + 8y² + 8y + 7

By using the long division method

Let us verify, Dividend = Divisor × Quotient + Remainder

4y³ + 8y² + 8y + 7 = (2y² – y + 1) × (2y + 5) + 11y + 2

= 4y³ + 10y² – 2y² – 5y + 2y + 5 + 11y + 2

= 4y³ + 8y² + 8y + 7

Hence, verified.

∴ The quotient is 2y + 5 and the Remainder is 11y + 2.

(vii) Dividend divisor

6y⁵ + 4y⁴ + 4y³ + 7y² + 27y + 6 2y³ + 1

By using the long division method

Let us verify, Dividend = Divisor × Quotient + Remainder

6y⁵ + 4y⁴ + 4y³ + 7y² + 27y + 6 = (2y³ + 1) × (3y² + 2y + 2) + 4y² + 25y + 4

= 6y⁵ + 4y⁴ + 4y³ + 3y² + 2y + 2 + 4y² + 25y + 4

= 6y⁵ + 4y⁴ + 4y³ + 7y² + 27y + 6

Hence, verified.

∴ The quotient is 3y² + 2y + 2 and the Remainder is 4y² + 25y + 4.

22. Divide 15y⁴+ 16y³ + 10/3y – 9y² – 6 by 3y – 2 Write down the coefficients of the terms in the quotient.

Solution:

We have,

(15y⁴+ 16y³ + 10/3y – 9y² – 6) / (3y – 2)

By using the long division method

∴ The quotient is 5y³ + 26y²/3 + 25y/9 + 80/27

So the coefficients of the terms in the quotient are:

Coefficient of y³ = 5

Coefficient of y² = 26/3

Coefficient of y = 25/9

Constant term = 80/27

23. Using the division of polynomials, state whether
(i) x + 6 is a factor of x² – x – 42
(ii) 4x – 1 is a factor of 4x² – 13x – 12
(iii) 2y – 5 is a factor of 4y⁴ – 10y³ – 10y² + 30y – 15
(iv) 3y² + 5 is a factor of 6y⁵ + 15y⁴ + 16y³ + 4y² + 10y – 35
(v) z² + 3 is a factor of z⁵ – 9z
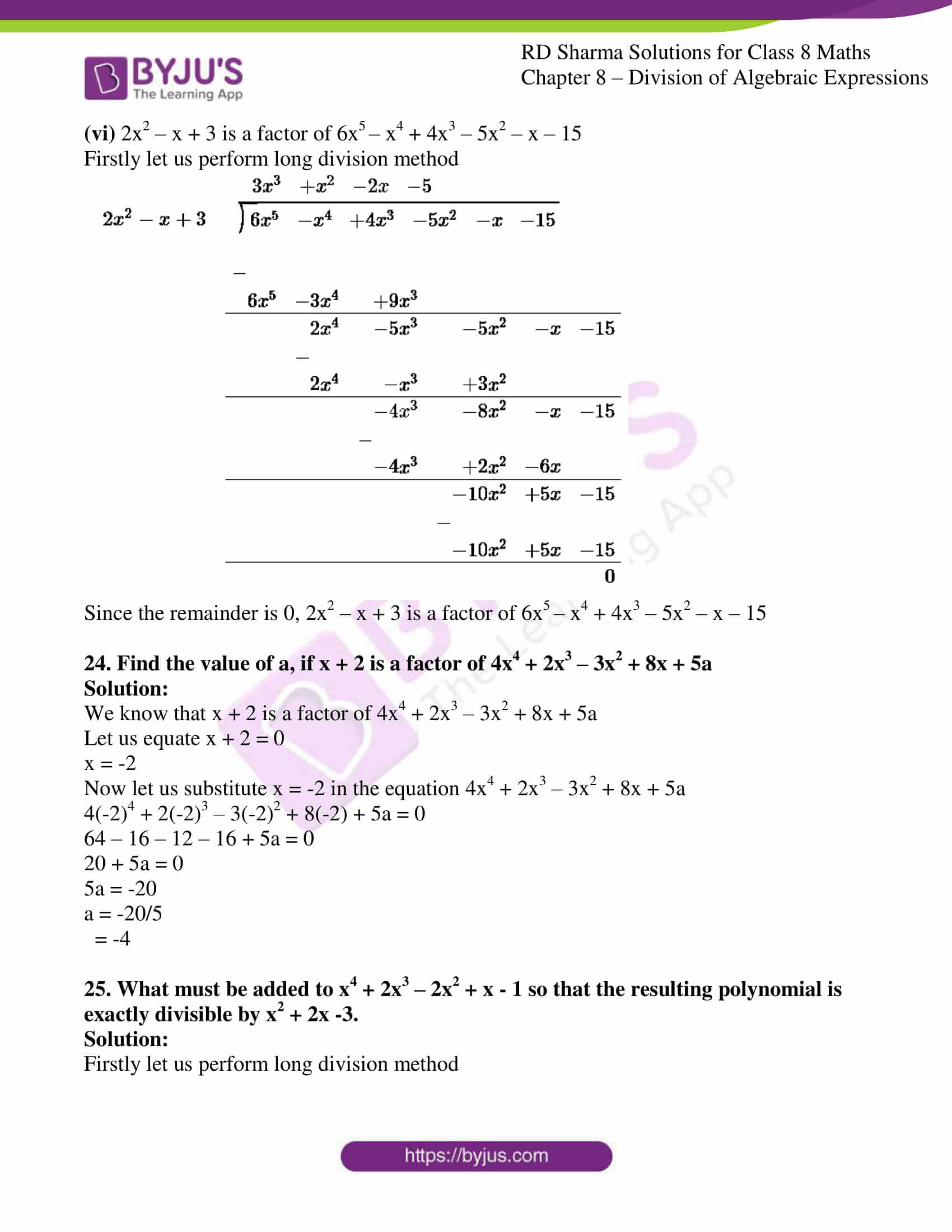
(vi) 2x² – x + 3 is a factor of 6x⁵ – x⁴ + 4x³ – 5x² – x – 15

Solution:

(i) x + 6 is a factor of x² – x – 42

Firstly let us perform long division method

Since the remainder is 0, we can say that x + 6 is a factor of x² – x – 42

(ii) 4x – 1 is a factor of 4x² – 13x – 12

Firstly let us perform long division method

Since the remainder is -15, 4x – 1 is not a factor of 4x² – 13x – 12

(iii) 2y – 5 is a factor of 4y⁴ – 10y³ – 10y² + 30y – 15

Firstly let us perform long division method

Since the remainder is 5y³ – 45y²/2 + 30y – 15, 2y – 5 is not a factor of 4y⁴ – 10y³ – 10y² + 30y – 15

(iv) 3y² + 5 is a factor of 6y⁵ + 15y⁴ + 16y³ + 4y² + 10y – 35 

Firstly let us perform long division method

Since the remainder is 0, 3y² + 5 is a factor of 6y⁵ + 15y⁴ + 16y³ + 4y² + 10y – 35 

(v) z² + 3 is a factor of z⁵ – 9z

Firstly let us perform long division method

Since the remainder is 0, z² + 3 is a factor of z⁵ – 9z

(vi) 2x² – x + 3 is a factor of 6x⁵ – x⁴ + 4x³ – 5x² – x – 15

Firstly let us perform long division method

Since the remainder is 0, 2x² – x + 3 is a factor of 6x⁵ – x⁴ + 4x³ – 5x² – x – 15

24. Find the value of a, if x + 2 is a factor of 4x⁴ + 2x³ – 3x² + 8x + 5a

Solution:

We know that x + 2 is a factor of 4x⁴ + 2x³ – 3x² + 8x + 5a

Let us equate x + 2 = 0

x = -2

Now let us substitute x = -2 in the equation 4x⁴ + 2x³ – 3x² + 8x + 5a

4(-2)⁴ + 2(-2)³ – 3(-2)² + 8(-2) + 5a = 0

64 – 16 – 12 – 16 + 5a = 0

20 + 5a = 0

5a = -20

a = -20/5

= -4

25. What must be added to x⁴ + 2x³ – 2x² + x – 1 so that the resulting polynomial is exactly divisible by x² + 2x -3.

Solution:

Firstly let us perform long division method

By long division method, we got the remainder as –x + 2,

∴ x – 2 has to be added to x⁴ + 2x³ – 2x² + x – 1 so that the resulting polynomial is exactly divisible by x² + 2x -3.

EXERCISE 8.5 PAGE NO: 8.15

1. Divide the first polynomial by the second polynomial in each of the following. Also, write the quotient and remainder:

(i) 3x² + 4x + 5, x – 2

(ii) 10x² – 7x + 8, 5x – 3

(iii) 5y³ – 6y² + 6y – 1, 5y – 1

(iv) x⁴ – x³ + 5x, x – 1

(v) y⁴ + y², y² – 2

Solution:

(i) 3x² + 4x + 5, x – 2

By using the long division method

∴ the Quotient is 3x + 10 and the Remainder is 25.

(ii) 10x² – 7x + 8, 5x – 3

By using the long division method

∴ the Quotient is 2x – 1/5 and the Remainder is 37/5.

(iii) 5y³ – 6y² + 6y – 1, 5y – 1

By using the long division method

∴ the Quotient is y² – y + 1 and the Remainder is 0.

(iv) x⁴ – x³ + 5x, x – 1

By using the long division method

∴ the Quotient is x³ + 5 and the Remainder is 5.

(v) y⁴ + y², y² – 2

By using the long division method

∴ the Quotient is y² + 3 and the Remainder is 6.

2. Find Whether or not the first polynomial is a factor of the second:

(i) x + 1, 2x² + 5x + 4

(ii) y – 2, 3y³ + 5y² + 5y + 2

(iii) 4x² – 5, 4x⁴ + 7x² + 15

(iv) 4 – z, 3z² – 13z + 4

(v) 2a – 3, 10a² – 9a – 5

(vi) 4y + 1, 8y² – 2y + 1

Solution:

(i) x + 1, 2x² + 5x + 4

Let us perform long division method,

Since the remainder is 1, the first polynomial is not a factor of the second polynomial.

(ii) y – 2, 3y³ + 5y² + 5y + 2

Let us perform long division method,

Since the remainder is 56, the first polynomial is not a factor of the second polynomial.

(iii) 4x² – 5, 4x⁴ + 7x² + 15

Let us perform long division method,

Since the remainder is 30, the first polynomial is not a factor of the second polynomial.

(iv) 4 – z, 3z² – 13z + 4

Let us perform long division method,

Since the remainder is 0, the first polynomial is a factor of the second polynomial.

(v) 2a – 3, 10a² – 9a – 5

Let us perform long division method,

Since the remainder is 4, the first polynomial is not a factor of the second polynomial.

(vi) 4y + 1, 8y² – 2y + 1

Let us perform long division method,

Since the remainder is 2, the first polynomial is not a factor of the second polynomial.

EXERCISE 8.6 PAGE NO: 8.17

Divide:

1. x² – 5x + 6 by x – 3

Solution:

We have,

(x² – 5x + 6) / (x – 3)

Let us perform long division method,

∴ the Quotient is x – 2

2. ax² – ay² by ax+ay

Solution:

We have,

(ax² – ay²)/ (ax+ay)

(ax² – ay²)/ (ax+ay) = (x – y) + 0/(ax+ay)

= (x – y)

∴ the answer is (x – y)

3. x⁴ – y⁴ by x² – y²

Solution:

We have,

(x⁴ – y⁴)/ (x² – y²)

(x⁴ – y⁴)/ (x² – y²) = x² + y² + 0/(x² – y²)

= x² + y²

∴ the answer is (x² + y²)

4. acx² + (bc + ad)x + bd by (ax + b)

Solution:

We have,

(acx² + (bc + ad) x + bd) / (ax + b)

(acx² + (bc + ad) x + bd) / (ax + b) = cx + d + 0/ (ax + b)

= cx + d

∴ the answer is (cx + d)

5. (a² + 2ab + b²) – (a² + 2ac + c²) by 2a + b + c

Solution:

We have,

= b – c

∴ the answer is (b – c)

6. 1/4x² – 1/2x – 12 by 1/2x – 4

Solution:

We have,

(1/4x² – 1/2x – 12) / (1/2x – 4)

Let us perform long division method,

∴ the Quotient is x/2 + 3