RD Sharma Solutions for Class 8 Maths Chapter 8 Division of Algebraic Expressions Exercise 8.4

Division of a polynomial by a binomial by using the long division method is the concept explained in Exercise 8.4 of RD Sharma Solutions for Class 8 Chapter 8 Division of Algebraic Expressions. Solutions are derived in a step-by-step format as the concept is based on the long division method. Our expert faculty have developed the solutions in order to help students focus on their weaker areas. Through regular practice, students can solve problems easily without any confusion. Also, they can download the RD Sharma Solutions for Class 8 Maths Exercise 8.4 in PDF from the links provided below.

RD Sharma Solutions for Class 8 Maths Exercise 8.4 Chapter 8 Division of Algebraic Expressions

Download PDF

carouselExampleControls111

Previous



Next

Access Answers to RD Sharma Solutions for Class 8 Maths Exercise 8.4 Chapter 8 Division of Algebraic Expressions

EXERCISE 8.4 PAGE NO: 8.11

Divide:

1. 5x³ – 15x² + 25x by 5x

Solution:

We have,

(5x³ – 15x² + 25x) / 5x

5x³/5x – 15x²/5x + 25x/5x

By using the formula aⁿ / a^m = a^n-m

5/5 x^3-1 – 15/5 x^2-1 + 25/5 x^1-1

x² – 3x + 5

2. 4z³ + 6z² – z by -1/2z

Solution:

We have,

(4z³ + 6z² – z) / -1/2z

4z³/(-1/2z) + 6z²/(-1/2z) – z/(-1/2z)

By using the formula aⁿ / a^m = a^n-m

-8 z^3-1 – 12z^2-1 + 2 z^1-1

-8z² – 12z + 2

3. 9x²y – 6xy + 12xy² by -3/2xy

Solution:

We have,

(9x²y – 6xy + 12xy²) / -3/2xy

9x²y/(-3/2xy) – 6xy/(-3/2xy) + 12xy²/(-3/2xy)

By using the formula aⁿ / a^m = a^n-m

(-9×2)/3 x^2-1y^1-1 – (-6×2)/3 x^1-1y^1-1 + (-12×2)/3 x^1-1y^2-1

-6x + 4 – 8y

4. 3x³y² + 2x²y + 15xy by 3xy

Solution:

We have,

(3x³y² + 2x²y + 15xy) / 3xy

3x³y²/3xy + 2x²y/3xy + 15xy/3xy

By using the formula aⁿ / a^m = a^n-m

3/3 x^3-1y^2-1 + 2/3 x^2-1y^1-1 + 15/3 x^1-1y^1-1

x²y + 2/3x + 5

5. x² + 7x + 12 by x + 4

Solution:

We have,

(x² + 7x + 12) / (x + 4)

By using the long division method

∴ (x² + 7x + 12) / (x + 4) = x + 3

6. 4y² + 3y + 1/2 by 2y + 1

Solution:

We have,

4y² + 3y + 1/2 by (2y + 1)

By using the long division method

∴ (4y² + 3y + 1/2) / (2y + 1) = 2y + 1/2

7. 3x³ + 4x² + 5x + 18 by x + 2

Solution:

We have,

(3x³ + 4x² + 5x + 18) / (x + 2)

By using the long division method

∴ (3x³ + 4x² + 5x + 18) / (x + 2) = 3x² – 2x + 9

8. 14x² – 53x + 45 by 7x – 9

Solution:

We have,

(14x² – 53x + 45) / (7x – 9)

By using the long division method

∴ (14x² – 53x + 45) / (7x – 9) = 2x – 5

9. -21 + 71x – 31x² – 24x³ by 3 – 8x

Solution:

We have,

-21 + 71x – 31x² – 24x³ by 3 – 8x

(-24x³ – 31x² + 71x – 21) / (3 – 8x)

By using the long division method

∴ (-24x³ – 31x² + 71x – 21) / (3 – 8x) = 3x² + 5x – 7

10. 3y⁴ – 3y³ – 4y² – 4y by y² – 2y

Solution:

We have,

(3y⁴ – 3y³ – 4y² – 4y) / (y² – 2y)

By using the long division method

∴ (3y⁴ – 3y³ – 4y² – 4y) / (y² – 2y) = 3y² + 3y + 2

11. 2y⁵ + 10y⁴ + 6y³ + y² + 5y + 3 by 2y³ + 1

Solution:

We have,

(2y⁵ + 10y⁴ + 6y³ + y² + 5y + 3) / (2y³ + 1)

By using the long division method

∴ (2y⁵ + 10y⁴ + 6y³ + y² + 5y + 3) / (2y³ + 1) = y² + 5y + 3

12. x⁴ – 2x³ + 2x² + x + 4 by x² + x + 1

Solution:

We have,

(x⁴ – 2x³ + 2x² + x + 4) / (x² + x + 1)

By using the long division method

∴ (x⁴ – 2x³ + 2x² + x + 4) / (x² + x + 1) = x² – 3x + 4

13. m³ – 14m² + 37m – 26 by m² – 12m + 13

Solution:

We have,

(m³ – 14m² + 37m – 26) / (m² – 12m + 13)

By using the long division method

∴ (m³ – 14m² + 37m – 26) / (m² – 12m + 13) = m – 2

14. x⁴ + x² + 1 by x² + x + 1

Solution:

We have,

(x⁴ + x² + 1) / (x² + x + 1)

By using the long division method

∴ (x⁴ + x² + 1) / (x² + x + 1) = x² – x + 1

15. x⁵ + x⁴ + x³ + x² + x + 1 by x³ + 1

Solution:

We have,

(x⁵ + x⁴ + x³ + x² + x + 1) / (x³ + 1)

By using the long division method

∴ (x⁵ + x⁴ + x³ + x² + x + 1) / (x³ + 1) = x² + x + 1

Divide each of the following and find the quotient and remainder:

16. 14x³ – 5x² + 9x – 1 by 2x – 1

Solution:

We have,

(14x³ – 5x² + 9x – 1) / (2x – 1)

By using the long division method

∴ Quotient is 7x² + x + 5, and the remainder is 4.

17. 6x³ – x² – 10x – 3 by 2x – 3

Solution:

We have,

(6x³ – x² – 10x – 3) / (2x – 3)

By using the long division method

∴ Quotient is 3x² + 4x + 1, and the remainder is 0.

18. 6x³ + 11x² – 39x – 65 by 3x² + 13x + 13

Solution:

We have,

(6x³ + 11x² – 39x – 65) / (3x² + 13x + 13)

By using the long division method

∴ Quotient is 2x – 5, and the remainder is 0.

19. 30x⁴ + 11x³ – 82x² – 12x + 48 by 3x² + 2x – 4

Solution:

We have,

(30x⁴ + 11x³ – 82x² – 12x + 48) / (3x² + 2x – 4)

By using the long division method

∴ Quotient is 10x² – 3x – 12, and the remainder is 0.

20. 9x⁴ – 4x² + 4 by 3x² – 4x + 2

Solution:

We have,

(9x⁴ – 4x² + 4) / (3x² – 4x + 2)

By using the long division method

∴ Quotient is 3x² + 4x + 2, and the remainder is 0.

21. Verify division algorithm i.e. Dividend = Divisor × Quotient + Remainder, in each of the following. Also, write the quotient and remainder:

Dividend divisor

(i) 14x² + 13x – 15 7x – 4

(ii) 15z³ – 20z² + 13z – 12 3z – 6

(iii) 6y⁵ – 28y³ + 3y² + 30y – 9 2x² – 6

(iv) 34x – 22x³ – 12x⁴ – 10x² – 75 3x + 7

(v) 15y⁴ – 16y³ + 9y² – 10/3y + 6 3y – 2

(vi) 4y³ + 8y + 8y² + 7 2y² – y + 1

(vii) 6y⁴ + 4y⁴ + 4y³ + 7y² + 27y + 6 2y³ + 1

Solution:

(i) Dividend divisor

14x² + 13x – 15 7x – 4

By using the long division method

Let us verify, Dividend = Divisor × Quotient + Remainder

14x² + 13x – 15 = (7x – 4) × (2x + 3) + (-3)

= 14x² + 21x – 8x -12 -3

= 14x² + 13x – 15

Hence, verified.

∴ The quotient is 2x + 3, and the remainder is -3.

(ii) Dividend divisor

15z³ – 20z² + 13z – 12 3z – 6

By using the long division method

Let us verify, Dividend = Divisor × Quotient + Remainder

15z³ – 20z² + 13z – 12 = (3z – 6) × (5z² + 10z/3 + 11) + 54

= 15z³ + 10z² + 33z – 30z² – 20z + 54

= 15z² – 20z² + 13z – 12

Hence, verified.

∴ The quotient is 5z² + 10z/3 + 11, and the remainder is 54.

(iii) Dividend divisor

6y⁵ – 28y³ + 3y² + 30y – 9 2x² – 6

By using the long division method

Let us verify, Dividend = Divisor × Quotient + Remainder

6y⁵ – 28y³ + 3y² + 30y – 9 = (2x² – 6) × (3y³ – 5y + 3/2) + 0

= 6y⁵ – 10y³ + 3y² – 18y³ + 30y – 9

= 6y⁵ – 28y³ + 3y² + 30y – 9

Hence, verified.

∴ The quotient is 3y³ – 5y + 3/2, and the remainder is 0.

(iv) Dividend divisor

34x – 22x³ – 12x⁴ – 10x² – 75 3x + 7

-12x⁴ – 22x³ – 10x² + 34x – 75

By using the long division method

Let us verify, Dividend = Divisor × Quotient + Remainder

-12x⁴ – 22x³ – 10x² + 34x – 75 = (3x + 7) × (-4x³ + 2x² – 8x + 30) – 285

= -12x⁴ + 6x³ – 24x² – 28x³ + 14x² + 90x – 56x + 210 -285

= -12x⁴ – 22x³ – 10x² + 34x – 75

Hence, verified.

∴ The quotient is -4x³ + 2x² – 8x + 30, and the remainder is -285.

(v) Dividend divisor

15y⁴ – 16y³ + 9y² – 10/3y + 6 3y – 2

By using the long division method

Let us verify, Dividend = Divisor × Quotient + Remainder

15y⁴ – 16y³ + 9y² – 10/3y + 6 = (3y – 2) × (5y³ – 2y² + 5y/3) + 6

= 15y⁴ – 6y³ + 5y² – 10y³ + 4y² – 10y/3 + 6

= 15y⁴ – 16y³ + 9y² – 10/3y + 6

Hence, verified.

∴ The quotient is 5y³ – 2y² + 5y/3, and the remainder is 6.

(vi) Dividend divisor

4y³ + 8y + 8y² + 7 2y² – y + 1

4y³ + 8y² + 8y + 7

By using the long division method

Let us verify, Dividend = Divisor × Quotient + Remainder

4y³ + 8y² + 8y + 7 = (2y² – y + 1) × (2y + 5) + 11y + 2

= 4y³ + 10y² – 2y² – 5y + 2y + 5 + 11y + 2

= 4y³ + 8y² + 8y + 7

Hence, verified.

∴ The quotient is 2y + 5, and the remainder is 11y + 2.

(vii) Dividend divisor

6y⁵ + 4y⁴ + 4y³ + 7y² + 27y + 6 2y³ + 1

By using the long division method

Let us verify, Dividend = Divisor × Quotient + Remainder

6y⁵ + 4y⁴ + 4y³ + 7y² + 27y + 6 = (2y³ + 1) × (3y² + 2y + 2) + 4y² + 25y + 4

= 6y⁵ + 4y⁴ + 4y³ + 3y² + 2y + 2 + 4y² + 25y + 4

= 6y⁵ + 4y⁴ + 4y³ + 7y² + 27y + 6

Hence, verified.

∴ Quotient is 3y² + 2y + 2, and the remainder is 4y² + 25y + 4.

22. Divide 15y⁴+ 16y³ + 10/3y – 9y² – 6 by 3y – 2 Write down the coefficients of the terms in the quotient.

Solution:

We have,

(15y⁴+ 16y³ + 10/3y – 9y² – 6) / (3y – 2)

By using the long division method

∴ The quotient is 5y³ + 26y²/3 + 25y/9 + 80/27

So, the coefficients of the terms in the quotient are:

Coefficient of y³ = 5

Coefficient of y² = 26/3

Coefficient of y = 25/9

Constant term = 80/27

23. Using the division of polynomials, state whether
(i) x + 6 is a factor of x² – x – 42
(ii) 4x – 1 is a factor of 4x² – 13x – 12
(iii) 2y – 5 is a factor of 4y⁴ – 10y³ – 10y² + 30y – 15
(iv) 3y² + 5 is a factor of 6y⁵ + 15y⁴ + 16y³ + 4y² + 10y – 35
(v) z² + 3 is a factor of z⁵ – 9z
(vi) 2x² – x + 3 is a factor of 6x⁵ – x⁴ + 4x³ – 5x² – x – 15

Solution:

(i) x + 6 is a factor of x² – x – 42

Firstly let us perform long division method

Since the remainder is 0, we can say that x + 6 is a factor of x² – x – 42

(ii) 4x – 1 is a factor of 4x² – 13x – 12

Firstly let us perform long division method

Since the remainder is -15, 4x – 1 is not a factor of 4x² – 13x – 12

(iii) 2y – 5 is a factor of 4y⁴ – 10y³ – 10y² + 30y – 15

Firstly let us perform long division method

Since the remainder is 5y³ – 45y²/2 + 30y – 15, 2y – 5 is not a factor of 4y⁴ – 10y³ – 10y² + 30y – 15

(iv) 3y² + 5 is a factor of 6y⁵ + 15y⁴ + 16y³ + 4y² + 10y – 35 

Firstly let us perform long division method

Since the remainder is 0, 3y² + 5 is a factor of 6y⁵ + 15y⁴ + 16y³ + 4y² + 10y – 35 

(v) z² + 3 is a factor of z⁵ – 9z

Firstly let us perform long division method

Since the remainder is 0, z² + 3 is a factor of z⁵ – 9z

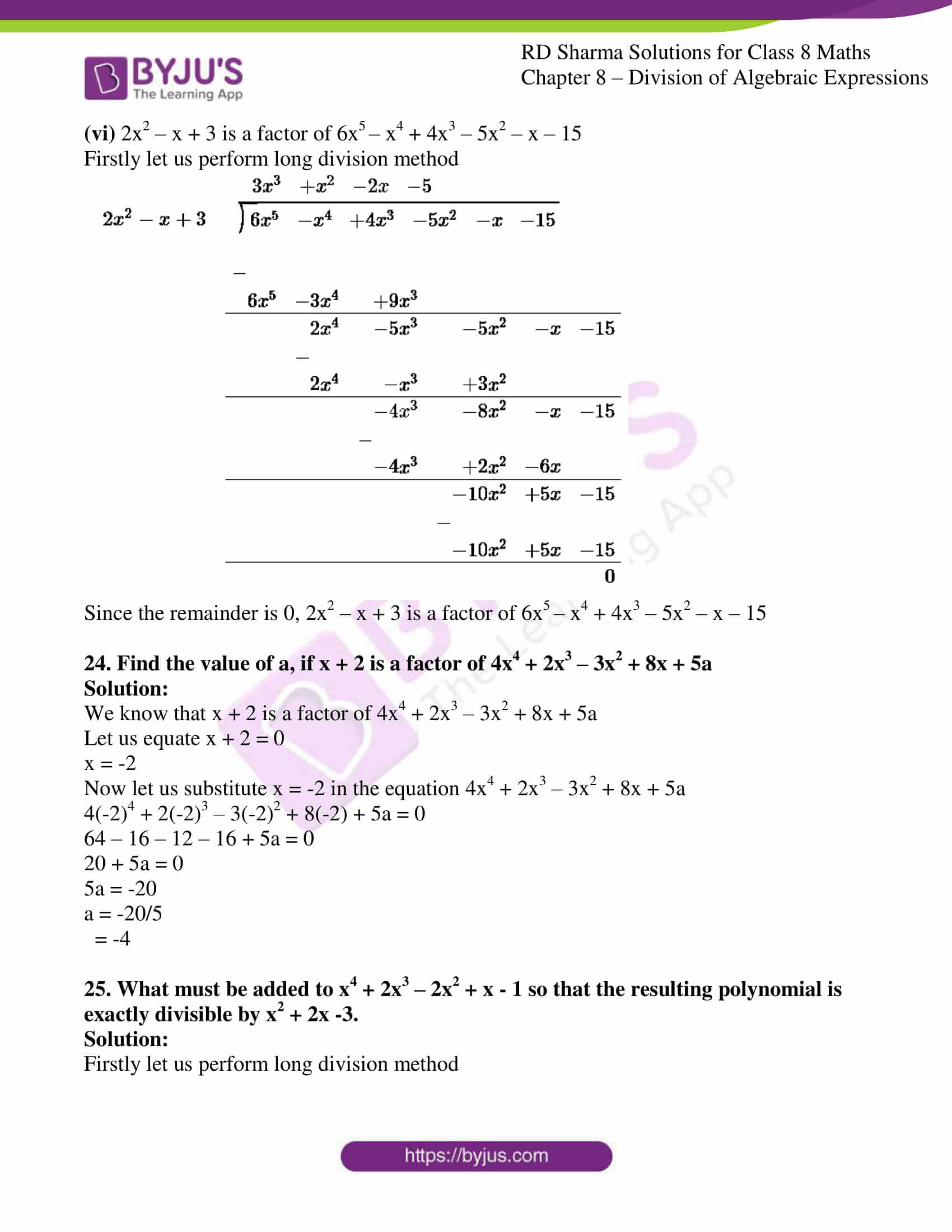
(vi) 2x² – x + 3 is a factor of 6x⁵ – x⁴ + 4x³ – 5x² – x – 15

Firstly let us perform long division method

Since the remainder is 0, 2x² – x + 3 is a factor of 6x⁵ – x⁴ + 4x³ – 5x² – x – 15

24. Find the value of a, if x + 2 is a factor of 4x⁴ + 2x³ – 3x² + 8x + 5a

Solution:

We know that x + 2 is a factor of 4x⁴ + 2x³ – 3x² + 8x + 5a

Let us equate x + 2 = 0

x = -2

Now let us substitute x = -2 in the equation 4x⁴ + 2x³ – 3x² + 8x + 5a

4(-2)⁴ + 2(-2)³ – 3(-2)² + 8(-2) + 5a = 0

64 – 16 – 12 – 16 + 5a = 0

20 + 5a = 0

5a = -20

a = -20/5

= -4

25. What must be added to x⁴ + 2x³ – 2x² + x – 1 so that the resulting polynomial is exactly divisible by x² + 2x -3.

Solution:

Firstly, let us perform long division method

By long division method, we got the remainder as –x + 2,

∴ x – 2 has to be added to x⁴ + 2x³ – 2x² + x – 1 so that the resulting polynomial is exactly divisible by x² + 2x -3.